积分变换习题解答
复变函数与积分变换第一章习题解答
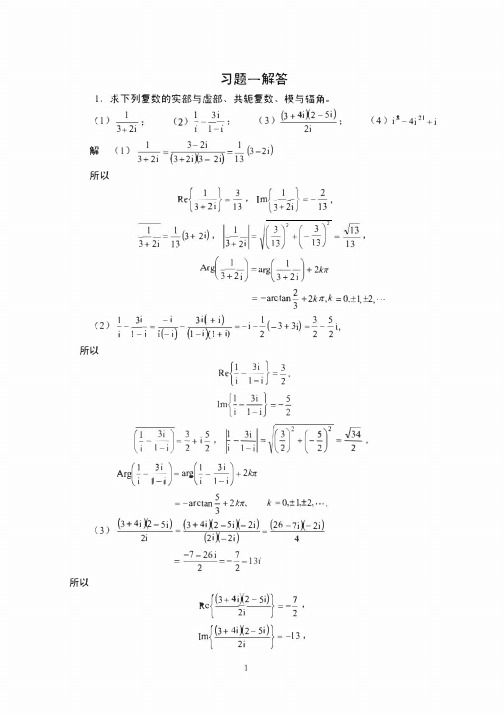
。
n
2) R(
3) 事实上
罕 P(z) =X+iY=X- i Y; 可 = 霄芦 (因)
P(z)
立 +a,, P( 司=a。了"+a1 产+···+a,
4
l 3. 如果 z =e;r, 试证明
1 (1) z" +— = 2cosnt ; n z
II
·+anz n = 页 =a +a1 z+a产 +··
习题 一 解答
1. 求下列复数的实部与虚部 、 共辄复数 、 模与辐角 。
(l)
解 所以
(1)
3+2i
1
(2)
-:--—
1
3+2i
1
3�2i
言, 叫卢}飞, 2 =�(言) +(-卢『 = =卢
(3+2i), ImL : 2J
=-
1 =—(32i) (3+2iX3-2i) 13
3-2i
1
1- 1
3i
(2)
(1+i)6;
J�(2e一气 �32e一l坛"
( 3) 划 ;
1 一3
l I 、 i
=32[cos(-子 )+isin(-子)]=-16"3-16i
(2)
(I+i)'= [ �(i+i )J
=(高冗/4)6 = 8e31ri12 = -8i。 .J3
2
) 4 (
(3)
卢= (ei1t+2k于= eirr (2k+l)/6,k = 0,1,2,3,4,5 。
积分变换习题解答1-2

1-21.求矩形脉冲函数,0()0,A t f t τ⎧≤≤⎪=⎨⎪⎩其他的Fourier 变换.解:[]()j j j j 01e e()()()e d e d 0j j t t t t A F f t f t t A t A τωωωωτωωω-----+∞⎡⎤=====⎢⎥-∞-⎣⎦⎰⎰F 2.设()F ω是函数()f t 的Fourier 变换,证明()F ω与()f t 有相同的奇偶性.证明:()F ω与()f t 是一个Fourier 变换对,即 ()()j e d t F f t t ωω-+∞=-∞⎰,()()j 1e d 2πt f t F ωωω+∞=-∞⎰ 如果()F ω为奇函数,即()()F F ωω-=-,则()()()()()()j j 11e d e d 2π2πt tf t F F ωωωωωω--+∞+∞-==---∞-∞⎰⎰ (令u ω-=)()j 1e d 2πut F u u -∞=+∞⎰(换积分变量u 为ω)()()j 1e d 2πtF f t ωωω+∞=-=--∞⎰ 所以()f t 亦为奇函数.如果()f t 为奇函数,即()()f t f t -=-,则()()()()()j j e d e d t t F f t t f t t ωωω----+∞+∞-==---∞-∞⎰⎰ (令t u -=)()j e d u f u u ω--∞=+∞⎰(换积分变量u 为t )()()j e d t f t t F ωω-+∞=-=--∞⎰所以()F ω亦为奇函数.同理可证()f t 与()F ω同为偶函数.4.求函数()()e 0t f t t -=≥的Fourier 正弦变换,并推证()20012sin πd e αωαωωαω+∞-=>+⎰解:由Fourier 正弦变换公式,有()()s s F f t ω⎡⎤=⎣⎦F ()0sin f t t t ω+∞=⎰d 0sin tt t ω+∞-=⎰e d ()2sin cos 10t t t ωωωω---+∞=+e 21ωω=+ 由Fourier 正弦逆变换公式,有()120022sin ()()sin 1s s s t f t F F t ωωωωωωωω+∞+∞-===⎡⎤⎣⎦+⎰⎰F d d ππ 由此,当0t α=>时,可得()()2sin ππd e 0122f αωαωωααω+∞-==>+⎰5.设()()f t F ω⎡⎤=⎣⎦F ,试证明:1)()f t 为实值函数的充要条件是()()F F ωω-=; 2)()f t 为虚值函数的充要条件是()()F F ωω-=-.证明: 在一般情况下,记()()()r i f t f t f t =+j 其中()r f t 和()i f t 均为t 的实值函数,且分别为()f t 的实部与虚部. 因此()()()()[]j e d j cos jsin d t r i F f t t f t f t t t t ωωωω-+∞+∞⎡⎤==+-⎣⎦-∞-∞⎰⎰ ()()()()cos sin d j sin cos d ri r i f t t f t t t f t t f t t t ωωωω+∞+∞⎡⎤⎡⎤=+--⎣⎦⎣⎦-∞-∞⎰⎰ ()()Re Im F j F ωω⎡⎤⎡⎤=+⎣⎦⎣⎦其中()()()Re cos sin d r i F f t t f t t t ωωω+∞⎡⎤⎡⎤=+⎣⎦⎣⎦-∞⎰, ()a ()()()Im sin cos d ri F f t t f t t t ωωω+∞⎡⎤⎡⎤=--⎣⎦⎣⎦-∞⎰()b 1)若()f t 为t 的实值函数,即()()(),0r i f t t f f t ==.此时,()a 式和()b 式分别为()()Re cos d rF f t t t ωω+∞⎡⎤=⎣⎦-∞⎰()()Im sin d rF f t t t ωω+∞⎡⎤=-⎣⎦-∞⎰所以()()()Re jIm F F F ωωω⎡⎤⎡⎤-=-+-⎣⎦⎣⎦()()()Re jIm F F F ωωω⎡⎤⎡⎤=-=⎣⎦⎣⎦反之,若已知()()F F ωω-=,则有()()()()Re jIm Re jIm F F F F ωωωω⎡⎤⎡⎤⎡⎤⎡⎤-+-=-⎣⎦⎣⎦⎣⎦⎣⎦此即表明()F ω的实部是关于ω的偶函数;()F ω的虚部是关于ω的奇函数.因此,必定有()()()cos d j sin d r rF f t t t f t t t ωωω+∞+∞=--∞-∞⎰⎰ 亦即表明()()r f t f t =为t 的实值函数.从而结论1)获证.2)若()f t 为t 的虚值函数,即()()()j ,0i r f t f f t t ==.此时,()a 式和()b 式分别为()()Re sin d i F f t t t ωω+∞⎡⎤=⎣⎦-∞⎰ ()()Im cos d i F f t t t ωω+∞⎡⎤=⎣⎦-∞⎰所以()()()Re jIm F F F ωωω⎡⎤⎡⎤-=-+-⎣⎦⎣⎦()()Re jIm F F ωω⎡⎤⎡⎤=-+⎣⎦⎣⎦()(){}Re jIm F F ωω⎡⎤⎡⎤=--⎣⎦⎣⎦()F ω=-反之,若已知()()F F ωω-=-,则有()()()()Re jIm Re jIm F F F F ωωωω⎡⎤⎡⎤⎡⎤⎡⎤-+-=-+⎣⎦⎣⎦⎣⎦⎣⎦此即表明()F ω的实部是关于ω的奇函数;()F ω的虚部是关于ω的偶函数.因此,必定有()()()sin d j cos d i iF f t t t f t t t ωωω+∞+∞==+-∞-∞⎰⎰, 亦即表明()()j i f t f t =为t 的虚值函数.从而结论2)获证.6.已知某函数的Fourier 变换sin ()F ωωω=,求该函数()f t .解:sin ()F ωωω=为连续的偶函数,由公式有()()j π1sin e d cos d 2π0tf t F t ωωωωωωω+∞+∞==-∞⎰⎰ ()()sin 1sin 111d d 2π02π0t t ωωωωωω+∞++∞-=+⎰⎰ 但由于当0a >时sin sin sin πd d()d 0002a a t a t t ωωωωωω+∞+∞+∞===⎰⎰⎰ 当0a <时sin sin()πd d 002a a ωωωωωω+∞+∞-=-=-⎰⎰当0a =时,sin d 0,0a ωωω+∞=⎰所以得 ()11211401t f t t t ⎧<⎪⎪⎪==⎨⎪⎪>⎪⎩,,,7.已知某函数的Fourier 变换为()()()00πδδF ωωωωω⎡⎤=++-⎣⎦,求该函数()f t .解:由函数()()()00δd t t g t t g t -=,易知()()()()j j j 001e d 2π11πδe d πδe d 2π2πt t t f t F ωωωωωωωωωωω+∞=-∞+∞+∞=++--∞-∞⎰⎰⎰j j 00011e e cos 22t t t ωωωωωωω=-==+=8.求符号函数(又称正负号函数)()1,0sgn 1,0t t t -<⎧=⎨>⎩的Fourier变换.解:容易看出()()()sgn t u t u t =--,而1[()]()πδ().j u t F ωωω=-+F 9.求函数()()()1δδδδ222aa t a t a t f t t ⎡⎤⎛⎫⎛⎫=++-+++- ⎪ ⎪⎢⎥⎝⎭⎝⎭⎣⎦的Fourier 变换.解 :()()()()j 1δδδδe d 222ta a F f t t a t a t t ωωω+∞--∞⎡⎤⎛⎫⎛⎫⎡⎤==++-+++- ⎪ ⎪⎢⎥⎣⎦⎝⎭⎝⎭⎣⎦⎰F j j j j 1e e e e 222t t t t a a t a t a t t ωωωω----⎡⎤⎢⎥=+++⎢⎥=-==-=⎢⎥⎣⎦cos cos 2aa ωω=+.10 .求函数()cos sin t f t t =的Fourier 变换. 解: 已知()()000sin j πδδt ωωωωω⎡⎤=+--⎡⎤⎣⎦⎣⎦F由()1cos sin sin 22f t t t t ==有()()()πjδ2δ22f t ωω⎡⎤⎡⎤=+--⎣⎦⎣⎦F 11.求函数()3sin f t t =的Fourier 变换.解:已知()0j 0e 2πδtωωω⎡⎤=-⎣⎦F ,由()()3j j 33j j -j 3j e e j sin e 3e 3e e 2j 8t t t t t t f t t --⎛⎫-===-+- ⎪⎝⎭即得()()()()()πjδ33δ13δ1δ34f t ωωωω⎡⎤⎡⎤=---++-+⎣⎦⎣⎦F12.求函数()πsin 53t t f ⎛⎫=+ ⎪⎝⎭的Fourier 变换.解: 由于()π1sin 5sin5cos5322f t t t t ⎛⎫=+=+ ⎪⎝⎭故()()()()()πjδ5δ55δ52f t ωωωω⎤⎡⎤⎡⎤=+--+++-⎥⎣⎦⎣⎦⎣⎦F . 14.证明:若()()j e t F ϕω⎡⎤=⎣⎦F ,其中()t ϕ为一实数,则()()()1cos 2t F F ϕωω⎡⎤⎡⎤=+-⎣⎦⎣⎦F ()()()1sin 2j t F F ϕωω⎡⎤⎡⎤=--⎣⎦⎣⎦F 其中()F ω-为()F ω的共轭函数.证明:因为 ()()j j e e d t t F t ϕωω+∞--∞=⋅⎰()()()j j j j ee d ee d t t tt F t t ϕϕωωω+∞+∞---∞-∞-==⋅⎰⎰()()()()()()j j j j 1e ee d cos e d cos 22t t t t F F t t t t ϕϕωωωωϕϕ-+∞+∞---∞-∞+⎡⎤⎡⎤+-===⎣⎦⎣⎦⎰⎰F 同理可证另一等式.17.求作如图的锯齿形波的频谱图.(图形见教科书).解 :02π,T ω=()1,00,ht t Tf t T ⎧≤≤⎪=⎨⎪⎩其他()00111d d 2TTh C f t t ht t TTT ===⎰⎰()()000j j j 02011e d e d e d TTTn t n t n t n ht h C F n f t t t t t TTT Tωωωω---===⋅=⎰⎰⎰00j j 211j e e d j j 2πTn t n t Thht T n n n ωωωω--⎡⎤=⋅+=⎢⎥-⎣⎦⎰()()()()()000j j 2πδ2πδπδδ.22πn n n n h h hF n h n n nωωωωωωω+∞+∞=-∞=-∞≠≠=+⋅-=+⋅-∑∑。
积分变换习题解答

∫ ∫ ∫ ∫ 证 f (t) = 1
( ) +∞
+∞
f
τ
e− jωτ dτ ejωt dω =
1
+∞ +∞ f (τ ) (cosωτ − jsin ωτ ) cosωtdτ dω
2π −∞ −∞
2π −∞ −∞
∫ ∫ ∫ ∫ + 1
2π
+∞ +∞ f (τ ) (cosωτ − jsin ωτ ) jsin ωtdτ dω =
−∞
1+i 1−ω
+
e⎡⎣1−i(1+ω )⎤⎦t 0
−∞
1−i 1+ω
+
e⎡⎣−1+i(1−ω)⎤⎦t +∞
0
−1+ i 1− ω
+
e⎡⎣−1−i(1+ω )⎤⎦t +∞
0
−1− i 1+ ω
⎫ ⎪ ⎬ ⎪
⎩
⎭
=
1 2
⎡
⎢ ⎣
1+
1
i (1−ω
)
+
1−
1
i (1+ ω )
+
1−
1
i (1− ω )
+∞ +∞
f
t
e−iωt dteiωt dω = 1
+∞ 1 1− t 2 e−iωt dteiωt dω
2π −∞ −∞
2π −∞ −1
∫ ∫ ( ) ∫ = 1
π
+∞ −∞
1 1−t2
0
cosωtdteiωtdω = 1 π
+∞ −∞
复变函数与积分变换习题解答
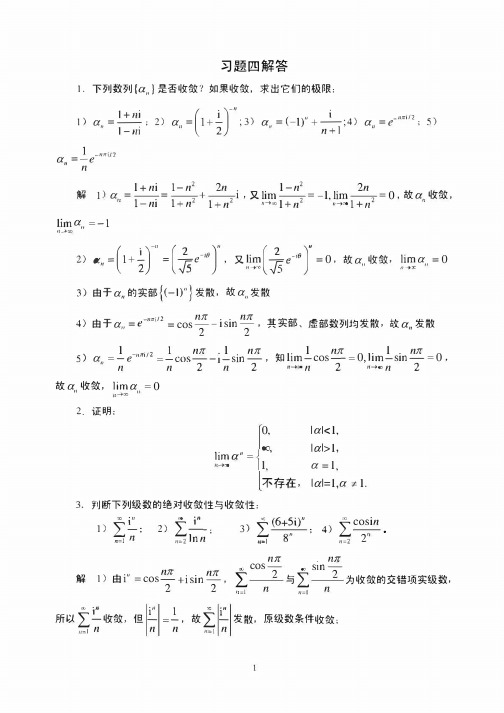
= 幕级数区—n!C+!..l.._zn
+
I
的收敛半径为R
=1/lim II 玉oo
a
___.斗 !!
a,,
=l114im00
c,, /(n + 1) c11十I /(n+2)
=1/lpl;
幕级数I:nc11z11一)的收敛半径为R
= 1/lim
a
一
all /l�CI)
lim nc" =1/I p I ;
(1+ —1 )”
=lim 00 II千
n n+ 1
= 0;
扣厂 (3) R=1//l1-i-m)00
II
lim1/ 11 11---'>00
+
i
I
=
1/忒
;
恩妇 (4) R=ll�
=l;
:I 匠)I 三叶三 曰勹 CS) R=l/
酝 =II
ch
=II
=I;
皿聂l (6) R=ll�
=l11�im00I ln in I= oo ;
。 I
I�
(n + l)c11+L
故以上三个幕级数有相同的收敛半径。
2 忙 9 设级数f n=O c"收敛,而 11=0 I发散,证明f 11=0 c11z11的收敛半径力l。
3
证明
由级数Len收敛,知幕级数LC11 Z11 在z= l处收敛,由Abel定理知I:c11z11
11=0
11 =0
11=0
习题四解答
1. 下列数列{a }是否收敛?如果收敛, 求出它们的极限:
"
芒, 气), 二, I) a,,=
积分变换课后题答案

第一章 傅里叶变换内容提要:一 傅里叶变换定义1定义2定义34傅里叶积分定理二 δ函数型序列的充分条件构成δ1.)(21)(,)(21)(,)()( 为傅里叶积分公式即称则若设:dw e dx e x f t f dw e w F t f dt e t f w F iwt iwx iwt iwt ⎰⎰⎰⎰∞+∞--∞+∞-+∞∞--+∞∞-⎥⎦⎤⎢⎣⎡===ππ=)(t f [])(1-w F ℱ;)()()(21逆变换的傅里叶为Fourier w F dw e w F iwt ⎰+∞∞-=π=)(w F [])(t f ;)()()(变换的傅里叶为Fourier t f dt e t f iwt -+∞∞-⎰=ℱ .)(21)(,)(21)(,)()( 为傅里叶积分公式即称则若设:dw e dx e x f t f dw e w F t f dt e t f w F iwt iwx iwt iwt ⎰⎰⎰⎰∞+∞--∞+∞-+∞∞--+∞∞-⎥⎦⎤⎢⎣⎡===ππ满足如下两个条件:若函数)(t f 限个极值点;类间断点,且至多有有上连续或有有限个第一在即条件上满足狄利克雷在实轴的任何有限区间],[)( ,)(],[)( )b a t f Dirichlet b a t f i .],[)( )的反常积分收敛在区间+∞-∞t f ii .)()(,)(21)]0()0([21)(dt e t f w F dw e w F t f t f t f iwtiwt -∞+∞-∞+∞-⎰⎰==-++其中且的傅里叶变换存在,则函数π函数列的该趋向下,,则在)(的某种趋向下,函数若在参数可积,且满足在实轴的任何有限区间设普通函数βεβϕβ++∞∞→==⎰0,1)()(-dt t f t f ).()( )0)(( ))(1()(1)(t t f t f t f δδβϕβϕβϕββ→>=即:型序列,构成一个型序列几个常用 2δ⎪⎩⎪⎨⎧<<===⎩⎨⎧<<=. 0)0( 1)1(1)( . 0)10( 1)( )1其它,,则令其它,εεεεβεεt t f t f t t f ).()(lim 00t t δδδεεε=→+→+型序列,即时为当.)()1(1)(,1)(,)1(1)( )2(22-2πεεεεδπεw w f w dt t f t t f R +===+=⎰+∞∞构造:显然).()(lim 00w w R δδδεεε=→+→+即型序列,时为当.)cos(21sin )()(,sin ,sin )( )3(-⎰⎰-+∞∞=====RRIR dw wt t Rt Rt Rf t dt tt t tt f ππδππ构造:因为).()(lim t t R IR R δδδ=+∞→+∞→型序列,即时为当.2)1(1)(,2,2)( )4(2222-22πβββδππββw G t t ew f w dt eet f -∞+∞--====⎰构造:因为).()(lim 00w w G δδδβββ=→+→+型序列,即时为当函数的积分3δ).)(()()(lim )()()1-00-0处处无穷次可微,定义:t f dt t f t t dt t f t t ⎰⎰+∞∞→+∞∞-=-+εεδδ三 傅立叶变换的性质四 几个常用函数傅里叶变换对1.线性性质2.位移性质)( t f 若ℱ, )(w F 3.微分性质)( n1k ∑=t f C k k . )(1∑=nk k k w F C ℱ )( )1 a t f ±ℱ ;)( )(为实数a w F e iwa ±t iw et f 0)( )2±.)( )(00为实数w w w F ℱ)( t f k 若),,2,1( )(n k w F k =ℱ)( t f 若ℱ, )(w F )( )1 )(t fn ;)( )()(为自然数n w F iw n ℱ)()( )2t f -it n .)( )()(为自然数n w F n ℱ)( t f 若ℱ)(w F 4.积分性质 则ℱ []).(1)(w F iw t g =).( )10)((lim )(1lim )()(lim)()()2000-00-000t f t f dt t f dtt f t t dt t f t t t t =<<+==-=-+++→+→+∞∞→+∞∞⎰⎰⎰θεθεδδδεεεεε函数的筛选性质:2sin 2τw w E).2( 0),2( )()1⎪⎩⎪⎨⎧><=ττt t E t f ℱ)0( )0( 0)0( )()2>⎩⎨⎧<>=-ββt t e t f t 1iw+βℱ习题1.11. 求下列函数的Fourier 变换. (1)ℱ)]([t f =dt e A t i ⎰-τω0=0τωωt i e i A --=)1(ωτωi e i A --.(2) ℱ)]([t f =dt te e t i t⎰+∞∞---ωcos =dt te t i ⎰+∞+-0)1(cos ω+dt te t i ⎰∞--0)1(cos ω由201cos a a dt te at +=⎰+∞-,2001cos cos aa dt te dt te at at +==⎰⎰+∞-∞-, 可知:ℱ)]([t f =22)1(11)1(11ωωωωi i i i -+-++++=22424ωω-+.2. 求Fourier 逆变换. ℱ)]([1ωF -=ωπωωβd e et i ⎰+∞∞--21=ωωπωβωβd e d e it it ⎰⎰∞-++∞+-+0)(0)([21=⎥⎦⎤⎢⎣⎡∞-++∞++-++-010121)()(ωβωβββπit it e it e it=22221t +ββπ=)(22t +βπβ.3. ℱ)]([t f =⎰--⋅ππωdt e t t i sin=-⎰--ππωt d e t i cos =-⎰---⋅--⋅ππωωωππdt e t i te t i t i cos cos=()⎰-----ππωωωωπt d e i e e t i t i t i sin cos=⎰----⋅+-ππωωωωωdt te i i e e t i t i t i sin )(=⎰---+-ππωωωωdt teeeti ti ti sin 2ℱ)(1w iwπδ+)( )5t u )( )3t δℱ 1)( 2w πδ1)4ℱℱ)]([t f =1sin 22-ωωπi由ℱ)()]([1t f F =-ω可知下面的等式成立.4. 求下列函数的Fourier 积分。
积分变换习题解答2-5

2-51.求下列常系数微分方程的解:1)()2e ,00t y y y '-==;8)()()()331,0000y y y y y y y '''''''''+++====;12)()()()()()420,0000,01y y y y y y y ''''''''++=====;16)()π10sin 2,00,12y y t y y ⎛⎫''+=== ⎪⎝⎭。
分析:解题步骤,首先取Laplace 变换将微分方程化为象函数的代数方程, 解代数方程求出象函数, 再取Laplace 逆变换得最后的解.解:1)方程两边取Laplace 变换,并结合初始条件可得()()21e 2t sY s Y s s ⎡⎤-==⎣⎦-L 即()()()1112121Y s s s s s ==-----. 从而方程的解为()()12e e t t y t Y s -⎡⎤==-⎣⎦L8)对方程两边取Laplace 变换,并结合初始条件,有()()()()32133s Y s s Y s sY s Y s s+++= 即()()()332113311Y s s s s s s s ==++++ 由留数计算法,由于10s =是()Y s 的一个一级极点,21s =-是()Y s 的一个三级极点,从而方程的解为()()()121Res e k st s s k f t Y s Y s =-=⎡⎤⎡⎤==⎣⎦⎣⎦∑L ()12232e 1d 1lim e 2!d 1stst s s s s s s s →=⎡⎤=+⎢⎥⎣⎦+ ()2231e 2211lim 2st s s t st s →--+=+2111e 2t t t -⎛⎫=-++ ⎪⎝⎭. 12)对方程两边取Laplace 变换,并结合初始条件,有()()()()()()()()()43220000220200s Y s s y s y sy y s Y s sy y Y s '''''''----+--+= 即()()22221111ss Y s s s s ==⋅+++ 从而方程的解为()()11cos sin sin 2y t Y s t t t t -⎡⎤==*=⎣⎦L . 16)对方程两边取Laplace 变换,并结合初始条件,有 ()()()()22200104s Y s sy y Y s s '--+=+ 即()()()()222020114y Y s s s s '=++++()222020113141y s s s '⎛⎫=-+ ⎪+++⎝⎭,从而 ()()()12010sin sin 20sin 33y t Y s t t y t -'⎡⎤==-+⎣⎦L . 为了确定()0y ',将条件π12y ⎛⎫= ⎪⎝⎭代入上式可得()1703y '=-,所以方程的解为()10sin sin 23y t t t =- 2.求下列变系数微分方程的解:1)()()40,03,00ty y ty y y ''''++===;3)()()()2120,02ty t y t y y '''+-+-==;5)()()()()10,000,0ty n y y y y n ''''+-+===≥.解: 1)方程两边取Laplace 变换,有[]40ty y ty '''++=L即[][][]40ty y ty '''++=L L L ,亦即()()()()()()2d d 00040d d s Y s sy y sY s y Y s s s'⎡⎤⎡⎤---+--=⎣⎦⎣⎦ 从而()()2d 40d Y s sY s s ++= 2d d 04Y s s Y s +=+ 两边积分可得()211ln ln 42Y s c ++=或()Y s =取其逆变换,有()()02y t cJ t =欲求c ,可由条件()03y =得到,即()()0003y cJ c ===,所以方程的解为()()032y t J t =其中()()()2001!12kk k x J x k k ∞=-⎛⎫= ⎪Γ+⎝⎭∑称为零阶第一类Bessel 函数.3)方程两边取Laplace 变换,有[]()()2120ty t y t y '''⎡⎤⎡⎤+-+-=⎣⎦⎣⎦L L L()()()()()2d d 0020d d s Y s sy y sY s y s s'⎡⎤⎡⎤------⎣⎦⎣⎦ ()()()()d2020d sY s y Y s Y s s ⎡⎤---=⎣⎦整理化简后可得()()()()2d21416d s s Y s s Y s s ++++=即()()()2d46d 11Y s Y s s s s +=++这是一阶线性非齐次微分方程,这里()()()246,11P s Q s s s ==++所以()()()()d d e e d P s s P s sY s Q s s c -⎡⎤⎰⎰=+⎢⎥⎣⎦⎰()()24161d 1s s c s ⎡⎤=++⎣⎦+⎰()4211cs s =+++从而方程的解为()()132e e 3!t t cy t Y s t ---⎡⎤==+⎣⎦L ()312etc t -=+(1c 为任意常数)5)方程两边取Laplace 变换,有[]()[][]10ty n y y '''+--=L L L即()()()2d 00d s Y s sy y s'⎡⎤---+⎣⎦()()()()100n sY s y Y s ⎡⎤---=⎣⎦ 整理化简后可得()()()2d 11d Y s n ss Y s s -+=两边积分可得()()11ln n Y s s cs -+=-即()()1111e e n s s n cY s cs s ---++==从而方程的解为()(2nn y t ct J =(c 为任意常数)其中n J 称为n 阶第一类Bessel 函数。
(完整版)复变函数与积分变换习题答案

一、将下列复数用代数式、三角式、指数式表示出来。
(1) i 解:2cossin22ii e i πππ==+(2) -1解:1cos sin i e i πππ-==+ (3)1+解:()/3122cos /3sin /3i e i πππ+==+ (4) 1cos sin i αα-+ 解:2221cos sin 2sin 2sincos2sin(sincos )2222222sincos()sin()2sin 222222i i i i i e πααααααααααπαπαα⎛⎫- ⎪⎝⎭-+=+=+⎛⎫=-+-= ⎪⎝⎭(5) 3z解:()3333cos3sin3i z r e r i θθθ==+ (6) 1i e +解:()1cos1sin1i i e ee e i +==+(7)11ii-+ 解:3/411cos3/4sin 3/411i i i i e i i i πππ--==-==+++二、计算下列数值(1) 解:1ar 21ar 21ar 2 b i ctg k a bi ctg abi ctgaπ⎛⎫+ ⎪⎝⎭==⎧⎪=⎨⎪⎩(2)解:6226363463222i k i i i i e i ee e iπππππππ⎛⎫⎛⎫++ ⎪ ⎪⎝⎭⎝⎭⎛⎫+ ⎪⎝⎭⎧=+⎪⎪⎪⎨====-+⎪⎪⎪=-⎩(3) i i 解:()2222ii k k i i e eππππ⎛⎫⎛⎫+-+ ⎪ ⎪⎝⎭⎝⎭==(4)解:()1/2222ii k k eeππππ⎛⎫⎛⎫++ ⎪ ⎪⎝⎭⎝⎭==(5) cos5α解:由于:()()552cos5i i e e ααα-+=,而:()()()()()()()()5555555555cos sin cos sin cos sin cos sin nni nn nni n n e i C i e i C i αααααααααα-=--==+==-=-∑∑所以:()()()()()()()()()()()555505555043253543251cos5cos sin cos sin 21 cos sin 112 5cos sin cos sin cos 5cos sin 10cos sin cos n n n nn n n n nn n C i i C i i C i ααααααααααααααααα--=--=⎡⎤=+-⎣⎦⎡⎤=+-⎣⎦=++=-+∑∑(6) sin5α解:由于:()()552sin 5i i ee ααα--=,所以:()()()()()()()()()()()()55550555505234245552341sin 5cos sin cos sin 21 cos sin 1121 sin cos sin sin cos sin 10cos sin 5sin cos n n n nn n n n nn n C i i i C i i i C i C i iααααααααααααααααα--=--=⎡⎤=--⎣⎦⎡⎤=--⎣⎦=++=-+∑∑ (7) cos cos2cos n ααα+++L L 解:()()221cos cos 2cos ()()2(1)1(1)11(1)(1)1 21122(1cos )1 2i i in i i in i in i i in i i in i in i i i n e e e e e e e e e e e e e e e e e e e e ααααααααααααααααααααααα----------⎡⎤+++=+++++++⎣⎦⎡⎤--+--⎡⎤--⎢⎥=+=⎢⎥---⎢⎥⎣⎦⎣⎦+=L L L L L L (1)(1)22(1cos )12cos 22cos(1)2cos cos 1cos(1)cos 22(1cos )2(1cos )1sin()sin22 2sin2i i n i n in in e e e e n n n n n ααααααααααααααααα+-+-⎡⎤---++⎢⎥-⎣⎦⎡⎤--++--++==⎢⎥--⎣⎦+-=(8) sin sin 2sin n ααα+++L L 解:()()221sin sin 2sin ()()2(1)1(1)11(1)(1)1 21122(1cos )1 2i i in i i in i in i i in i i in i in i i i n e e e e e e i e e e e e e e e e e i e e i e i αααααααααααααααααααααα---------⎡⎤+++=+++-+++⎣⎦⎡⎤-----⎡⎤--⎢⎥=-=⎢⎥---⎢⎥⎣⎦⎣⎦=L L L L L L (1)(1)112(1cos )12sin 2sin(1)2sin sin sin(1)sin 22(1cos )2(1cos )1cos()cos22 2sin2i n in i i n in e e e e e i i n i n n n i n αααααααααααααααααα+--+-⎡⎤--+-++-⎢⎥-⎣⎦⎡⎤-++-++==⎢⎥--⎣⎦-++=1.2 复变函数1、试证明函数f (z )=Arg(z ) (-π<Arg(z) ≤π),在负实轴上(包括原点)不连续。
高等教育出版社《复变函数》与《积分变换》第四版课后习题参考答案

⎝ 12
12 ⎠
6 2ei5π / 4 = 6 2⎜⎛ cos 5π + i sin 5π ⎟⎞ 。
⎝4
4⎠
15.若 (1+ i)n = (1− i)n ,试求 n 的值。
5
解 由题意即 ( 2eiπ / 4 )n = ( 2e−iπ / 4 )n , einπ / 4 = e−inπ / 4 , sin n π = 0 , 4
+
2kπ
= − arctan 5 + 2kπ, 3
k = 0,±1,±2,".
(3)
(3
+
4i)(2
2i
−
5i)
=
(3
+
4i)(2 − (2i)(−
5i)(− 2i)
2i)
=
(26
−
7i)(−
4
2i)
所以
= −7 − 26i = − 7 −13i
2
2
Re⎨⎧ (3
+
4i)(2
−
5i)⎫
⎬
=
−
7
,
⎩ 2i ⎭ 2
Im⎨⎧ ⎩
(3
+
4i)(2
2i
−
5i)⎫
⎬ ⎭
=
−13
,
1
⎡ ⎢ ⎣
(3
+
4i)(2
2i
−
5i)⎤
⎥ ⎦
=
−
7 2
+
l3i
(3 + 4i)(2 − 5i) = 5 29 ,
2i
2
Arg⎢⎣⎡ (3
+
4
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
⎧ 0, −∞ < t < −1 ⎪−1, −1 < t < 0 ⎪ (3) f ( t ) = ⎨ 0 < t <1 ⎪ 1, ⎪ ⎩ 0, 1 < t < +∞
解
(1)函数 f (t ) = ⎨
⎧1 − t 2 , | t |< 1 满足傅氏积分定理的条件,傅氏积分公式为 | t |> 1 ⎩ 0,
| t |< 1 | t |= 1 。 | t |> 1
习题二
1. 求矩形脉冲函数 f (t ) = ⎨
F (ω ) = ¶ ⎡ ⎣ f ( t )⎤ ⎦=
⎧ A, 0 ≤ t ≤ τ 的傅氏变换。 其他 ⎩ 0,
+∞ τ − jωt − jω t = f t e dt ( ) ∫ −∞ ∫ 0 Ae dt
⎧sin t , | t |≤ π , (3) f (t ) = ⎨ 证明 ⎩ 0, | t |> π ,
∫
+∞
0
⎧π sin ωπ sin ωt ⎪ sin t , | t |≤ π dω = ⎨ 2 2 1− ω ⎪ | t |> π ⎩ 0,
e dt = 2 ∫ e
0 +∞ −βt
解 (1) F (t ) = ¶ ⎡ ⎣ f ( t )⎤ ⎦=
−∞
1 2π
+∞ −∞
∫ ∫
−∞
+∞ −∞
+∞
+∞
−∞
f (τ ) e− jωτ dτ e jωt dω =
1 2π
∫ ∫
−∞
+∞
+∞
−∞
f (τ ) (cos ωτ − jsin ωτ ) cos ωtdτ dω
+∞
+
1 2π
+∞ −∞
∫ ∫
1
f (τ ) (cos ωτ − jsin ωτ ) jsin ωtdτ d ω = ∫
= =
设 f (t ) 是偶函数
π j∫ ∫
−∞
1
0
f (τ ) sin ωτ dτ e jωt d ω =
1 +∞ ( b(ω ) 是 ω 的奇函数) b (ω )e jωt d ω 。 2 j ∫−∞
+∞ 1 +∞ b cos t jsin t d ω ω + ω ω = ( )( ) ∫0 b (ω ) sin ωtdω 2 j ∫−∞
⎤ 2ω 2 + 4 1⎡ 1 1 1 1 = ⎢ + + + ⎥= 2 ⎣ 1 + i (1 − ω ) 1 − i (1 + ω ) 1 − i (1 − ω ) 1 + i (1 + ω ) ⎦ ω 4 + 4
f (t ) 的积分表达式为
f (t ) = 1 2π
+∞ 0
∫
+∞
−∞
F (ω )e i ωt dω =
f (t ) =
1 2π
∫ ∫
−∞
+∞
+∞
−∞
f (τ ) e− jωτ dτ e jωt dω = =
1 2π
∫ ∫
−∞
+∞
+∞
−∞
f (τ )( cos ωτ − jsin ωτ ) dτ e jωt dω
+∞ 1 +∞ jωt a ω e d ω = ( ) ∫0 a (ω ) cos ωtdω 2 ∫−∞
a(ω ) 是 ω 的偶函数。 (注也可由 1 题推证 2 题)
3.在题 2 中,设 f ( t ) = ⎨
⎧1, | t |≤ 1 ,试算出 a(ω ) ,并推证 ⎩0, | t |> 1
⎧π ⎪ 2 , | t |< 1 ⎪ +∞ sin ω cos ωt ⎪π d ω = ⎨ , | t |= 1 ∫0 ω ⎪4 ⎪ 0, | t |> 1 ⎪ ⎩
傅氏变换习题解答 习题一
1.试证:若 f (t ) 满足傅氏积分定理的条件,则有
f (t ) = ∫
其中
+∞
0
a (ω ) cos ωtd ω + ∫ b(ω ) sin ωtd ω
0
+∞
a (ω ) = b(ω ) =
证 f (t ) =
π∫ π∫
1
1
+∞
−∞ +∞
f (τ ) cos ωτ dτ , f (τ ) sin ωτ dτ
1 2π
∫ ∫
+∞
+∞
e−t
1 +∞ +∞ − t +i( 2−ω )t − t −i( 2+ω )t e i 2 t − e − i 2 t − iω t i ω t e −e dteiωt dω e dte d ω = 4π i ∫−∞ ∫0 2i
(
)
=
4π i ∫−∞
4π i ∫−∞ 1
1
⎡ −1−i ( 2 +ω )⎤ ⎣ −1+ i ( 2 −ω )⎤ ⎦t ⎦t ⎤ ⎡ e⎡ e⎣ i ωt − ⎢ ⎥ e dω ⎢ −1 + i ( 2 − ω ) − 1 − i ( 2 + ω ) ⎦ ⎥0 ⎣
=
1 2
{∫
0
−∞
e⎣
⎡1+ i (1−ω )⎦ ⎤t
dt + ∫ e ⎣
−∞
0
⎡1−i (1+ω ) ⎦ ⎤t
dt + ∫ e ⎣
0
dt + ∫ e ⎣
0
+∞
⎡ −1− i(1+ω ) ⎦ ⎤t
dt
}
+∞ +∞ 0 0 ⎡ ⎡ ⎡ ⎧ ⎡ ⎫ ⎣ −1+ i (1−ω ) ⎤ ⎦t ⎣ −1− i (1+ω )⎤ ⎦t ⎣1+ i (1−ω ) ⎤ ⎦t ⎣1− i (1+ω )⎤ ⎦t e e e e 1⎪ 0 0 ⎪ −∞ −∞ + + + = ⎨ ⎬ 2 ⎪ 1 + i (1 − ω ) 1 − i (1 + ω ) −1 + i (1 − ω ) −1 − i (1 + ω ) ⎪ ⎩ ⎭
+∞ 0
1
0
π
∫
+∞
−∞ +∞
f (τ ) cos ωτ dτ cos ωtdω
+∫
因
π
∫
+∞
−∞
f (τ ) sin ωτ dτ sin ωtd ω = ∫ a (ω ) cos ωtd ω + ∫ b(ω ) sin ωtdω
0
+∞
∫
+∞
−∞
f (τ ) sin ωτ cos ωtdτ d ω为ω的奇函数 , ∫
证 f (t ) 是偶函数
a(ω ) = 2 +∞ 2 sin ωt 1 2 sin ω f (t ) cos ωtdt = = ∫ π 0 π ω 0 π ω
+∞ 0 a(ω ) cos ωtdω =
f (t ) = ∫
π
2 +∞ sin ω cos ωt dω ∫0 ω
所以
π ⎧ ⎪ 2 ⎪ +∞ sin ω cos ωt π ⎪π 0 + 1 π dω = f ( t ) = ⎨ = ∫0 2 4 ω ⎪2 2 0 ⎪ ⎪ ⎩
π
∫
4
+∞
−∞
⎡ sin ωt ⎢ ⎣ ω
⎛ 2t cos ωt 2sin ωt t 2 sin ωt ⎞ ⎤ iωt −⎜ − + ⎟ ⎥ e dω ] 2 ω3 ω ⎠⎦0 ⎝ ω
π∫
2 ( sin ω − ω cos ω )
ω
3
e iω t d ω =
π∫
+∞
sin ω − ω cos ω
0
ω3
f (t ) = =
=
1 2π
∫ ∫
−∞ +∞
1 0
+∞
+∞
−∞
f ( t ) e− i ωt dtei ωt d ω =
2 i ωt
1 2π
1
∫ ∫ (1 − t ) e
+∞
1 2
− i ωt
−∞
−1
dtei ωt d ω
1
1
π
1
∫ ∫ (1 − t ) cos ωtdte
−∞
+∞ −∞
dω =
−∞
f (τ ) cos ωτ cos ωtdτ d ω为ω的偶函数 。
2.试证:若 f (t ) 满足傅氏积分定理的条件,当 f (t ) 为奇函数时,则有
f (t ) = b(ω )sin (ωt )dω
∫
+∞
0
其中
b (ω ) =
当 f (t ) 为偶函数时,则有
2
π
∫
+∞
0
f (τ ) sin (ωτ ) dτ
3.求下列函数的傅氏变换,并推证下列积分结果。 (1) f (t ) = e
− β |t |
(β > 0) ,证明
+∞
∫
+∞
0
cos ωt π − β |t| dω = e 2 2 β +ω 2β
(2) f (t ) = e −|t| cos t ,证明 ∫
0
ω2 + 2 π cos(ωt )dω = e −|t| cos t 2 ω4 + 4
