Chapter05_Mathematics of Finance_13e

合集下载

Mathematical finance

Mathematical financeMathematical finance is a field of applied mathematics, concerned with financial markets. The subject has a close relationship with the discipline of financial economics, which is concerned with much of the underlying theory. Generally, mathematical finance will derive and extend the mathematical or numerical models suggested by financial economics. Thus, for example, while a financial economist might study the structural reasons why a company may have a certain share price, a financial mathematician may take the share price as a given, and attempt to use stochastic calculus to obtain the fair value of derivatives of the stock (see: Valuation of options; Financial modeling). The fundamental theorem of arbitrage-free pricing is one of the key theorems in mathematical finance, while the Black–Scholes equation and formula are amongst the key results.In terms of practice, mathematical finance also overlaps heavily with the field of computational finance (also known as financial engineering). Arguably, these are largely synonymous, although the latter focuses on application, while the former focuses on modeling and derivation (see: Quantitative analyst), often by help of stochastic asset models. In general, there exist two separate branches of finance that require advanced quantitative techniques: derivatives pricing on the one hand, and risk- and portfolio management on the other hand. These are discussed below.Many universities around the world now offer degree and research programs in mathematical finance; see Master of Mathematical Finance.History: Q versus PThere exist two separate branches of finance that require advanced quantitative techniques: derivatives pricing on the one hand, and risk and portfolio management on the other hand. One of the main differences is that they use different probabilities, namely the risk-neutral probability, denoted by "Q", and the actual probability, denoted by "P".Derivatives pricing: the Q worldFurther information: Black–ScholesThe goal of derivatives pricing is to determine the fair price of a given security in terms of more liquid securities whose price is determined by the law of supply and demand. Examples of securities being priced are plain vanilla and exotic options, convertible bonds, etc.Once a fair price has been determined, the sell-side trader can make a market on the security. Therefore, derivatives pricing is a complex "extrapolation" exercise to define the current market value of a security, which is then used by the sell-side community.Derivatives pricing: the Q worldGoal"extrapolate the present"Environmentrisk-neutral probabilityProcesses continuous-time martingalesDimension lowTools Ito calculus, PDE’sChallenges calibrationBusiness sell-sideQuantitative derivatives pricing was initiated by Louis Bachelier in The Theory of Speculation (published 1900), with the introduction of the most basic and most influential of processes, the Brownian motion, and its applications to the pricing of options. Bachelier modeled the time series of changes in the logarithm of stock prices as a randomwalk in which the short-term changes had a finite variance. This causes longer-term changes to follow a Gaussian distribution. Bachelier's work, however, was largely unknown outside academia.The theory remained dormant until Fischer Black and Myron Scholes, along with fundamental contributions by Robert C. Merton, applied the second most influential process, the geometric Brownian motion, to option pricing. For this M. Scholes and R. Merton were awarded the 1997 Nobel Memorial Prize in Economic Sciences. Black was ineligible for the prize because of his death in 1995.The next important step was the fundamental theorem of asset pricing by Harrison and Pliska (1981), according to which the suitably normalized current price Pof a security is arbitrage-free, and thus truly fair, only if there exists awith constant expected value which describes its future evolution:stochastic process Pt(1 )A process satisfying (1) is called a "martingale". A martingale does not reward risk. Thus the probability of thenormalized security price process is called "risk-neutral" and is typically denoted by the blackboard font letter " ".The relationship (1) must hold for all times t: therefore the processes used for derivatives pricing are naturally set in continuous time.The quants who operate in the Q world of derivatives pricing are specialists with deep knowledge of the specific products they model.Securities are priced individually, and thus the problems in the Q world are low-dimensional in nature. Calibration is one of the main challenges of the Q world: once a continuous-time parametric process has been calibrated to a set of traded securities through a relationship such as (1), a similar relationship is used to define the price of new derivatives.The main quantitative tools necessary to handle continuous-time Q-processes are Ito’s stochastic calculus and partial differential equations (PDE’s).Risk and portfolio management: the P worldRisk and portfolio management aims at modelling the probability distribution of the market prices of all the securities at a given future investment horizon.This "real" probability distribution of the market prices is typically denoted by the blackboard font letter " ", as opposed to the "risk-neutral" probability " " used in derivatives pricing.Based on the P distribution, the buy-side community takes decisions on which securities to purchase in order to improve the prospective profit-and-loss profile of their positions considered as a portfolio.Risk and portfolio management: the P worldGoal"model the future"Environment real probabilityProcesses discrete-time seriesDimension largeTools multivariate statisticsChallenges estimationBusiness buy-sideThe quantitative theory of risk and portfolio management started with the mean-variance framework of Harry Markowitz (1952), who caused a shift away from the concept of trying to identify the best individual stock forinvestment. Using a linear regression strategy to understand and quantify the risk (i.e. variance) and return (i.e. mean) of an entire portfolio of stocks, bonds, and other securities, an optimization strategy was used to choose a portfolio with largest mean return subject to acceptable levels of variance in the return. Next, breakthrough advances were made with the Capital Asset Pricing Model (CAPM) and the Arbitrage Pricing Theory (APT) developed by Treynor (1962), Mossin (1966), William Sharpe (1964), Lintner (1965) and Ross (1976).For their pioneering work, Markowitz and Sharpe, along with Merton Miller, shared the 1990 Nobel Memorial Prize in Economic Sciences, for the first time ever awarded for a work in finance.The portfolio-selection work of Markowitz and Sharpe introduced mathematics to the "black art" of investment management. With time, the mathematics has become more sophisticated. Thanks to Robert Merton and Paul Samuelson, one-period models were replaced by continuous time, Brownian-motion models, and the quadratic utility function implicit in mean–variance optimization was replaced by more general increasing, concave utility functions.[1] Furthermore, in more recent years the focus shifted toward estimation risk, i.e., the dangers of incorrectly assuming that advanced time series analysis alone can provide completely accurate estimates of the market parameters [2]Much effort has gone into the study of financial markets and how prices vary with time. Charles Dow, one of the founders of Dow Jones & Company and The Wall Street Journal, enunciated a set of ideas on the subject which are now called Dow Theory. This is the basis of the so-called technical analysis method of attempting to predict future changes. One of the tenets of "technical analysis" is that market trends give an indication of the future, at least in the short term. The claims of the technical analysts are disputed by many academics.CriticismOver the years, increaingly sophisticated mathematical models and derivative pricing strategies have been developed, but their credibility was damaged by the financial crisis of 2007–2010.Contemporary practice of mathematical finance has been subjected to criticism from figures within the field notably by Nassim Nicholas Taleb in his book The Black Swan[3] and Paul Wilmott. Taleb claims that the prices of financial assets cannot be characterized by the simple models currently in use, rendering much of current practice at best irrelevant, and, at worst, dangerously misleading. Wilmott and Emanuel Derman published the Financial Modelers' Manifesto in January 2008[4] which addresses some of the most serious concerns.Bodies such as the Institute for New Economic Thinking are now attempting to establish more effective theories and methods.[5]In general, modeling the changes by distributions with finite variance is, increasingly, said to be inappropriate. In the 1960s it was discovered by Benoît Mandelbrot that changes in prices do not follow a Gaussian distribution, but are rather modeled better by Lévy alpha-stable distributions. The scale of change, or volatility, depends on the length of the time interval to a power a bit more than 1/2. Large changes up or down are more likely than what one would calculate using a Gaussian distribution with an estimated standard deviation.[3] See also Financial models with long-tailed distributions and volatility clustering.Mathematical finance articlesSee also Outline of finance: § Financial mathematics; § Mathematical tools; § Derivatives pricing. Mathematical tools•Asymptotic analysis•Mathematical models•Stochastic calculus•Brownian motion•Lévy process•Calculus•Monte Carlo method•Stochastic differential equations•Copulas•Numerical analysis•Stochastic volatility•Numerical partial differential equations•Crank–Nicolson method•Finite difference method •Differential equations•Real analysis•Value at risk•Expected value•Partial differential equations•Volatility•ARCH model•GARCH model•Ergodic theory•Probability•Feynman–Kac formula•Probability distributions•Binomial distribution•Log-normal distribution•Fourier transform•Quantile functions•Heat equation•Gaussian copulas•Radon–Nikodym derivative•Girsanov's theorem•Risk-neutral measure•Itô's lemma•Martingale representation theoremDerivatives pricing•The Brownian Motion Model of Financial Markets•Rational pricing assumptions •Risk neutral valuation•Arbitrage-free pricing •Forward Price Formula •Futures contract pricing •Swap Valuation •Options•Put–call parity (Arbitragerelationships for options)•Intrinsic value, Time value•Moneyness•Pricing models•Black–Scholes model•Black model•Binomial options model•Monte Carlo option model•Implied volatility, Volatility smile•SABR Volatility Model•Markov Switching Multifractal•The Greeks•Finite difference methods foroption pricing•Vanna Volga method•Trinomial tree•Garman-Kohlhagen model•Optimal stopping (Pricing ofAmerican options)•Interest rate derivatives•Black model•caps and floors•swaptions•Bond options•Short-rate models•Rendleman-Bartter model•Vasicek model•Ho-Lee model•Hull–White model•Cox–Ingersoll–Ross model•Black–Karasinski model•Black–Derman–Toy model•Kalotay–Williams–Fabozzi model•Longstaff–Schwartz model•Chen model•Forward rate-based models•LIBOR market model(Brace–Gatarek–Musiela Model, BGM)•Heath–Jarrow–Morton Model (HJM)Notes[1]Karatzas, Ioannis; Shreve, Steve (1998). Methods of Mathematical Finance. Secaucus, NJ, USA: Springer-Verlag New York, Incorporated.ISBN 9780387948393.[2]Meucci, Attilio (2005). Risk and Asset Allocation. Springer. ISBN 9783642009648.[3]Taleb, Nassim Nicholas (2007). The Black Swan: The Impact of the Highly Improbable. Random House Trade. ISBN 978-1-4000-6351-2.[4]"Financial Modelers' Manifesto" (/blogs/paul/index.cfm/2009/1/8/Financial-Modelers-Manifesto). PaulWilmott's Blog. January 8, 2009. . Retrieved June 1, 2012.[5]Gillian Tett (April 15, 2010). "Mathematicians must get out of their ivory towers" (/cms/s/0/cfb9c43a-48b7-11df-8af4-00144feab49a.html). Financial Times. .References•Harold Markowitz, Portfolio Selection, Journal of Finance, 7, 1952, pp. 77–91•William Sharpe, Investments, Prentice-Hall, 1985•Attilio Meucci, versus Q: Differences and Commonalities between the Two Areas of Quantitative Finance (http:// /abstract=1717163''P), GARP Risk Professional, February 2011, pp. 41-44Article Sources and Contributors6Article Sources and ContributorsMathematical finance Source: /w/index.php?oldid=500952080 Contributors: A.j.g.cairns, Acroterion, Ahd2007, Ahoerstemeier, Albertod4, Allemandtando, Amckern, Angelachou, Arthur Rubin, Author007, Avraham, Ayonbd2000, Baoura, Beetstra, Billolik, Brad7777, Btyner, Burakg, Burlywood, CapitalR, Celuici, Cfries, Charles Matthews, Christoff pale, Christofurio, Ciphers, Colonel Warden, Cursive, DMCer, DocendoDiscimus, DominicConnor, Drootopula, DuncanHill, Dysprosia, Edward, Elwikipedista, Eric Kvaalen, Evercat, Eweinber,FF2010, Fastfission, Feco, Financestudent, Fintor, Flowanda, Gabbe, Gary King, Gene Nygaard, Giftlite, Giganut, HGB, Halliron, Hannibal19, HappyCamper, Headbomb, Hroðulf, Hu12,Hégésippe Cormier, JBellis, JYolkowski, Jackol, Jamesfranklingresham, Jimmaths, Jmnbatista, JohnBlackburne, JonHarder, JonMcLoone, Jonhol, Jrtayloriv, Kaslanidi, Kaypoh, Kimys,Kolmogorov Complexity, Kuru, Lamro, Langostas, Looxix, MER-C, MM21, Mav, Mic, Michael Hardy, Michaltomek, Mikaey, Minesweeper, MrOllie, Msh210, Mydogategodshat,Nikossskantzos, Niuer, Nparikh, Oleg Alexandrov, Onyxxman, Optakeover, Paul A, Pcb21, PhotoBox, Pnm, Portutusd, Ppntori, Punanimal, Quantchina, Quantnet, Ralphpukei, Rasmus Faber, Rhobite, Riskbooks, Rodo82, Ronnotel, Ruud Koot, SUPER-QUANT-HERO, Sardanaphalus, Sentriclecub, Silly rabbit, SkyWalker, Smaines, Smesh, Stanislav87, SymmyS, Tassedethe, Taxman, Template namespace initialisation script, Tesscass, Tigergb, Timorrill, Timwi, Uxejn, Vabramov, Vasquezomlin, WebScientist, Willsmith, Woohookitty, Xiaobajie, YUL89YYZ, Yunli, Zfeinst, Zfr, 253 anonymous editsLicenseCreative Commons Attribution-Share Alike 3.0 Unported///licenses/by-sa/3.0/。

Mathematics of Finance

If we are investing an annuity payment, we often want to calculate what it will grow to.
FVannuity Pmt(1 k)n
1
Pmt(1 k)n
2
.... Pmt(1 k)0
(1 k)n 1 FV Pmt k
18
Example: Assume you have $1,200 to invest and want to know how long it will take for that sum to grow to $3,000 if invested in a CD at 7%:
9
Solving For k Or n (III)
7
Present Value
Assume that your parents are making the same calculation for your sibling’s (who is just starting high school) first tuition payment.
8
Solving For k Or n
1
Why “Mathematics” Matters
Characteristics of financial securities: Invest today in exchange for future cash flow promises. Control rights in case cash flows fail to materialize. Examples: Corporations issuing stocks. Corporations and Government issuing bonds. Individuals receiving bank/finance company loan Individuals make deposits in saving accounts What are these “promises” worth? Payments occur in the future. Payments may be risky.

S_FMA_4

Financial Mathematics for ActuariesChapter4Rates of ReturnLearning Objectives •Internal rate of return(yield rate)•One-period rate of return of a fund:time-weighted rate of return and dollar-weighted(money-weighted)rate of return•Rate of return over longer periods:geometric mean rate of return and arithmetic mean rate of return•Portfolio return and return of a short-selling strategy •Crediting interest:investment-year method and portfolio method •Inﬂation:real rate of return•Capital budgeting and project appraisal4.1Internal Rate of Return •Consider a project with initial investment C0.We assume the cash ﬂows occur at regular intervals.•The project lasts for n years and the future cashﬂows are denoted by C1,···,C n.•We adopt the convention that cash inﬂows to the project(invest-ments)are positive and cash outﬂows from the project(withdrawals) are negative.•We deﬁne the internal rate of return(IRR)(also called the yield rate)as the rate of interest such that the sum of the present values of the cashﬂows is equated to zero.•Denoting the internal rate of return by y ,we have n X j =0C j (1+y )j =0,(4.1)where j is the time at which the cash ﬂow C j occurs.•This equation can also be written as C 0=−n X j =1C j (1+y )j .(4.2)•Thus,the net present value of all future withdrawals (injections are negative withdrawals)evaluated at the IRR is equal to the initial investment.Example 4.1:A project requires an initial cash outlay of $2,000and is expected to generate $800at the end of year 1and $1,600at the endof year2,at which time the project will terminate.Calculate the IRR of the project.Solution:If we denote v=1/(1+y),we have,from(4.2)2,000=800v+1,600v2,or5=2v+4v2.Dropping the negative answer from the quadratic equation,we havev=−2+√4+4×4×52×4=0.8956.Thus,y=(1/v)−1=11.66%.Note that v<0implies y<−1,i.e.,the loss is larger than100%,which is precluded from consideration.2•There is generally no analytic solution for y in(4.1)when n>2, and numerical methods have to be used.The Excel function IRR enables us to compute the answer easily.Its usage is described as follows:Example4.2:An investor pays$5million for a5-year lease of a shopping mall.He will receive$1.2million rental income at the end of each year.Calculate the IRR of his investment.Solution:See Exhibit4.1.2•If the cashﬂows occur more frequently than once a year,such as monthly or quarterly,y computed from(4.1)is the IRR for the payment interval.•Suppose cashﬂows occur m times a year,the nominal IRR in an-nualized term is m·y,while the annual eﬀective rate of return is (1+y)m−1.Example4.3:A cash outlay of$100generates incomes of$20after4 months and8months,and$80after2years.Calculate the IRR of the investment.Solution:If we treat one month as the interest conversion period,the equation of value can be written as100=20(1+y1)4+20(1+y1)8+80(1+y1)24,where y1is the IRR on monthly interval.The nominal rate of return on monthly compounding is12y1.Alternatively,we can use the4-month interest conversion interval,and the equation of value is100=201+y4+20(1+y4)2+80(1+y4)6,where y4is the IRR on4-month interval.The nominal rate of return on4-monthly compounding is3y4.The eﬀective annual rate of return is y=(1+y1)12−1=(1+y4)3−1.The above equations of value have to be solved numerically for y1or y4. We obtain1.0406%as the IRR per month,namely,y1.The eﬀective annualized rate of return is then(1.010406)12−1=13.23%.Solving for y4with Excel,we obtain the answer4.2277%.Hence,the annualized eﬀective rate is(1.042277)3−1=13.23%,which is equal to the eﬀective rate computed using y1.2•When cashﬂows occur irregularly,we can deﬁne y as the annualized rate and express all time of occurrence of cashﬂows in years.•Suppose there are n+1cashﬂows occurring at time0,t1,···,t n, with cash amounts C0,C1,···,C n.Equation(4.2)is rewritten asC0=−nX j=1C j(1+y)t j,(4.3)which requires numerical methods for the solution of y.•We may also use the Excel function XIRR to solve for y.Unlike IRR, XIRR allows the cashﬂows to occur at irregular time intervals.The speciﬁcation of XIRR is as follows:Example4.4:A project requires an outlay of$2.35million in return for$0.8million after9months,$1million after15months and$1million after2years.What is the IRR of the project?Solution:Returns of the project occur at time(in years)0.75,1.25 and2.We solve for v numerically from the following equation using Excel Solver(see Exhibit4.2)235=80v0.75+100v1.25+100v2to obtain v=0.879,so thaty=10.879−1=13.77%,which is the eﬀective annualized rate of return of the project.Alterna-tively,we may use the Excel function XIRR as shown in Exhibit4.3.2•A project with no subsequent investment apart from the initial cap-ital is called a simple project.•For simple projects,(4.1)has a unique solution with y>−1,so that IRR is well deﬁned.Example4.5:A project requires an initial outlay of$8million,gen-erates returns of$50million1year later,and requires$50million to terminate at the end of year2.Solve for y in(4.1).Solution:We are required to solve8=50v−50v2,which has v=0.8and0.2as solutions.This implies y has multiple solu-tions of25%and400%.24.2One-Period Rate of Return•We consider methods of calculating the return of a fund over a1-period interval.The methodology adopted depends on the data available.•We start with the situation where the exact amounts of fund with-drawals and injections are known,as well as the time of their occur-rence.•Consider a1-year period with initial fund amount B0(equal to C0). Cashﬂow of amount C j occurs at time t j(in fraction of a year)for j=1,···,n,where0<t1<···<t n<1.•Note that C j are usually fund redemptions and new investments,and do not include investment incomes such as dividends and couponpayments.•Denoting the fund value before and after the transaction at time t j by B B j and B A j,respectively,we have B A j=B B j+C j for j=1,···,n.•The diﬀerence between B B j and B A j−1,i.e.,the balance before the transaction at time t j and after the transaction at time t j−1,is due to investment incomes,as well as capital gains and losses.•Let the fund balance at time1be B1,and deﬁne B B n+1=B1andB A0=B0(this notation will allow us to express the gross return as(4.4)below).Figure4.1illustrates the time diagram.•We now introduce two methods to calculate the1-year rate of re-turn:the time-weighted rate of return(TWRR)and the dollar-weighted rate of return(DWRR).•To compute the TWRR weﬁrst calculate the return over each subin-terval between the occurrences of transactions by comparing the fund balances just before the new transaction to the fund balance just af-ter the last transaction.•If we denote R j as the rate of return over the subinterval t j−1to t j,we have1+R j=B B jB j−1,for j=1,···,n+1.(4.4)•Then TWRR over the year,denoted by R T,isR T=⎡⎣n+1Y j=1(1+R j)⎤⎦−1.(4.5)•The TWRR requires data of the fund balance prior to each with-drawal or injection.In contrast,the DWRR does not require thisinformation.It only uses the information of the amounts of the withdrawals and injections,as well as their time of occurrence.•In principle,when cash of amount C j is injected(withdrawn)at time t j,there is a gain(loss)of capital of amount C j(1−t j)for the remaining period of the year.•Thus,the eﬀective capital of the fund over the1-year period,denoted by B,is given byB=B0+nX j=1C j(1−t j).•Denoting C=P n j=1C j as the net injection of cash(withdrawal if negative)over the year and I as the interest income earned over the year,we have B1=B0+I+C,so thatI=B1−B0−C.(4.6)Hence the DWRR over the1-year period,denoted by R D,isR D=IB=B1−B0−CB0+P n j=1C j(1−t j).(4.7)Example4.6:On January1,a fund was valued at100k(1k=1,000). On May1,the fund increased in value to112k and30k of new principal was injected.On November1,the fund value dropped to125k,and42k was withdrawn.At the end of the year,the fund was worth100k.Calculate the DWRR and the TWRR.Solution:As C=30−42=−12,there is a net withdrawal.From (4.6),the interest income earned over the year isI=100−100−(−12)=12.Hence,from (4.7),the DWRR isR D =12100+23×30−16×42=10.62%.The fund balance just after the injection on May 1is 112+30=142k,and its value just after the withdrawal on November 1is 125−42=83k.From (4.4),the fund-value relatives over the three subperiods are1+R 1=112100=1.120,1+R 2=125142=0.880,1+R 3=10083=1.205.Hence,from (4.5),the TWRR isR T =1.120×0.880×1.205−1=18.76%.2•TWRR compounds the returns of the fund over subperiods after purging the eﬀects of the timing and amount of cash injections and withdrawals.•As fund managers have no control over the timing of fund injection and withdrawal,the TWRR appropriately measures the performance of the fund manager.•DWRR is sensitive to the timing and amount of the cashﬂows.•If the purpose is to measure the performance of the fund,the DWRR is appropriate.•It allows superior market timing to impact the return of the fund.•For funds with frequent cash injections and withdrawals,the com-putation of the TWRR may not be feasible.The diﬃculty lies in the evaluation of the fund value B B j,which requires the fund to be constantly marked to market.•In some situations the exact timing of the cashﬂows may be diﬃcult to identify.•In this situation,we may approximate(4.7)by assuming the cash ﬂows to be evenly distributed throughout the1-year evaluation pe-riod.•Hence,we replace1−t j by its mean value of0.5so that P n j=1C j(1−t j)=0.5C,and(4.7)can be written asR D'IB0+0.5C=IB0+0.5(B1−B0−I))=I0.5(B1+B0−I).(4.8)Example4.7:For the data in Example4.6,calculate the approximate value of the DWRR using(4.8).Solution:With B0=B1=100,and I=12,the approximate R D isR D=120.5(100+100−12)=12.76%.24.3Rate of Return over Multiple Periods •We now consider the rate of return of a fund over a m-year period.•Weﬁrst consider the case where only annual data of returns are available.Suppose the annual rates of return of the fund have been computed as R1,···,R m.Note that−1≤R j<∞for all j.•The average return of the fund over the m-year period can be cal-culated as the mean of the sample values.We call this measure the arithmetic mean rate of return,denoted by R A,which is givenbyR A=1mmX j=1R j.(4.9)•An alternative is to use the geometric mean to calculate the average, called the geometric mean rate of return,denoted by R G,whichis given byR G=⎡⎣m Y j=1(1+R j)⎤⎦1m−1=[(1+R1)(1+R2)···(1+R m)]1m−1.(4.10)Example4.8:The annual rates of return of a bond fund over the last 5years are(in%)as follows:6.48.92.5−2.17.2Calculate the arithmetic mean rate of return and the geometric mean rate of return of the fund.Solution:The arithmetic mean rate of return isR A=(6.4+8.9+2.5−2.1+7.2)/5=22.9/5=4.58%,and the geometric mean rate of return isR G=(1.064×1.089×1.025×0.979×1.072)15−1=(1.246)15−1=4.50%.2 Example4.9:The annual rates of return of a stock fund over the last 8years are(in%)as follows:15.218.7−6.9−8.223.2−3.916.91.8 Calculate the arithmetic mean rate of return and the geometric mean rate of return of the fund.Solution:The arithmetic mean rate of return isR A=(15.2+18.7+···+1.8)/8=7.10%and the geometric mean rate of return isR G=(1.152×1.187×···×1.018)1−1=6.43%.2•Given any sample of data,the arithmetic mean is always larger than the geometric mean.•If the purpose is to measure the return of the fund over the holding period of m years,the geometric mean rate of return is the appro-priate measure.•The arithmetic mean rate of return describes the average perfor-mance of the fund for one year taken randomly from the sample period.•If there are more data about the history of the fund,alternative mea-sures of the performance of the fund can be used.The methodology of the time-weighted rate of return in Section4.2can be extended to beyond1period(year).•Suppose there are n subperiods,with returns denoted by R1,···, R n,over a period of m years.Then we can measure the m-year return by compounding the returns over each subperiod to form the TWRR using the formulaR T=⎡⎣n Y j=1(1+R j)⎤⎦1−1.(4.11)•We can also compute the return over a m-year period using the IRR. We extend the notations for cashﬂows in Section4.2to the m-year period.•Suppose cashﬂows of amount C j occur at time t j for j=1,···,n, where0<t1<···<t n<m.Let the fund value at time0and time m be B0and B1,respectively.We treat−B1as the last transaction, i.e.,fund withdrawal of amount B1.•The rate of return of the fund is calculated as the IRR which equates the discounted values of B0,C1,···,C n,and−B1to zero.•This is referred to as the DWRR over the m-year period.We denote it as R D,which solves the following equationB0+nX j=1C j(1+R D)t j−B1(1+R D)m=0.(4.12)•The example below concerns the returns of a bond fund.When a bond makes the periodic coupon payments,the bond values drop and the coupons are cash amounts to be withdrawn from the fund.Example4.10:A bond fund has an initial value of$20million.The fund records coupon payments in six-month periods.Coupons received from January1through June30are regarded as paid on April1.Likewise, coupons received from July1through December31are regarded as paid on October1.For the2-year period2008and2009,the fund values and coupon payments were recorded in Table4.1.Table4.1:Cashﬂows of fundTime Coupon received Fund value beforemm/dd/yy($millions)date($millions)01/01/0820.004/01/080.8022.010/01/08 1.0222.804/01/090.9721.910/01/090.8523.512/31/0925.0Calculate the TWRR and the DWRR of the fund.Solution:As the coupon payments are withdrawals from the fund(the portfolio of bonds),the fund drops in value after the coupon payments. For example,the bond value drops to22.0−0.80=21.2million on April 1,2008after the coupon payments.Thus,the TWRR is calculated asR T=∙2220×22.8022.0−0.8×21.9022.80−1.02×23.5021.90−0.97×25.023.50−0.85¸0.5−1=21.42%.To calculate the DWRR we solve R D from the following equation20=0.8(1+R D)+1.02(1+R D)+0.97(1+R D)+0.85(1+R D)+25(1+R D)=0.8v+1.02v3+0.97v5+0.85v7+25v8,where v=(1+R D)−0.25.We let(1+y)−1=v and use Excel to obtain y= 4.949%,so that the annual eﬀective rate of return is R D=(1.04949)4−1= 21.31%.24.4Portfolio Return•We now consider the return of a portfolio of assets.•Suppose a portfolio consists of N assets denoted by A1,···,A N.Let the value of asset A j in the portfolio at time0be A0j,for j= 1,···,N.•We allow A0j to be negative for some j,so that asset A j is sold short in the portfolio.•The portfolio value at time0is B0=P N j=1A0j.Let the asset values at time1be A1j,so that the portfolio value is B1=P N j=1A1j.•Denote R P as the return of the portfolio in the period from time0to time1.Thus,R P=B1−B0B0=B1B0−1.•We deﬁnew j=A0j B0,which is the proportion of the value of asset A j in the initial portfolio, so thatNX j=1w j=1,and w j<0if asset j is short sold in the portfolio.•We also denoteR j=A1j−A0jA0j=A1jA0j−1,which is the rate of return of asset j.Thus,1+R P=B1 B0=1B0N X j=1A1j=NX j=1A0j B0×A1j A0j=NX j=1w j(1+R j),which impliesR P=NX j=1w j R j,(4.13)so that the return of the portfolio is the weighted average of the returns of the individual assets.•Eq(4.13)is an identity,and applies to realized returns as well as returns as random variables.If we take the expectations of(4.13),we obtainE(R P)=NX j=1w j E(R j),(4.14)so that the expected return of the portfolio is equal to the weighted average of the expected returns of the component assets.•The variance of the portfolio return is given byVar(R P)=NX j=1w2j Var(R j)+N X h=1N X j=1|{z}h=jw h w j Cov(R h,R j).(4.15)•For example,consider a portfolio consisting of two funds,a stock fund and a bond fund,with returns denoted by R S and R B,respec-tively.Likewise,we use w S and w B to denote their weights in the portfolio.•Then,we haveE(R P)=w S E(R S)+w B E(R B),(4.16)andVar(R P)=w2S Var(R S)+w2B Var(R B)+2w S w B Cov(R S,R B),(4.17)where w S+w B=1.Example4.11:A stock fund has an expected return of0.15and variance of0.0625.A bond fund has an expected return of0.05and variance of0.0016.The correlation coeﬃcient between the two funds is −0.2.(a)What is the expected return and variance of the portfolio with80%in the stock fund and20%in the bond fund?(b)What is the expected return and variance of the portfolio with20%in the stock fund and80%in the bond fund?(c)How would you weight the two funds in your portfolio so that yourportfolio has the lowest possible variance?Solution:For(a),we use(4.16)and(4.17),with w S=0.8and w B= 0.2,to obtainE(R P)=(0.8)(0.15)+(0.2)(0.05)=13%Var(R P)=(0.8)2(0.0625)+(0.2)2(0.0016)+2(0.8)(0.2)(−0.2)q(0.0016)(0.0625) =0.03942.√0.03942=19.86%. Thus,the portfolio has a standard deviation ofFor(b),we do similar calculations,with w S=0.2and w B=0.8,toobtain E(R P)=7%,Var(R P)=0.002884and a standard deviation of √0.002884=5.37%.Hence,we observe that the portfolio with a higher weightage in stock has a higher expected return but also a higher standard deviation,i.e.,higher risk.For(c)we rewrite(4.17)asVar(R P)=w2S Var(R S)+(1−w S)2Var(R B)+2w S(1−w S)Cov(R S,R B). To minimize the variance,we diﬀerentiate Var(R P)with respect to w S to obtain2w S Var(R S)−2(1−w S)Var(R B)+2(1−2w S)Cov(R S,R B).Equating the above to zero,we solve for w S to obtainw S=Var(R B)−Cov(R S,R B)Var(R B)+Var(R S)−2Cov(R S,R B)=5.29%.The expected return of this portfolio is5.53%,its variance is0.001410, and its standard deviation is3.75%,which is lower than the standard deviation of the bond fund of4%.Hence,this portfolio dominates the bond fund,in the sense that it has a higher expected return and a lower standard deviation.Note that the fact that the above portfolio indeed minimizes the variance can be veriﬁed by examining the second-order condition.24.5Short sales•A short sale is the sale of a security that the seller does not own.It can be executed through a margin account with a brokerageﬁrm.•The seller borrows the security from the brokerageﬁrm to deliver to the buyer.•The sale is based on the belief that the security price will go down in the future so that the seller will be able to buy back the security at a lower price,thus keeping the diﬀerence in price as proﬁt.•Proceeds from the short sale are kept in the margin account,and cannot be invested to earn income.•The seller is required to place cash or securities into the margin account.The initial percentage margin m is the percentage ofthe proceeds of the short sold security that the seller must place into the margin account.•If P0is the price of the security when it is sold short,the initial deposit D is P0m.The seller will earn interest from the deposit.•At any point in time,there is a maintenance margin m∗,which is the minimum percentage of the seller’s equity in relation to the current value of the security sold short.•If the current security price is P1,the equity E is P0+D−P1and E/P1must be larger than m∗.•If E/P1falls below m∗,the seller will get a margin call from the broker instructing him to top up his margin account.Example4.12:A person sold1,000shares of a stock short at$20. If the initial margin is50%,how much should he deposit in his margin account?If the maintenance margin is30%,how high can the price go up before there is a margin call?Solution:The initial deposit is1,000×20×0.5=$10,000.At price P1,the percent of equity is10,000+1,000(20−P1),1,000P1which must be more than0.3.Thus,the maximum P1is30=$23.08.1.32•To calculate the rate of return of a short sale strategy we note that the capital is the deposit D in the margin account.The return includes the interest earned in the deposit.The seller,however, pays the dividend to the buyer if there is any dividend payout.The net rate of return will thus take account of the interest earned and the dividend paid.Example4.13:A person sells a stock short at$30.The stock pays a dividend of$1at the end of the year,after which the man covers his short position by buying the stock back at$27.The initial margin is50%and interest rate is4%.What is his rate of return over the year? Solution:The capital investment per share is$15.The gross return after one year is(30−27)−1+15×0.04=$2.6.Hence,the return overthe1-year period is2.6=17.33%.15Note that in the above calculation we have assumed that there was no margin call throughout the year.24.6Crediting Interest:Investment-Y ear Method andPortfolio Method•A fund pools the investments of individual investors.The investors may invest new money into the fund at any time.•While the fund invests the aggregate of the investments,there is an issue of how to credit interest to the individual investors’accounts.•A simple method is to credit the average return of the fund to all investors.This is called the portfolio method.•The portfolio method may not be equitable when the individual investments are made at diﬀerent times.•For example,at a time when returns to securities are going up,new investments are likely to achieve higher returns compared to old。

第1章 Introductory Econometrics for Finance(金融计量经济学导论-东北财经大学陈磊)

1.2 The Special Characteristics of Financial Data
• 宏观经济计量分析的数据问题：
• 小样本；测量误差与数据修正
1-13
• 金融数据的观测频率高，数据量大 • 金融数据的质量高
这些意味着可以采用更强有力的分析技术，研究结果也更可靠。
• 金融数据包含很多噪音（noisy），更难以从随机的和无关的变动中分辨出趋势和规律 • 通常不满足正态分布 • 高频数据经常包含反映市场运行方式的、但人们并不感兴趣的其它模式(pattern) ，需要在建模时加以考虑
1-15
Types of Data
• Problems that Could be Tackled Using a Time Series Regression - How the value of a country’s stock index has varied with that country’s macroeconomic fundamentals. - How the value of a company’s stock price has varied when it announced the value of its dividend payment. - The effect on a country’s currency of an increase in its interest rate • Cross-sectional data(截面数据) are data on one or more variables collected at a single point in time, e.g. - Cross-section of stock returns on the New York Stock Exchange - A sample of bond credit ratings for UK banks

货币金融学(第十二版)英文版题库及答案chapter 13

Economics of Money, Banking, and Financial Markets, 12e (Mishkin)Chapter 13 Central Banks and the Federal Reserve System13.1 Origins of the Federal Reserve System1) The First Bank of the United StatesA) was disbanded in 1811 when its charter was not renewed.B) had its charter renewal vetoed in 1832.C) was fundamental in helping the Federal Government finance the War of 1812.D) None of the above.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking2) The Second Bank of the United StatesA) was disbanded in 1811 when its charter was not renewed.B) had its charter renewal vetoed in 1832.C) is considered to be the primary cause of the bank panic of 1907.D) None of the above.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking3) The public's fear of centralized power and distrust of moneyed interests led to the demise of the first two experiments in central banking, otherwise known asA) the First Bank of the United States and the Second Bank of the United States.B) the First Bank of the United States and the Central Bank of the United States.C) the First Central Bank of the United States and the Second Central Bank of the United States.D) the First Bank of North America and the Second Bank of North America.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking4) The financial panic of 1907 resulted in such widespread bank failures and substantial losses to depositors that the American public finally became convinced thatA) the First Bank of the United States had failed to serve as a lender of last resort.B) the Second Bank of the United States had failed to serve as a lender of last resort.C) the Federal Reserve System had failed to serve as a lender of last resort.D) a central bank was needed to prevent future panics.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking5) What makes the Federal Reserve so unique compared to other central banks around the world is itsA) centralized structure.B) decentralized structure.C) regulatory functions.D) monetary policy functions.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking13.2 Structure of the Federal Reserve System1) Which of the following is NOT an entity of the Federal Reserve System?A) Federal Reserve BanksB) the Comptroller of the CurrencyC) the Board of GovernorsD) the Federal Open Market CommitteeAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking2) Which of the following is an entity of the Federal Reserve System?A) the U.S. Treasury SecretaryB) the FOMCC) the Comptroller of the CurrencyD) the FDICAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking3) The three largest Federal Reserve banks (New York, Chicago, and San Francisco) combined hold more than ________ percent of the assets of the Federal Reserve System.A) 25B) 33C) 50D) 67Answer: CQues Status: Previous EditionAACSB: Analytical Thinking4) The Federal Reserve Banks are ________ institutions since they are owned by the ________.A) quasi-public; private commercial banks in the district where the Reserve Bank is locatedB) public; private commercial banks in the district where the Reserve Bank is locatedC) quasi-public; Board of GovernorsD) public; Board of GovernorsAnswer: AQues Status: Previous EditionAACSB: Reflective Thinking5) Each Federal Reserve bank has nine directors. Of these ________ are appointed by the member banks and ________ are appointed by the Board of Governors.A) three; sixB) four; fiveC) five; fourD) six; threeAnswer: DQues Status: Previous EditionAACSB: Reflective Thinking6) The nine directors of the Federal Reserve Banks are split into three categories: ________ are professional bankers, ________ are leaders from industry, and ________ are to represent the public interest and are not allowed to be officers, employees, or stockholders of banks.A) 5; 2; 2B) 2; 5; 2C) 4; 2; 3D) 3; 3; 3Answer: DQues Status: Previous EditionAACSB: Reflective Thinking7) Member commercial banks have purchased stock in their district Fed banks; the dividend paid by that stock is limited by law to ________ percent annually.A) fourB) fiveC) sixD) eightAnswer: CQues Status: Previous EditionAACSB: Reflective Thinking8) The Federal Reserve Bank of ________ houses the open market desk.A) BostonB) New YorkC) ChicagoD) San FranciscoAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking9) The president from which Federal Reserve Bank always has a vote in the Federal Open Market Committee?A) PhiladelphiaB) BostonC) San FranciscoD) New YorkAnswer: DQues Status: Previous EditionAACSB: Reflective Thinking10) An important function of the regional Federal Reserve Banks isA) setting reserve requirements.B) clearing checks.C) determining monetary policy.D) setting margin requirements.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking11) Which of the following functions is NOT performed by any of the twelve regional Federal Reserve Banks?A) check clearingB) conducting economic researchC) setting interest rates payable on time depositsD) issuing new currencyAnswer: CQues Status: Previous EditionAACSB: Reflective Thinking12) All ________ are required to be members of the Fed.A) state chartered banksB) national banks chartered by the Office of the Comptroller of the CurrencyC) banks with assets less than $100 millionD) banks with assets less than $500 millionAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking13) Of all commercial banks, about ________ belong to the Federal Reserve System.A) 10%B) one halfC) one thirdD) 90%Answer: CQues Status: Previous EditionAACSB: Reflective Thinking14) Prior to 1980, member banks left the Federal Reserve System due toA) the high cost of discount loans.B) the high cost of required reserves.C) a desire to avoid interest rate regulations.D) a desire to avoid credit controls.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking15) The Fed's support of the Depository Institutions Deregulation and Monetary Control Act of 1980 stemmed in part from itsA) concern over declining Fed membership.B) belief that all banking regulations should be eliminated.C) belief that interest rate ceilings were too high.D) belief that depositors had to become more knowledgeable of banking operations. Answer: AQues Status: Previous EditionAACSB: Reflective Thinking16) Banks subject to reserve requirements set by the Federal Reserve System includeA) only nationally chartered banks.B) only banks with assets less than $100 million.C) only banks with assets less than $500 million.D) all banks whether or not they are members of the Federal Reserve System.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking17) The Depository Institutions Deregulation and Monetary Control Act of 1980A) established higher reserve requirements for nonmember than for member banks.B) established higher reserve requirements for member than for nonmember banks.C) abolished reserve requirements.D) established uniform reserve requirements for all banks.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking18) There are ________ members of the Board of Governors of the Federal Reserve System.A) 5B) 7C) 12D) 19Answer: BQues Status: Previous EditionAACSB: Reflective Thinking19) Members of the Board of Governors areA) chosen by the Federal Reserve Bank presidents.B) appointed by the newly elected president of the United States, as are cabinet positions.C) appointed by the president of the United States and confirmed by the Senate.D) never allowed to serve more than 7-year terms.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking20) Each governor on the Board of Governors can serveA) only one nonrenewable fourteen-year term.B) one full nonrenewable fourteen-year term plus part of another term.C) only one nonrenewable eight-year term.D) one full nonrenewable eight-year term plus part of another term.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking21) The Chairman of the Board of Governors is chosen from among the seven governors and serves a ________, renewable term.A) one-yearB) two-yearC) four-yearD) eight-yearAnswer: CQues Status: Previous EditionAACSB: Reflective Thinking22) While the discount rate is "established" by the regional Federal Reserve Banks, in truth, the rate is determined byA) Congress.B) the president of the United States.C) the Senate.D) the Board of Governors.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking23) Which of the followings is a duty of the Board of Governors of the Federal Reserve System?A) setting margin requirements, the fraction of the purchase price of the securities that has to be paid for with cashB) setting the maximum interest rates payable on certain types of time deposits under Regulation QC) regulating credit with the approval of the president under the Credit Control Act of 1969D) All governors advise the president of the United States on economic policy.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking24) Which of the followings is NOT a current duty of the Board of Governors of the Federal Reserve System?A) setting margin requirements, the fraction of the purchase price of the securities that has to be paid for with cashB) setting the maximum interest rates payable on certain types of time deposits under Regulation QC) approving the discount rate "established" by the Federal Reserve banksD) voting on the conduct of open market operationsAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking25) The Federal Open Market Committee usually meets ________ times a year.A) fourB) sixC) eightD) twelveAnswer: CQues Status: Previous EditionAACSB: Reflective Thinking26) The Federal Reserve entity that makes decisions regarding the conduct of open market operations is theA) Board of Governors.B) chairman of the Board of Governors.C) Federal Open Market Committee.D) Open Market Advisory Council.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking27) The Federal Open Market Committee consists of theA) five senior members of the seven-member Board of Governors.B) seven members of the Board of Governors and seven presidents of the regional Fed banks.C) seven members of the Board of Governors and five presidents of the regional Fed banks.D) twelve regional Fed bank presidents and the chairman of the Board of Governors. Answer: CQues Status: Previous EditionAACSB: Reflective Thinking28) The majority of members of the Federal Open Market Committee areA) Federal Reserve Bank presidents.B) members of the Federal Advisory Council.C) presidents of member banks.D) the seven members of the Board of Governors.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking29) Each Fed bank president attends FOMC meetings; although only ________ Fed bank presidents vote on policy, all ________ provide input.A) three; tenB) five; tenC) three; twelveD) five; twelveAnswer: DQues Status: Previous EditionAACSB: Reflective Thinking30) Although reserve requirements and the discount rate are not actually set by the ________, decisions concerning these policy tools are effectively made there.A) Federal Reserve Bank of New YorkB) Board of GovernorsC) Federal Open Market CommitteeD) Federal Reserve BanksAnswer: CQues Status: Previous EditionAACSB: Reflective Thinking31) The research document given to the Federal Open Market Committee that contains information on the state of the economy in each Federal Reserve district is called theA) beige book.B) green book.C) blue book.D) black book.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking32) The teal book is the Fed research document containingA) the forecast of national economic variables for the next three years.B) forecasts of the money aggregates conditional on different monetary policy stances.C) information on the state of the economy in each Federal Reserve district.D) both A and B.E) A, B and C.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking33) The Federal Open Market Committee's "balance of risks" is an assessment of whether, in the future, its primary concern will beA) higher exchange rates or higher unemployment.B) higher inflation or a stronger economy.C) higher inflation or a weaker economy.D) lower inflation or a stronger economy.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking34) Subject to the approval of the Board of Governors, the decision of choosing the president ofa district Federal Reserve Bank is made byA) all nine district bank directors.B) the six district bank directors elected by the member banks.C) three district bank directors who are professional bankers.D) district bank directors who are not professional bankers.E) class A and class B directors.Answer: DQues Status: Previous EditionAACSB: Ethical Understanding and Reasoning Abilities35) Why does the Federal Reserve Bank of New York play a special role within the Federal Reserve System?Answer: The New York district contains the largest banks in the country. The New York Fed supervises and examines these banks to insure their soundness and the safety of the nation's financial system. The New York Fed conducts open market operations and foreign exchange transactions for the Fed and Treasury. The New York Fed belongs to the Bank for International Settlements, so its president and the chairman of the Board of Governors represent the U.S. at the monthly meetings of the world's central banks. The New York Fed president is the only president of a regional Fed who is a permanent voting member of the FOMC.Ques Status: Previous EditionAACSB: Reflective Thinking36) Who are the voting members of the Federal Open Market Committee and why is this committee important? Where does the power lie within this committee?Answer: The FOMC determines the monetary policy of the United States through its decisions about open market operations. It also effectively determines the discount rate and reserve requirements. The seven members of the Board of Governors, the president of the New York Fed, and four of the other eleven regional bank presidents are voting members on a rotating basis. Within the FOMC, the chairman of the Board of Governors wields the power.Ques Status: Previous EditionAACSB: Reflective Thinking13.3 How Independent is the Fed?1) Instrument independence is the ability of ________ to set monetary policy ________.A) the central bank; goalsB) Congress; goalsC) Congress; instrumentsD) the central bank; instrumentsAnswer: DQues Status: Previous EditionAACSB: Reflective Thinking2) The ability of a central bank to set monetary policy instruments isA) political independence.B) goal independence.C) policy independence.D) instrument independence.Answer: DQues Status: Previous EditionAACSB: Reflective Thinking3) Goal independence is the ability of ________ to set monetary policy ________.A) the central bank; goalsB) Congress; goalsC) Congress; instrumentsD) the central bank; instrumentsAnswer: AQues Status: Previous EditionAACSB: Reflective Thinking4) The ability of a central bank to set monetary policy goals isA) political independence.B) goal independence.C) policy independence.D) instrument independence.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking5) Members of Congress are able to influence monetary policy, albeit indirectly, through their ability toA) withhold appropriations from the Board of Governors.B) withhold appropriations from the Federal Open Market Committee.C) propose legislation that would force the Fed to submit budget requests to Congress, as must other government agencies.D) instruct the General Accounting Office to audit the foreign exchange market functions of the Federal Reserve.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking6) Explain two concepts of central bank independence. Is the Fed politically independent? Why do economists think central bank independence is important?Answer: Instrument independence is the ability of the central bank to set its instruments, and goal independence is the ability of a central bank to set its goals. The Fed enjoys both types of independence. The Fed is largely independent of political pressure due to its earnings and the conditions of appointment of the Board of Governors and its chairman. However, some political pressure can be applied through the threat or enactment of legislation affecting the Fed. Independence is important because there is some evidence that independent central banks pursue lower rates of inflation without harming overall economic performance.Ques Status: Previous EditionAACSB: Reflective Thinking13.4 Should the Fed Be Independent?1) The case for Federal Reserve independence does NOT include the idea thatA) political pressure would impart an inflationary bias to monetary policy.B) a politically insulated Fed would be more concerned with long-run objectives and thus be a defender of a sound dollar and a stable price level.C) policy is always performed better by an elite group such as the Fed.D) a Federal Reserve under the control of Congress or the president might make the so-called political business cycle more pronounced.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking2) The political business cycle refers to the phenomenon that just before elections, politicians enact ________ policies. After the elections, the bad effects of these policies (for example,________ ) have to be counteracted with ________ policies.A) expansionary; higher unemployment; contractionaryB) expansionary; a higher inflation rate; contractionaryC) contractionary; higher unemployment; expansionaryD) contractionary; a higher inflation rate; expansionaryAnswer: BQues Status: Previous EditionAACSB: Analytical Thinking3) The strongest argument for an independent Federal Reserve rests on the view that subjecting the Fed to more political pressures would impartA) an inflationary bias to monetary policy.B) a deflationary bias to monetary policy.C) a disinflationary bias to monetary policy.D) a countercyclical bias to monetary policy.Answer: AQues Status: Previous EditionAACSB: Ethical Understanding and Reasoning Abilities4) Critics of the current system of Fed independence contend thatA) the current system is undemocratic.B) voters have too much say about monetary policy.C) the president has too much control over monetary policy on a day-to-day basis.D) the Board of Governors is held responsible for policy missteps.Answer: AQues Status: Previous EditionAACSB: Diverse and Multicultural Work Environments5) Recent research indicates that inflation performance (low inflation) has been found to be best in countries withA) the most independent central banks.B) political control of monetary policy.C) money financing of budget deficits.D) a policy of always keeping interest rates low.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking6) Make the case for and against an independent Federal Reserve.Answer: Case for: 1. An independent Federal Reserve can shield the economy from the political business cycle, and it will be less likely to have an inflationary bias to monetary policy. 2. Control of the money supply is too important to leave to inexperienced politicians.Case against: 1. It is undemocratic to have monetary policy be controlled by a small number of individuals that are not accountable. 2. In the past, an independent Fed has not used its freedom wisely. 3. Its independence may encourage it to pursue its own self-interest rather than the public's interest.Ques Status: Previous EditionAACSB: Ethical Understanding and Reasoning Abilities13.5 Explaining Central Bank Behavior1) The theory of bureaucratic behavior suggests that the objective of a bureaucracy is to maximizeA) the public's welfare.B) profits.C) its own welfare.D) conflict with the executive and legislative branches of government.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking2) The theory of bureaucratic behavior when applied to the Fed helps to explain why the FedA) was supportive of congressional attempts to limit the central bank's autonomy.B) was so secretive about the conduct of future monetary policy.C) sought less control over banks in the 1980s.D) was willing to take on powerful groups that may threaten its autonomy.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking3) What is the theory of bureaucratic behavior and how can it be used to explain the behavior of the Federal Reserve?Answer: The theory of bureaucratic behavior concludes that the main objective of any bureaucracy is to maximize its own welfare, which is related to power and prestige. This can explain why the Federal Reserve has defended its autonomy, avoids conflict with Congress and the president, and its push to gain more control over banks.Ques Status: Previous EditionAACSB: Analytical Thinking13.6 Structure and Independence of the European Central Bank1) Under the European System of Central Banks, the Executive Board is similar in structure to the ________ of the Federal Reserve System.A) Board of GovernorsB) Federal Open Market CommitteeC) Federal Reserve BanksD) Federal Advisory CouncilAnswer: AQues Status: Previous EditionAACSB: Reflective Thinking2) Under the European System of Central Banks, the Governing Council is similar in structure to the ________ of the Federal Reserve System.A) Board of GovernorsB) Federal Open Market CommitteeC) Federal Reserve BanksD) Federal Advisory CouncilAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking3) Under the European System of Central Banks, the National Central Banks have the same role as the ________ of the Federal Reserve System.A) Board of GovernorsB) Federal Open Market CommitteeC) Federal Reserve BanksD) Federal Advisory CouncilAnswer: CQues Status: Previous EditionAACSB: Reflective Thinking4) Members of the Executive Board of the European System of Central Banks are appointed to ________ year, nonrenewable terms.A) fourB) eightC) tenD) fourteenAnswer: BQues Status: Previous EditionAACSB: Reflective Thinking5) Which of the following statements comparing the European System of Central Banks and the Federal Reserve System is TRUE?A) The budgets of the Federal Reserve Banks are controlled by the Board of Governors, while the National Central Banks control their own budgets and the budget of the European Central Bank.B) The European Central Bank has similar power over the National Central Banks when compared to the level of power the Board of Governors has over the Federal Reserve Banks. C) Just like the Federal Reserve System, monetary operations are centralized in the European System of Central Banks with the European Central Bank.D) None of the above.Answer: AQues Status: RevisedAACSB: Reflective Thinking6) The Governing Council usually meets ________ times a year.A) fourB) sixC) eightD) twelveAnswer: DQues Status: Previous EditionAACSB: Reflective Thinking7) In the Governing Council, the decision of what policy to implement is made byA) majority vote of the Executive Board members.B) majority vote of the heads of the National Banks.C) consensus.D) majority vote of all members of the Governing Council.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking8) The central bank which is generally regarded as the most independent in the world because its charter cannot be changed by legislation is theA) Bank of England.B) Bank of Canada.C) European Central Bank.D) Bank of Japan.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking9) Explain the similarities and differences between the European System of Central Banks and the Federal Reserve System.Answer: The similarities between the two are in their structure. The National Central Banks of the member countries of the Eurosystem have the same role as the Federal Reserve Banks in the Federal Reserve System. The Executive Board and the Governing Council of the Eurosystem resemble the Board of Governors and the Federal Open Market Committee of the Federal Reserve System, respectively. There are three major differences between the two. The first difference is concerning the control of the budgets. In the Fed, the Board of Governors controls the budgets of the Reserve Banks while in the Eurosystem, the National Banks control the budget of the European Central Bank. The second difference is the monetary operations of the Eurosystem are conducted by the National Banks, so they are not as centralized as the monetary operations in the Federal Reserve System.Ques Status: RevisedAACSB: Reflective Thinking13.7 Structure and Independence of Other Foreign Central Banks1) On paper, the Bank of Canada has ________ instrument independence and ________ goal independence when compared to the Federal Reserve System.A) less; lessB) less; moreC) more; lessD) more; moreAnswer: AQues Status: Previous EditionAACSB: Reflective Thinking2) The oldest central bank, having been founded in 1694, is theA) Bank of England.B) Deutsche Bundesbank.C) Bank of Japan.D) Federal Reserve System.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking3) While legislation enacted in 1998 granted the Bank of Japan new powers and greater autonomy, its critics contend that its independence isA) limited by the Ministry of Finance's veto power over a portion of its budget.B) too great because it need not pursue a policy of price stability even if that is the popular will of the people.C) too great since the Ministry of Finance no longer has veto power over the bank's budget.D) limited since the Ministry of Finance can dismiss senior bank officials.Answer: AQues Status: Previous EditionAACSB: Reflective Thinking4) Regarding central bank independenceA) the Fed is more independent than the European Central Bank.B) the European Central Bank is more independent than the Fed.C) the trend in industrialized nations has been to reduce central bank independence.D) the Bank of England has the longest tradition of independence of any central bank in the world.Answer: BQues Status: Previous EditionAACSB: Reflective Thinking5) The trend in recent years is that more and more governmentsA) have been granting greater independence to their central banks.B) have been reducing the independence of their central banks to make them more accountable for poor economic performance.C) have mandated that their central banks focus on controlling inflation.D) have required their central banks to cooperate more with their Ministers of Finance. Answer: AQues Status: Previous EditionAACSB: Reflective Thinking6) Which of the following statements about central bank structure and independence is TRUE?A) In recent years, with the exception of the Bank of England and the Bank of Japan, most countries have reduced the independence of their central banks, subjecting them to greater democratic control.B) Before the Bank of England was granted greater independence, the Federal Reserve was the most independent of the world's central banks.C) Both theory and experience suggest that more independent central banks produce better monetary policy.D) While the European Central Bank is independent, it is not as independent as the Federal Reserve.Answer: CQues Status: Previous EditionAACSB: Reflective Thinking。

methods of mathematical finance 金融数学方法

methods of mathematical finance 金融数学方法There are various methods used in mathematical finance to model and analyze financial markets. Some of the key methods include:1. Probability Theory: Mathematical finance heavily relies on probability theory to model uncertain future outcomes. It allows for the calculation of expected values, standard deviations, and other statistical properties of financial variables.2. Stochastic Calculus: Stochastic calculus is used to model and analyze the dynamics of financial variables that follow stochastic processes. It provides tools to derive differential equations and solve them using techniques such as Itô's lemma and stochastic differential equations.3. Option Pricing Theory: Option pricing theory is a fundamental concept in mathematical finance. It includes models like the Black-Scholes model, which determine the fair price of financial derivatives based on underlying asset prices, volatility, time, and other factors.4. Monte Carlo Simulation: Monte Carlo simulation is a popular method used to analyze and value complex financial instruments and portfolios. It involves generating a large number of random simulations to estimate the probability distribution of potential outcomes.5. Optimization Techniques: Optimization techniques are used to find the optimal allocation strategy for investment portfolios. These techniques aim to maximize returns while consideringconstraints such as risk tolerance or liquidity requirements.6. Time Series Analysis: Time series analysis is used to model and analyze financial data that evolves over time. It involves techniques like autoregressive integrated moving average (ARIMA) models and volatility modeling to forecast future prices and evaluate risk.7. Financial Econometrics: Econometrics applies statistical methods to analyze economic and financial data. In mathematical finance, financial econometrics is used to estimate and test various models, validate assumptions, and forecast future market behavior.8. Numerical Methods: Numerical methods like finite difference methods and finite element methods are used to solve complex mathematical equations or partial differential equations that arisein option pricing and risk management.These are just a few of the many methods used in mathematical finance. The field continues to evolve, with new techniques and models constantly being developed to capture the complexities and dynamics of financial markets.。

Mathematical Techniques in Finance (4)

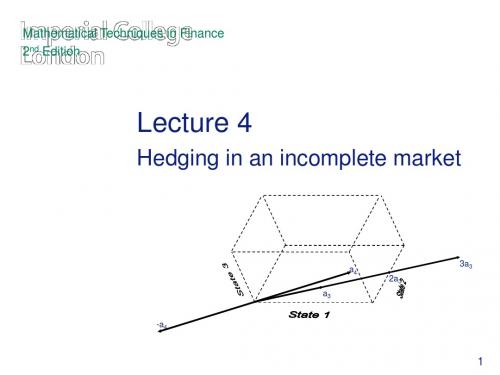
12
Incomplete markets without redundant basis assets (2.1) • Example 2.2 (p. 27): delta hedging
13
Hedging problem with redundant basis assets and incomplete market (2.1)
15
Least Squares Hedge – Example (2.2.1) • Example 2.4 (p. 31)
– Focus asset: b* = [1 2 3] – Basis assets: (A•1)* = [1 1 0]
2
Complete market (1.9, p.14) • If dimension of marketed subspace spanned by basis assets equals the number of scenarios we say market is complete • In a complete market any focus asset which does not require extra scenarios can be hedged perfectly
• Suppose A is invertible (square, full rank)
–Complete market, no redundant basis assets
• Perfect hedge Ax = b: • Multiply by A-1 from the left x = A-1b • Two ways to find the price of focus asset b 1) By replication: Focus asset price = S*x 2) Using a pricing kernel: Focus asset price = ψ*b

《货币金融学(第十三版)》英文版教学课件mishkin_econ13e_ppt_10

The Spread of Government Deposit Insurance Throughout the World: Is This a Good Thing?
• Has government deposit insurance helped improve the performance of the financial system and prevent banking crises? The answer seems to be “no.” Research at the World Bank seems to answer “no,” since on average, the adoption of explicit government deposit insurance is associated with less banking sector stability and a higher incidence of banking crises. Furthermore, on average, deposit insurance seems to retard financial development.
Copyright © 2022, 2019, 2016 Pearson Education, Inc. All Rights Reserved
Types of Financial Regulation: Restrictions on Asset Holdings
• Attempts to restrict financial institutions from too much risk taking: – Bank regulations ▪ Promote diversification ▪ Prohibit holdings of common stock – Capital requirements ▪ Minimum leverage ratio (for banks) ▪ Basel Accord: risk-based capital requirements ▪ Regulatory arbitrage

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

2 3 5
$457.31
Since NPV <0, business venture is not profitable if one considers the time value of money. It would be better to invest the $20,000 in a bank paying 7%, since the venture is equivalent to investing only $20,000 -$457.31= $19,542.69
2
3 5
$10,000
8000 6000
Assume an interest rate of 7% compounded annually and find the net present value of the cash flows.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance 5.2 Present Value
P S1 r
n
Example 1 – Present Value
Find the present value of $1000 due after three years if the interest rate is 9% compounded monthly. Solution: S=1000, r =0.09/12, n =3(12) For interest rate, r 0.09 / 12 0.0075. 36 Principle value is P 10001.0075 $764.15.
INTRODUCTORY MATHEMATICAL ANALYSIS
For Business, Economics, and the Life and Social Sciences
Chapter 5 Mathematics of Finance
2011 Pearson Education, Inc.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance 5.2 Present Value
Net Present Value
Net Present Value NPV Sum of present values - Initial investment
• To solve problems with interest is compounded continuously.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance
Chapter Outline
5.1) Compound Interest 5.2) Present Value 5.3) Interest Compounded Continuously
Chapter 5: Mathematics of Finance
ቤተ መጻሕፍቲ ባይዱ
Chapter Objectives
• To solve interest problems which require logarithms. • To solve problems involving the time value of money.
900 6001.015
n
1.015n 1.5 n ln1.015 ln 1.5
n ln 1.015 ln 1.5 ln 1.5 n 27.233 ln 1.015
It will take
27 .233 4
1 6.8083 6 years,9 2 months .
2011 Pearson Education, Inc.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance
5.1 Compound Interest
• Compound amount S at the end of n interest periods at the periodic rate of r is as
S P1 r
n
Example 1 – Compound Interest
Suppose that $500 amounted to $588.38 in a savings account after three years. If interest was compounded semiannually, find the nominal rate of interest, compounded semiannually, that was earned by the money.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance 5.1 Compound Interest
Example 2 – Compound Interest
How long will it take for $600 to amount to $900 at an annual rate of 6% compounded quarterly? Solution: The periodic rate is r = 0.06/4 = 0.015.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance
5.2 Present Value
• P that must be invested at r for n interest periods so that the present value, S is given by
Example 2 – Net Present Value You can invest $20,000 in a business that guarantees you cash flows at the end of years 2, 3, and 5 as indicated in the table. Year Cash Flow
Example 5 – Net Present Value
Solution: Substracting the initial investment from the sum of the present values of the cash flows gives
NPV 10,0001.07 80001.07 60001.07 20,000
5001 r 588.38 1 r 6 588.38 500
6
588.38 1 r 500
6
r 6
588.38 1 0.0275 500
The semiannual rate was 2.75%, so the nominal rate was 5.5 % compounded semiannually.
2011 Pearson Education, Inc.
Chapter 5: Mathematics of Finance 5.1 Compound Interest
Example 1 – Compound Interest
Solution: Let r be the semiannual rate. There are 2 × 3 = 6 interest periods.