2013国际物理奥林匹克竞赛理论试卷(2)
奥林匹克物理竞赛试题及答案

奥林匹克物理竞赛试题及答案国际奥林匹克物理竞赛是国际中学生的物理大赛,高中同学可以用来提升物理解题能力。
下面店铺给大家带来奥林匹克物理竞赛试题,希望对你有帮助。
奥林匹克物理竞赛试题国际物理奥林匹克竞赛简介竞赛设立由参赛成员国组成的国际物理奥林匹克委员会。
竞赛章程规定:目的是为增进中学物理教学的国际交流,通过竞赛促进开展物理学科的课外活动,以加强不同国家青年之间的友好关系和人民间的相互了解合作。
同时帮助参赛者发展物理方面的创造力,把从学校学到的知识用于解决实际问题的能力。
国际物理奥林匹克竞赛每年举办一次。
由各会员国轮流主办,并由各代表团团长和一名主办国指定的主席组成国际委员会。
国际委员会的任务是公平合理地评卷,监督章程规定的执行情况,决定竞赛结果。
每一会员国可选派5名高中学生或技术学校学生参加竞赛。
参加者的年龄到竞赛开始的那一天不能超过20岁。
参赛代表队要有2名团长,2名团长是国际委员会的成员,条件是能胜任解答赛题,能参加竞赛试卷的讨论和评分工作,并能通晓一种国际物理奥林匹克的工作语言。
国际物理奥林匹克的工作语言是英文、法文、德文和俄文。
代表团到达主办国时,团长要将参加学生及团长的情况告诉主办国家组织人员。
竞赛于每年6月底举行。
竞赛分两天进行。
第一天进行3道理论计算题竞赛,另一天的竞赛内容是1—2道实验题。
中间有一天的休息。
参赛者可使用计算尺、不带程序编制的计算器和对数表、物理常数表和制图工具,但不能使用数学和物理公式一览表。
竞赛题由参加国提供题目,主办国命题。
在竞赛前,赛题要保密。
竞赛题内容包括中学物理的4个部分(力学、热力学和分子物理学、光学及原子和核物理学、电磁学) ,解题要求用标准的中等数学而不要用高等数学。
主办国提出评卷标准并指定评卷人。
每题满分为10分。
各代表团团长同时对自己团员竞赛卷的复制品进行评定,最后协商决定成绩。
评奖标准是以参赛者前三名的平均分数计为100%,参赛者达90% 以上者为一等奖,78—90%者为二等奖,65—78%者为三等奖,同时发给证书。
2013-2014年物理奥林匹克竞赛国家集训队 热学练习题答案解析汇总

2013-2014年物理奥林匹克竞赛国家集训队热学练习题姓名:所在中学:成绩:注意:必须写出完整步骤,否则得不到步骤分。
答卷请勿涂改,无法看清的地方一律不给分。
1、(12分)一端开口,横截面积处处相等的长管中充有压强p的空气。
先对管子加热,使它形成从开口端温度1000K均匀变为闭端200K的温度分布,然后把管子开口端密封,再使整体温度降为100K,试问管中最后的压强是多大?2、(12分)一容积为1 升的容器,盛有温度为300 K,压强为30⨯10Pa的氩气,氩的摩尔质量为0.040 kg。
若器壁上有一面积为1.0×10㎝的小孔,氩气将通过小孔从容器内逸出,经过多长时间容器里的原子数减少为原有原子数的 1/e?3、(12分)若认为地球的大气是等温的, 则把所有大气分子压缩为一层环绕地球表面的、压强为一个大气压的厚度为H的均匀气体球壳,试证:这层球壳厚度H 就是大气标高。
4、(12分)标准状态下氦气的粘度为η1,氩气的粘度为η2,他们的摩尔质量分别为M1和M2.。
试问:(1)氦原子和氦原子碰撞的碰撞截面σ1和氩原子与氩原子的碰撞截面σ2之比等于多少?(2)氦的导热系数κ1与氩的导热系数κ2之比等于多少?(3)氦的扩散系数D1与氩的扩散系数D2之比等于多少?(4)此时测得氦气的粘度-3 24η1=1.87⨯10-3N⋅s⋅m-2和氩气的粘度η2=2.11⨯10-3N⋅s⋅m-2。
用这些数据近似的估算碰撞截面σ1,σ2。
5、(12分)在热水瓶里灌进质量为m=1.00 kg的水,热水瓶胆的内表面S=700 cm,瓶胆内外容器的间隙d=5.00 mm,间隙内气体压强p=1.00 Pa,假设热水瓶内的热量只是通过间隙内的气体的热传导而散失。
试确定需要多少时间容器内的水温从90℃降为80℃,取环境温度为20℃。
6、 (12分)加热室A(1000C)中蒸发出来的铍原子(相对原子质量为9)经小孔逸出,再经狭缝准直器B而形成原子束,最后进入另一真空室D中,(1)原子束将与真空室背景分子进行碰撞,若进行1m后其原子束强度(单位时间内通过的原子数)减少为1/e。
2013年八年级上册物理竞赛题含答案(1-3章)
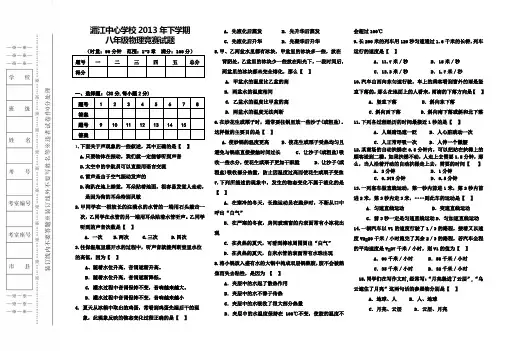
湄江中心学校2013年下学期八年级物理竞赛试题(时量:90分钟范围:1-3章满分:100分)一、选择题:(30分,每小题2分)1.下面关于声现象的一些叙述,其中正确的是【】A.只要物体在振动,我们就一定能够听到声音B.太空中的宇航员可以直接用语言交流C.雷声是由于空气振动发声的D.狗趴在地上睡觉,耳朵贴着地面,很容易发觉人走动,是因为狗的耳朵特别灵敏2.甲同学在一根较长的注满水的水管的一端用石头敲击一次,乙同学在水管的另一端用耳朵贴着水管听声。
乙同学听到的声音次数是【】A.一次 B.两次 C.三次 D.四次3.往保温瓶里灌开水的过程中,听声音就能判断壶里水位的高低,因为【】A、随着水位升高,音调逐渐升高。
B.随着水位升高,音调逐渐降低。
C. 灌水过程中音调保持不变,音响越来越大。
D.灌水过程中音调保持不变,音响越来越小4. 夏天从冰箱中取出的鸡蛋,常看到鸡蛋先湿后干的现象,此现象反映的物态变化过程正确的是【】A. 先液化后蒸发B. 先升华后蒸发C. 先液化后升华D. 先凝华后升华5.甲、乙两盆水里都有冰块,甲盆里的冰块多一些,放在背阴处,乙盆里的冰块少一些放在阳光下,一段时间后,两盆里的冰块都未完全熔化,那么【】A. 甲盆水的温度比乙盆的高B. 两盆水的温度相同C. 乙盆水的温度比甲盆的高D. 两盆水的温度无法判断6.在炒花生或栗子时,通常要往锅里放一些沙子(或粗盐)。
这样做的主要目的是【】A.使炒锅的温度更高 B.使花生或栗子受热均匀且避免与锅底直接接触时间过长 C.让沙子(或粗盐)吸收一些水分,使花生或栗子更加干酥脆 D.让沙子(或粗盐)吸收部分热量,防止因温度过高而使花生或栗子变焦7.下列所描述的现象中,发生的物态变化不属于液化的是【】A.在寒冷的冬天,长跑运动员在跑步时,不断从口中呼出“白气”B.在严寒的冬夜,房间玻璃窗的内表面常有小冰花出现C.在炎热的夏天,可看到棒冰周围冒出“白气”D.在炎热的夏天,自来水管的表面常有水珠出现8.将小锅放入盛有水的大锅中构成双层锅熬胶,胶不会被熬焦而失去粘性,是因为【】A.夹层中的水起了散热作用B.夹层中的水不善于传热C.夹层中的水吸收了很大部分热量D.夹层中的水温度保持在100℃不变,使胶的温度不会超过100℃9.长200米的列车用120秒匀速通过1.6千米的长桥,列车运行的速度是【】A.11.7米/秒 B.15米/秒C.13.3米/秒 D.1.7米/秒10.汽车由西向东匀速行驶,车上的乘客看到窗外的雨是竖直下落的,那么在地面上的人看来,雨滴的下落方向是【】A.竖直下落B.斜向东下落C.斜向西下落D.斜向南下落或斜和北下落11.下列各过程经历的时间最接近1秒的是【】A.人眼睛迅速一眨 B.人心脏跳动一次C.人正常呼吸一次 D.人伸一个懒腰12.某商场的自动扶梯在0.5分钟内,可以把站在扶梯上的顾客送到二楼。
2013物理竞赛及答案
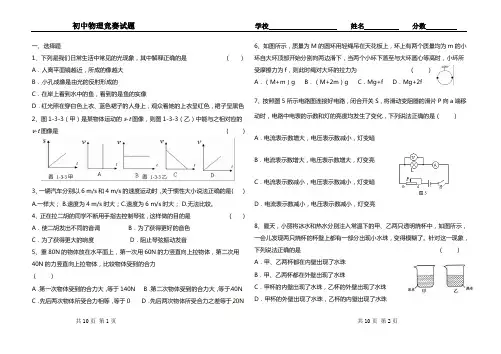
共10页 第1页共10页 第2页一、选择题1、下列是我们日常生活中常见的光现象,其中解释正确的是 ( ) A .人离平面镜越近,所成的像越大 B .小孔成像是由光的反射形成的C .在岸上看到水中的鱼,看到的是鱼的实像D .红光照在穿白色上衣、蓝色裙子的人身上,观众看她的上衣呈红色,裙子呈黑色 2、图1-3-3(甲)是某物体运动的s -t 图像,则图1-3-3(乙)中能与之相对应的v -t 图像是 ( )3、一辆汽车分别以6 m/s 和4 m/s 的速度运动时,关于惯性大小说法正确的是( ) A.一样大; B.速度为4 m/s 时大;C.速度为6 m/s 时大; D.无法比较。
4、正在拉二胡的同学不断用手指去控制琴弦,这样做的目的是 ( ) A .使二胡发出不同的音调 B .为了获得更好的音色 C .为了获得更大的响度 D .阻止琴弦振动发音5、重80N 的物体放在水平面上,第一次用60N 的力竖直向上拉物体,第二次用40N 的力竖直向上拉物体,比较物体受到的合力 ( ) A .第一次物体受到的合力大,等于140N B .第二次物体受到的合力大,等于40N C .先后两次物体所受合力相等,等于0 D .先后两次物体所受合力之差等于20N6、如图所示,质量为M 的圆环用轻绳吊在天花板上,环上有两个质量均为m 的小环自大环顶部开始分别向两边滑下,当两个小环下落至与大环圆心等高时,小环所受摩擦力为f ,则此时绳对大环的拉力为 ( ) A .(M+m )g B .(M+2m )g C .Mg+f D .Mg+2f7、按照图5所示电路图连接好电路,闭合开关S ,将滑动变阻器的滑片P向a端移动时,电路中电表的示数和灯的亮度均发生了变化,下列说法正确的是( )A .电流表示数增大,电压表示数减小,灯变暗B .电流表示数增大,电压表示数增大,灯变亮C .电流表示数减小,电压表示数减小,灯变暗D .电流表示数减小,电压表示数减小,灯变亮8、夏天,小丽将冰水和热水分别注入常温下的甲、乙两只透明烧杯中,如图所示,一会儿发现两只烧杯的杯壁上都有一部分出现小水珠,变得模糊了。
国际物理奥林匹克竞赛试题.doc

国际物理奥林匹克竞赛试题理论试题题1A 某蹦迪运动员系在一根长弹性绳子的一端,绳的另一端固定在一座高桥上,他自静止高桥向下面的河流下落,末与水面相触,他的质量为m,绳子的自然长度为L,绳子的力常数(使绳子伸长lm所需的力)为k,重力场强度为g。
求出下面各量的表达式。
(a)运动员在第一次达到瞬时静止前所落下的距离y。
(b)他在下落过程中所达到的最大速率v。
(c)他在第一次达到瞬时静止前的下落过程所经历的时间t。
设运动员可以视为系于绳子一端的质点,与m相比绳子的质量可忽略不计,当绳子在伸长时服从胡克定律,在整个下落过程中空气的阻力可忽略不计。
B 一热机工作于两个相同材料的物体之间,两物体的温度分别为T A和T B(T A>T B),每个物体的质量均为m,比热恒定,均为s。
设两个物体的压强保持不变,且不发生相变。
(a)假定热机能从系统获得理论上允许的最大机械能,求出两物体A和B最终达到的温度T?的表达式,给出解题全部过程。
(b)由此得出允许获得的最大功的表达式。
(c)假定热机工作于两箱水之间,每箱水的体积为2.50m3,一箱水的温度为350K,另一箱水的温度为300K。
计算可获得的最大机械能。
已知水的比热容= 4.19×103kg-1K-1,水的密度=1.00 x 103kgm.-3C 假定地球形成时同位素238U和235U已经存在,但不存在它们的衰变产物。
238U和235U的衰变被用来确定地球的年龄T。
(a)同位素238U以4.50×109年为半衰期衰变,衰变过程中其余放射性衰变产物的半衰期比这都短得多,作为一级近似,可忽略这些衰变产物的存在,衰变过程终止于铅的同位素206Ph。
用238U的半衰期、现在238U的数目238N表示出由放射衰变产生的206Pb原子的数目206n。
(运算中以109年为单位为宜)(b)类似地,235U在通过一系列较短半衰期产物后,以0.710×109年为半衰期衰变,终止于稳定的同位素207Pb。
国际物理奥林匹克竞赛试题

国际物理奥林匹克竞赛试题一、在双缝干涉实验中,当光屏上某点到双缝的路程差为半个波长时,该点将出现:A. 明条纹B. 暗条纹C. 无法确定明暗D. 既是明条纹又是暗条纹(答案:A)二、一个物体在地球表面附近做匀速圆周运动,其向心加速度与地球同步卫星的向心加速度相比:A. 两者相等B. 物体的大C. 卫星的大D. 无法比较(答案:B)三、关于光的波动性与粒子性,下列说法正确的是:A. 光只具有波动性,不具有粒子性B. 光只具有粒子性,不具有波动性C. 光在某些情况下表现出波动性,在某些情况下表现出粒子性D. 光的波动性与粒子性是相互矛盾的,不能同时存在(答案:C)四、在量子力学中,描述粒子位置不确定度与动量不确定度之间关系的是:A. 牛顿第二定律B. 能量守恒定律C. 海森堡不确定性原理D. 薛定谔方程(答案:C)五、关于电磁感应现象,下列说法错误的是:A. 闭合电路的一部分导体在磁场中做切割磁感线运动时,导体中会产生感应电流B. 感应电流的磁场总是试图阻碍产生它的磁通量的变化C. 感应电动势的大小与线圈匝数成正比D. 在电磁感应现象中,机械能转化为电能(答案:C)六、在相对论中,关于时间膨胀效应,下列说法正确的是:A. 高速运动的物体内部时间流逝速度比静止时快B. 高速运动的物体内部时间流逝速度比静止时慢C. 时间膨胀效应与物体的运动速度无关D. 时间膨胀效应只在接近光速运动时才显著(答案:B)七、关于量子纠缠,下列说法正确的是:A. 量子纠缠是一种超距作用,违反了相对论中的光速限制B. 量子纠缠是量子系统之间的一种特殊关联,不能通过经典通信实现C. 量子纠缠只存在于相同的粒子之间D. 量子纠缠可以被观测而不影响系统的状态(答案:B)八、在杨-米尔斯理论中,描述基本粒子相互作用的是:A. 引力场B. 电磁场C. 规范场D. 标量场(答案:C)。
2013物理试题及答案
2013物理试题及答案一、选择题(每题2分,共20分)1. 光在真空中的传播速度是()。
A. 3×10^8 m/sB. 3×10^5 km/sC. 3×10^7 m/sD. 3×10^6 km/s答案:A2. 根据牛顿第二定律,力等于质量与加速度的乘积,其公式表示为()。
A. F=maB. F=mvC. F=m/aD. F=a/m答案:A3. 电流通过导体时产生的热量与电流的平方、导体的电阻和时间成正比,其公式为()。
A. Q=I^2RtB. Q=IR^2tC. Q=I^2tRD. Q=It^2R答案:A4. 电磁波的波长与频率成反比,其关系式为()。
A. λf=cB. λf=1/cC. λf=c^2D. λf=1答案:A5. 根据能量守恒定律,能量既不能被创造也不能被消灭,只能从一种形式转化为另一种形式,其表述为()。
A. ΔE=0B. ΔE=QC. ΔE=WD. ΔE=Q+W答案:A6. 以下哪个选项是描述电磁感应现象的()。
A. 电流的磁效应B. 磁场的电效应C. 电场的磁效应D. 磁场的热效应答案:B7. 根据热力学第一定律,系统内能的增加等于系统吸收的热量与对外做的功之和,其公式表示为()。
A. ΔU=Q-WB. ΔU=Q+WC. ΔU=-Q-WD. ΔU=-Q+W答案:B8. 光的折射定律描述了光从一种介质进入另一种介质时,入射角与折射角的关系,其公式为()。
A. n1sinθ1 = n2sinθ2B. n1cosθ1 = n2cosθ2C. n1tanθ1 = n2tanθ2D. n1θ1 = n2θ2答案:A9. 原子核由质子和中子组成,其中质子带正电,中子不带电,质子数决定了原子的()。
A. 质量B. 电荷C. 核力D. 元素种类答案:D10. 根据库仑定律,两点电荷之间的静电力与它们的电荷量的乘积成正比,与它们之间的距离的平方成反比,其公式为()。
2013国际物理奥林匹克竞赛理论试卷(1)
After falling a time in the atmosphere, an outer shell of Maribo of thickness will have been heated to a temperature significantly larger than . This thickness can be estimated by dimensional analysis as the simple product of powers of the thermodynamic parameters: . 1.3a Determine by dimensional (unit) analysis the value of the four powers , , , and . 1.3b Calculate the thickness after a fall time , and determine the ratio . 0.6 0.4
Through the atmosphere and melting?
The friction from the air on a meteoroid moving in the higher atmosphere depends in a complicated way on the shape and velocity of the meteoroid, and on the temperature and density of the atmosphere. As a reasonable approximation the friction force in the upper atmosphere is given by the expression , where is a constant, the density of the atmosphere, the projected cross-section area of the meteorite, and its speed. The following simplifying assumptions are made to analyze the meteoroid: The object entering the atmosphere was a sphere of mass , radius , temperature , and speed . The density of the atmosphere is constant (its value 40 km above the surface of the Earth), , and the friction coefficient is . 1.2a Estimate how long time after entering the atmosphere it takes the meteoroid to have its speed reduced by 10 % from to . You can neglect the gravitational force on the meteoroid and assume, that it maintains its mass and shape. 0.7
国际物理奥赛试题及答案
国际物理奥赛试题及答案一、选择题(每题3分,共30分)1. 光在真空中的速度是多少?A. 299,792,458 m/sB. 299,792,458 km/sC. 299,792,458 cm/sD. 299,792,458 mm/s答案:A2. 根据量子力学,一个粒子的波函数代表什么?A. 粒子的位置B. 粒子的速度C. 粒子的概率密度D. 粒子的能量答案:C3. 以下哪项不是电磁波的特性?A. 波长B. 频率C. 质量D. 能量答案:C4. 根据牛顿第三定律,作用力和反作用力的关系是什么?A. 相等且相反B. 相等且相同C. 不相等且相反D. 不相等且相同答案:A5. 热力学第一定律表述了什么?A. 能量守恒定律B. 熵增定律C. 热力学第二定律D. 热力学第三定律答案:A6. 一个物体在自由落体运动中,其加速度是多少?A. 9.8 m/s²B. 10 m/s²C. 11 m/s²D. 12 m/s²答案:A7. 以下哪种力不属于基本力?A. 重力B. 电磁力C. 强核力D. 弱核力答案:A8. 一个完全弹性碰撞中,动量守恒和能量守恒是否都成立?A. 是B. 否答案:A9. 光的双缝干涉实验中,干涉条纹的间距与什么有关?A. 光的波长B. 双缝间的距离C. 观察屏的距离D. 所有以上因素答案:D10. 根据相对论,时间膨胀和长度收缩的效应是否只发生在高速运动的物体上?A. 是B. 否答案:A二、填空题(每题2分,共20分)1. 光年是______单位,表示光在一年内通过的距离。
答案:长度2. 绝对零度是温度的下限,其数值为______开尔文。
答案:03. 一个物体的动能与其速度的平方成正比,其比例系数是______。
答案:1/24. 根据库仑定律,两个点电荷之间的力与它们电荷量的乘积成正比,与它们距离的平方成______。
答案:反比5. 根据麦克斯韦方程组,变化的磁场可以产生______。
2009-2013年全俄物理奥林匹克理论部分附答案
2009年全俄物理奥林匹克(理论部分)九年级问题9-1吊着的圆木起重机用绳索缓慢地从水中将圆木提起来。
绳索吊在圆木的一段,圆木可以看作密度一定的细圆柱。
圆木的质量为m ,长度为L 。
水和木头的密度之比43γ=。
重力加速度为g 。
⑴起重机至少要做多少功W ,才能将圆木完全拉出水面?⑵画出绳索的张力T 与圆木的上端被拉出水面的高度h 的关系图像。
⑶起重机将圆木从一个倾斜角提到另一个倾斜角,使得其上端升高5L h ∆=。
这期间,它做的功h W 等于多少?图1图2问题9-2传送带上的糖果实验员格鲁克一次参观糖果工厂时,注意到糖果从包装机中掉到传送带上的时候,速度与水平面夹角α=60°(从上方看如图2所示),速度先减小后增大。
糖果的初速度为0v ,与传送带的速度u 大小相等,且位于传送带所在平面上。
糖果落到传送带上的一瞬间,相对于传送带的速度0'v 等于多少?求出糖果相对于站着不动的格鲁克的速度的最小值v min 。
问题9-3 双桥在如图3所示的电路中,接线端C和D之间的电压15U V。
已知R>>r。
CD⑴求在A和B之间连接的理想电压表的示数。
⑵如果在A和B之间连接的是理想电流表,标出在每个电阻上以及电流表上的电流方向。
图3 图4问题9-4 中途注入水的茶壶理论家巴格想要喝茶。
他拿了一把带有小温度计的隔热的茶壶,并接上电源。
温度计的示数为T0=20℃。
经过t1=1min,水被加热到T1=40℃,他往茶壶里又注入了一些水。
在t2=3.5min的时刻,水的温度达到了T2=50℃。
巴格不再往茶壶里注水了。
又过了5分钟,水烧开了。
图4为茶壶里水的温度在加热和注水的过程中的变化图像。
注入的水的温度T x等于多少?假设水是快速混合均匀的,温度计表示水温的当前值。
十年级问题10-1 洞中的小球在水平桌面上挖了一个半径为R的半球形洞。
将质量为m的小球用长度为L=R的不可延伸的轻线系在洞口上的点A处。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
IntroductionIn this problem we study an efficient process of steam production that has been demonstrated to work experimentally. An aqueous solution of spherical nanometer-sized silver spheres (nanoparticles) with only about particles per liter is illuminated by a focused light beam. A fraction of the light is absorbed by the nanoparticles, which are heated up and generate steam locally around them without heating up the entire water solution. The steam is released from the system in the form of escaping steam bubbles. Not all details of the process are well understood at present, but the core process is known to be absorption of light through the so-called collective electron oscillations of the metallic nanoparticles. The device is known as a plasmonic steam generator.Figure 2.1(a)A spherical charge-neutral nanoparticle of radius R placed at the center of the coordinate system. (b) A sphere with a positive homogeneous charge density (red), and containing a smaller spherical charge-neutral region (0, yellow) of radius , with its center displaced by. (c) The sphere with positive charge density of the nanoparticle silver ions is fixed in the center of the coordinate system. The center of the spherical region with negative spherical charge density –(blue) of the electron cloud is displaced by , where . (d)An external homogeneous electric field . For time-dependent , the electron cloud moves with velocity . (e) The rectangular vessel () containing the aqueous solution of nanoparticles illuminated by monochromatic light propagating along the -axis with angular frequency and intensity .A single spherical silver nanoparticleThroughout this problem we consider a spherical silver nanoparticle of radius and with its center fixed at the origin of the coordinate system, see Fig. 2.1(a). All motions, forces and driving fields are parallel to the horizontal -axis (with unit vector ). The nanoparticle contains free (conduction) electrons moving within the whole nanoparticle volume without being bound to any silver atom. Each silver atom is a positive ion that has donated one such free electron.The electric field in a charge-neutral region inside a charged sphereFor the rest of the problem assume that the relative dielectric permittivity of all materials is . Inside a charged sphere of homogeneous charge density and radius R is created a small spherical charge-neutral region of radius by adding the opposite charge density , with its center displa-ced by from the center of the R-sphere, see Fig. 2.1(b).The restoring force on the displaced electron cloudIn the following, we study the collective motion of the free electrons, and therefore model them as a single negatively charged sphere of homogeneous charge density with a center position , which can move along the x-axis relative to the center of the positively charged sphere (silver ions) fixed at the origin of the coordinate system, see Fig. 2.1(c). Assume that an external force displaces the electron cloud to a new equilibrium position with . Except for tiny net charges at opposite ends of the nanoparticle, most of its interior remains charge-neutral.The spherical silver nanoparticle in an external constant electric fieldA nanoparticle is placed in vacuum and influenced by an external force due to an applied static homogeneous electric field , which displaces the electron cloud the small distance , where .The equivalent capacitance and inductance of the silver nanoparticleFor both a constant and a time-dependent field , the nanoparticle can be modeled as an equivalent electric circuit.The equivalent capacitance can be found by relating the work , done on the separation of charges , to the energy of a capacitor, carrying charge . The charge separation will cause a certain equivalent voltage across the equivalent capacitor.For a time-dependent field ,the electron cloud moves with velocity , Fig. 2.1(d). It has the kinetic energy and forms an electric current flowing through the fixed yz-plane. The kinetic energy of the electron cloud can be attributed to the energy of an equivalent inductor of inductance carrying the current .The plasmon resonance of the silver nanoparticleFrom the above analysis it follows that the motion, arising from displacing the electron cloud from its equilibrium position and then releasing it, can be modeled by an ideal LC-circuit oscillating at resonance. This dynamical mode of the electron cloud is known as the plasmon resonance, which oscillates at the so-called angular plasmon frequency .The silver nanoparticle illuminated with light at the plasmon frequencyIn the rest of the problem, the nanoparticle is illuminated by monochromatic light at the angular plasmon frequency with the incident intensity . As the wavelength is large, , the nanoparticle can be considered as being placed in a homogeneous harmonical-ly oscillating field ( ) . Driven by , the center ( )of the electron cloud oscillates at the same frequency with velocity and constant amplitude . This oscillating electron motion leads to absorption of light. The energy captured by the particle is either converted into Joule heating inside the particle or re-emitted by the particle as scattered light.Joule heating is caused by random inelastic collisions, where any given free electron once in a while hits a silver ion and loses its total kinetic energy, which is converted into vibrations of the silver ions (heat). The average time between the collisions is , where for silver nanoparticle we use .The incident light beam loses some time-averaged power by scattering on the oscillating elec-tron cloud (re-emission). depends on the scattering source amplitude , charge , angular fre-quency and properties of the light (the speed of light and permittivity in vacuum). In terms of these four variables, is given by .The above equivalent circuit elements are combined into an LCR series circuit model of the silver nanoparticle, which is driven by a harmonically oscillating equivalent voltage ( ) determined by the electric field of the incident light.Steam generation by lightAn aqueous solution of silver nanoparticles is prepared with a concentration. It is placed inside a rectangular transparent vessel of size andilluminated by light at the plasmon frequency with the same intensity at normal incidence as above, see Fig. 2.1(e). The temperature of the water is and we assume, in fair agreement with observations, that in steady state all Joule heating of the nanoparticle goes tothe production of steam of temperature , without raising the temperature of the water. The thermodynamic efficiency of the plasmonic steam generator is defined by the power ratio , where is the power going into the production of steam in the entire vessel, while is the total power of the incoming light that enters the vessel.Most of the time any given nanoparticle is surrounded by steam instead of water, and it can thus be described as being in vacuum.。
