基于有限元法的方钢管混凝土柱的延性系数研究
钢筋混凝土柱构件弹塑性位移与延性计算方法

钢筋混凝土柱构件弹塑性位移与延性计算方法蔡准【摘要】介绍了一种通过积分计算侧移的方法,利用各截面的曲率分布,计算屈服位移和极限位移,通过计算把握钢筋混凝土柱构件的位移性能获得柱构件的弹塑性位移,并求得该状况下柱构件的位移延性,从而由曲率分布获得构件位移延性性能,该方法简单实用,便于操作,值得推广。
%This thesis introduces integral calculating sidesway method,uses the section curvature,and calculates yield displacement and ultimate displacement.Through calculation,it grasps the displacement performance of reinforced concrete member,and obtains the elastic-plastic displacement of the column member,and acquires the displacement ductility of the column member,and further gets the displacement ductility performance through curvature distribution.This method is simple and practical and operational.Thus,it is worth promoting.【期刊名称】《山西建筑》【年(卷),期】2012(038)010【总页数】3页(P30-32)【关键词】钢筋混凝土柱构件;截面曲率;极限位移;位移延性【作者】蔡准【作者单位】西安建筑科技大学,陕西西安710055【正文语种】中文【中图分类】TU375.30 引言结构基于位移性能的抗震设计方法中,经常涉及结构的延性的概念。
方钢管高强混凝土短柱有限元分析

1 材 料 本 构 模 型 . 1
111 钢 材 本 构 模 型 . . 冷 弯 型 方 钢 管 高 强 混 凝 土 轴 压 短 柱 试 件 的 钢 管 采 用 冷 弯 型 方 钢 管 .考 虑 到 冷 弯 型 方 钢 管 角 部 区域 和 平 板 区域 的 力 学 性 能 有 所 不 同 ,本 文将 冷 弯 型 方 钢 管 分 割 为 两 部 分 区
_. 厂. Ⅵ1
1
m
( a )钢 材应 力I 与应 变忙) 系 曲线 关
Il冷 弯方钢 管 平板 区域 与角 部区 域 划分 b
图 1 冷 弯 型 方 钢 管 本 构 模 型 域 : 部 区 域 和 平 板 区域 【 如 图 1 示 。 中方 钢 管 的平 板 角 引 , 所 其 区域 力 学 性 能 采 用 A d lR h m n和 Sv k m r t 出 的 b e— a a a i u aa 6 a n1 给 公式来描述 , 公式 () 示 。 如 1所
的 受 力 特 点 , 于 钢 管 混 凝 土 的受 压 机 理 不 能 充 分 认 识 。 对 因
由 于 钢 管 混凝 土 组 合 结 构 的 快 速 发 展 .对 其 力 学 性 能 验 研 究 由 于 经 费 庞 大 、 期 长 等 缺 点 . 得 有 限 元 计 算 方 法 被 越 来 越 多 的研 周 使
【 日 2 1— .5 收稿  ̄1 0 2 01 7
【 作者简介】龚 ̄15,迁 院 筑 程 ,士助 ,主 郎 ,( -宿 学 建 工 系 硕 ,教 现 9) 8
要事合 构面研。 从 组 结 方 的 究
江 苏 建 筑
口
21 0 1年第 6期 ( 第 1 5 ) 总 4 期
3 7
方钢管混凝土柱局部屈曲有限元分析

k=0. 1
,/ 1 6+1. r= . 5
;
2 理论分 析
文献 [ ] 6 主要分 析 了在偏 心受 压情况下 , 矩形 钢管混 凝土柱
的局部失稳 。由于钢管混凝 土柱 属于受压构件 , 因此本文将文献 p:
[] 6 中的应 力梯度系数 的取值范 围修改为 0 < ≤1 来描述偏心
距的变化 , 也将核心混 凝土视 为刚性 支承 , 将加载 边 以及 非加载
收 稿 日期 :0 00 —1 2 1 31
j 0 再 . 7 5 5 万
’。) ; (> 。 3
为约束系数 , =A / r 。 ・ A・
A
B( )] ( e) e 0 ≤
D
园
a )
, £
f 1 厂 _1¨ % 卢 ( )
( 。。 e>e) 一
其中, = [. 4 02( ) 一 . 8 5 058 ] a 1 9+ . 。 o 1 5 , 00 e+ . ); ( 74 79
要是 由于钢管的局部 屈 曲引起 的。因此 , 在不 同 的受 力情况下 , 减 少 。 对不发生整体屈曲失稳 的钢管混凝土柱进行局部屈 曲研究是很有 3 有 限元模拟 必要的, 文 中的钢 管混凝 土柱 长细 比小于 允许 长细 比( b 本 L/ ≤
10 。 5 )
有限元方法是一个功能强大 的数值分析工具 , 目前已经被广
・
9 ・ 2
第3 6卷 第 1 9期 20 10年 7月
山 西 建 筑
S HANX I ARCHI TECTURE
Vo. 6 No. 9 13 1
方钢管混凝土柱抗震性能影响因素的研究
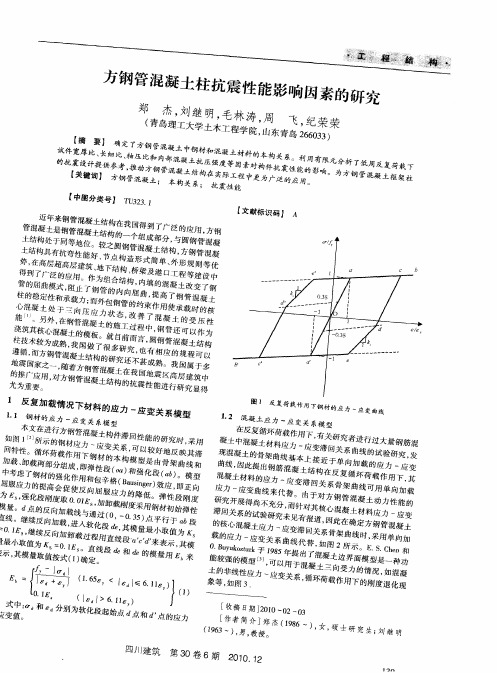
大小 直 接 影 响试 件 的极 限 荷 载 。征 轴 压 比 和 混 凝 土 强 度 相 同 的情 况下 , 厚 比 小 的 试 件 其 极 限 强 度 大 , 厚 比大 的 试 宽 宽 件 其 极 限 强 度 反 而 小 。宽 厚 比 与 含 钢 率 成 反 比。 随 着 宽 厚 比 的减 小 , 件 弹 性 阶 段 刚度 和 水 平 承 载 力 都 有 所 提 高 , 构 下 降段 的 下 降 幅度 也 略有 减 小 。但 对 曲 线 形 状 的影 响 则 不 大 , 主要 影 响 曲线 的数 值 ( 5 。 图 )
压 比越 大 则 延 性 越 差 。轴 压 比对 骨 架 曲 线 的 形 状 影 响 较 大 : 轴 压 比越 大 , 件 的 水 平 承 载 力 越 小 , 化 阶 段 的 刚 度 也 越 杆 强
小 。当轴压 比达到 一定数 值时 , 曲线将 会 出现 下 降段 , 其 而
且 下 降 段 的 下 降 幅 度 随 轴 压 比 的增 加 而 增 大 , 件 的位 移 延 构 性 则 越 来 越 小 。 另 外 , 压 比对 曲线 弹 性 阶段 的 刚 度 几 乎 没 轴 有 影 响 , 是 因 为在 弹 性 阶 段 , 件 的 变 形 小 , 这 构 P一△效 应 并 不 明 显 , 且 随着 轴 压 比 的 增 大 , 心 混 凝 土 受 拉 开 裂 面 积 而 核 会 减 少 , 一 因素 又 会 使 构 件 的 刚度 略有 增 加 ( 7 。 这 图 )
的应 用趋向于采用高强混凝 , 这就 提醒我们在 设计 中应采
取其 它措 施 以 提 高 其 延 性 ( 6 。 )
在弹性 阶段刚度 和水平 承载力 等 的影 响都 较小 , 随着 的
钢骨混凝土异形柱延性性能研究的开题报告

钢骨混凝土异形柱延性性能研究的开题报告
一、选题背景:
钢筋混凝土异形柱作为高层建筑结构中的一种常见结构形式,具有良好的力学性能和美观性。
但由于其截面形状的不规则性,其在震动条件下的性能表现较为复杂,破坏模式也较为多样。
因此,对于钢骨混凝土异形柱的延性性能研究具有重要意义。
二、研究目的:
本研究旨在通过理论分析和试验研究的手段,探究钢骨混凝土异形柱在受力条件下的延性性能和抗震性能,分析其断裂韧度、强度和刚度等参数,以及研究其在不同荷载水平下的变形及破坏形态等。
三、研究方法:
1. 理论分析:通过数值模拟方法,建立异形柱在受力条件下的数学模型,结合现有理论计算方法研究其延性性能和抗震性能。
2. 试验研究:在实验室中进行钢骨混凝土异形柱的力学性能试验,通过实验数据分析其延性性能和抗震性能。
四、研究内容:
1. 钢骨混凝土异形柱的设计和分类。
2. 异形柱在受力条件下的理论分析,包括抗弯承载能力、抗剪承载能力和抗压承载能力等。
3. 实验试验设计,确定试验参数及荷载方案,制备试验样品。
4. 实验结果分析,探究异形柱的断裂韧度、强度和刚度等参数,并分析其在不同荷载水平下的变形及破坏形态等。
五、研究意义:
钢骨混凝土异形柱在高层建筑结构中占据着重要的地位,其力学性能和抗震性能也备受关注,因此该研究对于提高高层建筑的耐震性能和安全性具有重大意义。
六、研究进度:
目前已经完成了相关文献的查阅和整理,已经初步确定了研究方向和方法,即将进入项目实施阶段。
带加劲肋方钢管混凝土柱抗震性能有限元分析

带加劲肋方钢管混凝土柱抗震性能有限元分析【摘要】基于一340m高的超高层建筑为背景,主要研究其内部方钢管混凝土柱的受力特性。
设计了相应的方钢管混凝土柱模型构件,针对此钢管混凝土柱,在Abaqus有限元软件中选取适当的本构模型、单元类型、界面摩擦系数等,模拟分析了拟静力荷载作用下钢管混凝土柱的滞回曲线及应力云图。
以轴压比、长细比、混凝土抗压强度、钢管壁厚、钢管钢材屈服强度、肋条等为主要变化因素,对方钢管混凝土柱利用有限元模拟方法进行模拟计算,得出不同参数对此钢管混凝土柱受力性能的影响。
【关键词】巨型钢管混凝土柱;抗震性能;非线性有限元分析1 引言高层建筑中,由于建筑物层数多、高度高,为了满足强度、刚度、稳定性,舒适性的要求,导致竖向构件需要承受巨大的竖向荷载,风荷载、以及地震作用引起的水平荷载、倾覆力矩。
钢——混凝土组合结构充分发挥钢和混凝土这两种材料的优势,使结构达到最好的各自性能。
钢管混凝土结构,就是一种介于钢结构和钢筋混凝土结构之间的钢——混凝土组合结构,具有承载力高、塑性和韧性好、抗震性能好、施工方便等特点。
到目前为止,国内外众多学者对钢管混凝土结构进行了研究并取得了很多研究成果。
Tomii和Sakino0主要进行钢管混凝土柱受恒定轴压作用和往复荷载作用下滞回性能进行研究,主要考虑轴压比和截面宽厚比对构件的影响以及反复荷载作用下钢管和混凝土之间的粘结强度的变化规律。
Kang和Moon0对36个方钢管混凝土构件恒压下进行单调水平加载和往复加载进行了试验研究。
国内学者对钢管混凝土力学性能和设计方法开展了深入的研究工作,吕西林、余勇0进行了12根方钢管混凝土压弯构件在往复荷载作用下的滞回试验,主要考察了截面宽厚比、轴压比和混凝土强度三个参数。
韩林海0进行10根圆钢管高性能混凝土和8根方钢管高性能混凝土压弯构件的滞回性能试验研究,分析了轴压比、钢材强度、混凝土强度对钢管高性能混凝土荷载-位移滞回关系曲线、承载力和耗能等的影响,考察了荷载——位移滞回曲线抗弯刚度退化的情况,并初步探讨了钢管高性能混凝土构件的承载力的计算方法。
方形截面不锈钢管混凝土短柱轴压性能有限元研究

引用格式:董尧韡, 王飞. 方形截面不锈钢管混凝土短柱轴压性能有限元研究[J]. 中国测试,2023, 49(8): 61-66. DONG Yaowei,WANG Fei. Study on bearing behavior of concrete-filled square stainless steel tube stub columns under axial compression[J]. China Measurement & Test, 2023, 49(8): 61-66. DOI: 10.11857/j.issn.1674-5124.2021070069方形截面不锈钢管混凝土短柱轴压性能有限元研究董尧韡1, 王 飞2(1. 山西工程科技职业大学,山西 晋中 030619; 2. 太原理工大学土木工程学院,山西 太原 030024)摘 要: 不锈钢管混凝土(CFSST )结构在工程的应用愈发广泛,但目前对其轴压性能的研究较少。
该文利用ABAQUS 创建方形不锈钢管混凝土短柱(CFSSST )模型,并利用收集到试验结果对其进行验证。
采用已被验证的模型进行参数分析,研究截面宽厚比和混凝土强度对其轴心受压性能的影响,并将参数分析轴压承载力结果与GB 50936—2014《钢管混凝土结构技术规范》、欧洲规范EN 1994-1-1和美国钢结构规范AISC 360-16中钢管混凝土(CFST)短柱轴压承载力的计算值进行对比,结果表明:规范的计算值偏于保守。
最后,基于GB 50936—2014《钢管混凝土结构技术规范》提出适用于CFSSST 短柱轴压承载力的计算公式,收集到的试验结果与计算值吻合良好。
关键词: 方形截面不锈钢管混凝土短柱; 轴压承载性能; 有限元研究; 宽厚比中图分类号: TU398.9文献标志码: A 文章编号: 1674–5124(2023)08–0061–06Study on bearing behavior of concrete-filled square stainlesssteel tube stub columns under axial compressionDONG Yaowei 1, WANG Fei 2(1. Shanxi Vocational University of Engineering Science and Technology, Jinzhong 030619, China; 2. College ofArchitecture and Civil Engineering, Taiyuan University of Technology, Taiyuan 030024, China)Abstract : Concrete-filled stainless steel tube structures is widely used in engineering practice, however, the research on its axial compression performance is limited. In this paper, the compressive behavior of CFSSST stub columns has been investigated. The finite element (FE) software ABAQUS was to used to establish the FE analysis. Based on the experiments results, the validity of the FE model was verified. The influence of width-thickness ratio of the section and concrete strength on the compressive behavior of CFSSST stub columns was investigate by using the FE model, and parametric analysis results, which were compared with predicted load values by codes GB 50936—2014, EN 1994-1-1 and AISC 360-16, indicate that the predicted load values are the conservative. Finally, based on the code GB 50936—2014, the calculation formula for the ultimate capacity of CFSSST stub columns is proposed. Predicted values using the calculation formula agree well with the collected test results.Keywords : concrete-filled square stainless steel tube stub columns; axial compressive behavior; finite element analysis; width-thickness ratio收稿日期: 2021-07-11;收到修改稿日期: 2021-12-27基金项目: 国家自然科学基金(51378290)作者简介: 董尧韡(1984-),女,山西临汾市人,讲师,硕士,研究方向为建筑结构等。
方钢管混凝土柱的延性系数

方钢管混凝土柱的延性系数
韩林海;陶忠
【期刊名称】《地震工程与工程振动》
【年(卷),期】2000(20)4
【摘要】在空钢管中填充混凝土可以避免或延缓钢管过早地发生局部屈曲,可以有效地提高构件的延性,从而增强构件的抗震性能。
本文研究方钢管混凝土柱的延性性能。
本文首先提出方钢管混凝土柱延性系数的计算方法,然后,在大规模参数分析结果的基础上,考察了轴压比、长细比、含钢率、钢材屈服极限和混凝土抗压强度等参数对延性系数的影响规律。
本文的研究成果可供有关工程实践参考。
【总页数】10页(P56-65)
【关键词】方钢管混凝土柱;约束效应系数;恢复力模型;轴压比;延性系数
【作者】韩林海;陶忠
【作者单位】福州大学土木建筑工程学院
【正文语种】中文
【中图分类】TU392.3;TU398
【相关文献】
1.内置CFRP管的方钢管混凝土轴压短柱失效分析及延性优化 [J], 郎;杨志坚;李泽良
2.空心圆钢管混凝土柱延性系数的研究 [J], 张凤亮;查晓雄;倪艳春
3.方钢管混凝土柱延性的影响因素分析 [J], 杜云晶;张灵坤
4.方钢管混凝土柱的累积延性 [J], 张娜;祝令民
5.方套方复合钢管混凝土短柱压扭性能及延性分析 [J], 陈全有;李吉人;赵阳;王庆利
因版权原因,仅展示原文概要,查看原文内容请购买。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
(a)
(b)
Fig.1 Simplified model of square-shaped CFT column In this paper, the heights of all the square-shaped CFT columns are two meters. The slenderness ratios are respectively set as 20, 30 and 40, which are determined by the width of cross section. The steel ratios are respectively set as 0.08, 0.12, 0.16, 0.20 and 0.24, and they are determined by the wall thickness of steel tubes. The axial compression ratios of columns are set as 0.5, 0.6, 0.7 and 0.8, the value of axial loads are determined by the cross section form and the cross-sectional area of the components. 3.
Abstract: Ductility coefficient is not only an important parameter to assess the ductility performance of components, but also an index to estimate the aseismic behavior of columns. The ductility coefficient is mainly affected by some factors, such as slenderness ratio, axial compression ratio and steel ratio. In this paper, using the general finite element analysis software ABAQUS, authors have numerically analyzed the mechanical performance of square-shaped concrete-filled steel tubular (CFT) columns. Meanwhile, the authors have studied the influence of different factors, including the slenderness ratio, axial compression ratio and steel ratio, to the ductility coefficients. Through the analysis, it is concluded that slenderness ratio is the most effect on ductility coefficient, followed by axial compression ratio and the steel ratio is the least. Keywords: Ductility coefficient; Square-shaped concrete-filled steel tubular columns; Finite element method; Slenderness ratio; Axial compression ratio; Steel ratio
1. Introduction
Concrete-filled steel tubular (CFT) structures, as a type of new composite structure, are widely used in high-rise buildings and complex structures due to their perfect seismic performance and good economic performance. In CFT structure subjected to axial compressive load, the deformation of concrete is restrained by the steel tube, so the compressive strength and deformation capacity of concrete would be higher than plain concrete. At the same time, the steel tube filled by concrete would get higher stability and compressive strength. In a structure, column plays an important role in bearing and load transmission, so it is the key to influence the safety of overall performance structure. Researchers have widely studied the bearing capacity of CFT columns. Gardner and Jacobson [1] have made investigation about the performance of CFT columns under the axial load based on theoretical analysis and experimental results, at the same time the results were compared to the loads allowed by the NBC and ACI recommendations. Knowles and Park [2] have studied the effect of slenderness ratio on the ultimate strength of CFT columns under axial and eccentric loads. Han et al. [3][4] have conducted some experiments on the bearing strength of long and short CFT columns with different slenderness ratios. Ductility coefficient is not only an important parameter to assess the ductility performance of components, but also an index to estimate the aseismic behaviour of columns. Some scholars have studied the ductility coefficient of CFT columns. Han and Tao [5][6] have analyzed the influence of some factors, including slenderness ratio, axial compression ratio, steel ratio, yield strength of steel and compressive strength concrete, to ductility coefficient based on theoretical analysis. Wang and Lu have analysed the effects of slenderness ratio and steel ratio on the ductility of square-shaped CFT frame structure based on the nonlinear finite element analysis model. Based on the analysis above, the authors create the finite element analysis model of square-shaped CFT columns by general finite element analysis software ABAQUS, in which the slenderness
9
பைடு நூலகம்
3:1 (2014)
Journal of Materials and Applications
ratio , axial compression ratio n and steel ratio are the three main factors. In this model, the load-displacement curves of CFT columns with different parameters are obtained through the push-over simulation, and the influence of these factors to ductility coefficients are discussed.
Bao-Jie He1, Miao Ye2 1. School of Environment and Architecture, University of Shanghai for Science and Technology, Shanghai, 200093 2. School of Environment and Architecture, University of Shanghai for Science and Technology, Shanghai, 200093 E-mail: yemiao0927@
