数学分析第1章概论
数分第一章第一节实数

数学分析第一章实数和数列极限《数学分析》又名《微积分》。
(其实我们讲的《数学分析》内容要比通常的《微积分》内容多)主要内容:微分学;积分学;微分与积分的关系。
学习研究微积分的重要基本工具是极限理论(又称无穷小分析),极限理论包括实数理论,数列极限,函数极限,数项级数和函数项级数等。
极限运算其实是一种无穷次运算,这就是区别于有限次运算(只是量变)的代数学几何学的标志。
极限理论是分析学科的灵魂,在分析学中无处不在。
(就如武术中的太极和八卦,在武术中无处不在,起到至高无上的作用。
)极限的无限次运算作用,就是哲学上量变(无限累加)到质变的飞跃。
极限理论的思想方法技巧(又称无穷小分析)不仅是《数学分析》主要工具,也是后继的分析学科(常微分方程,偏微分方程,实分析,复变函数,复分析,Fourier分析,调和分析,逼近理论,实变函数,泛函分析,测度论,概率论等)发展的主要工具,深刻的理论和结论,都要靠极限理论来发掘完成。
极限又有数列的极限(级数)和函数的极限等(既有区别又有联系)。
《数学分析》的基础是建立在实数理论之上,实数是《数学分析》的工作空间,实数理论和极限理论是数学严密严格化的标志(这样才能保证不出错误,否则就会混乱不清,甚至出错。
)实数理论的深刻认识的建立靠的是极限理论。
因此我们得从实数理论和数列的极限理论谈起。
第一节 实数与数轴1 实数的再认识数系的发展自然数: ,,,3,2,1,0n ;分数:q p ,(q p ,为自然数,且0≠q ),负整数: ,,,3,2,1n ----; 负分数:q p -,(q p ,为自然数,且0≠q )整数: ,,,3,2,1,0n , ,,,3,2,1n ---- ;有理数:q p ,(q p ,为整数,且0 q ); (整数和分数统称有理数; 或有理数就是分数,或整数; 或整数,有限小数,无限循环的小数通称有理数。
)在有理数中可引入:加法运算,减法运算,乘法运算,除法运算(除数不能是0);有理数经过加、减、乘、除(除数不能是0)四则运算之后仍为有理数。
数学分析第一册第一章
S的最小的上界 称作 的上确界 的最小的上界,称作 的上确界. 的最小的上界 称作S的上确界 满足: 定义2 定义 设S是R中的一个数集 若数 η 满足: 是 中的一个数集 (i) 对一切 x ∈ S , 有 x ≤ η , η 即是 的上界; 即是S的上界 的上界; (ii) 对任何 α < η , 存在x0 ∈ S , 使得 x0 > α , 即 则称数
事实上,对任何正数 无论多么大 无论多么大), 事实上,对任何正数M(无论多么大 ,取 则 n0 ∈ N + , 且 n0
n0 = [ M ] + 1, ([ M ]表示对M 取整)
问题: 问题 设 S
有无上界; 有无上界 = [0,1]. (1) S有无上界 (2) S若有上界 有几个上界 若有上界,有几个上界 若有上界 有几个上界; (3) S有无最小的上界 有无最小的上界. 有无最小的上界
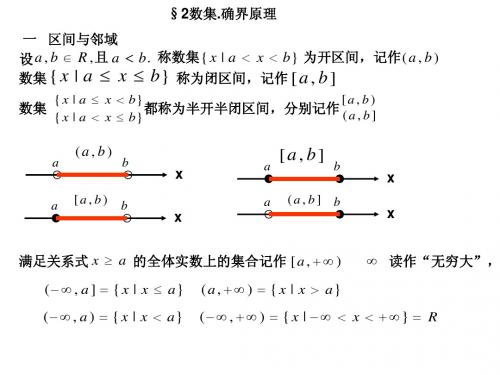
数集.确界原理 §2数集 确界原理 数集 一 区间与邻域 为开区间, 设 a, b ∈ R, 且 a < b. 称数集 {x | a < x < b} 为开区间,记作 ( a, b) 称为闭区间,记作 数集 称为闭区间,
{x | a ≤ x ≤ b}
(a, b)
数集
{x | a ≤ x < b} [a, b) 都称为半开半闭区间, 都称为半开半闭区间,分别记作 ( a, b] { x | a < x ≤ b} b
例2 设
满足: 定义2 定义 设S是R中的一个数集 若数η 满足: 是 中的一个数集 (i) 对一切 x ∈ S , 有 x ≤ η , η 即是 的上界; 即是S的上界 的上界; η 又是 的最小上界, 的最小上界 (ii) 对任何 α < η , 存在x ∈ S , 使得 x0 > α ,即 又是S的最小上界, 则称数 证明: S = [0,1]. 证明 sup S = 1. 的上界; 的上界 证: (i) 对一切 x ∈ S , 有 x ≤ 1, η = 1 是S的上界; (ii) 对任何 α < 1, 取 x0 = 1 ∈ S , 则有 x0 > α , 故 sup S = 1. 例2 设 证明: = [0,1).证明: sup S = 1. 的上界; 的上界 证: (i) 对一切 x ∈ S , 有 x ≤ 1, η = 1 是S的上界; 则有任取 x0 ∈ S , (ii) 对任何 α < 1. 若 α < 0, 1+ α , 有 α < x0 . 有 α < x0 . 若 0 ≤ α < 1, 取 x0 = 2 sup S = 1. 例3 设 S 所以
华东师大第五版数学分析第一章第一节

令 = − , 则为正数且 = + , 但这与假设 < + 相矛盾. 从而
必有 ≤ .
1.2 绝对值与不等式
,
≥ 0,
定义: = ቊ
−, < 0.
实数绝对值的性质:
➢ 正定性: = − ≥ 0; 当且仅当 = 0时有 = 0.
其中0 , 0 为非负整数, , ( = 1,2, ⋯ )为整数, 0 ≤ ≤ 9, 0 ≤
≤ 9, 若有
= ,
= 0,1,2, ⋯
则称与相等,记为 = ;若0 > 0 或存在非负整数,使得
= ( = 0,1,2, ⋯ ) 而+1 > +1 ,
• 实数具有阿基米德(Archimedes)性,即对任何, ∈ R, 若 > >
0, 则存在正整数, 使得 > .
• 实数集具有稠密性, 即任何两个不相等的实数之间必有另一个实
数, 且既有有理数,也有无理数.
• 实数集与数轴上的点有着一一对应关系.
例2 设, ∈ R. 证明:若对任何正数, 有 < + , 则 ≤ .
似分别规定为
= −0 . 1 2 ⋯ − 10− 与ҧ = −0 . 1 2 ⋯ .
注:
0 ≤ 1 ≤ 2 ≤ ⋯
ҧ0 ≥ ҧ1 ≥ ҧ2 ≥ ⋯
实数的不足近似与过剩近似是用有限小数研究无限小数的重要
工具.
命题
设 = 0 . 1 2 ⋯ 与 = 0 . 1 2 ⋯为两个实数,则 >
的等价条件是:存在非负整数,使得
数学分析1

第1章 集合与映射 █ █1《数学分析Ⅰ》第1讲 教学内容:数学分析总概第1章 集合与映射一、数学分析总概牛顿(Newton.I 1642-1727)英国数学物理学家,在1665-1666年间发表著名公式()()()baf x dx F b F a =-⎰。
莱布尼兹(Leibniz.G.W 1646-1716)德国数学家,在1673-1676年间发表著名公式()()()b af x dx F b F a =-⎰。
二、集合 §1.1集合概念:一些事物所汇聚的总体通常称为一个集合,总体中的每一个成员,叫做该集合的元素。
一般用大写英文字母表示集合,小写英文字母表示集合中的元素,例如:,,...A B C 通常表示集合;,,...,...a b c x y 等表示集合中的元素。
-自然数集; -整数集; -有理数集; -实数集; {|0}x x +==>▇ ▇ 数学分析2有限集 可列集 无限极 空集 子集 ∙集合的运算:(1)并集:A B{|A B x x A =∈ 或}x B ∈见(图1-1)(2)交集:A B{|A B x x A =∈ 且}x B ∈见(图1-2)(3)差集:A B -{|A B x x A -=∈且}x B ∉见(图1-3)(4)设 A X ⊂,即A 为X 的子集,补集:CA X A =-称为A 的补集。
见(图1-4)(5)无限并:设12,,...,...n A A A 是一 列集合,定义1{|,}nn n x n x A ∞=A=∃∈∈(6)无限交:设12,,...,...n A A A 是一 列集合,定义1{|,}nn n Ax n x A ∞==∀∈∈设Γ是任意的一个非空集合(拓扑集),α∀∈Γ,对应有集合A α, {:}A αα∈Γ称为集合族,无论Γ是有限集、可列集、还是不可列集(不可数集),都可定义(1) 不可数并:{|,}A x x A αααα∈Γ=∃∈Γ∈ (2) 不可数交:{|,}A x x A αααα∈Γ=∀∈Γ∈第1章 集合与映射 █ █3命题1.1 设{ A α:α∈Γ}中每一个集合都是某个大集合X 的子集,记 A C=X -A ,其中A ⊂X ,则 (3) ()c αα∈ΓA =c αα∈ΓA (4)()c αα∈ΓA =c αα∈ΓA 上面公式(9)和(10)通常称为DeMorgan 公式(隶末根定理)。
数学分析讲义(第一章)

Ⅱ 典型例题与方法
1. 利用极限定义验证极限
前提:知道数列(函数)的极限值;
关键:寻找 N (δ ) .
基本方法:
(1)求最小的 N :从不等式 an − a < ε 直接解出 n ;
(2)适当放大法:不等式 an − a < ε 较为复杂,无法直接解出,或求解的过程较繁,
为此先将表达式 an − a 进行化简,并适当放大,使之成为关于 n 的简单函数 H (n) (仍为无
(5). lim f (x) = A ⇔ ∀ε > 0, ∃M > 0, 当 x > M 时,有 f (x) − A < ε . x→+∞
(6) lim f (x) = A ⇔ ∀ε > 0, ∃M > 0, 当 x < −M 时,有 f (x) − A < ε . x→−∞ 2
特别地,若函数以零为极限,则称之为该情形下的无穷小量.理解无穷小量阶的比较的定
义及其意义,掌握等价无穷小量在极限计算中的应用,熟记常用的等价无穷小量:当 x → 0
时,
x ~ sin x ~ tan x ~ arcsin x ~ arctan x ~ ln(1 + x) ~ e x −1,
1 − cos x ~ x2 , (1 + x)α ~ αx, a x − 1 ~ x ln a . 2
n →∞
yn xn
= ⎪⎨+ ∞, ⎪⎩− ∞.
二 函数极限
1 定义 函数极限的六种形式:
(1)
lim f (x) = A ⇔ ∀ε > 0, ∃δ > 0, 当 0 <
x → x0
x − x0
< δ 时,有
数学分析第一章

Chapter1.Metric Spaces§1.Metric SpacesA metric space is a set X endowed with a metricρ:X×X→[0,∞)that satisfies the following properties for all x,y,and z in X:1.ρ(x,y)=0if and only if x=y,2.ρ(x,y)=ρ(y,x),and3.ρ(x,z)≤ρ(x,y)+ρ(y,z).The third property is called the triangle inequality.We will write(X,ρ)to denote the metric space X endowed with a metricρ.If Y is a subset of X,then the metric space(Y,ρ|Y×Y)is called a subspace of(X,ρ).Example1.Letρ(x,y):=|x−y|for x,y∈I R.Then(I R,ρ)is a metric space.The set I R equipped with this metric is called the real line.Example2.Let I R2:=I R×I R.For x=(x1,x2)∈I R2and y=(y1,y2)∈I R2,defineρ(x,y):=(x1−y1)+(x2−y2).Thenρis a metric on I R2.The set I R2equipped with this metric is called the Euclidean plane.More generally,for k∈I N,the Euclidean k space I R k is the Cartesian product of k copies of I R equipped with the metricρgiven byρ(x,y):=kj=1(x j−y j)21/2,x=(x1,...,x k)and y=(y1,...,y k)∈I R k.Example3.Let X be a nonempty set.For x,y∈X,defineρ(x,y):=1if x=y, 0if x=y.In this case,ρis called the discrete metric on X.Let(X,ρ)be a metric space.For x∈X and r>0,the open ball centered at x∈X with radius r is defined asB r(x):={y∈X:ρ(x,y)<r}.A subset A of X is called an open set if for every x∈A,there exists some r>0 such thatB r(x)⊆A.1Theorem1.1.For a metric space(X,ρ)the following statements are true.1.X and∅are open sets.2.Arbitrary unions of open sets are open sets.3.Finite intersections of open sets are open sets.Proof.Thefirst statement is obviously true.For the second statement,we let(A i)i∈I be a family of open subsets of X and wish to prove that∪i∈I A i is an open set.Suppose x∈∪i∈I A i.Then x∈A ifor some i0∈I.Since A i0is an open set,there exists some r>0such that B r(x)⊆A i.Consequently,B r(x)⊆∪i∈I A i.This shows that∪i∈I A i is an open set.For the third statement,we let{A1,...,A n}be afinite collection of open subsets of X and wish to prove that∩n i=1A i is an open set.Suppose x∈∩n i=1A i.Then x∈A i for every i∈{1,...,n}.For each i∈{1,...,n},there exists r i>0such that B ri(x)⊆A i. Set r:=min{r1,...,r n}.Then r>0and B r(x)⊆∩n i=1A i.This shows that∩n i=1A i is an open set.Let(X,ρ)be a metric space.A subset B of X is called an closed set if its complement B c:=X\B is an open set.The following theorem is an immediate consequence of Theorem1.1.Theorem1.2.For a metric space(X,ρ)the following statements are true.1.X and∅are closed sets.2.Arbitrary intersections of closed sets are closed sets.3.Finite unions of closed sets are closed sets.Let(X,ρ)be a metric space.Given a subset A of X and a point x in X,there are three possibilities:1.There exists some r>0such that B r(x)⊆A.In this case,x is called an interiorpoint of A.2.For any r>0,B r(x)intersects both A and A c.In this case,x is called a boundarypoint of A.3.There exists some r>0such that B r(x)⊆A c.In this case,x is called an exteriorpoint of A.For example,if A is a subset of the real line I R bounded above,then sup A is a boundary point of A.Also,if A is bounded below,then inf A is a boundary point of A.A point x is called a closure point of A if x is either an interior point or a boundary point of A.We denote by A the set of closure points of A.Then A⊆A.The set A is called the closure of A.2Theorem1.3.If A is a subset of a metric space(X,ρ),then A is the smallest closed set that includes A.Proof.Let A be a subset of a metric space.Wefirst show that A is closed.Suppose x/∈A. Then x is an exterior point of A;hence there exists some r>0such that B r(x)⊆A c.If y∈B r(x),thenρ(x,y)<r.Forδ:=r−ρ(x,y)>0,by the triangle inequality we have Bδ(y)⊆B r(x).It follows that Bδ(y)⊆A c.This shows y/∈A.Consequently,B r(x)⊆A c. Therefore,A c is open.In other words,A is closed.Now assume that B is a closed subset of X such that A⊆B.Let x∈B c.Then there exists r>0such that B r(x)⊆B c⊆A c.This shows x∈A c.Hence,B c⊆A c.It follows that A⊆B.Therefore,A is the smallest closed set that includes A.A subset A of a metric space(X,ρ)is said to be dense in X if A=X.§pletenessLet(x n)n=1,2,...be a sequence of elements in a metric space(X,ρ).We say that (x n)n=1,2,...converges to x in X and write lim n→∞x n=x,ifρ(x n,x)=0.limn→∞From the triangle inequality it follows that a sequence in a metric space has at most one limit.Theorem2.1.Let A be a subset of a metric space(X,ρ).Then a point x∈X belongs to A if and only if there exists a sequence(x n)n=1,2,...in A such that lim n→∞x n=x. Proof.If x∈A,then B1/n(x)∩A=∅for every n∈I N.Choose x n∈B1/n(x)∩A for each n∈I N.Thenρ(x n,x)<1/n,and hence lim n→∞x n=x.Suppose x/∈A.Then there exists some r>0such that B r(x)∩A=∅.Consequently, for any sequence(x n)n=1,2,...in A,we haveρ(x n,x)≥r for all n∈I N.Thus,there is no sequence of elements in A that converges to x.A sequence(x n)n=1,2,...in a metric space(X,ρ)is said to be a Cauchy sequence if for any givenε>0there exists a positive integer N such thatm,n>N impliesρ(x m,x n)<ε.Clearly,every convergent sequence is a Cauchy sequence.If a metric space has the property that every Cauchy sequence converges,then the metric space is said to be complete.For example,the real line is a complete metric space.3The diameter of a set A is defined byd(A):=sup{ρ(x,y):x,y∈A}.If d(A)<∞,then A is called a bounded set.Theorem2.2.Let(X,ρ)be a complete metric space.Suppose that(A n)n=1,2,...is a sequence of closed and nonempty subsets of X such that A n+1⊆A n for every n∈I N and lim n→∞d(A n)=0.Then∩∞n=1A n consists of precisely one element.Proof.If x,y∈∩∞n=1A n,then x,y∈A n for every n∈I N.Hence,ρ(x,y)≤d(A n)for all n∈I N.Since lim n→∞ρ(A n)=0,it follows thatρ(x,y)=0,i.e.,x=y.To show∩∞n=1A n=∅,we proceed as follows.Choose x n∈A n for each n∈I N.Since A m⊆A n for m≥n,we haveρ(x m,x n)≤d(A n)for m≥n.This in connection with the assumption lim n→∞d(A n)=0shows that(x n)n=1,2,...is a Cauchy sequence.Since (X,ρ)is complete,there exists x∈X such that lim n→∞x n=x.We have x m∈A n for all=A n.This is true for all n∈I N.Therefore,x∈∩∞n=1A n.m≥n.Hence,x∈A§pactnessLet(X,ρ)be a metric space.A subset A of X is said to be sequentially compact if every sequence in A has a subsequence that converges to a point in A.For example,afinite subset of a metric space is sequentially compact.The real line I R is not sequentially compact.But a bounded closed interval in the real line is sequentially compact.A subset A of a metric space is called totally bounded if,for every r>0,A can be covered byfinitely many open balls of radius r.For example,a bounded subset of the real line is totally bounded.On the other hand, ifρis the discrete metric on an infinite set X,then X is bounded but not totally bounded. Theorem3.1.Let A be a subset of a metric space(X,ρ).Then A is sequentially compact if and only if A is complete and totally bounded.Proof.Suppose that A is sequentially compact.Wefirst show that A is complete.Let (x n)n=1,2,...be a Cauchy sequence in A.Since A is sequentially compact,there exists a )k=1,2,...that converges to a point x in A.For anyε>0,there exists subsequence(x nka positive integer N such thatρ(x m,x n)<ε/2whenever m,n>N.Moreover,there exists some k∈I N such that n k>N andρ(x n,x)<ε/2.Thus,for n>N we havek4ρ(x n,x)≤ρ(x n,x nk )+ρ(x nk,x)<ε.Hence,lim n→∞x n=x.This shows that A iscomplete.Next,if A is not totally bounded,then there exists some r>0such that A cannot be covered byfinitely many open balls of radius r.Choose x1∈A.Suppose x1,...,x n∈A have been chosen.Let x n+1be a point in the nonempty set A\∪n i=1B r(x i).If m,n∈I N and m=n,thenρ(x m,x n)≥r.Therefore,the sequence(x n)n=1,2,...has no convergent subsequence.Thus,if A is sequentially compact,then A is totally bounded.Conversely,suppose that A is complete and totally bounded.Let(x n)n=1,2,...be a sequence of points in A.We shall construct a subsequence of(x n)n=1,2,...that is a Cauchy sequence,so that the subsequence converges to a point in A,by the completeness of A.For this purpose,we construct open balls B k of radius1/k and corresponding infinite subsets I k of I N for k∈I N recursively.Since A is totally bounded,A can be covered byfinitely many balls of radius1.Hence,we can choose a ball B1of radius1such that the set I1:={n∈I N:x n∈B1}is infinite.Suppose that a ball B k of radius1/k and an infinite subset I k of I N have been constructed.Since A is totally bounded,A can be covered by finitely many balls of radius1/(k+1).Hence,we can choose a ball B k+1of radius1/(k+1) such that the set I k+1:={n∈I k:x n∈B k+1}is infinite.Choose n1∈I1.Given n k,choose n k+1∈I k+1such that n k+1>n k.By our construction,I k+1⊆I k for all k∈I N.Therefore,for all i,j≥k,the points x niandx nj are contained in the ball B k of radius1/k.It follows that(x nk)k=1,2,...is a Cauchysequence,as desired.Theorem3.2.A subset of a Euclidean space is sequentially compact if and only if it is closed and bounded.Proof.Let A be a subset of I R k.If A is sequentially compact,then A is totally bounded and complete.In particular,A is bounded.Moreover,as a complete subset of I R k,A is closed.Conversely,suppose A is bounded and closed in I R k.Since I R k is complete and A is closed,A is complete.It is easily seen that a bounded subset of I R k is totally bounded.Let(A i)i∈I be a family of subsets of X.We say that(A i)i∈I is a cover of a subset A of X,if A⊆∪i∈I A i.If a subfamily of(A i)i∈I also covers A,then it is called a subcover. If,in addition,(X,ρ)is a metric space and each A i is an open set,then(A i)i∈I is said to be an open cover.Let(G i)i∈I be an open cover of A.A real numberδ>0is called a Lebesgue number for the cover(G i)i∈I if,for each subset E of A having diameter less thanδ,E⊆G i for5some i∈I.Theorem3.3.Let A be a subset of a metric space(X,ρ).If A is sequentially compact, then there exists a Lebesgue numberδ>0for any open cover of A.Proof.Let(G i)i∈I be an open cover of A.Suppose that there is no Lebesgue number for the cover(G i)i∈I.Then for each n∈I N there exists a subset E n of A having diameter less than1/n such that E n∩G c i=∅for all i∈I.Choose x n∈E n for n∈I N.Since A is sequentially compact,there exists a subsequence(x nk)k=1,2,...which converges to a point x in A.Since(G i)i∈I is a cover of A,x∈G i for some i∈I.But G i is an open set.Hence, there exists some r>0such that B r(x)⊆G i.We canfind a positive integer k such that1/n k<r/2andρ(x nk ,x)<r/2.Let y be a point in E nk.Since x nkalso lies in the setE nk with diameter less than1/n k,we haveρ(x nk,y)<1/n k.Consequently,ρ(x,y)≤ρ(x,x nk)+ρ(x nk,y)<r2+1n k<r.This shows E nk ⊆B r(x)⊆G i.However,E nkwas so chosen that E nk∩G c i=∅.Thiscontradiction proves the existence of a Lebesgue number for the open cover(Gi)i∈I.A subset A of(X,ρ)is said to be compact if each open cover of A possesses afinite subcover of A.If X itself is compact,then(X,ρ)is called a compact metric space. Theorem3.4.Let A be a subset of a metric space(X,ρ).Then A is compact if and only if it is sequentially compact.Proof.If A is not sequentially compact,then A is an infinite set.Moreover,there exists a sequence(x n)n=1,2,...in A having no convergent subsequence.Consequently,for each x∈A,there exists an open ball B x centered at x such that{n∈I N:x n∈B x}is afinite set.Then(B x)x∈A is an open cover of A which does not possess afinite subcover of A. Thus,A is not compact.Now suppose A is sequentially compact.Let(G i)i∈I be an open cover of A.By Theorem3.3,there exists a Lebesgue numberδ>0for the open cover(G i)i∈I.By Theorem 3.1,A is totally bounded.Hence,A is covered by afinite collection{B1,...,B m}of open balls with radius less thanδ/2.For each k∈{1,...,m},the diameter of B k is less thanδ.Hence,B k⊆G ik for some i k∈I.Thus,{G ik:k=1,...,m}is afinite subcover of A.This shows that A is compact.6§4.Continuous FunctionsLet(X,ρ)and(Y,τ)be two metric spaces.A function f from X to Y is said to be continuous at a point a∈X if for everyε>0there existsδ>0(depending onε)such thatτ(f(x),f(a))<εwheneverρ(x,a)<δ.The function f is said to be continuous on X if f is continuous at every point of X.Theorem4.1.For a function f from a metric space(X,ρ)to a metric space(Y,τ),the following statements are equivalent:1.f is continuous on X.2.f−1(G)is an open subset of X whenever G is an open subset of Y.3.If lim n→∞x n=x holds in X,then lim n→∞f(x n)=f(x)holds in Y.4.f(A)⊆f(A)holds for every subset A of X.5.f−1(F)is a closed subset of X whenever F is a closed subset of Y.Proof.1⇒2:Let G be an open subset of Y and a∈f−1(G).Since f(a)∈G and G is open,there exists someε>0such that Bε(f(a))⊆G.By the continuity of f,there exists someδ>0such thatτ(f(x),f(a))<εwheneverρ(x,a)<δ.This shows Bδ(a)⊆f−1(G). Therefore,f−1(G)is an open set.2⇒3:Assume lim n→∞x n=x in X.Forε>0,let V:=Bε(f(x)).In light of statement2,f−1(V)is an open subset of X.Since x∈f−1(V),there exists someδ>0 such that Bδ(x)⊆f−1(V).Then there exists a positive integer N such that x n∈Bδ(x) for all n>N.It follows that f(x n)∈V=Bε(f(x))for all n>N.Consequently, lim n→∞f(x n)=f(x).3⇒4:Let A be a subset of X.If y∈f(A),then there exists x∈A such that y=f(x).Since x∈A,there exists a sequence(x n)n=1,2,...of A such that lim n→∞x n=x. By statement3we have lim n→∞f(x n)=f(x).It follows that y=f(x)∈f(A).This shows f(A)⊆f(A).4⇒5:Let F be a closed subset of Y,and let A:=f−1(F).By statement4we have f(A)⊆⊆F=F.It follows that A⊆f−1(F)=A.Hence,A is a closed subset of X.5⇒1:Let a∈X andε>0.Consider the closed set F:=Y\Bε(f(a)).By statement5,f−1(F)is a closed subset of X.Since a/∈f−1(F),there exists someδ>0 such that Bδ(a)⊆X\f−1(F).Consequently,ρ(x,a)<δimpliesτ(f(x),f(a))<ε.So f is continuous at a.This is true for every point a in X.Hence,f is continuous on X.As an application of Theorem4.1,we prove the Intermediate Value Theorem for continuous functions.7Theorem 4.2.Suppose that a,b ∈I R and a <b .If f is a continuous function from [a,b ]to I R ,then f has the intermediate value property,that is,for any real number d between f (a )and f (b ),there exists c ∈[a,b ]such that f (c )=d .Proof.Without loss of any generality,we may assume that f (a )<d <f (b ).Since the interval (−∞,d ]is a closed set,the set F :=f −1((−∞,d ])={x ∈[a,b ]:f (x )≤d }is closed,by Theorem 4.1.Let c :=sup F .Then c lies in F and hence f (c )≤d .It follows that a ≤c <b .We claim f (c )=d .Indeed,if f (c )<d ,then by the continuity of f we could find r >0such that c <c +r <b and f (c +r )<d .Thus,we would have c +r ∈F and c +r >sup F .This contradiction shows f (c )=d .The following theorem shows that a continuous function maps compact sets to compact sets.Theorem 4.3.Let f be a continuous function from a metric space (X,ρ)to a metric space (Y,τ).If A is a compact subset of X ,then f (A )is compact.Proof.Suppose that (G i )i ∈I is an open cover of f (A ).Since f is continuous,f −1(G i )is open for every i ∈I ,by Theorem 4.1.Hence,(f −1(G i ))i ∈I is an open cover of A .By thecompactness of A ,there exists a finite subset {i 1,...,i m }of I such that A ⊆∪m k =1f−1(G i k ).Consequently,f (A )⊆∪mk =1G i k .This shows that f (A )is compact.Theorem 4.4.Let A be a nonempty compact subset of a metric space (X,ρ).If f is a continuous function from A to the real line I R ,then f is bounded and assumes its maximum and minimum.Proof.By Theorem 4.3,f (A )is a compact set,and so it is bounded and closed.Let t :=inf f (A ).Then t ∈f (A )=f (A ).Hence,t =min f (A )and t =f (a )for some a ∈A .Similarly,Let s :=sup f (A ).Then s ∈f (A )=f (A ).Hence,s =max f (A )and s =f (b )for some b ∈A .A function f from a metric space (X,ρ)to a metric space (Y,τ)is said to be uni-formly continuous on X if for every ε>0there exists δ>0(depending on ε)such that τ(f (x ),f (y ))<εwhenever ρ(x,y )<δ.Clearly,a uniformly continuous function is continuous.A function from (X,ρ)to (Y,τ)is said to be a Lipschitz function if there exists a constant C f such that τ(f (x ),f (y ))≤C f ρ(x,y )for all x,y ∈X .Clearly,a Lipschitz function is uniformly continuous.8Example.Let f and g be the functions from the interval(0,1]to the real line I R given by f(x)=x2and g(x)=1/x,x∈(0,1],respectively.Then f is uniformly continuous, while g is continuous but not uniformly continuous.Theorem4.5.Let f be a continuous function from a metric space(X,ρ)to a metric space(Y,τ).If X is compact,then f is uniformly continuous on X.Proof.Letε>0be given.Since f is continuous,for each x∈X there exists r x>0suchthatτ(f(x),f(y))<ε/2for all y∈B rx (x).Then(B rx(x))x∈X is an open cover of X.Since X is compact,Theorem3.3tells us that there exists a Lebesgue numberδ>0for this open cover.Suppose y,z∈X andρ(y,z)<δ.Then{y,z}⊆B rx(x)for some x∈X. Consequently,τ(f(y),f(z))≤τ(f(y),f(x))+τ(f(x),f(z))<ε/2+ε/2=ε.This shows that f is uniformly continuous on X.9。
数学分析第一章 1.1汇总

第一章教学安排的说明章节题目:实数集与函数学时分配:共5学时§ 1 实数(1学时)§ 2 数集.确界原理(2学时)§ 3 函数概念 ( 1学时 )§ 4 具有某些特性的函数 (1学时 )教学目的:通过教学,使学生正确理解函数、极限与连续的基本概念,熟练掌握极限的运算。
教学要求:1、掌握实数的各条性质,初步理解上下确界的定义及确界原理的实质。
2、正确理解和掌握函数的概念、性质,四则运算,复合函数,反函数的定义。
3、掌握基本初等函数的性质及其图形。
4、掌握初等函数的性质,了解几个常见非初等函数的定义及性质。
5、理解函数的单调性,周期性,奇偶性等,会对初等函数是否具备这些性质。
其他:注: 第一章大部分内容中学学过。
课堂教学方案课题名称、授课时数:§ 1 实数 1学时§ 2 数集 确界原理 2学时授课类型:理论课教学方法与手段:讲授为主(部分内容自学)教学目的与要求:1.掌握实数的基本概念、基本性质和最常见的不等式,并熟练运用实数的有序性、稠密性和封闭性、实数绝对值的有关性质以及几个常见的不等式2.掌握实数的区间与邻域概念,掌握集合的有界性和确界概念,要求理解实数确界的定义及确界原理,并在有关命题的证明中正确地加以运用。
教学重点: 1.实数集的概念性质及应用,;2.数集有界、无界及确界的概念,确界原理。
教学难点:数集确界的定义及其应用,确界原理的证明。
教学内容首先简要介绍“数学分析”课程的内容:分三个学期;所有内容可分为四部分:1)极限理论,包括数列极限、函数极限及函数的连续性;2)一元函数的微积分,包括导数和微分及其应用、不定积分、定积分及其应用、反常积分;这之间包括第七章实数的完备性;3)级数理论,包括数项级数、函数项级数、幂级数、傅里叶级数;4)多元函数的极限与连续,多元函数的微积分,包括多元函数的偏导数与全微分、隐函数定理及其应用、含参变量积分、二重积分、三重积分、曲线积分及曲面积分.数学分析是数学专业的一门重要理论基础课,在之后要学习的课程:复变函数、常微分方程、实变函数都是它最直接的后继课,学好数学分析对这些后继课程的学习是极其重要的,故一定要打好数学分析课程这个理论基础.第一章 实数集与函数§ 1 实 数复习引新:一、实数集及性质1.实数集:回顾中学中关于实数集的定义.2.实数集性质:四则运算封闭性;三歧性( 即有序性 );Rrchimedes 性; 稠密性: 由有理数和无理数的稠密性, 给出实数稠密性的定义;实数集的 几何表示 ─── 数轴:3.两实数相等的充要条件:b a b a =⇔<->∀εε||,0二. 重要不等式1. 绝对值不等式: 定义[1]P3 的六个不等式.2. 其他不等式:(1)(2) 均值不等式(3) Bernoulli 不等式:有不等式(4) 由二项展开式对有)...2,1(,!)1)...(1()1(n k h C h k k n n n h kk n k n ==+-->+ .在应用时根据需要确定右边的某一项(k 的值)。
数学分析第一章

前页 后页 返回
设
k
是满足
k n
a
的最大的正整数,即
k +1 n
> a.
于是, a < k + 1 < k + 2 < b, 则 k + 1, k + 2 是
nn
nn
a 与 b 之间的有理数, 而 k + 1 + π 是 a 与 b 之间 n 4n
的无理数.
例2 若a,b R,对 > 0,a < b + ,则 a b.
3.实数集的大小关系具有传递性.即若a > b, b > c,则有
a>c.
4.实数具有阿基米德性 , 即对任何 a, b R, 若 b > a > 0
则存在正整数 n, 使得na > b.
5.实数集R具有稠密性.即任何两个不相等的实数之间必 有另一个实数,且既有有理数,也有无理数.
6.实数集R与数轴上的点具有一一对应关系.即任一实数 都对应数轴上唯一的一点,反之,数轴上的每一点也都唯 一的代表一个实数.
证 倘若a > b,设 a b > 0, 则 a b + ,
与 a < b + 矛盾.
前页 后页 返回
(6)实数与数轴上的点一一对应
实数集 R与数轴上的点可建立一一对应关系.
1. 这种对应关系,粗略地可这样描述: 设 P 是数轴上的一点 (不妨设在 0的右边), 若 P 在 整数 n与 n + 1之间,则 a0 n. 把(n, n + 1]十等分, 若点 P 在第 i 个区间,则 a1 i. 类似可得到 an, n 2, 3, L . 这时, 令点 p 对应于 a0 .a1a2 L an L .
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
六. 课程学时与总分
• 课程总学时224学时,14学分, • 具体分配如下: • 第一学期《数学分析(1)》88学时,5.5学分 • 第二学期《数学分析(2)》88学时,5.5学分 • 第三学期《数学分析(3)》48学时,3学分
数学分析的主要内容
变动观点
极限方法 工具 基础
变量
对象
数学分析
内容
关系
预习环节
了解大致内容、熟悉基本结构、找出难 点、试图解决之
听讲环节
会作笔记(概要,重点, 难点,疑点) 、紧跟 讲解、 擅于应答。
复习环节
整理笔记、完成作业、查阅参考书、使 用工具书;
小结环节
写总结(定义、定理、性质、典型解题 方法);制表格 (条件、性质、结论、
几何意义)。
3、重视独立思考,依靠自学取胜;
2. 我们用符号“”表示“存 符号“”称 在”. 为存在量词.
例:命题“对任意的实数x, 都存在实数y, 使得x+y=1”可表示为“xR, yR, 使x+y=1”
3. 我们用符号“”表示“充分条件” 或 “推出” 这一意思. 比如, 若用p, q分别表示两个命题或陈述句. 则“ p q”表示“ 若p成立, 则q也成 立”. 即p是q成立的充分条件.
3、高等(变量)数学时期 (1750年 ~ 1820年) 笛卡尔创建了解析几何;牛顿-莱布尼兹创建了微积分学;
分析学、微分方程、概率论、射影几何取得很大成就。
4、近代数学时期 (1820年~1945年) 非欧几何、集合论导致科学革命;拓扑学、数理逻辑、 复变函数、近世代数、泛函分析、微分几何相继问世。
5、科学数学化时期 (1945年~
)
原子弹、电子计算机、运筹学、模糊数学、数学建模。
马克思:只有成功运用数学时,一门学科才算真正完善。
二、为何要学数学
1、训练思维的需要(数学是思维体操);
2、经济与科技发展的需要(科技是第一生产力, 数学是科技的基础);
3、军事斗争的需要(世一战为化学战、世二战为 物理战、海湾战争为数学战);
4. 我们用符号“”表示“当且仅当” 或 “充要条件” 这一意思. 比如“p q”表示“p成立当且仅当q成 立” 或者说p成立的充要条件是q成立.
一. 集合与实数的性质
1.集合 ❖集合
集合是指具有某种特定性质的事物的总体. 集合可用大写的字母A, B, C, D 等标识. ❖元素 组成集合的事物称为集合的元素. 集合的元素可用小写的字母a, b, c, d 等标识. a是集合M的元素记为aM, 读作a属于M. a不是集合M的元素记为aM, 读作a不属于M.
四. 数学分析简介
• 数学分析是高等学校数理科学专业的一门专业基 础课,通过本课程的教学使学生对极限思想和方 法有较深刻的认识,使学生的思维能力得到锻炼 和提高。特别是基于强化基础、偏重一元微积分 系统知识的教学,学生应能正确理解数学分析的 基本概念,基本掌握数学分析中常用的论证方法, 获得较熟练的演算技能和初步应用的能力。本课 程不仅对许多后继课程的学习有直接影响,而且 对学生数学基本功的训练与良好专业素质的培养 起着十分重要的作用。
1、数学萌芽 (数形) 时期 (公元前2000~公元前600) 贸易、测量、航海的需要而整理形成,如埃及金字塔的 建筑。特点:片断、零散、 缺乏逻辑、没有形成体系。
2、初等(常量) 数学时期 (公元前600~1750年) 古希腊数学科学地位独立;欧氏“几何原本”确立数学成完 整科学;初等几何、算术、代数、三角等成独立学科。
中心 函数
对象
极限论
微分学 积分学
(单变量和多变量)
级数论
教材及参考资料
• 1.教材:数学分析(第三版),欧阳光中,高等 教育出版社
• 2.参考资料 • 1)《数学分析讲义》(第三版),刘玉链等编,
高等教育出版社,1992 • 2)《数学分析学习指导》(上、下册),吴良森
等编,高等教育出版社,2004 • 3)《数学分析的思想方法》,朱匀华等编,中山
4、数学是科学技术的载体,为学习后继课程提供 必须的数学工具(物理、计算机、电子、机械、 经济、运筹、统计、会计等等);
5、未来从事科学研究的需要(数学位于三大重点 基础学科之首,为此硕士研究生入学考分数由 100→150 ) 。
三、如何学好数学;
1、树立自信,亲近数学;
2、抓好四个环节,突出两个重点;
❖集合的表示 •列举法
把集合的全体元素一一列举出来. 例如A{a, b, c, d, e, f, g}. •描述法 若集合M是由元素具有某种性质P的元素x的全体所 组成, 则M可表示为
M{x | x具有性质P }. 例如M{(x, y)| x, y为实数, x2y21}.
❖几个数集 所有自然数构成的集合记为N, 称为自然数集. 所有实数构成的集合记为R, 称为实数集. 所有整数构成的集合记为Z, 称为整数集. 所有有理数构成的集合记为Q, 称为有理集.
大学出版社,1998 • 4)《吉米多维奇数学分析习题集解答》,山东科技出 Nhomakorabea社,1983
第一章 变量与函数
§1 实数 §2 函数的概念 §3 复合函数与反函数 §4 基本初等函数
1.1 实数
一 .集合与实数的性质 二. 绝对值与不等式
几个常用符号
1. 我们用符号“” 表示“任取” 或“对于任意的”或“对于所有的” , 符号“” 称为全称量词.
五. 数学分析与其它课程关系
• 数学分析与另外两门基础课(高等代数、 解析几何)相互协调,并以其自身为主干 构成现代数学各分支的共同基础。几乎所 有专业课都需要该课程的支撑。其后续课 程主要有实变函数、复变函数、泛函分析、 点集拓扑等。它是学习常微分方程、偏微 分方程、概率论、数学模型等应用性较强 课程必备的直接基础,也对数值计算、数 学实验、逻辑学、计算科学等学科的学习 有着潜在的深远影响。
数学分析电子教案
重庆邮电大学数理学院
高等数学教学部
沈世云 62460842 shensy@
数学 不仅是一种工具,
而且是一种思维模式;
数学 不仅是一种知识,
而且是一种素养;
数学 不仅是一种科学,
而且是一种文化;
能否运用数学观念定量思维是衡量 民族科学文化素质的一个重要标志.
一、简明数学史
