甲醇—水汽液平衡

合集下载

甲醇-水精馏化工原理课程设计

10000kg/h 甲醇~水精馏装置设计一、概述..................................................... 错误!未定义书签。

设计依据................................................ 错误!未定义书签。

技术来源................................................ 错误!未定义书签。

设计任务及要求.......................................... 错误!未定义书签。

二、计算过程................................................. 错误!未定义书签。

1 设计方案及设计工艺的确定............................... 错误!未定义书签。

设计方案............................................ 错误!未定义书签。

.设计工艺的确定.......................................... 错误!未定义书签。

、工艺流程简介........................................... 错误!未定义书签。

2. 塔型选择.............................................. 错误!未定义书签。

3. 操作条件的确定........................................ 错误!未定义书签。

操作压力............................................ 错误!未定义书签。

进料状态............................................ 错误!未定义书签。

加热方式的确定....................................... 错误!未定义书签。

化工原理课程设计——水与甲醇精馏精典版

目录前言............................................... 错误!未定义书签。

第一节设计方案.................................................... 5 1.1操作条件的确定 ................................................ 5 1.操作压力的确定 ................................................ 5 2.进料状态 ...................................................... 5 3．加热方式 ..................................................... 6 4.回流比 ........................................................ 6 1.2确定设计方案的原则 ............................................ 7 第二节工艺流程图................................................... 7 第三节板式精馏塔的工艺计算........................................ 8 3.1 物料衡算 ...................................................... 8 3.3 理论塔板数的计算 .............................................. 9 3.4实际板数的确定 ............................................... 11 第四节塔径塔板工艺尺寸的确定...................................... 13 4.1 各设计参数 .. (13)4.1.1 操作压力精m p ............................ 错误!未定义书签。

南工大化工原理第六章习题解答

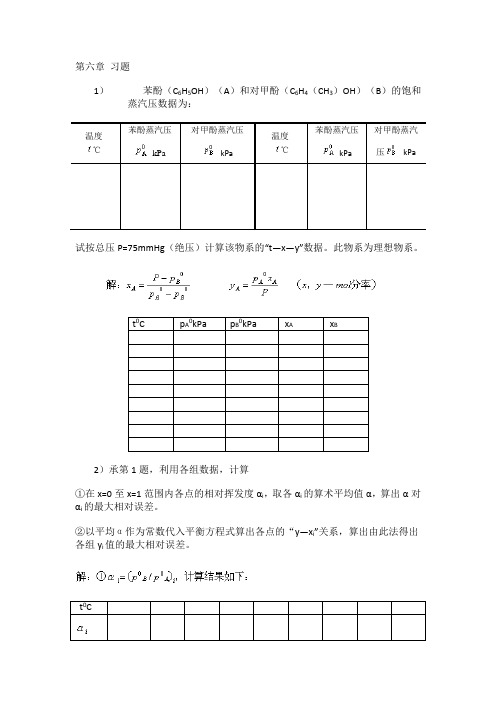
第六章习题1）苯酚（C6H5OH）（A）和对甲酚（C6H4（CH3）OH）（B）的饱和蒸汽压数据为：温度℃苯酚蒸汽压kPa对甲酚蒸汽压kPa温度℃苯酚蒸汽压kPa对甲酚蒸汽压 kPa试按总压P=75mmHg（绝压）计算该物系的“t—x—y”数据。

此物系为理想物系。

t0C p A0kPa p B0kPa x A x B2）承第1题，利用各组数据，计算①在x=0至x=1范围内各点的相对挥发度αi，取各αi的算术平均值α，算出α对αi的最大相对误差。

②以平均α作为常数代入平衡方程式算出各点的“y—x i”关系，算出由此法得出各组y i值的最大相对误差。

t0Ct0Cx i0y i0最大误差=3）已知乙苯（A）与苯乙烯（B）的饱和蒸汽压与温度的关系可按下式算得：式中p0的单位是mmHg，T的单位是K。

问：总压为60mmHg（绝压）时，A与B的沸点各为多少℃在上述总压和65℃时，该物系可视为理想物系。

此物系的平衡汽、液相浓度各为多少摩尔分率4）苯（A）和甲苯（B）混合液可作为理想溶液，其各纯组分的蒸汽压计算式为式中p0的单位是mmHg，t的单位是℃。

试计算总压为850mmHg（绝压）下含苯25%（摩尔百分率）的该物系混合液的泡点。

5）试计算总压为760mmHg（绝压）下，含苯、甲苯（摩尔分率）的混合蒸汽的露点。

若令该二元物系降温至露点以下3℃，求平衡的汽、液相摩尔之比。

6）有一苯（A）、甲苯（B）、空气（C）的混合气体，其中空气占2%，苯与甲苯浓度相等（均指摩尔百分数），气体压强为760mmHg（绝压）。

若维持压强不变，令此三元物系降温至95℃，求所得平衡汽相的组成。

A、B组分均服从拉乌尔定律。

已知95℃时，。

设X A算得的X‘A7）常压下将含苯（A）60%，甲苯（B）40%（均指摩尔百分数）的混合液闪蒸（即平衡蒸馏），得平衡汽、液相，汽相摩尔数占总摩尔数的分率——汽化率（1-q）为。

物系相对挥发度α=，试求：闪蒸所得平衡汽、液相的浓度。

化工基本知识王志魁第五版知识题解答-蒸馏

pA pA x 11.83 0．59 6.98kPa
乙醇分压
pB pB 1 x =5.93 1 0．59 =2.43kPa
总压 p pA pB 6.98 2.43 9.41kPa 甲醇的汽相组成（摩尔分数）为 y 6.98 0.742
9.41 【6-5】总压为 120kPa，正戊烷(A)与正己烷(B)汽相混合物的组成为 0.6（摩尔分数），冷却冷凝到 55℃，汽液相呈平衡状态。试求液相量与汽相量之比值（摩尔比）。此物系为理想物系。55℃下纯组分的饱和蒸
假设 t 70℃，计算 pA 125.31kPa，pB 31.17kPa
液相组成
x
p pB pA pB
101.325 31.17 =0．745 125.31 31.17
0.3
计算的 x 值大于已知的 x 值，故所假设的温度 t 偏小，再假设大些的 t ，重新计算。将 3 次假设的 t 与
假设 t 75℃
计算 pA 151.1kPa，pB 41kPa
液相组成
x
p pB pA pB
101.33 41 151.1 41
0.548
0.4
计算的 x 值大于已知的 x 值，故所假设的温度 t 偏小，重新假设大一点的 t 进行计算。将 3 次假设的 t 与
计算的 x 值列于下表，并在习题 6-3 附图 1 上绘成一条曲线，可知 x 0.4 时的平衡温度 t 79.5℃ 。
方程；（3）计算 y x 的系列相平衡数据，并与习题 6-1 作比较。
解（1） 80.1℃时
1
pA pB
101.33 38.8
2.61
110.6℃时
2
234.6 101.33
2.32

甲醇水汽液平衡

ropt 1.01670 1.01772 1.02178 1.02687 1.03195 1.03703 1.04212 R/Rmin 1.000 1.001 1.005 1.01 1.015 1.02 1.025年总费用1344560.33 1329387.811318482.11314831.5% 2.37% 1.22% 0.39% 0.07% 0.06% 0.08% 0.11% ropt 1.04720 1.05228 1.05533 1.05737 1.06754 1.07770 1.08787 R/Rmin 1.03 1.035 1.038 1.04 1.05 1.06 1.07年总费用% 0.25% 0.36% 0.42% 0.46% 0.77% 1.05% 1.41% ropt 1.09804 1.10820 1.11837 1.22004 1.32171 1.42338 1.52505 R/Rmin 1.08 1.09 1.1 1.2 1.3 1.4 1.5年总费用% 1.73% 2.06% 2.67% 6.57% 10.67% 14.94% 19.25% ropt 1.62672 1.72839 1.83006 1.93173 2.0334 0 0R/Rmin 1.6 1.7 1.8 1.9 2 0 0年总费用1557936.51607201.2% 36.56% 41.37% 46.48% 51.27% 56.16% 31.05% 35.19%附录二甲醇—水汽液平衡数据(摩尔组成)t x y t x y100.00 0.00 0.000 75.30 0.40 0.729 96.40 0.02 0.134 73.10 0.50 0.779 93.50 0.04 0.234 71.20 0.60 0.825 91.20 0.06 0.304 69.30 0.70 0.870 89.30 0.08 0.365 67.60 0.80 0.915 87.70 0.10 0.418 66.00 0.90 0.958 84.40 0.15 0.517 65.00 0.95 0.979 81.70 0.20 0.579 64.50 1.00 1.000 78.00 0.30 0.665附录一甲醇—水系统的主要物理性质附录三优化设计程序源代码优化程序'定义全局变量Dim J1#, J2#, J3#, J4#, JJ#Dim N#, R#, Ropt#Dim lilunbanshu#, jinliaoweizhi%, tajing#, chukouwendu#, chuanremianji#, zongtagao#, tiliuduanbanshu#, jinliuduanbanshu#Dim XF#, F#, q#, XD#, D#, td#, rD#, po#, u#, Rmin#, t1#, Cw#, Cp#, SI#, HETP#Dim Co#, HA#, f1#, f2#, a#, b#, FL#, θ#, ρ#, bo#, Fc#'优化所需参数Public Sub Form_Load()XF = 0.3151: F = 402.34: q = 1XD = 0.982: D = 128.97: td = 64.93: rD = 35373.48: po = 101.3u = 5.4464: Rmin = 1.0167t1 = 20: Cw = 0.0002: Cp = 4.1875: Co = 0.03: cpa = 15674.4HETP = 0.462: HA = 6f1 = 1: f2 = 6.5: a = 487: b = 0.72: SI = 3.73FL = 6.22: θ= 7200: ρ= 7860: bo = 0.005: Fc = 0.125Text1.Text = 402.34Text2.Text = 0.3151Text3.Text = 128.97Text4.Text = 0.982Text5.Text = 35373.48Text6.Text = 64.93Text7.Text = 1Text8.Text = 1.0167Text9.Text = 7200Text10.Text = 3.73Text11.Text = 0.125Text12.Text = 6.22Text13.Text = 0.005Text14.Text = 7860Text15.Text = 5.4464Text16.Text = 0.462Text17.Text = 6Text18.Text = 15674.4Text19.Text = 0.0002Text20.Text = 4.1875Text21.Text = 20Text22.Text = 2000Text23.Text = 1Text24.Text = 6.5Text25.Text = 487Text26.Text = 0.72Text27.Text = 0.03Text28.Text = 1.01Text29.Text = 2Text30.Text = 0.0001Text31.Text = " "Text32.Text = " "Text33.Text = " "Text34.Text = " "Text35.Text = " "Text36.Text = " "Text37.Text = " "Text38.Text = " "Text39.Text = " "Text40.Text = " "Text41.Text = " "Text42.Text = " "Text43.Text = " "Text44.Text = " "Text45.Text = " "Text46.Text = " "Text47.Text = " "End Sub'主程序Private Sub Command1_Click() '菲波拿契法求RoptDim Aa#, Bb#, W#(1 To 50), i%, K%, N#, M%, R1#, R2#, ε# Dim JJ1#, JJ2#Aa = 1.01 * Rmin: Bb = 2 * Rmin '搜索区间[Aa,Bb]W(1) = 1: W(2) = 2: W(3) = 3: i = 1: ε= 0.0001Do While W(i + 2) <= ((Bb - Aa) / ε)i = i + 1W(i + 2) = W(i) + W(i + 1)LoopR1 = Aa + (Bb - Aa) * W(i) / W(i + 2): JJ1 = j(R1)N = i + 2: K = 1: M = 0Do While K <> N - 1If M = 0 ThenR2 = Aa + (Bb - Aa) * W(N - K) / W(N - K + 1)JJ2 = j(R2)ElseR1 = Aa + (Bb - Aa) * W(N - K - 1) / W(N - K + 1)JJ1 = j(R1)End IfIf JJ1 < JJ2 ThenBb = R2: R2 = R1: JJ2 = JJ1: M = 1ElseAa = R1: R1 = R2: JJ1 = JJ2: M = 0End IfK = K + 1LoopR = (Aa + Bb) / 2Ropt = RJJ = j(R)Text31.Text = RoptText32.Text = RminText45.Text = Ropt / RminText33.Text = lilunbanshuText34.Text = zongtagaoText40.Text = J1Text41.Text = J2Text42.Text = J3Text43.Text = J4Text44.Text = JJText37.Text = tajingText38.Text = chukouwenduText39.Text = chuanremianjiText46.Text = Ropt * DText47.Text = (Ropt + 1) * DText35.Text = tiliuduanbanshu * HETPText36.Text = jinliuduanbanshu * HETPEnd Sub'J函数Public Function j(R#) As DoubleCall jjj1(R#, J1#)Call jjj2(R#, J2#)Call jjj3(R#, J3#)Call jjj4(R#, J4#)j = J1 + J2 + J3 + J4End Function'求J1Public Sub jjj1(R#, J1#)Dim DT#, H#, Ws#, CH#Call tabanshu(R#, N#)DT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)H = N * HETP + HAWs = 3.14 * DT * (H + 0.8116 * DT) * bo * ρ'ρ为碳钢的密度CH = FL * Exp(6.95 + 0.1808 * Log(Ws) + 0.02468 * (Log(Ws)) ^ 2 + 0.0158 * H / DT)J1 = SI * (Fc + 0.06) * CHtajing = DTzongtagao = HEnd Sub'求J2Public Sub jjj2(R#, J2#)Dim xx1#, xx0#, CD#, ff#, df#, t2#, AD#, KD#KD = 2000: xx1 = 70Do '牛顿迭代法求冷却水最佳出口温度t2xx0 = xx1CD = 1.3 * SI * a * b * f1 * f2 * Fc * ((R + 1) * D * rD / (td - t1)) ^ (b - 1) / KD ^ bff = -Cw * θ/ Cp + CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (1 - b) * (xx0 - 1 - Log(xx0))df = CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (2 - b) * ((b - 1) * (xx0 - 1 - Log(xx0)) ^ 2 / (xx0 - 1) ^ 2 + Log(xx0))xx1 = xx0 - ff / dfLoop Until Abs(xx1 - xx0) < 0.000001t2 = td - (td - t1) / xx1 't2optchukouwendu = t2AD = (R + 1) * D * rD * Log((td - t1) / (td - t2)) / KD / (t2 - t1) '传热面积chuanremianji = ADJ2 = Cw * θ* (R + 1) * D * rD / Cp / (t2 - t1) + 1.3 * SI * Fc * f1 * f2 * a * AD ^ bEnd Sub'求J3Public Sub jjj3(R#, J3#)Dim Z#, Cz#Cz = 0.03Z = ((R + 1) * D - (1 - q) * F) * 18J3 = Z * Cz * θEnd Sub'求J4Public Sub jjj4(R#, J4#)Dim ho#, cpa!, HETP!cpa = 15674.4: HETP = 0.462Call tabanshu(R#, N#)ho = N * HETPDT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)J4 = 3.14 / 4 * DT ^ 2 * ho * cpa * FcEnd Sub'塔板数的计算Public Sub tabanshu(R#, N#)Dim ye#, XW#Dim X!(100), Y!(100), xx!(100), i%, n1#td = 64.93: F = 402.34: XD = 0.982: XF = 0.3151: ηd = 0.999: D = 128.97: Rmin = 1.0167V = (R + 1) * D: W = F + V - D: XW = (F * XF - D * XD) / Wi = 1: Y(1) = 0.982: X(1) = 0.9702DoIf X(i) > XF ThenY(i + 1) = R * X(i) / (R + 1) + XD / (R + 1)n1 = i + 1 + (X(i) - XF) / (X(i) - X(i + 1))ElseY(i + 1) = W * (X(i) - XW) / VIf X(i) < XW Then Exit DoEnd Ifi = i + 1xx(i) = (Y(i) / (3.3874 * (1 - Y(i)))) ^ (1 / 0.7977)X(i) = xx(i) / (1 + xx(i))LoopN = i - 1 + (X(i - 1) - XW) / (X(i - 1) - X(i))lilunbanshu = Ntiliuduanbanshu = n1jinliuduanbanshu = N - n1End Sub调整ROPT程序：'定义全局变量Dim J1#, J2#, J3#, J4#, JJ#Dim N#, R#, Ropt#Dim lilunbanshu#, jinliaoweizhi%, tajing#, chukouwendu#, chuanremianji#, zongtagao#, tiliuduanbanshu#, jinliuduanbanshu#Dim XF#, F#, q#, XD#, D#, td#, rD#, po#, u#, Rmin#, t1#, Cw#, Cp#, SI#, HETP#Dim Co#, HA#, f1#, f2#, a#, b#, FL#, θ#, ρ#, bo#, Fc#'优化所需参数Public Sub Form_Load()XF = 0.3151: F = 402.34: q = 1XD = 0.982: D = 128.97: td = 64.93: rD = 35373.48: po = 101.3u = 5.4464: Rmin = 1.0167t1 = 20: Cw = 0.0002: Cp = 4.1875: Co = 0.03: cpa = 15674.4HETP = 0.462: HA = 6f1 = 1: f2 = 6.5: a = 487: b = 0.72: SI = 3.73FL = 6.22: θ= 7200: ρ= 7860: bo = 0.005: Fc = 0.125Text1.Text = 402.34 Text2.Text = 0.3151 Text3.Text = 128.97 Text4.Text = 0.982 Text5.Text = 35373.48 Text6.Text = 64.93 Text7.Text = 1Text8.Text = 1.0167 Text9.Text = 7200 Text10.Text = 3.73 Text11.Text = 0.125 Text12.Text = 6.22 Text13.Text = 0.005 Text14.Text = 7860 Text15.Text = 5.4464 Text16.Text = 0.462 Text17.Text = 6Text18.Text = 15674.4 Text19.Text = 0.0002 Text20.Text = 4.1875 Text21.Text = 20 Text22.Text = 2000 Text23.Text = 1Text24.Text = 6.5 Text25.Text = 487 Text26.Text = 0.72 Text27.Text = 0.03 Text28.Text = 1.01 Text29.Text = 2Text30.Text = 0.0001 Text31.Text = " " Text32.Text = " " Text33.Text = " " Text34.Text = " " Text35.Text = " " Text36.Text = " " Text37.Text = " " Text38.Text = " " Text39.Text = " " Text40.Text = " " Text41.Text = " " Text42.Text = " " Text43.Text = " " Text44.Text = " "Text45.Text = " "Text46.Text = " "Text47.Text = " "End Sub'主程序Private Sub Command1_Click() '菲波拿契法求Ropt R = Text31.TextRopt = RJJ = j(R)Text32.Text = RminText45.Text = Ropt / RminText33.Text = lilunbanshuText34.Text = zongtagaoText40.Text = J1Text41.Text = J2Text42.Text = J3Text43.Text = J4Text44.Text = JJText37.Text = tajingText38.Text = chukouwenduText39.Text = chuanremianjiText46.Text = Ropt * DText47.Text = (Ropt + 1) * DText35.Text = tiliuduanbanshu * HETPText36.Text = jinliuduanbanshu * HETPEnd Sub'J函数Public Function j(R#) As DoubleCall jjj1(R#, J1#)Call jjj2(R#, J2#)Call jjj3(R#, J3#)Call jjj4(R#, J4#)j = J1 + J2 + J3 + J4End Function'求J1Public Sub jjj1(R#, J1#)Dim DT#, H#, Ws#, CH#Call tabanshu(R#, N#)DT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)If DT < 1 ThenDT = Int(DT * 10 + 1) / 10ElseDT = Int(DT * 5 + 1) * 0.2End IfH = N * HETP + HAWs = 3.14 * DT * (H + 0.8116 * DT) * bo * ρ'ρ为碳钢的密度CH = FL * Exp(6.95 + 0.1808 * Log(Ws) + 0.02468 * (Log(Ws)) ^ 2 + 0.0158 * H / DT)J1 = SI * (Fc + 0.06) * CHtajing = DTzongtagao = HEnd Sub'求J2Public Sub jjj2(R#, J2#)Dim xx1#, xx0#, CD#, ff#, df#, t2#, AD#, KD#KD = 2000: xx1 = 70Do '牛顿迭代法求冷却水最佳出口温度t2xx0 = xx1CD = 1.3 * SI * a * b * f1 * f2 * Fc * ((R + 1) * D * rD / (td - t1)) ^ (b - 1) / KD ^ bff = -Cw * θ/ Cp + CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (1 - b) * (xx0 - 1 - Log(xx0))df = CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (2 - b) * ((b - 1) * (xx0 - 1 - Log(xx0)) ^ 2 / (xx0 - 1) ^ 2 + Log(xx0))xx1 = xx0 - ff / dfLoop Until Abs(xx1 - xx0) < 0.000001t2 = td - (td - t1) / xx1 't2optchukouwendu = t2AD = (R + 1) * D * rD * Log((td - t1) / (td - t2)) / KD / (t2 - t1) '传热面积chuanremianji = ADJ2 = Cw * θ* (R + 1) * D * rD / Cp / (t2 - t1) + 1.3 * SI * Fc * f1 * f2 * a * AD ^ bEnd Sub'求J3Public Sub jjj3(R#, J3#)Dim Z#, Cz#Cz = 0.03Z = ((R + 1) * D - (1 - q) * F) * 18J3 = Z * Cz * θEnd Sub'求J4Public Sub jjj4(R#, J4#)Dim ho#, cpa!, HETP!cpa = 15674.4: HETP = 0.462Call tabanshu(R#, N#)ho = N * HETPDT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)J4 = 3.14 / 4 * DT ^ 2 * ho * cpa * FcEnd Sub'塔板数的计算Public Sub tabanshu(R#, N#)Dim ye#, XW#Dim X!(100), Y!(100), xx!(100), i%, n1#td = 64.93: F = 402.34: XD = 0.982: XF = 0.3151: ηd = 0.999: D = 128.97: Rmin = 1.0167 V = (R + 1) * D: W = F + V - D: XW = (F * XF - D * XD) / Wi = 1: Y(1) = 0.982: X(1) = 0.9702DoIf X(i) > XF ThenY(i + 1) = R * X(i) / (R + 1) + XD / (R + 1)n1 = i + 1 + (X(i) - XF) / (X(i) - X(i + 1))ElseY(i + 1) = W * (X(i) - XW) / VIf X(i) < XW Then Exit DoEnd Ifi = i + 1xx(i) = (Y(i) / (3.3874 * (1 - Y(i)))) ^ (1 / 0.7977) X(i) = xx(i) / (1 + xx(i))LoopN = i - 1 + (X(i - 1) - XW) / (X(i - 1) - X(i))lilunbanshu = Ntiliuduanbanshu = n1jinliuduanbanshu = N - n1End Sub目录1 前言------------------------------------------------------------------------------------------------12 方案论证2.1 精馏塔类型----------------------------------------------------------------------------------1 2.2 精馏压力-------------------------------------------------------------------------------------1 2.3 进料方式-------------------------------------------------------------------------------------1 2.4 填料类型-------------------------------------------------------------------------------------2 2.5 加热方式-------------------------------------------------------------------------------------22.6 塔材料类型----------------------------------------------------------------------------------23 数学模型的建立3.1 精馏塔塔体年投资折旧费及维修费用-------------------------------------------------3 3.2 冷凝器年运转费用-------------------------------------------------------------------------4 3.3 直接蒸汽加热费用-------------------------------------------------------------------------53.4 填料年折旧费-------------------------------------------------- --54 数学模型的求解4.1 数学模型决策变量分析-------------------------------------------------------------------5 4.2 主要工艺参数的求解----------------------------------------------------------------------54.2.1 塔径的计算-----------------------------------------------------------------------------54.2.2 塔板数的计算-------------------------------------------------------------------------64.2.2.1 相平衡关系的表示--------------------------------------------------------------64.2.2.2 N的计算--------------------------------------------------------------------------64.2.3 冷凝器年运转费用的计算------------------ ----------------------- ----------------74.2.3.1 冷却水用量及冷凝器传热面积的计算- -------------------------------------74.2.3.2 冷凝器冷却水最佳出口温度的确定-----------------------------------------74.2.4 直接加热蒸气费用的计算----------------------------------------------------------8 4.3 数学模型的求解------------------------------------------------------- --------------------84.3.1 单变量最优化方法--------------------------------------------- ----------------------84.3.2 优化设计程序框图-------------------------------------------- -----------------------84.3.2.1 函数调用关系--------------------------------------------------------------------95 优化设计计算5.1 数据预处理---------------------------------------------------------------------------------105.1.1 进塔物料的计算----------------------------------------------------------------------105.1.2 塔顶蒸气温度的计算----------------------------------------------------------------105.1.3 等板高度的计算----------------------------------------------------------------------10Ⅰ5.1.4 产品汽化潜热的计算----------------------------------------------------------------115.1.5 最小回流比的确定-------------------------------------------------------------------115.1.6 填料单价的计算----------------------------------------------------------------------115.2. 塔径的计算---------------------------------------------------------------------------------13 5.3 填料层高度的计算-------------------------------------------------------------------------13 5.4 精馏塔塔体年投资折旧费及维修费用的计算-----------------------------------------13 5.5 冷凝器年运转费用的计算----------------------------------------------------------------145.5.1 冷凝器冷却水最佳出口温度的确定----------------------------------------------145.5.2 冷却水用量及冷凝器传热面积的计算-------------------------------------------145.5.3 精馏塔塔体年投资折旧费及维修费用的计算----------------------------------15 5.6 再沸器年运转费用的计算----------------------------------------------------------------15 5.7 填料年折旧费用的计算-------------------------------------------------------------------15 5.8 汽液负荷-------------------------------------------------------------------------------------155.8.1 气相负荷-------------------------------------------------------------------------------155.8.2 液相负荷-------------------------------------------------------------------------------155.9 年总费用与回流比的关系--------------------------------------------------------------156 填料塔水力学性能校核6.1 泛点率校核--------------------------------------------------------------------------------- 17 6.2 径比校核-------------------------------------------------------------------------------------17 6.3 喷淋密度校核-------------------------------------------------------------------------------176.4 填料塔压降----------------------------------------------------------------------------------177 附属设备的设计与选型7.1 塔顶冷凝器--------------------------------------------------------------------------------- 187.1.1 冷凝器传热量-------------------------------------------------------------------------187.1.2 冷凝器传热推动力-------------------------------------------------------------------187.1.3 初估冷凝器传热面积----------------------------------------------------------------197.1.4 冷凝器传热系数的校核-------------------------------------------------------------197.1.5 冷凝器传热面积的校核-------------------------------------------------------------227.1.6 冷凝器壳程、管程流动阻力-------------------------------------------------------22 7.2 接管选型------------------------------------------------------------------------------------ 247.2.1 进料口接管的选型-------------------------------------------------------------------247.2.2 冷却水接管的选型-------------------------------------------------------------------257.2.3 塔顶蒸气接管选型------------------------------------------------------------------ 25Ⅱ7.2.4 塔顶产品接管选型-------------------------------------------------------------------257.2.5 塔底产品接管选型-------------------------------------------------------------------267.2.6 塔顶产品回流接管选型-------------------------------------------------------------267.2.7 塔底加热蒸气接管选型------------------------------------------------------------- 26 7.3 冷却水输送泵7.3.1 塔高的计算---------------------------------------------------------------------------277.3.2 冷却水输送泵选型------------------------------------------------------------------27 7.4 填料支承结构-------------------------------------------------------------------------------28 7.5 液体分布装置-------------------------------------------------------------------------------287.7 液体收集再分布装置----------------------------------------------------------------------298 设计结果汇总------------------------------------------------------------------------------------299 设计心得------------------------------------------------------------------------------------------31 参考文献---------------------------------------------------------------------------------------------- 31 附录一甲醇和水部分物性参数-----------------------------------------------------------------32 附录二甲醇—水汽液平衡数据（摩尔组成）-------------------------------------------------33 附录三优化设计程序源代码--------------------------------------------------------------------34化工原理课程设计学生姓名：黄圣楠学号：081000115专业班级：10级生工（1）班____指导教师：张星___2013年1月24日。

甲醇乙醇水汽液平衡

This article was downloaded by: [Dalhousie University]On: 15 January 2013, At: 07:11Publisher: Taylor & FrancisInforma Ltd Registered in England and Wales Registered Number: 1072954 Registered office: Mortimer House, 37-41 Mortimer Street, London W1T 3JH, UKPhysics and Chemistry of Liquids: AnInternational JournalPublication details, including instructions for authors andsubscription information:/loi/gpch20Phase equilibria of binary mixturescontaining methyl acetate, water,methanol or ethanol at 101.3 k PaV.H. Álvarez a , S. Mattedi b , M. Iglesias c , R. Gonzalez-Olmos c &J.M. Resa da Chemical Engineering School, State University of Campinas, P.O.Box 6066, Campinas-SP 13081-970, Brazilb Chemical Engineering Department, Polytechnic School, FederalUniversity of Bahia, Rua Aristides Novis, 2 Federação, 40210-630Salvador-BA, Brazilc PF&PT Research T eam, Department of Chemical Engineering,T echnical High School of Engineering, University of Santiago deCompostela, Rúa Lope Gómez de Marzoa, 15782 Santiago deCompostela, Españad Departamento de Ingeniería Química, Universidad del PaísVasco, Apartado 450, 01006 Vitoria, EspañaVersion of record first published: 27 Jan 2011.PLEASE SCROLL DOWN FOR ARTICLEsources. The publisher shall not be liable for any loss, actions, claims, proceedings,demand, or costs or damages whatsoever or howsoever caused arising directly or indirectly in connection with or arising out of the use of this material.D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013Physics and Chemistry of Liquids Vol.49,No.1,January 2011,52–71Phase equilibria of binary mixtures containing methyl acetate,water,methanol or ethanol at 101.3kPaV.H.Alvarez a ,S.Mattedi b *,M.Iglesias c ,R.Gonzalez-Olmos c and J.M.Resa d aChemical Engineering School,State University of Campinas,P.O.Box 6066,Campinas-SP 13081-970,Brazil;b Chemical Engineering Department,Polytechnic School,Federal University of Bahia,Rua Aristides Novis,2Federac ¸a ˜o,40210-630Salvador-BA,Brazil;cPF&PT Research Team,Department of Chemical Engineering,Technical High School of Engineering,University of Santiago de Compostela,Ru´a Lope Go ´mez de Marzoa,15782Santiago de Compostela,Espan ˜a;dDepartamento de Ingenierı´aQuı´m ica,Universidad del Paı´s Vasco,Apartado 450,01006Vitoria,Espan ˜a(Received 3April 2009;final version received 1May 2009)Isobaric vapor–liquid equilibria data at 101.3kPa were reported for the binary mixtures (methyl acetate þ(water or methanol or ethanol),methanol þ(water or ethanol)and (ethanol þwater)).The experimental data were tested for thermodynamic consistency by means of the Wisniak method and were demonstrated to be consistent.The experimental data were correlated using Wilson,NRTL and UNIQUAC models for the activity coefficients and predicted using the UNIFAC and PSRK equation of state for testing theirs capability.The results show that the obtained data for the studied binary systems are more reliable than other published data.Keywords:phase equilibria;associating binary mixture;correlation,modelling errors1.IntroductionThermodynamic measurements and phase equilibria of ethanol,water and the different flavour components (alcohols,aldehydes and acetates,so-called congeners)in distillated alcoholic beverages are of practical interest to the food industry since industrial procedures applied are closely related to their temperature and pressure dependence in order to obtain a high quality final product.In the last few years,published studies have highlighted a clear need for accurate information about these types of mixtures,in order to develop and optimise industrial techniques.Despite the considerable effort invested in the field of thermodynamic properties,a great scarcity of data is observed in the available literature for mixtures of components present in commercial distillated alcoholic beverages.Such properties are strongly dependent on hydrogen bond potency of hydroxyl or polar groups,chain length,isomeric structures and molecular package.After decades of study,there is still much room for improvement in our ability to understand the behavior of these systems and add accurate data to the available literature.Simulation and optimisation are not used in*Corresponding author.Email:silvana@ufba.brISSN 0031–9104print/ISSN 1029–0451online ß2011Taylor &FrancisDOI:10.1080/00319100903012403D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013the right manner in this matter,with an overestimation of equipment size or high energy-consuming conditions being usually applied due to inaccurate calculations.The difficulties of simulation in these types of processes,as well as possible errors derived from that,have been commented upon previously [1].As a continuation of previous work related to alcoholic beverages [2–4],this work is part of a research project whose objective is to measure thermodynamic properties and vapour–liquid equilibrium (VLE)data for different systems involved in most distillation processes to benefit subsequent studies of modelling and simulation.In this work,the VLE at 101.3kPa was determined for binary systems:methyl acetate þwater,methyl acetate þmethanol,methyl acetate þethanol,methanol þwater,methanol þethanol and ethanol þwater.These mixtures also have some special characteristics.The concentration of the solute in the vapor phase is small and shows molecular association.Thermodynamic consistency was achieved to validate the new experimental data.In this way,data obtained have lower deviations when compared with previously published data;thereby,the information of available literature was improvement.The –’approximation was used to fit the experimental data and obtain the UNIFAC Dortmund model [5],which was used for VLE prediction.Also,the predictive Soave–Redlich–Kwong (PSRK)model proposed by Holderbaum and Gmehling [6]was used in the ’–’approximation.2.Experimental sectionAll chemicals were Lichrosolv quality (Merck Farma y Quımica S.A.).The pure components were recently acquired and kept in an inert argon atmosphere after the bottles were opened.The materials were degassed ultrasonically and dried over molecular sieves Type 4A or 3A,1/16in.Chromatographic (GLC)analysis gave purities of 0.998for methyl acetate,methanol and ethanol,with maximum water contents of 6.8Â10À3, 1.5Â10À2and 2.2Â10À2mass%(Metrohm 737KF coulometer),respectively.Water was millipore quality with organic total mass 55ppb and resistivity of 18.2M cm.The densities and refractive indices at 298.15K,as well as normal boiling points,were within recommended values and are shown in Table 1.Table 1.Observed physical properties of pure compounds and literature data (densities ( ),refractive indices (n D )at 298.15K,and normal boiling points (T b )).Mw (kg kmol À1)(kg m À3)n DT b (K)Obs.Lit.Obs.Lit.Obs.Lit.Methyl acetate 74.080a 0.926740.9273b 1.35850 1.3589b 329.82330.4a 0.9279c 1.3614c 330.09d Water 18.015a 0.99700.99705c 1.33250 1.33250c 373.15373.15a Methanol 32.042a 0.786650.78664b 1.32645 1.32652b 337.86337.7a 0.78664c 1.32652c 337.85d Ethanol46.069a0.785020.78509b 1.359221.35941b 352.07351.4a 0.78504c1.35941c351.44dNote:a See [7];b See [8];c See [9];d See [10].Physics and Chemistry of Liquids 53D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013The system used to measure VLE data was a dynamic recirculating apparatusdescribed previously [11,12].The equilibrium temperature was measured with a digital platinum 100resistance thermometer with an accuracy of Æ0.1K.For the pressure measurement,a digital manometer regulator (Divatronic DT1model),manufactured by Leybold with an accuracy of Æ0.1kPa,was used.Both vapour and liquid phase compositions for the systems were determined by measurements of physical properties (density and refractive index)and application of mathematical correlations,published earlier by the authors [13–16].The accuracy of the composition measurements on each phase was estimated as better than Æ0.001in molar fraction for each mixture.The VLE experimental data at 101.3kPa of the studied binary systems are compiled in Table 2.Table 2.Observed vapour-liquid equilibrium data for different binary systems.x 1y 1T (K)1 2 1 2 s 1 s 2Methyl acetate (1)þwater (2)0.0020.14095.6423.732 1.0090.9790.9910.9350.9930.0050.29590.3923.206 1.2260.9780.9920.9410.9940.0140.57777.8121.348 2.0150.9770.9950.9550.9960.0220.68271.2719.808 2.6560.9770.9970.9610.9970.0290.73966.9018.413 3.2160.9770.9990.9650.9970.0420.79461.9016.207 4.0320.977 1.0010.9690.9980.7120.83557.45 1.158 4.9640.978 1.0030.9720.9980.8000.86156.99 1.080 5.0790.978 1.0040.9730.9980.8730.89456.67 1.041 5.1640.979 1.0060.9730.9980.8730.89556.67 1.041 5.1640.979 1.0060.9730.9980.9300.93356.54 1.024 5.2070.981 1.0080.9730.9980.9910.98956.621.0205.2030.983 1.0110.9730.998Methyl acetate (1)þmethanol (2)0.0090.02764.00 2.417 1.0090.9750.9830.9670.9830.0540.14561.90 2.259 1.0960.9750.9830.9690.9840.0740.18661.14 2.194 1.1300.9750.9830.9690.9840.1030.24060.12 2.101 1.1770.9740.9830.9700.9850.1040.24260.09 2.097 1.1780.9740.9830.9700.9850.1210.26959.58 2.048 1.2030.9740.9830.9710.9850.1230.27259.52 2.042 1.2060.9740.9830.9710.9850.1450.30658.88 1.978 1.2380.9740.9840.9710.9860.1480.30958.82 1.971 1.2410.9740.9840.9710.9860.1650.33258.39 1.924 1.2630.9750.9840.9720.9860.1990.37357.63 1.834 1.3040.9750.9850.9720.9860.2160.39157.30 1.794 1.3220.9750.9850.9720.9860.2660.43856.45 1.680 1.3700.9750.9860.9730.9870.2950.46256.05 1.621 1.3930.9760.9860.9730.9870.3270.48655.65 1.558 1.4170.9760.9870.9730.9870.3540.50555.36 1.509 1.4350.9760.9880.9740.9870.3710.51655.19 1.480 1.4460.9760.9880.9740.9870.4190.54554.80 1.406 1.4710.9770.9890.9740.9870.4400.55754.65 1.375 1.4800.9770.9890.9740.9870.4850.58254.37 1.3151.4990.9780.9900.9740.988(Continued )54V.H.A´lvarez et al.D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013Table 2.Continued.x 1y 1T (K) 1 2 1 2 s 1 s 20.5190.59954.21 1.274 1.5100.9780.9900.9740.9880.5370.60954.13 1.254 1.5160.9780.9910.9750.9880.6320.65853.87 1.164 1.5350.9790.9930.9750.9880.6360.66053.86 1.160 1.5360.9790.9930.9750.9880.6730.68053.83 1.132 1.5390.9800.9930.9750.9880.6960.69353.83 1.116 1.5400.9800.9940.9750.9880.7190.70753.84 1.102 1.5400.9810.9950.9750.9880.7420.72153.87 1.089 1.5390.9810.9950.9750.9880.7950.75754.02 1.063 1.5320.9830.9970.9750.9880.8850.83754.65 1.035 1.4980.986 1.0010.9740.9870.9240.88155.12 1.030 1.4730.988 1.0030.9740.9870.9810.96556.191.029 1.4160.992 1.0080.9730.987Methyl acetate (1)þethanol (2)0.0110.05076.972.541 1.0110.9790.9790.9560.9800.0390.16574.47 2.420 1.1160.9780.9780.9580.9810.0890.31070.97 2.231 1.2870.9790.9790.9610.9830.1210.37869.19 2.121 1.3850.9790.9800.9630.9840.1740.46566.77 1.955 1.5350.9800.9810.9650.9850.2570.55864.02 1.734 1.7290.9820.9830.9670.9870.2670.56663.75 1.711 1.7490.9820.9830.9670.9870.2920.58763.11 1.653 1.7990.9820.9840.9680.9870.3160.60562.58 1.604 1.8420.9830.9840.9680.9870.3250.61162.39 1.585 1.8580.9830.9840.9680.9870.3360.61962.17 1.563 1.8760.9830.9850.9690.9870.3690.63961.55 1.502 1.9280.9840.9850.9690.9880.3740.64261.46 1.493 1.9360.9840.9850.9690.9880.4370.67660.48 1.392 2.0240.9850.9870.9700.9880.5340.72259.28 1.266 2.1380.9860.9880.9710.9890.5510.73059.10 1.248 2.1560.9860.9890.9710.9890.5760.74058.85 1.223 2.1810.9870.9890.9710.9890.6360.76758.30 1.169 2.2380.9880.9900.9720.9890.6370.76758.29 1.168 2.2390.9880.9900.9720.9890.6610.77858.09 1.150 2.2600.9880.9910.9720.9890.6920.79257.84 1.128 2.2870.9890.9910.9720.9890.6990.79657.79 1.124 2.2920.9890.9920.9720.9890.7520.82157.42 1.094 2.3340.9900.9930.9720.9890.7600.82557.37 1.089 2.3400.9900.9930.9720.9890.7650.82857.34 1.087 2.3430.9910.9930.9720.9890.7680.83057.32 1.085 2.3450.9910.9930.9720.9890.8080.85157.09 1.069 2.3730.9920.9940.9720.9900.8160.85657.04 1.066 2.3790.9920.9950.9730.9900.8610.88456.84 1.052 2.4040.9930.9960.9730.9900.8620.88456.83 1.052 2.4050.9930.9960.9730.9900.8820.89856.75 1.047 2.4160.9940.9970.9730.9900.9240.93056.64 1.040 2.4330.9960.9990.9730.990Methanol (1)þwater (2)0.00010.00199.65 2.425 1.0130.9860.9920.9560.9920.0010.00999.46 2.384 1.0190.9860.9920.9560.9920.0100.07597.79 2.313 1.0820.9850.9920.9580.9920.0640.32190.812.0171.4000.9850.9930.9640.994(Continued )Physics and Chemistry of Liquids55D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013Table 2.Continued.x 1y 1T (K) 1 2 1 2 s 1 s 20.1030.42587.38 1.8511.5960.9850.9940.9670.9940.2170.59681.04 1.5162.0520.9860.9960.9720.9950.3060.67277.93 1.355 2.3310.9870.9970.9740.9960.3160.67977.62 1.340 2.3610.9870.9980.9750.9960.3830.72275.80 1.256 2.5470.9880.9990.9760.9960.4430.75774.35 1.198 2.7080.988 1.0000.9770.9960.4440.75774.32 1.197 2.7120.988 1.0000.9770.9960.5320.80272.44 1.133 2.9390.989 1.0010.9780.9970.6320.84770.52 1.0843.1950.990 1.0020.9790.9970.6760.86769.73 1.068 3.3070.991 1.0030.9800.9970.6890.87269.49 1.064 3.3430.991 1.0030.9800.9970.6960.87569.37 1.062 3.3600.991 1.0030.9800.9970.7680.90668.12 1.044 3.5520.992 1.0040.9810.9970.7700.90668.11 1.043 3.5530.992 1.0040.9810.9970.8270.93067.16 1.034 3.7080.992 1.0050.9810.9970.8960.95866.05 1.026 3.8980.993 1.0060.9820.9970.9140.96665.75 1.025 3.9520.993 1.0070.9820.9970.9330.97365.46 1.0244.0040.993 1.0070.9820.9970.9370.97565.40 1.024 4.0150.993 1.0070.9820.9970.9720.98964.86 1.023 4.1150.994 1.0080.9830.9970.9770.99164.78 1.023 4.1300.994 1.0080.9830.9970.9770.99164.771.023 4.1320.994 1.0080.9830.997Methanol (1)þethanol (2)0.0180.03477.71 1.152 1.0060.9850.9790.9750.9790.0960.16776.08 1.139 1.0730.9860.9790.9760.9800.1710.27774.65 1.127 1.1360.9870.9790.9770.9810.1790.28974.49 1.126 1.1430.9870.9790.9770.9810.1820.29374.44 1.125 1.1450.9870.9790.9770.9810.2460.37673.31 1.115 1.1990.9880.9800.9780.9820.2750.41172.83 1.110 1.2230.9890.9800.9780.9820.2870.42672.62 1.108 1.2340.9890.9800.9780.9820.2940.43472.51 1.107 1.2390.9890.9800.9780.9820.3120.45572.22 1.104 1.2540.9900.9810.9780.9820.3260.46972.01 1.102 1.2650.9900.9810.9780.9830.4000.54770.90 1.090 1.3250.9910.9820.9790.9830.4230.56970.57 1.087 1.3440.9920.9820.9790.9830.4420.58770.31 1.084 1.3590.9920.9830.9790.9830.4590.60270.09 1.081 1.3710.9930.9830.9800.9840.5340.66769.12 1.070 1.4290.9950.9840.9800.9840.5690.69668.69 1.065 1.4560.9950.9850.9800.9840.5800.70568.56 1.063 1.4640.9960.9850.9800.9840.5980.71968.35 1.061 1.4780.9960.9850.9810.9850.5990.72068.34 1.060 1.4780.9960.9850.9810.9850.6820.78267.42 1.050 1.5390.9980.9870.9810.9850.7260.81366.98 1.044 1.5690.9990.9880.9810.9850.7610.83866.63 1.041 1.593 1.0000.9890.9820.9850.7630.83966.61 1.041 1.595 1.0000.9890.9820.9850.8760.91665.57 1.032 1.671 1.0040.9910.9820.9860.9410.95965.041.030 1.712 1.0060.9930.9820.986Ethanol (1)þwater (2)0.0150.17095.99 5.8380.9740.9810.9910.9650.9930.0320.27692.695.1331.0990.9800.9920.9680.993(Continued )56V.H.A´lvarez et al.D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 20133.Data treatment3.1.VLE consistency dataPhase equilibrium data should be tested in order to assure and guarantee an acceptable quality and reliability of VLE data.Available literature offers different procedures to test the thermodynamic consistency of a set of data for isothermal or isobaric condition.The thermodynamic consistency of the measured VLE data have been tested with the Wisniak method [17]to reject possible inconsistent equilibrium points from the experimental determined collection.According to this test,two experimental points (a)and (b)are thermodynamically consistent when:D 5D maxð1ÞTable 2.Continued.x 1y 1T (K) 1 2 1 2 s 1 s 20.0460.33690.68 4.624 1.1860.9800.9920.9690.9940.0680.39888.50 3.978 1.2880.9800.9920.9710.9940.0790.42087.72 3.717 1.3280.9800.9920.9720.9940.1190.47385.80 2.991 1.4300.9790.9930.9730.9950.1630.50884.54 2.454 1.5030.9790.9930.9740.9950.1900.52483.99 2.215 1.5360.9790.9940.9750.9950.2060.53283.72 2.094 1.5530.9790.9940.9750.9950.2320.54483.33 1.933 1.5770.9790.9940.9750.9950.2360.54683.27 1.908 1.5810.9790.9940.9750.9950.2390.54783.23 1.896 1.5840.9790.9940.9750.9950.2810.56582.70 1.698 1.6180.9790.9940.9760.9950.2860.56782.64 1.675 1.6220.9790.9940.9760.9950.2910.56982.59 1.658 1.6250.9790.9940.9760.9950.3030.57482.45 1.614 1.6340.9790.9950.9760.9950.3440.59082.01 1.486 1.6630.9790.9950.9760.9950.3670.59981.79 1.427 1.6780.9790.9950.9760.9950.3800.60581.66 1.396 1.6870.9790.9950.9760.9950.3920.61081.55 1.371 1.6950.9790.9950.9760.9950.3970.61281.50 1.360 1.6980.9790.9950.9770.9950.4100.61781.38 1.336 1.7070.9790.9950.9770.9950.4120.61881.36 1.332 1.7080.9790.9950.9770.9950.4810.64880.76 1.224 1.7510.9800.9960.9770.9950.5270.66980.40 1.171 1.7770.9800.9960.9770.9950.6170.71579.77 1.094 1.8250.9800.9980.9780.9960.6880.75479.36 1.053 1.8570.9810.9990.9780.9960.7220.77579.19 1.037 1.8710.9810.9990.9780.9960.7570.79779.04 1.023 1.8840.982 1.0000.9780.9960.8510.86278.770.997 1.9090.983 1.0020.9780.9960.8980.89978.720.988 1.9150.984 1.0030.9780.9960.9080.90878.720.987 1.9160.984 1.0040.9780.9960.9310.92878.730.984 1.9160.984 1.0040.9780.9960.9440.94178.750.983 1.9160.985 1.0050.9780.9960.9470.94378.750.983 1.9160.985 1.0050.9780.9960.9670.96378.790.9821.9140.9851.0060.9780.996x 1,Liquid-phase mole fraction;y 1,vapour-phase mole fraction;T ,boiling temperature; 1and2,activity coefficients; 1and 2,fugacity coefficients; s 1and s2,fugacity coefficients at saturation at 101.3kPaPhysics and Chemistry of Liquids57D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013where D max is the maximum deviation with a value of 3,D is the local deviation,which is expressed as:D ¼100L ÀW L þW,ð2Þwhere L and W are each side temperature function integrals on liquid compositionfor the Wisniak test [13].The correlations for heat of vapourisation (J kmol À1)and density liquid (kmol m À3)used are:D vap H ¼A 1ÀT r ðÞðB þCT r þDT 2r Þð3Þ ¼AB 1þð1ÀT =C ÞDðÞ,ð4Þwhere,T is the temperature in K,T r is the reduced temperature and the constants A ,B ,C and D are shown in Table 3.The physical properties used were taken from Diadem Public v1.2[10],and the activity coefficients were calculated as shown in the next section.Table 4shows the values for the integrals L and W calculated for the thermodynamic consistent test and the values for the deviation D .Also,this table shows that the condition D 5D max satisfies all systems.Therefore,the thermo-dynamic consistency of the binary VLE data reported in this work is confirmed.Table 3.Coefficients for heat of vapourisation and density liquid,Equations (3)and (4).Compound D T (K)A B C D Ethanol 159–514a557890000.3124500159–514b 1.62880.274695140.23178Methanol 175–512a 504510000.3359400175–512b 2.32670.27073512.50.24713Methyl acetate 175–506a 449200000.368500175–506b 1.130.2593506.550.2764Water273–647a 520530000.31990À0.2120.25795300–380b,c5.77830.3124462.25450.05977aInterval for heat vapourisation,b interval for liquid density,c calculated from [14].Table 4.Results of the thermodynamic consistency test;L,W and D are variables defined in Equation (2).System (1)þ(2)L W D Methyl acetate þwater 19.8420.30 1.15Methyl acetate þmethanol 5.10 5.00 1.05Methyl acetate þethanol 6.59 6.81 1.61Methanol þwater 7.527.560.30Methanol þethanol 1.48 1.470.18Ethanol þwater7.217.260.3758V.H.A´lvarez et al.D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 20133.2.Equilibrium equation and activity coefficientsThe activity coefficients ( i )of the components were calculated from the following equation:i ¼y i Èi Px i P o i,ð5Þwere x i and y i are the liquid and vapor mole fractions in equilibrium,Èi is the vaporphase correction factor,P is the total pressure and P o i is the vapour pressure of pure component i .These vapour pressures were calculated from the Antoine equation:log P ¼A i ÀB ii,ð6Þwhere,P is the vapor pressure in mmHg,T is temperature in C and the constants A i.,B i and C i are reported in Table 5.The value constants for the pure compounds were obtained in literature by Riddick et al .[9].The vapour phase correction factor is given by:Èi ¼ i sat i exp ÀV i ðP ÀP o iÞRT !,ð7Þwhere i is the fugacity coefficient of component i in the mixture, sat iis the fugacity coefficient at saturation condition and V i is the molar volume of component i in the liquid phase calculated using the correlation of the liquid density.Fugacity coefficients were calculated with PSRK model,where the expression proposed by Mathias and Copeman [18]is used to evaluate (T )in the PSRK model:ðT Þ¼1þc 1ð1ÀT 0:5r Þþc 2ð1ÀT 0:5r Þ2þc 3ð1ÀT 0:5r Þ3ÂÃ2for T r 51,ð8Þwhere,T r is the reduced temperature and T c is the critical temperature,while c 1,c 2and c 3are empirical parameters.These parameters for the pure compounds were calculated in this work and are shown in Table 5.The physical properties for the pure components used in the PSRK model were taken from [10]and shown in Table 6.The calculated fugacity and activity coefficients are shown in Table 2for all data points.Table 5.Antoine and Mathias and Copeman pound A i a B i a C i a D T (K)b C 1c c 2c C 3cEthanol 8.3221718.10237.52296.9–463.2 1.4125300.287222À1.496099Methanol7.8981474.08229.13292.0–461.3 1.433991À0.7681150.226212Methyl acetate 7.0651157.622219.724277.1–462.7 1.069537À0.759819 1.492479Water8.0121695.167230.41276.6–590.91.093544À0.6730560.699288aSee [19];b see [18];c calculated in this work.Physics and Chemistry of Liquids59D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 20133.3.Modelling –Correlation modelThe VLE data were correlated in the –’approximation,where the PSRK equation of state was used to evaluate the fugacity coefficients,as the thermodynamic model in a bubble-point calculation.The description of the models applied here (Wilson,NRTL,and UNIQUAC)is freely available in the literature [7]and hence it is not discussed here.In the ’approximation,the Wilson,NRTL and UNIQUAC models were used instead of the UNIFAC model to calculate the excess Gibbs energy in the PSRK model.Theoretically,the range for the parameters A ij (Wilson,NRTL and UNIQUAC)is defined as (À104,104)J mol À1.Since this is a very wide range based on physical considerations,it is extremely likely that it will contain the globally optimal parameter values.Renon and Prausnitz [18]explain that the range for ij with theoretical bases can have values from 0.2to 0.55.To evaluate these parameters,the regression was performed using a genetic algorithm code,implemented and fully explained in the study by Alvarez et al .[19],with the minimisation of the overall objective function (Q ).Q ¼X N j ¼1½y exp 1jÀy cal 1j = y2þX N j ¼1½T cal ÀT exp = T ÀÁ2,ð9Þwhere y is the accuracy in the vapour mole fraction (10À3), T is the accuracy in the temperature (10À1),N is the number of data sets,y i is the molar fraction of the component i and the superscript ‘exp’and ‘cal’are the experimental and calculated values,respectively.The fitting parameters of these models and deviations are shown Table 7,the relative percent deviations in temperature and vapour phase compositions are calculated by Valderrama and Alvarez [20]:D T j j %¼100N XN i ¼1T cali ÀT exp i T expi ð10ÞD y %¼100N X N i ¼1y cal i Ày exp i y exp i,ð11Þwhere N is the number of data sets,T is the temperature,y i is the vapour molarfraction of the component i and the superscript ‘exp’and ‘cal’are the experimental and calculated values,respectively.Also,this table shows that all models present similar deviations in temperature and concentration in vapour phase,with a slightly better performance of the UNIQUAC model.The modelling of VLE data areTable 6.Physical properties for components:T c,critical temperature;P c,critical pressure;!,acentric factor;and uniquac parameters r and q .Compound T c (K)P c (bar)!r q Ethanol 514.061.50.644 2.11 1.97Methanol512.581.00.566 1.43 1.43Methyl acetate 506.647.50.331 2.80 2.58Water647.1221.20.3450.921.4D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013presented in T Àx 1Ày 1diagrams shown in Figures 1–6.In Figure 7,comparisons between models desviations using Equation (11)are shown for all binary systems,it is easy to observe that UNIQUAC model has a good agreement between experimental and calculated composition vapor phase.Table 7.Correlation parameters for activity coefficients and average deviation for the studied systems.ModelA 12(KJ mol À1)A 21(KJ mol À1)j D T j %(K)j D y 1j %j D y 2j %Methyl acetate (1)þwater (2)Wilson3249.1878660.5650.27 2.9512.96NRTL ( 12¼0.415)3276.9667494.2400.050.73 2.36UNIQUAC c 2216.998959.0940.100.42 1.46UNIFAC ––0.060.75 2.08PSRK––0.39 3.25 6.52Methyl acetate (1)þmethanol (2)Wilson À75.0113815.6350.020.190.47NRTL ( 12¼0.534)1927.7051181.4910.020.190.50UNIQUAC c 2922.085À594.6130.020.220.43UNIFAC ––0.020.310.52PSRK ––0.16 2.63 1.86Methyl acetate (1)þethanol (2)Wilson 467.4572721.9650.020.230.95NRTL ( 12¼0.550)1772.0651504.5650.020.25 1.00UNIQUAC c1667.680À178.6690.030.36 1.03UNIFAC ––0.060.61 1.30PSRK ––0.23 1.93 2.24Methanol (1)þwater (2)Wilson 173.8432373.1030.040.54 3.87NRTL ( 12¼0.550)75.5062425.8120.04 1.01 3.08UNIQUAC c À1289.7642072.2820.050.35 2.12UNIFAC ––0.080.45 1.39PSRK ––0.070.530.77Methanol (1)þethanol (2)Wilson À309.2071565.2990.030.230.66NRTL ( 12¼0.200)4229.869À2822.0700.020.260.60UNIQUACc1412.023À763.4080.030.230.64UNIFAC ––0.020.380.97PSRK ––0.020.440.71Ethanol (1)+water (2)Wilson2083.97763953.53670.250.39 1.16NRTL ( 12¼0.550)734.705007.820.180.92 1.46UNIQUAC c À495.04021988.10910.210.75 1.58UNIFAC ––0.220.330.82PSRK ––0.09 1.84 2.46Mean Wilson 0.100.76 3.34NRTL0.060.56 1.50UNIQUAC 0.070.39 1.21UNIFAC 0.080.47 1.18PSRK0.161.772.43D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013Figure 1.T Àx 1Ày 1diagram for methyl acetate (1)þwater (2)at 101.3kPa:(.)experimentalliquid phase;( )experimental vapour phase;(—)UNIQUAC correlation;(---)UNIFACprediction.Figure 2.T Àx 1Ày 1diagram for methyl acetate (1)þmethanol (2)at 101.3kPa:(.)experimental liquid phase;( )experimental vapour phase;(—)UNIQUAC correlation;(---)UNIFAC prediction.D o w n l o a d e d b y [D a l h o u s i e U n i v e r s i t y ] a t 07:11 15 J a n u a r y 2013。

甲醇-水填料精馏塔的课程设计

摘要：填料塔为连续接触式的气液传质设备，与板式塔相比，不仅结构简单，而且具有生产能力大，分离填料材质的选择，可处理腐蚀性的材料，尤其对于压强降较低的真空精馏操作，填料塔更显示出优越性。

本文以甲醇-水的混合液为研究对象，因甲醇-水系统在常压下相对挥发度相差较大，较易分离，所以此设计采用常压精馏。

根据物料性质、操作条件等因素选择填料塔，此设计采用泡点进料、塔底再沸器和塔顶回流的方式，将甲醇—水进行分离的填料精馏塔。

通过甲醇—水的相关数据，对全塔进行了物料衡算和热料衡算，得出精馏产品的流量、组成和进料流量、组成之间的关系，进而得到精馏塔的理论板数。

分析了进料、塔顶、塔底、提馏段、精馏段的流量及其物性参数。

对精馏段和提留段的塔径及填料层高度进行了计算，以确定塔的结构尺寸。

对塔内管径、液体分布器、筒体壁厚进行了选型计算，从而得到分离甲醇—水混合物液的填料精馏塔。

关键词：填料塔；流量；回流比；理论板数；工艺尺寸第一章：设计任务书 (1)一、设计题目 (1)二、操作条件 (1)三、填料类型 (1)四、设计内容 (2)第二章：工艺设计计算 (2)一、设计方案的确定 (2)二、精馏塔的物料衡算 (3)三、理论塔板数的确定 (3)四、精馏塔的工艺条件及有关物性数据的计算 (8)五、精馏塔塔体工艺尺寸的计算 (10)六、填料层压降的计算 (13)七、筒体壁厚的计算 (14)八、管径的计算 (14)九、液体分布器简要设计 (16)第三章：结论 (18)一、设计感想 (18)二、全章主要主要符号说明 (19)三、参考资料： (20)第一章：设计任务书一、设计题目在抗生素类药物生产过程中，需要用甲醇溶媒洗涤晶体，洗涤过滤后产生废甲醇溶液，其组成为含甲醇46%、水54%（质量分数），另含有少量的药物固体微粒。

为使废甲醇溶液重复利用，拟建立一套填料精馏塔，以对废甲醇溶媒进行精馏得到含水量≤0.3%（质量分数）的甲醇溶媒。

设计要求废甲醇溶媒的处理量为4t/h,塔底废水中甲醇含量≤0.5%（质量分数）。

甲醇水气液平衡数据

甲醇水气液平衡数据介绍甲醇和水是常见的溶液系统，在工业中有广泛的应用。

了解甲醇水溶液中的气液平衡数据对于工艺设计和操作控制都非常重要。

本文将对甲醇水溶液的气液平衡数据进行全面、详细、完整且深入地探讨。

甲醇水溶液的气液平衡甲醇水溶液的气液平衡是指在一定温度和压力下，甲醇和水之间在气相和液相之间的平衡。

这个平衡与溶液的浓度、温度、压力等因素密切相关。

影响因素甲醇水溶液的气液平衡受到以下几个主要因素的影响：1.温度：温度是影响气液平衡的重要因素之一。

随着温度的升高，甲醇水溶液的气相组分增加，液相组分减少。

2.压力：压力对气液平衡的影响主要表现在饱和汽相压力和液相浓度之间的关系上。

3.浓度：甲醇水溶液的浓度对气液平衡有着重要的影响。

随着甲醇浓度的增加，液相的沸点升高，汽相的过饱和度和逸度增加。

实验方法为了获得甲醇水溶液的气液平衡数据，可以采用以下实验方法：1.饱和蒸发法：将甲醇水溶液放入闭口容器中，保持一定温度和压力条件，等待溶液的气液平衡达到稳定。

利用饱和蒸发法可以测定饱和汽相压力和液相浓度。

2.沸点测定法：通过测量甲醇水溶液的沸点来间接获得气液平衡数据。

沸点随着甲醇浓度的升高而升高。

3.硬件模拟：通过建立物理模型，通过模拟计算得到甲醇水溶液的气液平衡数据。

数据分析和应用甲醇水溶液的气液平衡数据对于工业领域具有重要意义。

可以通过数据分析和应用来改进工艺设计和操作控制。

1.蒸馏过程优化：利用甲醇水溶液的气液平衡数据，可以优化蒸馏过程中的操作条件，提高分离效率和产量。

2.故障诊断：通过监测甲醇水溶液的气液平衡数据，可以及时发现系统运行中的问题并进行相应的故障诊断。

3.溶剂选择：在某些工艺中，需要选择合适的溶剂来达到特定的分离效果。

甲醇水溶液的气液平衡数据可以作为选择溶剂的依据。

4.反应控制：在某些反应中，甲醇和水的浓度变化对反应速率和产物选择性有着重要影响。

通过了解甲醇水溶液的气液平衡数据，可以控制反应的过程和结果。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

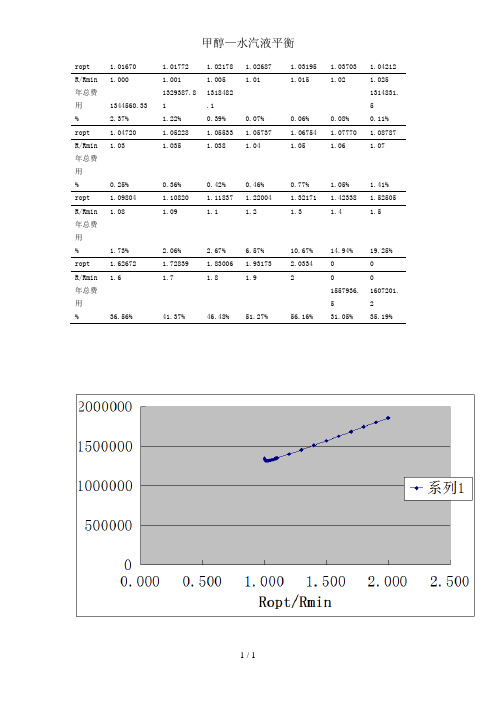
ropt 1.01670 1.01772 1.02178 1.02687 1.03195 1.03703 1.04212 R/Rmin 1.000 1.001 1.005 1.01 1.015 1.02 1.025年总费用1344560.331329387.811318482.11314378131418813144461314831.5% 2.37% 1.22%0.39%0.07%0.06%0.08%0.11% ropt 1.04720 1.05228 1.05533 1.05737 1.06754 1.07770 1.08787 R/Rmin 1.03 1.035 1.038 1.04 1.05 1.06 1.07年总费用1316669131808413189261319446132355513271491331896%0.25%0.36%0.42%0.46%0.77% 1.05% 1.41% ropt 1.09804 1.10820 1.11837 1.22004 1.32171 1.42338 1.52505 R/Rmin 1.08 1.09 1.1 1.2 1.3 1.4 1.5年总费用1336073134051613484191399738145355015096671566271% 1.73% 2.06% 2.67% 6.57%10.67%14.94%19.25% ropt 1.62672 1.72839 1.83006 1.93173 2.033400R/Rmin 1.6 1.7 1.8 1.9200年总费用162348516806581741322179836518565011557936.51607201.2%36.56%41.37%46.48%51.27%56.16%31.05%35.19%附录二甲醇—水汽液平衡数据(摩尔组成)t x y t x y 100.000.000.00075.300.400.729 96.400.020.13473.100.500.779 93.500.040.23471.200.600.825 91.200.060.30469.300.700.870 89.300.080.36567.600.800.915 87.700.100.41866.000.900.958 84.400.150.51765.000.950.979 81.700.200.57964.50 1.00 1.000 78.000.300.665附录一甲醇—水系统的主要物理性质附录三优化设计程序源代码优化程序'定义全局变量Dim J1#, J2#, J3#, J4#, JJ#Dim N#, R#, Ropt#Dim lilunbanshu#, jinliaoweizhi%, tajing#, chukouwendu#, chuanremianji#, zongtagao#, tiliuduanbanshu#, jinliuduanbanshu#Dim XF#, F#, q#, XD#, D#, td#, rD#, po#, u#, Rmin#, t1#, Cw#, Cp#, SI#, HETP# Dim Co#, HA#, f1#, f2#, a#, b#, FL#, θ#, ρ#, bo#, Fc#'优化所需参数Public Sub Form_Load()XF = 0.3151: F = 402.34: q = 1XD = 0.982: D = 128.97: td = 64.93: rD = 35373.48: po = 101.3u = 5.4464: Rmin = 1.0167t1 = 20: Cw = 0.0002: Cp = 4.1875: Co = 0.03: cpa = 15674.4HETP = 0.462: HA = 6f1 = 1: f2 = 6.5: a = 487: b = 0.72: SI = 3.73FL = 6.22: θ= 7200: ρ= 7860: bo = 0.005: Fc = 0.125Text1.T ext = 402.34Text2.T ext = 0.3151Text3.T ext = 128.97Text4.T ext = 0.982Text5.T ext = 35373.48Text7.T ext = 1Text8.T ext = 1.0167 Text9.T ext = 7200 Text10.T ext = 3.73 Text11.T ext = 0.125 Text12.T ext = 6.22 Text13.T ext = 0.005 Text14.T ext = 7860 Text15.T ext = 5.4464 Text16.T ext = 0.462 Text17.T ext = 6Text18.T ext = 15674.4 Text19.T ext = 0.0002 Text20.T ext = 4.1875 Text21.T ext = 20 Text22.T ext = 2000 Text23.T ext = 1Text24.T ext = 6.5 Text25.T ext = 487 Text26.T ext = 0.72 Text27.T ext = 0.03Text29.T ext = 2 Text30.T ext = 0.0001 Text31.T ext = " " Text32.T ext = " " Text33.T ext = " " Text34.T ext = " " Text35.T ext = " " Text36.T ext = " " Text37.T ext = " " Text38.T ext = " " Text39.T ext = " " Text40.T ext = " " Text41.T ext = " " Text42.T ext = " " Text43.T ext = " " Text44.T ext = " " Text45.T ext = " " Text46.T ext = " " Text47.T ext = " " End Sub'主程序Private Sub Command1_Click() '菲波拿契法求RoptDim Aa#, Bb#, W#(1 To 50), i%, K%, N#, M%, R1#, R2#, ε# Dim JJ1#, JJ2#Aa = 1.01 * Rmin: Bb = 2 * Rmin '搜索区间[Aa,Bb]W(1) = 1: W(2) = 2: W(3) = 3: i = 1: ε= 0.0001Do While W(i + 2) <= ((Bb - Aa) / ε)i = i + 1W(i + 2) = W(i) + W(i + 1)LoopR1 = Aa + (Bb - Aa) * W(i) / W(i + 2): JJ1 = j(R1)N = i + 2: K = 1: M = 0Do While K <> N - 1If M = 0 ThenR2 = Aa + (Bb - Aa) * W(N - K) / W(N - K + 1)JJ2 = j(R2)ElseR1 = Aa + (Bb - Aa) * W(N - K - 1) / W(N - K + 1)JJ1 = j(R1)End IfIf JJ1 < JJ2 ThenBb = R2: R2 = R1: JJ2 = JJ1: M = 1 ElseAa = R1: R1 = R2: JJ1 = JJ2: M = 0 End IfK = K + 1LoopR = (Aa + Bb) / 2Ropt = RJJ = j(R)Text31.T ext = RoptText32.T ext = RminText45.T ext = Ropt / RminText33.T ext = lilunbanshuText34.T ext = zongtagaoText40.T ext = J1Text41.T ext = J2Text42.T ext = J3Text43.T ext = J4Text44.T ext = JJText37.T ext = tajingText38.T ext = chukouwenduText39.T ext = chuanremianjiText46.T ext = Ropt * DText47.T ext = (Ropt + 1) * DText35.T ext = tiliuduanbanshu * HETP Text36.T ext = jinliuduanbanshu * HETP End Sub'J函数Public Function j(R#) As DoubleCall jjj1(R#, J1#)Call jjj2(R#, J2#)Call jjj3(R#, J3#)Call jjj4(R#, J4#)j = J1 + J2 + J3 + J4End Function'求J1Public Sub jjj1(R#, J1#)Dim DT#, H#, Ws#, CH#Call tabanshu(R#, N#)DT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)H = N * HETP + HAWs = 3.14 * DT * (H + 0.8116 * DT) * bo * ρ'ρ为碳钢的密度CH = FL * Exp(6.95 + 0.1808 * Log(Ws) + 0.02468 * (Log(Ws)) ^ 2 + 0.0158 * H / DT) J1 = SI * (Fc + 0.06) * CHtajing = DTzongtagao = HEnd Sub'求J2Public Sub jjj2(R#, J2#)Dim xx1#, xx0#, CD#, ff#, df#, t2#, AD#, KD#KD = 2000: xx1 = 70Do '牛顿迭代法求冷却水最佳出口温度t2xx0 = xx1CD = 1.3 * SI * a * b * f1 * f2 * Fc * ((R + 1) * D * rD / (td - t1)) ^ (b - 1) / KD ^ bff = -Cw * θ/ Cp + CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (1 - b) * (xx0 - 1 - Log(xx0))df = CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (2 - b) * ((b - 1) * (xx0 - 1 - Log(xx0)) ^ 2 / (xx0 - 1) ^ 2 + Log(xx0))xx1 = xx0 - ff / dfLoop Until Abs(xx1 - xx0) < 0.000001t2 = td - (td - t1) / xx1 't2optchukouwendu = t2AD = (R + 1) * D * rD * Log((td - t1) / (td - t2)) / KD / (t2 - t1) '传热面积chuanremianji = ADJ2 = Cw * θ* (R + 1) * D * rD / Cp / (t2 - t1) + 1.3 * SI * Fc * f1 * f2 * a * AD ^ b End Sub'求J3Public Sub jjj3(R#, J3#)Dim Z#, Cz#Cz = 0.03Z = ((R + 1) * D - (1 - q) * F) * 18J3 = Z * Cz * θEnd Sub'求J4Public Sub jjj4(R#, J4#)Dim ho#, cpa!, HETP!cpa = 15674.4: HETP = 0.462Call tabanshu(R#, N#)ho = N * HETPDT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)J4 = 3.14 / 4 * DT ^ 2 * ho * cpa * FcEnd Sub'塔板数的计算Public Sub tabanshu(R#, N#)Dim ye#, XW#Dim X!(100), Y!(100), xx!(100), i%, n1#td = 64.93: F = 402.34: XD = 0.982: XF = 0.3151: ηd = 0.999: D = 128.97: Rmin = 1.0167V = (R + 1) * D: W = F + V - D: XW = (F * XF - D * XD) / Wi = 1: Y(1) = 0.982: X(1) = 0.9702DoIf X(i) > XF ThenY(i + 1) = R * X(i) / (R + 1) + XD / (R + 1)n1 = i + 1 + (X(i) - XF) / (X(i) - X(i + 1))ElseY(i + 1) = W * (X(i) - XW) / VIf X(i) < XW Then Exit DoEnd Ifi = i + 1xx(i) = (Y(i) / (3.3874 * (1 - Y(i)))) ^ (1 / 0.7977)X(i) = xx(i) / (1 + xx(i))LoopN = i - 1 + (X(i - 1) - XW) / (X(i - 1) - X(i))lilunbanshu = Ntiliuduanbanshu = n1jinliuduanbanshu = N - n1End Sub调整ROPT程序：'定义全局变量Dim J1#, J2#, J3#, J4#, JJ#Dim N#, R#, Ropt#Dim lilunbanshu#, jinliaoweizhi%, tajing#, chukouwendu#, chuanremianji#,zongtagao#, tiliuduanbanshu#, jinliuduanbanshu#Dim XF#, F#, q#, XD#, D#, td#, rD#, po#, u#, Rmin#, t1#, Cw#, Cp#, SI#, HETP# Dim Co#, HA#, f1#, f2#, a#, b#, FL#, θ#, ρ#, bo#, Fc#'优化所需参数Public Sub Form_Load()XF = 0.3151: F = 402.34: q = 1XD = 0.982: D = 128.97: td = 64.93: rD = 35373.48: po = 101.3u = 5.4464: Rmin = 1.0167t1 = 20: Cw = 0.0002: Cp = 4.1875: Co = 0.03: cpa = 15674.4HETP = 0.462: HA = 6f1 = 1: f2 = 6.5: a = 487: b = 0.72: SI = 3.73FL = 6.22: θ= 7200: ρ= 7860: bo = 0.005: Fc = 0.125Text1.T ext = 402.34Text2.T ext = 0.3151Text3.T ext = 128.97Text4.T ext = 0.982Text5.T ext = 35373.48Text6.T ext = 64.93Text7.T ext = 1Text8.T ext = 1.0167Text9.T ext = 7200Text11.T ext = 0.125 Text12.T ext = 6.22 Text13.T ext = 0.005 Text14.T ext = 7860 Text15.T ext = 5.4464 Text16.T ext = 0.462 Text17.T ext = 6Text18.T ext = 15674.4 Text19.T ext = 0.0002 Text20.T ext = 4.1875 Text21.T ext = 20 Text22.T ext = 2000 Text23.T ext = 1Text24.T ext = 6.5 Text25.T ext = 487 Text26.T ext = 0.72 Text27.T ext = 0.03 Text28.T ext = 1.01 Text29.T ext = 2Text30.T ext = 0.0001 Text31.T ext = " "Text33.T ext = " "Text34.T ext = " "Text35.T ext = " "Text36.T ext = " "Text37.T ext = " "Text38.T ext = " "Text39.T ext = " "Text40.T ext = " "Text41.T ext = " "Text42.T ext = " "Text43.T ext = " "Text44.T ext = " "Text45.T ext = " "Text46.T ext = " "Text47.T ext = " "End Sub'主程序Private Sub Command1_Click() '菲波拿契法求RoptRopt = RJJ = j(R)Text32.T ext = RminText45.T ext = Ropt / RminText33.T ext = lilunbanshuText34.T ext = zongtagaoText40.T ext = J1Text41.T ext = J2Text42.T ext = J3Text43.T ext = J4Text44.T ext = JJText37.T ext = tajingText38.T ext = chukouwenduText39.T ext = chuanremianjiText46.T ext = Ropt * DText47.T ext = (Ropt + 1) * DText35.T ext = tiliuduanbanshu * HETP Text36.T ext = jinliuduanbanshu * HETP End Sub'J函数Public Function j(R#) As DoubleCall jjj1(R#, J1#)Call jjj2(R#, J2#)Call jjj3(R#, J3#)Call jjj4(R#, J4#)j = J1 + J2 + J3 + J4End Function'求J1Public Sub jjj1(R#, J1#)Dim DT#, H#, Ws#, CH#Call tabanshu(R#, N#)DT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po) If DT < 1 ThenDT = Int(DT * 10 + 1) / 10ElseDT = Int(DT * 5 + 1) * 0.2End IfH = N * HETP + HAWs = 3.14 * DT * (H + 0.8116 * DT) * bo * ρ'ρ为碳钢的密度CH = FL * Exp(6.95 + 0.1808 * Log(Ws) + 0.02468 * (Log(Ws)) ^ 2 + 0.0158 * H / DT) J1 = SI * (Fc + 0.06) * CHtajing = DTzongtagao = HEnd Sub'求J2Public Sub jjj2(R#, J2#)Dim xx1#, xx0#, CD#, ff#, df#, t2#, AD#, KD#KD = 2000: xx1 = 70Do '牛顿迭代法求冷却水最佳出口温度t2xx0 = xx1CD = 1.3 * SI * a * b * f1 * f2 * Fc * ((R + 1) * D * rD / (td - t1)) ^ (b - 1) / KD ^ bff = -Cw * θ/ Cp + CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (1 - b) * (xx0 - 1 - Log(xx0))df = CD * ((xx0 - 1) / xx0 / Log(xx0)) ^ (2 - b) * ((b - 1) * (xx0 - 1 - Log(xx0)) ^ 2 / (xx0 - 1) ^ 2 + Log(xx0))xx1 = xx0 - ff / dfLoop Until Abs(xx1 - xx0) < 0.000001t2 = td - (td - t1) / xx1 't2optchukouwendu = t2AD = (R + 1) * D * rD * Log((td - t1) / (td - t2)) / KD / (t2 - t1) '传热面积chuanremianji = ADJ2 = Cw * θ* (R + 1) * D * rD / Cp / (t2 - t1) + 1.3 * SI * Fc * f1 * f2 * a * AD ^ b End Sub'求J3Public Sub jjj3(R#, J3#)Dim Z#, Cz#Cz = 0.03Z = ((R + 1) * D - (1 - q) * F) * 18J3 = Z * Cz * θEnd Sub'求J4Public Sub jjj4(R#, J4#)Dim ho#, cpa!, HETP!cpa = 15674.4: HETP = 0.462Call tabanshu(R#, N#)ho = N * HETPDT = Sqr((R + 1) * D * 22.4 / (3600 * 0.785 * u) * (273 + td) / 273 * 101.3 / po)J4 = 3.14 / 4 * DT ^ 2 * ho * cpa * FcEnd Sub'塔板数的计算Public Sub tabanshu(R#, N#)Dim ye#, XW#Dim X!(100), Y!(100), xx!(100), i%, n1#td = 64.93: F = 402.34: XD = 0.982: XF = 0.3151: ηd = 0.999: D = 128.97: Rmin = 1.0167V = (R + 1) * D: W = F + V - D: XW = (F * XF - D * XD) / Wi = 1: Y(1) = 0.982: X(1) = 0.9702DoIf X(i) > XF ThenY(i + 1) = R * X(i) / (R + 1) + XD / (R + 1)n1 = i + 1 + (X(i) - XF) / (X(i) - X(i + 1))ElseY(i + 1) = W * (X(i) - XW) / VIf X(i) < XW Then Exit DoEnd Ifi = i + 1xx(i) = (Y(i) / (3.3874 * (1 - Y(i)))) ^ (1 / 0.7977) X(i) = xx(i) / (1 + xx(i))LoopN = i - 1 + (X(i - 1) - XW) / (X(i - 1) - X(i))lilunbanshu = Ntiliuduanbanshu = n1jinliuduanbanshu = N - n1End Sub目录1 前言------------------------------------------------------------------------------------------------12 方案论证2.1 精馏塔类型----------------------------------------------------------------------------------12.2 精馏压力-------------------------------------------------------------------------------------1 2.3 进料方式-------------------------------------------------------------------------------------1 2.4 填料类型-------------------------------------------------------------------------------------2 2.5 加热方式-------------------------------------------------------------------------------------2 2.6 塔材料类型----------------------------------------------------------------------------------23 数学模型的建立3.1 精馏塔塔体年投资折旧费及维修费用-------------------------------------------------33.2 冷凝器年运转费用-------------------------------------------------------------------------43.3 直接蒸汽加热费用-------------------------------------------------------------------------53.4 填料年折旧费-------------------------------------------------- --54 数学模型的求解4.1 数学模型决策变量分析-------------------------------------------------------------------54.2 主要工艺参数的求解----------------------------------------------------------------------54.2.1 塔径的计算-----------------------------------------------------------------------------54.2.2 塔板数的计算-------------------------------------------------------------------------64.2.2.1 相平衡关系的表示--------------------------------------------------------------64.2.2.2 N的计算--------------------------------------------------------------------------64.2.3 冷凝器年运转费用的计算------------------ ----------------------- ----------------74.2.3.1 冷却水用量及冷凝器传热面积的计算- -------------------------------------74.2.3.2 冷凝器冷却水最佳出口温度的确定-----------------------------------------74.2.4 直接加热蒸气费用的计算----------------------------------------------------------84.3 数学模型的求解------------------------------------------------------- --------------------84.3.1 单变量最优化方法--------------------------------------------- ----------------------84.3.2 优化设计程序框图-------------------------------------------- -----------------------84.3.2.1 函数调用关系--------------------------------------------------------------------95 优化设计计算5.1 数据预处理---------------------------------------------------------------------------------105.1.1 进塔物料的计算----------------------------------------------------------------------105.1.2 塔顶蒸气温度的计算----------------------------------------------------------------105.1.3 等板高度的计算----------------------------------------------------------------------10Ⅰ5.1.4 产品汽化潜热的计算----------------------------------------------------------------115.1.5 最小回流比的确定-------------------------------------------------------------------115.1.6 填料单价的计算----------------------------------------------------------------------115.1.7 空塔气速的计算----------------------------------------------------------------------115.2. 塔径的计算---------------------------------------------------------------------------------135.3 填料层高度的计算-------------------------------------------------------------------------135.4 精馏塔塔体年投资折旧费及维修费用的计算-----------------------------------------135.5 冷凝器年运转费用的计算----------------------------------------------------------------145.5.1 冷凝器冷却水最佳出口温度的确定----------------------------------------------145.5.2 冷却水用量及冷凝器传热面积的计算-------------------------------------------145.5.3 精馏塔塔体年投资折旧费及维修费用的计算----------------------------------155.6 再沸器年运转费用的计算----------------------------------------------------------------155.7 填料年折旧费用的计算-------------------------------------------------------------------155.8 汽液负荷-------------------------------------------------------------------------------------155.8.1 气相负荷-------------------------------------------------------------------------------155.8.2 液相负荷-------------------------------------------------------------------------------155.9 年总费用与回流比的关系--------------------------------------------------------------156 填料塔水力学性能校核6.1 泛点率校核--------------------------------------------------------------------------------- 176.2 径比校核-------------------------------------------------------------------------------------17 6.3 喷淋密度校核-------------------------------------------------------------------------------176.4 填料塔压降----------------------------------------------------------------------------------177 附属设备的设计与选型7.1 塔顶冷凝器--------------------------------------------------------------------------------- 187.1.1 冷凝器传热量-------------------------------------------------------------------------187.1.2 冷凝器传热推动力-------------------------------------------------------------------187.1.3 初估冷凝器传热面积----------------------------------------------------------------197.1.4 冷凝器传热系数的校核-------------------------------------------------------------197.1.5 冷凝器传热面积的校核-------------------------------------------------------------227.1.6 冷凝器壳程、管程流动阻力-------------------------------------------------------227.2 接管选型------------------------------------------------------------------------------------ 247.2.1 进料口接管的选型-------------------------------------------------------------------247.2.2 冷却水接管的选型-------------------------------------------------------------------257.2.3 塔顶蒸气接管选型------------------------------------------------------------------ 25Ⅱ7.2.4 塔顶产品接管选型-------------------------------------------------------------------257.2.5 塔底产品接管选型-------------------------------------------------------------------267.2.6 塔顶产品回流接管选型-------------------------------------------------------------267.2.7 塔底加热蒸气接管选型------------------------------------------------------------- 267.3 冷却水输送泵7.3.1 塔高的计算---------------------------------------------------------------------------277.3.2 冷却水输送泵选型------------------------------------------------------------------277.4 填料支承结构-------------------------------------------------------------------------------287.5 液体分布装置-------------------------------------------------------------------------------287.6 填料压紧装置------------------------------------------------------------------------------ 297.7 液体收集再分布装置----------------------------------------------------------------------298 设计结果汇总------------------------------------------------------------------------------------299 设计心得------------------------------------------------------------------------------------------3 1参考文献---------------------------------------------------------------------------------------------- 31附录一甲醇和水部分物性参数-----------------------------------------------------------------32附录二甲醇—水汽液平衡数据（摩尔组成）-------------------------------------------------33附录三优化设计程序源代码--------------------------------------------------------------------34化工原理课程设计学生姓名：黄圣楠学号：081000115专业班级：10级生工（1）班____指导教师：张星___2013年1月24日。