武汉理工大学网络专升本线性代数与概率统计阶段作业一
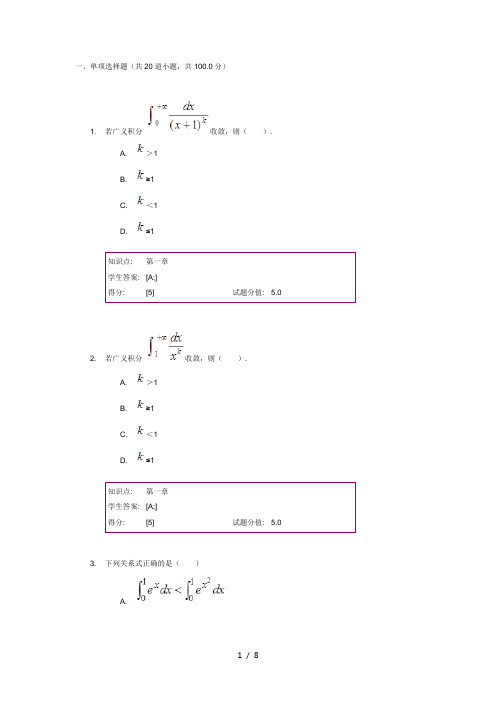
下半年华工继续教育线性代数与概率统计随堂练习参考答案

1.(单项选择题 ) 计算?A.;B.;C.;D..答题: A. B. C. D.(已提交)参照答案: A问题分析:2.(单项选择题 )队列式?A. 3;B. 4;C. 5;D. 6.答题: A. B. C. D.(已提交)参照答案: B问题分析:3. ( 单项选择题 )计算队列式. A. 12;B. 18;C. 24;D. 26.答题: A. B. C. D.(已提交)参照答案: B问题分析:4. ( 单项选择题 )利用队列式定义计算n 阶队列式:=? A.;B.;C.;D..答题: A. B. C. D.(已提交)参照答案: C问题分析:5. ( 单项选择题 )计算队列式睁开式中,的系数。
A. 1, 4;B. 1,-4;C. -1 ,4;D. -1 ,-4.答题: A. B. C. D.(已提交)参照答案: B问题分析:6. ( 单项选择题 )计算队列式=?A. -8;B. -7;C. -6;D. -5.答题: A. B. C. D.(已提交)参照答案: B问题分析:7. ( 单项选择题 )计算队列式=?A. 130 ;B. 140;C. 150;D. 160.答题: A. B. C. D.(已提交)参照答案: D问题分析:8. ( 单项选择题 )四阶队列式的值等于多少?A.;B.;C.;D..答题: A. B. C. D.(已提交)参照答案: D问题分析:9. ( 单项选择题 )队列式=?A.;B.;C.;D..答题: A. B. C. D.(已提交)参照答案: B问题分析:10. (单项选择题 )已知,则?A. 6m;B. -6m;C. 12m;D. -12m.答题: A. B. C. D.(已提交)参照答案: A11.(单项选择题)设=,则?A. 15|A|;B. 16|A|;C. 17|A|;D. 18|A|.答题: A. B. C. D.(已提交)参照答案: D问题分析:12. ( 单项选择题 )设矩阵,求=?A. -1;B. 0;C. 1;D. 2.答题: A. B. C. D.(已提交)参照答案: B问题分析:13. ( 单项选择题 )计算队列式=?A. -1500;B. 0;C. -1800;D. -1200.答题: A. B. C. D.(已提交)参照答案: C问题分析:14. ( 单项选择题 )齐次线性方程组有非零解,则=?A. -1;B. 0;C. 1;D. 2.答题: A. B. C. D.(已提交)参照答案: C问题分析:15. ( 单项选择题 )齐次线性方程组有非零解的条件是=?A.1或-3 ;B.1或3 ;C.-1或3 ;D.-1或-3 .答题: A. B. C. D.(已提交)参照答案: A问题分析:16. ( 单项选择题 )假如非线性方程组系数队列式,那么,以下正确的结论是哪个?A.无解 ;B.独一解 ;C.一个零解和一个非零解;D.无量多个解 .答题: A. B. C. D.(已提交)参照答案: B问题分析:17. ( 单项选择题 )假如齐次线性方程组的系数队列式,那么,下列正确的结论是哪个?A.只有零解 ;B.只有非零解 ;C.既有零解,也有非零解;D.有无量多个解.答题: A. B. C. D.(已提交)参照答案: A问题分析:18. ( 单项选择题 )齐次线性方程组总有___解;当它所含方程的个数小于未知量的个数时,它必定有___ 解。
线性代数与概率论统计(专升本)试卷1

《线性代数与概率论统计》姓名 年级 层次 专业 准考证号一、填空题(每小题4分,共20分。
)1.若,A B 都是3阶方阵,且A =2,B = 3E ,则T A B = .2. 设3阶方阵11124133A -⎛⎫ ⎪=- ⎪ ⎪--⎝⎭x 相似于矩阵1101220003-⎛⎫⎪= ⎪ ⎪⎝⎭B .则常数=x .3.设A ,B 为互不相容的两个事件,()0.2P A =,()0.3P B =,则()P A B = .4. 甲、乙两人独立的对同一目标射击一次,其命中率分别为0.6和0.5,则目标被命中的概率为 .5.设~()X P λ,则()D X = . 二、选择题(每小题4分,共20分。
)1. 设, A B 为n 阶方阵,E 为n 阶单位矩阵,则下列等式成立的是( ).(A) ()()22A B A B A B -+=-; (B) ()()2A E A E A E -+=-; (C) AB BA =; (D) ()A B E A B E +=++. 2. 若方阵A 满足230A E -=,则A 必有一个特征值为( ). (A) 2; (B) 3; (C) 3/2; (D) 2/3.3. 设A ,B 为两个随机事件,且A B ⊂,则下列各式中正确的是( ).(A) ()()P A B P A =; (B) ()()P AB P B =; (C) (|)()P B A P B =; (D) ()()()P B A P B P A -=-. 4. 设随机变量Y X ,独立,且)1,1(~),1,0(~N Y N X ,则( ).(A) 21}0{=≤+Y X P ; (B) 21}1{=≤+Y X P ;(C) 21}0{=≤-Y X P ; (D) 21}1{=≤-Y X P ;5. 设12,,,n X X X 是来自正态总体X2(,)N μσ的一个样本,则下列各式中正确的是( ).(A) 2~X μσ⎛⎫- ⎪⎝⎭2(1)χ; (B) 2~X n ⎛⎫- ⎪⎝⎭μσ2(1)χ;(C) 2~X μσ⎛⎫- ⎪⎝⎭(1)t ; (D) 2~X n ⎛⎫- ⎪⎝⎭μσ(1)t .三、线代计算题(每小题10分,共30分。
课程:线性代数(专升本)试题和答案

课程:线性代数(专升本)--习题和答案1.(单选题) 对于元齐次线性方程组,以下命题中,正确的是( )(本题3.5分)A、若的列向量组线性无关,则有非零解;B、若的行向量组线性无关,则有非零解;C、若的列向量组线性相关,则有非零解;D、若的行向量组线性相关,则有非零解。
学生答案:未答题标准答案:C解析:得分:2.(单选题) 设A是方阵,如有矩阵关系式AB=AC,则必有( )(本题3.5分)A、 A =0B、B C时A=0C、A0时B=CD、|A|0时B=C学生答案:未答题标准答案:D解析:得分:3.(单选题) 设λ0是矩阵A的特征方程的3重根,A的属于λ0的线性无关的特征向量的个数为k,则必有( )(本题3.5分)B、k<3C、k=3D、k>3学生答案:未答题标准答案:A解析:得分:4.(单选题) 已知为四维列向量组,且行列式,,则行列式( )(本题3.5分)A、;B、 B.;C、;D、。
学生答案:未答题标准答案:D解析:得分:5.(单选题) 设A=(a ij)3×3,|A|=2,A ij表示|A|中元素a ij的代数余子式(i,j=1,2,3),则(a11A21+a12A22+a13A23)2+(a21A21+a22A22+a23A23)2+(a31A21+a32A22+a33A23)2=( ).(本题3.0分)B、 2C、 3D、 4学生答案:未答题标准答案:D解析:得分:6.(单选题) 设两个向量组α1,α2,…,αs和β1,β2,…,βs均线性相关,则( )(本题3.5分)A、有不全为0的数λ1,λ2,…,λs使λ1α1+λ2α2+…+λsαs=0和λ1β1+λ2β2+…λsβs=0B、有不全为0的数λ1,λ2,…,λs使λ1(α1+β1)+λ2(α2+β2)+…+λs(αs+βs)=0C、有不全为0的数λ1,λ2,…,λs使λ1(α1-β1)+λ2(α2-β2)+…+λs(αs-βs)=0D、有不全为0的数λ1,λ2,…,λs和不全为0的数μ1,μ2,…,μs使λ1α1+λ2α2+…+λsαs=0和μ1β1+μ2β2+…+μsβs=0学生答案:未答题标准答案:D解析:得分:7.(单选题) 设A是一个n(≥3)阶方阵,下列陈述中正确的是( )(本题3.5分)A、如存在数λ和向量α使Aα=λα,则α是A的属于特征值λ的特征向量B、如存在数λ和非零向量α,使(λE-A)α=0,则λ是A的特征值C、A的2个不同的特征值可以有同一个特征向量D、如λ1,λ2,λ3是A的3个互不相同的特征值,α1,α2,α3依次是A的属于λ1,λ2,λ3的特征向量,则α1,α2,α3有可能线性相关学生答案:未答题标准答案:B解析:得分:8.(单选题)( ).(本题3.0分)A、 3B、 5C、 6D、8学生答案:未答题标准答案:C解析:得分:9.(单选题) 设矩阵A=,已知α=是它的一个特征向量,则α所对应的特征值为( ).(本题3.0分)A、 1B、 2D、 4学生答案:未答题标准答案:A解析:得分:10.(单选题) 已知,则以下选项中正确的是( )(本题3.5分)A、;B、;C、;D、。
(武汉函授)线性代数与概率统计作业

武汉理工大学成人教育《线性代数与概率统计》作业试题备注:所有答案写在后面答题纸上,期末考试前答题纸回交学习中心(后面附答题纸)第一部分选择题单项选择题(本大题共14小题)在每小题列出的四个选项中只有一个是符合题目要求的,请将其代码填在题后的括号内。
1.设行列式a aa a11122122=m,a aa a13112321=n,则行列式a a aa a a111213212223++等于()A. m+nB. -(m+n)C. n-mD. m-n2.设矩阵A=100020003⎛⎝⎫⎭⎪⎪⎪,则A-1等于()A.130012001⎛⎝⎫⎭⎪⎪⎪⎪⎪⎪B.100120013⎛⎝⎫⎭⎪⎪⎪⎪⎪⎪C.13000100012⎛⎝⎫⎭⎪⎪⎪⎪⎪D.120013001⎛⎝⎫⎭⎪⎪⎪⎪⎪⎪3.设矩阵A=312101214---⎛⎝⎫⎭⎪⎪⎪,A*是A的伴随矩阵,则A *中位于(1,2)的元素是()A. –6B. 6C. 2D. –24.设A是方阵,如有矩阵关系式AB=AC,则必有()A. A =0B. B≠C时A=0C. A≠0时B=CD. |A|≠0时B=C5.已知3×4矩阵A的行向量组线性无关,则秩(A T)等于()A. 1B. 2C. 3D. 46.设两个向量组α1,α2,…,αs和β1,β2,…,βs均线性相关,则()A.有不全为0的数λ1,λ2,…,λs使λ1α1+λ2α2+…+λsαs=0和λ1β1+λ2β2+…λsβs=0B.有不全为0的数λ1,λ2,…,λs使λ1(α1+β1)+λ2(α2+β2)+…+λs(αs+βs)=0C.有不全为0的数λ1,λ2,…,λs使λ1(α1-β1)+λ2(α2-β2)+…+λs(αs-βs)=0D.有不全为0的数λ1,λ2,…,λs和不全为0的数μ1,μ2,…,μs使λ1α1+λ2α2+…+λsαs=0和μ1β1+μ2β2+…+μsβs=07.设矩阵A的秩为r,则A中()A.所有r-1阶子式都不为0B.所有r-1阶子式全为0C.至少有一个r阶子式不等于0D.所有r阶子式都不为08.设Ax=b是一非齐次线性方程组,η1,η2是其任意2个解,则下列结论错误的是()A.η1+η2是Ax=0的一个解B.12η1+12η2是Ax=b的一个解C.η1-η2是Ax=0的一个解D.2η1-η2是Ax=b的一个解9.设n阶方阵A不可逆,则必有()A.秩(A)<nB.秩(A)=n-1C.A=0D.方程组Ax=0只有零解10.设A是一个n(≥3)阶方阵,下列陈述中正确的是()A.如存在数λ和向量α使Aα=λα,则α是A的属于特征值λ的特征向量B.如存在数λ和非零向量α,使(λE-A)α=0,则λ是A的特征值C.A的2个不同的特征值可以有同一个特征向量D.如λ1,λ2,λ3是A的3个互不相同的特征值,α1,α2,α3依次是A的属于λ1,λ2,λ3的特征向量,则α1,α2,α3有可能线性相关11.设λ0是矩阵A的特征方程的3重根,A的属于λ0的线性无关的特征向量的个数为k,则必有()A. k≤3B. k<3C. k=3D. k>312.设A是正交矩阵,则下列结论错误的是()A.|A|2必为1B.|A|必为1C.A-1=A TD.A的行(列)向量组是正交单位向量组13.设A是实对称矩阵,C是实可逆矩阵,B=C T AC.则()A.A与B相似B. A与B不等价C. A与B有相同的特征值D. A与B合同14.下列矩阵中是正定矩阵的为()A.2334⎛⎝⎫⎭⎪ B.3426⎛⎝⎫⎭⎪C.100023035--⎛⎝⎫⎭⎪⎪⎪D.111120102⎛⎝⎫⎭⎪⎪⎪第二部分非选择题二、填空题,不写解答过程,将正确的答案写在每小题的空格内。
武汉理工大学高起本高等数学(下)阶段作业一标准答案
1-10英语写法The English language is a rich and diverse means of communication that has evolved over centuries. From the simple counting of one to ten, the English language offers a wide range of numerical expressions that convey meaning and nuance. Understanding the written form of these numbers is an essential building block for any student of the language.The number one is expressed as "one" in English. This simple monosyllabic word is derived from the Old English "an" and the Proto-Germanic "*ainaz," ultimately tracing back to the Proto-Indo-European root "*oinos." The word "one" conveys the concept of a single, individual unit, and is a fundamental building block of numerical systems.Moving to the number two, the English word is "two." This too is a monosyllabic word, derived from the Old English "twā" and the Proto-Germanic "*twai." The Proto-Indo-European root is "*dwo," reflecting the binary nature of this number. "Two" represents the idea of a pair, of duality, and is a crucial component of counting andquantifying.The number three is expressed as "three" in English. This word derives from the Old English "thrēo" and the Proto-Germanic "*þrīz," with the Proto-Indo-European root being "*tréyes." The word "three" conveys the concept of a triad, a trinity, or a set of three elements. It is a pivotal number in many cultural and religious traditions.The number four is written as "four" in English. This word originates from the Old English "fēower" and the Proto-Germanic "*fedwōr," with the Proto-Indo-European root being "*kwetwóres." The word "four" represents the idea of a quadrant, a set of four elements, and is a fundamental building block of many mathematical and spatial concepts.Moving to the number five, the English word is "five." This word derives from the Old English "fīf" and the Proto-Germanic "*fimf," with the Proto-Indo-European root being "*pénkwe." The word "five" conveys the concept of a quintuple, a set of five elements, and is a significant number in many cultural and numerical systems.The number six is expressed as "six" in English. This word originates from the Old English "siex" and the Proto-Germanic "*sehss," with the Proto-Indo-European root being "*swéḱs." The word "six" represents the idea of a hexad, a set of six elements, and is animportant number in various mathematical and geometric contexts.The number seven is written as "seven" in English. This word derives from the Old English "seofon" and the Proto-Germanic "*sebun," with the Proto-Indo-European root being "*septḿ." The word "seven" conveys the concept of a heptad, a set of seven elements, and is a significant number in numerous cultural, religious, and mythological traditions.The number eight is expressed as "eight" in English. This word originates from the Old English "eahta" and the Proto-Germanic "*ahtau," with the Proto-Indo-European root being "*oḱtṓ." The word "eight" represents the idea of an octad, a set of eight elements, and is an important number in various mathematical and scientific contexts.The number nine is written as "nine" in English. This word derives from the Old English "nīgan" and the Proto-Germanic "*newn," with the Proto-Indo-European root being "*newṃ." The word "nine" conveys the concept of a ennead, a set of nine elements, and is a significant number in numerous cultural and numerical systems.Finally, the number ten is expressed as "ten" in English. This word originates from the Old English "tēn" and the Proto-Germanic"*tehun," with the Proto-Indo-European root being "*déḱm̥." Theword "ten" represents the idea of a decad, a set of ten elements, and is a fundamental building block of the decimal numerical system that is widely used throughout the world.Understanding the written forms of these numbers from one to ten is crucial for any student of the English language. These basic numerical expressions not only serve as the foundation for counting and quantifying, but also hold deeper cultural and symbolic significance in various contexts. Mastering the written forms of these numbers is an essential step in developing proficiency in the English language.。
武汉理工大学whut线性代数考试试题及其参考答案

标准答案及评分标准用纸课程名称:线性代数 ( A 卷)一、填空题(每小题3分,共15分)1、23-; 2、E; 3、-15; 4、5t ≠; 5、 2 二、选择题(每小题3分,共15分)1、C2、A3、B4、C 5 、D 三、解答题(每小题8分,共32分)1、 121000121000(1)2121000121121n n n x xn x n x n n D x x n n x x n n n n-+-++⎡⎤==+⎢⎥⎣⎦+-+--L L L L MMLM M M M L MM L L LL………………(4分)(1)12(1)(1)2n n n n n x x --+⎡⎤=-+⎢⎥⎣⎦………………………………………………………………(8分) 2、 由题意(1,2)B AE = ……………………………………………………………………………………(4分)又BX A =,即(1,2)AE X A =,所以1(1,2)X E -=(1,2)E =……………………………………………(8分)3、 记1200A A A ⎛⎫=⎪⎝⎭,则1111200A A A ---⎛⎫= ⎪⎝⎭, ……………………………………………………………(2分) 又*11211,10A A ⎛⎫==⎪-⎝⎭,故112110A -⎛⎫= ⎪-⎝⎭…………………………………………………………(4分)*21211,31A A -⎛⎫=-= ⎪-⎝⎭,故122131A --⎛⎫= ⎪-⎝⎭………………………………………………………(6分)所以121010*******031A -⎛⎫ ⎪-⎪= ⎪- ⎪-⎝⎭。
…………………………………………………………………(8分)4、记()1234,,,A αααα=,对A 进行行初等变换,将其化为行最简形:1211241012213631A -⎛⎫ ⎪- ⎪= ⎪--- ⎪-⎝⎭~1211003200320064-⎛⎫ ⎪- ⎪ ⎪- ⎪-⎝⎭~121100320000000-⎛⎫⎪- ⎪ ⎪⎪⎝⎭~112032001300000000⎛⎫-⎪⎪⎪-⎪ ⎪⎪ ⎪⎝⎭…………………(4分)()2R A =,又显然13,αα线性无关,所以13,αα即为原向量组的一个最大无关组;………………………(6分)且212αα=,4131233ααα=--。
武汉理工大学线性代数考试试题

⎝⎭武汉理工大学教务处试题标准答案及评分标准用纸 课程名称:线性代数 ( A 卷) 一、选择题(每小题3分,共12分) 1.B 2.C 3.B 4.D二、填空题(每小题3分,共12分)1.2;2.113021002⎛⎫ ⎪- ⎪ ⎪⎝⎭; 3.a=1;4. 2,2,5;(注:本小题每个数字为一分,错一个则减一分)三、解答题(每小题8分,共40分)1. 解:从第二列起,将其后各列加到第一列,有:1(1)1110111011011101(1)1011101111111111c n n n n D n n n ÷---==---121(1)(2)(1)12200010010(1)01001111(1)(1)(1)(1)(1)n n n nr r r r r r n n n n n n n n -----+----=--=-⋅--=--4分注:若采用其他方法计算出正确结果也应给满分,其正确的步骤也相应给分。
2. 由题,有E A B E A +=-)(2 2分且2202030360,402A E --==≠--故2()A E -可逆。
2分在等式左右两边左乘21()A E --得21()()B A E A E -=-+ 2分 11001001/2()010*********A E ---⎛⎫⎛⎫⎪ ⎪=-== ⎪ ⎪⎪ ⎪-⎝⎭⎝⎭3.解:2分2分2分2分11111131132231213331 3--------=-=-=-⎛⎫=- ⎪⎝⎭*()A A A A A A A A A 2分 1133-=∴= ,A A ,上式=311339⎛⎫-⨯=- ⎪⎝⎭2分注:若前面所有步骤正确,最后计算出现符号错误,扣一分。
4.解:令矩阵123413011031(,,,)271241420A αααα⎛⎫⎪-- ⎪== ⎪⎪⎝⎭,并通过初等行变化化成最简形,有:1301103010310110271200014142A r -⎛⎫⎛⎫⎪ ⎪--⎪ ⎪= ⎪ ⎪⎪ ⎪⎝⎭⎝⎭4分 故向量组A 的的一个最大无关组为124,,ααα, 2分 且3123ααα=-+。
网络提交《线性代数与概率统计》模拟题一(2013.11,90分钟)

华南理工大学网络教育学院《线性代数与概率统计》模拟试题一一.单项选择题(每小题5分,共8小题,总计40分)1.计算11221212x x x x ++=++?( A )A .12x x -B .12x x +C .21x x -D .212x x -2.用行列式的定义计算行列式143122317905311x x x x ---中展开式4x ,3x 的系数。
BA .1, 4B .1,-4C .-1,4D .-1,-43.设,A B 为n 阶对称矩阵,则下面结论中不正确的是( C )A .AB +为对称矩阵B .对任意的,n n P ⨯ TP AP 为对称矩阵 C .AB 为对称矩阵D .若,A B 可换,则AB 为对称矩阵4.111111111111kkAkk⎛⎫⎪⎪=⎪⎪⎝⎭,且()3r A=,则k=?(B )A.1B.-3C.1或-3D.-15.写出下列随机试验的样本空间及下列事件的集合表示:掷一颗骰子,出现奇数点。
D A.样本空间为{1,2,3,4,5,6}Ω=,事件“出现奇数点”为{2,4,6}B.样本空间为{1,3,5}Ω=,事件“出现奇数点”为{1,3,5}C.样本空间为{2,4,6}Ω=,事件“出现奇数点”为{1,3,5}D.样本空间为{1,2,3,4,5,6}Ω=,事件“出现奇数点”为{1,3,5}6.设A,B为随机事件,()0.2P A=,()0.45P B=,()0.15P AB=,(|)P A B=?BA.1 6B.1 3C.1 2D.2 37.已知随机变量X在[0,1]服从均匀分布,试求[]XE e为()A.eB.1e-C.1 eD.21e+8.抛掷一枚匀称的骰子,出现的点数为随机变量X ,求“出现的点数不超过3”的概率为( C )A .16B .13C .12D .34二.计算题(每小题8分,共6小题,总计48分)1.已知行列式2512371446125927-----,写出元素43a 的代数余子式43A ,并求43A 的值.解:()()()54281*26573224435264722644732521433443=--=⎪⎪⎭⎫⎝⎛--+-----=----=-=+M A2.设1100010000100021A ⎡⎤⎢⎥⎢⎥=⎢⎥⎢⎥-⎣⎦,求2A .解:⎪⎪⎪⎪⎪⎭⎫⎝⎛=10000100001000212A3..解齐次线性方程组123412341234123424023450413140750x x x x x x x x x x x x x x x x --++=⎧⎪+--=⎪⎨--+=⎪⎪--+=⎩.解:对系数矩阵施以初等变换:A=⎢⎢⎢⎢⎣⎡-→⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡----→⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡----→⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡--------→⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡--------0000100100000000321025010000000032104121963018126032104121571114134154324121与原方程组同解的方程组为:⎩⎨⎧=-+=+-03025432431x x x x x x 所以:方程组的一般解为⎩⎨⎧--=+=4324313225x x x x x x (其中,4,3x x 为自由未知量)4.袋中有10个球,分别编有号码1到10,从中任取一球,设A={取得球的号码是偶数},B={取得球的号码是奇数},C={取得球的号码小于5},问下列运算表示什么事件:(1)A+B ;(2)AB ;(3)AC ;(4)AC ;(5)B C +;(6)A-C. (1)A+B={取得球的号码是整数}(2)AB={取得球的号码既是奇数又是偶数} (3) AC={取得球的号码是2.4}(4)AC ={取得球的号码是1.3.5.6.7.8.9.10} (5)C B +={取得球的号码是6.8} (6)A-C={取得球的号码是6.8.10}5.一批产品有10件,其中4件为次品,现从中任取3件,求取出的3件产品中有次品的概率。
