实验四.哈夫曼编码的贪心算法设计
贪心算法构造哈夫曼树

软件02 1311611006 张松彬利用贪心算法构造哈夫曼树及输出对应的哈夫曼编码问题简述:两路合并最佳模式的贪心算法主要思想如下:(1)设w={w0,w1,......wn-1}是一组权值,以每个权值作为根结点值,构造n棵只有根的二叉树(2)选择两根结点权值最小的树,作为左右子树构造一棵新二叉树,新树根的权值是两棵子树根权值之和(3)重复(2),直到合并成一颗二叉树为一、实验目的(1)了解贪心算法和哈夫曼树的定义(2)掌握贪心法的设计思想并能熟练运用(3)设计贪心算法求解哈夫曼树(4)设计测试数据,写出程序文档二、实验内容(1)设计二叉树结点数据结构,编程实现对用户输入的一组权值构造哈夫曼树(2)设计函数,先序遍历输出哈夫曼树各结点3)设计函数,按树形输出哈夫曼树代码:#include <stdio.h>#include <string.h>#include <time.h>#include <stdlib.h>typedef struct Node{ //定义树结构int data;struct Node *leftchild;struct Node *rightchild;}Tree;typedef struct Data{ //定义字符及其对应的频率的结构int data;//字符对应的频率是随机产生的char c;};void Initiate(Tree **root);//初始化节点函数int getMin(struct Data a[],int n);//得到a中数值(频率)最小的数void toLength(char s[],int k);//设置有k个空格的串svoid set(struct Data a[],struct Data b[]);//初始化a,且将a备份至bchar getC(int x,struct Data a[]);//得到a中频率为x对应的字符void prin(struct Data a[]);//输出初始化后的字符及对应的频率int n;void main(){//srand((unsigned)time(NULL));Tree *root=NULL,*left=NULL,*right=NULL,*p=NULL; int min,num;int k=30,j,m;struct Data a[100];struct Data b[100];int i;char s[100]={'\0'},s1[100]={'\0'};char c;set(a,b);prin(a);Initiate(&root);Initiate(&left);Initiate(&right);Initiate(&p);//设置最底层的左节点min=getMin(a,n);left->data=min;left->leftchild=NULL;left->rightchild=NULL;//设置最底层的右节点min=getMin(a,n-1);right->data=min;right->leftchild=NULL;right->rightchild=NULL;root->data=left->data+right->data;Initiate(&root->leftchild);Initiate(&root->rightchild);//将设置好的左右节点插入到root中root->leftchild=left;root->rightchild=right;for(i=0;i<n-2;i++){min=getMin(a,n-2-i);Initiate(&left);Initiate(&right);if(min<root->data)//权值小的作为左节点{left->data=min;left->leftchild=NULL;left->rightchild=NULL;p->data=min+root->data;Initiate(&p->leftchild);Initiate(&p->rightchild);p->leftchild=left;p->rightchild=root;root=p;}else{right->data=min;right->leftchild=NULL;right->rightchild=NULL;p->data=min+root->data;Initiate(&p->leftchild);Initiate(&p->rightchild);p->leftchild=root;p->rightchild=right;root=p;}Initiate(&p);}num=n-1;p=root;printf("哈夫曼树如下图:\n");while(num){if(num==n-1){for(j=0;j<k-3;j++)printf(" ");printf("%d\n",root->data);}for(j=0;j<k-4;j++)printf(" ");printf("/ \\\n");for(j=0;j<k-5;j++)printf(" ");printf("%d",root->leftchild->data);printf(" %d\n",root->rightchild->data);if(root->leftchild->leftchild!=NULL){root=root->leftchild;k=k-2;}else{root=root->rightchild;k=k+3;}num--;}num=n-1;Initiate(&root);root=p;printf("各字符对应的编码如下:\n");while(num){if(root->leftchild->leftchild==NULL){strcpy(s1,s);m=root->leftchild->data;c=getC(m,b);printf("%c 【%d】:%s\n",c,m,strcat(s1,"0"));}if(root->rightchild->leftchild==NULL){strcpy(s1,s);m=root->rightchild->data;c=getC(m,b);printf("%c 【%d】:%s\n",c,m,strcat(s1,"1"));}if(root->leftchild->leftchild!=NULL){strcat(s,"0");root=root->leftchild;}if(root->rightchild->leftchild!=NULL){strcat(s,"1");root=root->rightchild;}num--;}}int getMin(struct Data a[],int n){int i,t;for(i=1;i<n;i++){if(a[i].data<a[0].data){t=a[i].data;a[i].data=a[0].data;a[0].data=t;}}t=a[0].data;for(i=0;i<n-1;i++){a[i]=a[i+1];}return t;}void toLength(char s[],int k){int i=0;for(;i<k;i++)strcat(s," ");}void Initiate(Tree **root){*root=(Tree *)malloc(sizeof(Tree));(*root)->leftchild=NULL;(*root)->rightchild=NULL;}void set(struct Data a[],struct Data b[]) {int i;srand((unsigned)time(NULL));n=rand()%10+2;for(i=0;i<n;i++){a[i].data=rand()%100+1;a[i].c=i+97;b[i].data=a[i].data;b[i].c=a[i].c;if(i>=0&&a[i].data==a[i-1].data)i--;}}char getC(int x,struct Data b[]){int i;for(i=0;i<n;i++){if(b[i].data==x){break;}}return b[i].c;}void prin(struct Data a[]){int i;printf("字符\t出现的频率\n");for(i=0;i<n;i++){printf("%c\t %d\n",a[i].c,a[i].data);}}。
实验三.哈夫曼编码的贪心算法设计

实验四 哈夫曼编码的贪心算法设计(4学时)[实验目的]1. 根据算法设计需要,掌握哈夫曼编码的二叉树结构表示方法;2. 编程实现哈夫曼编译码器;3. 掌握贪心算法的一般设计方法。
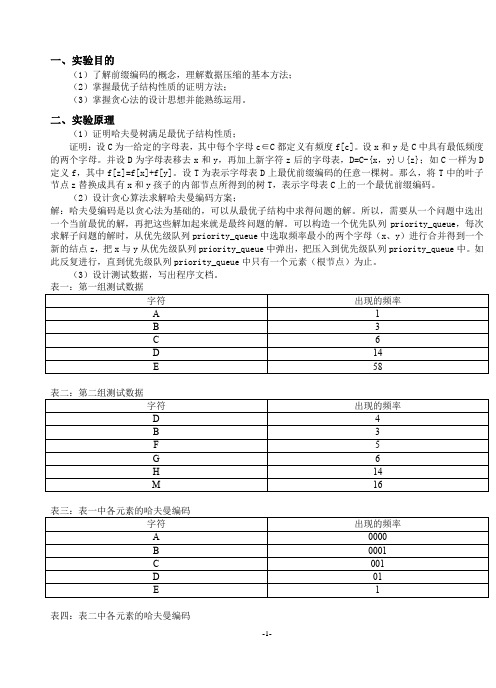
实验目的和要求(1)了解前缀编码的概念,理解数据压缩的基本方法;(2)掌握最优子结构性质的证明方法;(3)掌握贪心法的设计思想并能熟练运用(4)证明哈夫曼树满足最优子结构性质;(5)设计贪心算法求解哈夫曼编码方案;(6)设计测试数据,写出程序文档。
实验内容设需要编码的字符集为{d 1, d 2, …, dn },它们出现的频率为 {w 1, w 2, …, wn },应用哈夫曼树构造最短的不等长编码方案。
核心源代码#include <stdio.h>#include <stdlib.h>#include <string.h>typedef struct{unsigned int weight; //用来存放各个结点的权值unsigned int parent,LChild,RChild; //指向双亲、孩子结点的指针} HTNode, *HuffmanTree; //动态分配数组,存储哈夫曼树typedef char *HuffmanCode; //动态分配数组,存储哈夫曼编码∑=ji k k a//选择两个parent为0,且weight最小的结点s1和s2 void Select(HuffmanTree *ht,int n,int *s1,int *s2){int i,min;for(i=1; i<=n; i++){if((*ht)[i].parent==0){min=i;break;}}for(i=1; i<=n; i++){if((*ht)[i].parent==0){if((*ht)[i].weight<(*ht)[min].weight)min=i;}}*s1=min;for(i=1; i<=n; i++){if((*ht)[i].parent==0 && i!=(*s1)){min=i;break;}}for(i=1; i<=n; i++){if((*ht)[i].parent==0 && i!=(*s1)){if((*ht)[i].weight<(*ht)[min].weight)min=i;}}*s2=min;}//构造哈夫曼树ht,w存放已知的n个权值void CrtHuffmanTree(HuffmanTree *ht,int *w,int n) {int m,i,s1,s2;m=2*n-1; //总共的结点数*ht=(HuffmanTree)malloc((m+1)*sizeof(HTNode));for(i=1; i<=n; i++) //1--n号存放叶子结点,初始化{(*ht)[i].weight=w[i];(*ht)[i].LChild=0;(*ht)[i].parent=0;(*ht)[i].RChild=0;}for(i=n+1; i<=m; i++) //非叶子结点的初始化{(*ht)[i].weight=0;(*ht)[i].LChild=0;(*ht)[i].parent=0;(*ht)[i].RChild=0;}printf("\n哈夫曼树为: \n");for(i=n+1; i<=m; i++) //创建非叶子结点,建哈夫曼树{ //在(*ht)[1]~(*ht)[i-1]的范围内选择两个parent为0且weight最小的结点,其序号分别赋值给s1、s2 Select(ht,i-1,&s1,&s2);(*ht)[s1].parent=i;(*ht)[s2].parent=i;(*ht)[i].LChild=s1;(*ht)[i].RChild=s2;(*ht)[i].weight=(*ht)[s1].weight+(*ht)[s2].weight;printf("%d (%d, %d)\n",(*ht)[i].weight,(*ht)[s1].weight,(*ht)[s2].weight);}printf("\n");}//从叶子结点到根,逆向求每个叶子结点对应的哈夫曼编码void CrtHuffmanCode(HuffmanTree *ht, HuffmanCode *hc, int n){char *cd; //定义的存放编码的空间int a[100];int i,start,p,w=0;unsigned int c;hc=(HuffmanCode *)malloc((n+1)*sizeof(char *)); //分配n个编码的头指针cd=(char *)malloc(n*sizeof(char)); //分配求当前编码的工作空间cd[n-1]='\0'; //从右向左逐位存放编码,首先存放编码结束符for(i=1; i<=n; i++) //求n个叶子结点对应的哈夫曼编码{a[i]=0;start=n-1; //起始指针位置在最右边for(c=i,p=(*ht)[i].parent; p!=0; c=p,p=(*ht)[p].parent) //从叶子到根结点求编码{if( (*ht)[p].LChild==c){cd[--start]='1'; //左分支标1a[i]++;}else{cd[--start]='0'; //右分支标0a[i]++;}}hc[i]=(char *)malloc((n-start)*sizeof(char)); //为第i个编码分配空间strcpy(hc[i],&cd[start]); //将cd复制编码到hc}free(cd);for(i=1; i<=n; i++)printf(" 权值为%d的哈夫曼编码为:%s\n",(*ht)[i].weight,hc[i]);for(i=1; i<=n; i++)w+=(*ht)[i].weight*a[i];printf(" 带权路径为:%d\n",w);}void main(){HuffmanTree HT;HuffmanCode HC;int *w,i,n,wei;printf("**哈夫曼编码**\n" );printf("请输入结点个数:" );scanf("%d",&n);w=(int *)malloc((n+1)*sizeof(int)); printf("\n输入这%d个元素的权值:\n",n); for(i=1; i<=n; i++){printf("%d: ",i);fflush(stdin);scanf("%d",&wei);w[i]=wei;}CrtHuffmanTree(&HT,w,n); CrtHuffmanCode(&HT,&HC,n);}实验结果实验体会哈夫曼编码算法:每次将集合中两个权值最小的二叉树合并成一棵新二叉树,n-1次合并后,成为最终的一棵哈夫曼树。
贪心算法流程图

贪心算法流程图贪心算法是一种在每一步选择中都采取当前状态下最优决策的算法,以期望能够获得全局最优解。
在实际应用中,贪心算法通常用来解决最优化问题,比如最小生成树、哈夫曼编码等。
贪心算法的流程图可以帮助我们更直观地理解其工作原理和实现过程。
首先,我们来看一下贪心算法的流程图。
在图中,首先我们需要确定问题的解空间,然后根据问题的特点选择合适的贪心策略。
接着,我们需要确定每一步的最优选择,并且不断更新当前状态,直到达到最优解或者无法继续优化为止。
在实际应用中,贪心算法的流程图可以根据具体问题的特点进行调整和优化。
下面我们以一个简单的例子来说明贪心算法的流程图。
假设现在有一组活动,每个活动都有一个开始时间和结束时间,我们希望安排尽可能多的活动,使得它们之间不会相互冲突。
这个问题可以用贪心算法来解决。
首先,我们需要对活动按照结束时间进行排序,然后从第一个活动开始,依次检查每个活动的开始时间是否晚于上一个活动的结束时间。
如果是,则将该活动加入最优解集合中,并更新当前状态。
如果不是,则将该活动舍弃。
通过这样的贪心策略,我们可以得到安排最多活动的最优解。
整个流程可以用一个简单的流程图来表示,从而更直观地理解贪心算法的工作原理。
贪心算法的流程图不仅可以帮助我们理解算法的实现过程,还可以指导我们在实际应用中进行调整和优化。
通过对问题解空间的划分和贪心策略的选择,我们可以更快地找到最优解,提高算法的效率和性能。
总之,贪心算法的流程图是我们理解和应用贪心算法的重要工具,它可以帮助我们更直观地理解算法的工作原理,指导我们进行问题求解和算法优化。
希望通过本文的介绍,读者能对贪心算法有一个更深入的理解,并在实际应用中取得更好的效果。
哈弗曼树编码实验报告
一、实验目的(1)了解前缀编码的概念,理解数据压缩的基本方法;(2)掌握最优子结构性质的证明方法;(3)掌握贪心法的设计思想并能熟练运用。
二、实验原理(1)证明哈夫曼树满足最优子结构性质;证明:设C为一给定的字母表,其中每个字母c∈C都定义有频度f[c]。
设x和y是C中具有最低频度的两个字母。
并设D为字母表移去x和y,再加上新字符z后的字母表,D=C-{x,y}∪{z};如C一样为D 定义f,其中f[z]=f[x]+f[y]。
设T为表示字母表D上最优前缀编码的任意一棵树。
那么,将T中的叶子节点z替换成具有x和y孩子的内部节点所得到的树T,表示字母表C上的一个最优前缀编码。
(2)设计贪心算法求解哈夫曼编码方案;解:哈夫曼编码是以贪心法为基础的,可以从最优子结构中求得问题的解。
所以,需要从一个问题中选出一个当前最优的解,再把这些解加起来就是最终问题的解。
可以构造一个优先队列priority_queue,每次求解子问题的解时,从优先级队列priority_queue中选取频率最小的两个字母(x、y)进行合并得到一个新的结点z,把x与y从优先级队列priority_queue中弹出,把压入到优先级队列priority_queue中。
如此反复进行,直到优先级队列priority_queue中只有一个元素(根节点)为止。
(3)设计测试数据,写出程序文档。
表四:表二中各元素的哈夫曼编码三、实验设备1台PC及VISUAL C++6.0软件四、代码#include <iostream>#include <queue>#include <vector>#include <iomanip>#include <string>#include<cctype>using namespace std;structcodeInformation{double priority;charcodeName;intlchild,rchild,parent;bool test;bool operator < (constcodeInformation& x) const {return !(priority<x.priority);} };bool check(vector<codeInformation>qa,const char c){for (int i=0 ;i<(int)(qa.size());i++){if(qa[i].codeName==c) return true;} return false;}voidaline(char c,int n){for (int i=0;i<n;i++)cout<<c;}intInputElement(vector<codeInformation>* Harffcode,priority_queue<codeInformation>* pq) {int i=1,j=1;codeInformation wk;while(i){aline('-',80);cout<<"请输入第"<<j<<"个元素的字符名称(Ascll码):"<<flush;cin>>wk.codeName;while(check(* Harffcode,wk.codeName)){cout<<"字符已存在,请输入一个其他的字符:";cin>>wk.codeName;}cout<<"请输入第"<<j<<"个元素的概率(权值):"<<flush;cin>>wk.priority;wk.lchild=wk.rchild=wk.parent=-1;wk.test=false;Harffcode->push_back(wk);pq->push(wk);j++;cout<<"1…………继续输入下一个元素信息!"<<endl;cout<<"2…………已完成输入,并开始构造哈夫曼树!"<<endl;cin>>i;if (i==2) i=0;}int count=1;j=Harffcode->size();int selectElement(vector<codeInformation>*,priority_queue<codeInformation>*);for (int k=j;k<2*j-1;k++){aline('*',80);cout<<"第"<<count<<"次合并:"<<endl;int i1=selectElement(Harffcode,pq);int i2=selectElement(Harffcode,pq);(*Harffcode)[i1].parent=(*Harffcode)[i2].parent=k;wk.lchild=wk.rchild=wk.parent=-1;wk.codeName='#';(*Harffcode).push_back(wk);wk.priority=(*Harffcode)[k].priority=(*Harffcode)[i1].priority+(*Harffcode)[i2].priority;(*Harffcode)[k].lchild=i1;(*Harffcode)[k].rchild=i2;wk.test=false;pq->push(wk); c ount++;cout<<"所合成的节点名称:#(虚节点)\t"<<"概率(权值):"<<(*Harffcode)[k].priority<<endl;}aline('*',80);return j;}voidshowChar(const char c){if(isspace(c))cout<<"#";cout<<c;}int selectElement(vector<codeInformation>*Harffcode,priority_queue<codeInformation>*qurgh){for (int i=0;i<(int)(*Harffcode).size();i++){if (((*Harffcode)[i].priority==(*qurgh).top().priority)&&((*Harffcode)[i].test==false)){cout<<"所选择的节点的信息:"<<"频率(权值):"<<setw(5)<<(*qurgh).top().priority<<"\t 名为:";showChar((*qurgh).top().codeName);cout<<endl;(*qurgh).pop();(*Harffcode)[i].test=true;return i;}}}voidhuffmanCode(vector<codeInformation>Harffcode,int n){for (int i1=0;i1<(int)Harffcode.size();i1++){cout<<"array["<<i1<<"]的概率(权值):"<<Harffcode[i1].priority<<"\t"<<"名为:";showChar(Harffcode[i1].codeName);cout<<"\t父节点的数组下标索引值:"<<Harffcode[i1].parent<<endl;}aline('&',80);for (int i=0;i<n;i++){string s=" "; int j=i;while(Harffcode[j].parent>=0){if (Harffcode[Harffcode[j].parent].lchild==j) s=s+"0";else s=s+"1";j=Harffcode[j].parent;}cout<<"\n概率(权值)为:"<<setw(8)<<Harffcode[i].priority<<" 名为:";showChar(Harffcode[i].codeName);cout<<"的符号的编码是:";for (int i=s.length();i>0;i--)cout<<s[i-1];}}voidchoise(){cout<<endl;aline('+',80);cout<<"\n1……………………继续使用该程序"<<endl;cout<<"2……………………退出系统"<<endl;}void welcome(){cout<<"\n"<<setw(56)<<"欢迎使用哈夫曼编码简易系统\n"<<endl;}int main(){welcome();system("color d1");int i=1,n;vector<codeInformation>huffTree; priority_queue<codeInformation>qpTree;while(i!=2){n=InputElement(&huffTree,&qpTree);huffmanCode(huffTree, n);choise();cin>>i;huffTree.clear();while(qpTree.empty()) qpTree.pop();}return 0;}五、实验过程原始记录( 测试数据、图表、计算等)程序测试结果及分析:图(2)输入第一组测试数据开始输入第一组测试数据,该组数据信息如表一所示。
数据结构实验哈夫曼树编码

实验四哈夫曼树编码一、实验目的1、掌握哈夫曼树的一般算法;2、掌握用哈夫曼树对字符串进行编码;3、掌握通过哈夫曼树对字符编码进行译码得过程。
二、实验基本要求1、设计数据结构;2、设计编码算法;3、分析时间复杂度和空间复杂度三、程序实现此程序中包含六个函数:Select()、HuffmanTree()、BianMa()、BianMa2()、YiMa()、Sum(),其功能及实现过程如下:#include <iostream.h>struct element//哈夫曼树结点类型{int weight;int lchild,rchild,parent;};struct Char//字符编码表信息{char node;int weight;char code[20];};void Select(element hT[],int &i1,int &i2,int k)//在hT[]中查找最小值及次小值{int min1=9999,min2=9999;i1=i2=0;for(int i=0;i<k;i++)if(hT[i].parent==-1)if(hT[i].weight<min1){min2=min1;i2=i1;min1=hT[i].weight;i1=i;}else if(hT[i].weight<min2){min2=hT[i].weight;i2=i;}}void HuffmanTree(element huffTree[],Char zifuma[],int n) //构建哈夫曼树{int i,k,i1,i2;for(i=0;i<2*n-1;i++) //初始化{huffTree[i].parent=-1;huffTree[i].lchild=-1;huffTree[i].rchild=-1;}for(i=0;i<n;i++) //构造n棵只含有根结点的二叉树huffTree[i].weight=zifuma[i].weight;for(k=n;k<2*n-1;k++) //n-1次合并{Select(huffTree,i1,i2,k); //在huffTree中找权值最小的两个结点i1和i2huffTree[i1].parent=k; //将i1和i2合并,则i1和i2的双亲是khuffTree[i2].parent=k;huffTree[k].weight=huffTree[i1].weight+huffTree[i2].weight;huffTree[k].lchild=i1;huffTree[k].rchild=i2;}}void BianMa(element huffTree[],Char zifuma[],int n)//根据哈夫曼树编码{int i,m,k,j,l;char temp[20];if(n==1){ zifuma[0].code[0]='0';zifuma[0].code[1]=0;}else {for(i=0;i<n;i++){j=0;k=huffTree[i].parent;l=i;while(k!=-1){if(huffTree[k].lchild==l)temp[j++]='0';else temp[j++]='1';l=k;k=huffTree[k].parent;}k=j-1;for(m=0;m<j;m++)zifuma[i].code[m]=temp[k--];zifuma[i].code[m]=0;}}void BianMa2(Char zifuma[],char zifu[],char bianma[],int n)//根据编码表对字符串编码{int i,j,k,m;i=k=0;while(zifu[i]){for(j=0;j<n;j++)if(zifu[i]==zifuma[j].node){m=0;while(zifuma[j].code[m])bianma[k++]=zifuma[j].code[m++];}i++;}bianma[k]=0;}void YiMa(element huffTree[],Char zifuma[],char bianma[],char yima[],int n)//根据编号的码元译成字符串{int i,j,k;i=j=0;if(n==1)while(bianma[i++])yima[j++]=zifuma[0].node;else{while(bianma[i]){k=2*(n-1);while(!(huffTree[k].lchild==-1&&huffTree[k].rchild==-1))if(bianma[i++]=='0')k=huffTree[k].lchild;elsek=huffTree[k].rchild;yima[j++]=zifuma[k].node;}}yima[j]=0;}void Sum(char zifu[],Char bianma[],int &n)//计算字符串中字符种类的个数及其出现次数{i=j=0;while(zifu[i]){for(int k=0;k<j;k++)if(bianma[k].node==zifu[i]){bianma[k].weight++;break;}if(k==j){bianma[j].node=zifu[i];bianma[j++].weight=1;}i++;}n=j;}void main(){int n,i;char a[50],b[200],c[50];element huffTree[100];Char w[50];cout<<"请输入需要编码的字符串:\n";cin.getline(a,49);Sum(a,w,n);cout<<"该字符串中共有"<<n<<"类字符。
哈夫曼编码贪心算法

哈夫曼编码贪心算法
一、哈夫曼编码
哈夫曼编码(Huffman Coding)是一种著名的数据压缩算法,也称作霍夫曼编码,由美国信息论家杰弗里·哈夫曼在1952年提出[1]。
哈夫曼编码可以有效地将资料压缩至最小,它的原理是将资料中出现频率最高的字元编码为最短的码字,而出现频率低的字元编码为较长的码字,从而显著提高了信息的保密性和容量。
二、贪心算法
贪心算法(Greedy Algorithm)是一种计算机算法,它试图找到一种满足条件的最佳解决方案,通常每一步都是做出在当前状态下最佳的选择,而不考虑将来可能发生的结果。
哈夫曼编码贪心算法是利用贪心算法来实现哈夫曼编码的。
该算法的步骤如下:
1. 首先统计出每一个字符出现的次数,并以此建立森林。
森林
中的每一棵树都用一个节点表示,每个节点的数值为字符出现的次数。
2. 从森林中挑选出两个出现次数最少的字符,将它们作为左右
子树合成一颗新的树,新树的根节点的数值为两个孩子节点的和。
3. 将新树加入森林中,并删除左右子树对应的原节点。
4. 重复上述步骤,直到森林中只剩一颗树,这颗树就是哈夫曼树。
5. 从哈夫曼树根节点出发,逐层往下搜索,左子节点编码为“0”,右子节点编码为“1”,最终得到每个字符的哈夫曼编码。
贪心算法设计与应用

实验报告课程算法设计与分析实验实验名称贪心算法设计与应用第 1 页一、实验目的理解贪心算法的基本原理,掌握贪心算法设计的基本方法及其应用;二、实验内容(一)Huffman编码和译码问题:1.问题描述给定n个字符在文件中的出现频率,利用Huffman树进行Huffman编码和译码。
设计一个程序实现:1.输入含n(n<=10)个字符的字符集S以及S中各个字符在文件中的出现频率,建立相应的Huffman树,求出S中各个字符的Huffman编码。
2.输入一个由S中的字符组成的序列L,求L的Huffman 编码。
3. 输入一个二进制位串B,对B进行Huffman译码,输出对应的字符序列;若不能译码,则输出无解信息。
提示:对应10 个字符的Huffman树的节点个数<211。
2.测试数据Inputn=5字符集合S={a, b, c, d, e},对应的频率分别为a: 20b: 7c: 10d: 4e: 18字符序列L=ebcca二进制位串B=01100111010010OutputS中各个字符的Huffman编码:(设Huffman树中左孩子的权<=右孩子的权)a: 11b: 010c: 00d: 011e: 10L的Huffman 编码:10010000011B对应的字符序列: dcaeeb若输入的B=01111101001,则无解(二) 加油问题(Problem Set 1702):1.问题描述一个旅行家想驾驶汽车从城市A到城市B(设出发时油箱是空的)。
给定两个城市之间的距离dis、汽车油箱的容量c、每升汽油能行驶的距离d、沿途油站数n、油站i离出发点的距离d[i]以及该站每升汽油的价格p[i],i=1,2,…,n。
设d[1]=0<d[2]<…<d[n]。
要花最少的油费从城市A到城市B,在每个加油站应加多少油,最少花费为多少?2.具体要求Input输入的第一行是一个正整数k,表示测试例个数。
贪心算法实现Huffman编码

算法分析与设计实验报告第次实验附录:完整代码#include <iostream>#include <string>#include<stdio.h>#include <time.h>#include <iomanip>#include <vector>#include<algorithm>using namespace std;class Huffman{public:char elementChar;//节点元素int weight;//权重char s;//哈夫曼编码Huffman* parent;//父节点Huffman* leftChild;//左孩子Huffman* rightChild;//右孩子public:Huffman();Huffman(char a, int weight);bool operator < (const Huffman &m)const { return weight < m.weight;} };Huffman::Huffman(){this->s = ' ';this->elementChar = '*';//非叶子节点this->parent = this->leftChild = this->rightChild = NULL;}Huffman::Huffman(char a, int weight):elementChar(a),weight(weight) {this->s = ' ';this->elementChar = '*';//非叶子节点this->parent = this->leftChild = this->rightChild = NULL;}//递归输出哈夫曼值void huffmanCode(Huffman & h){if(h.leftChild == NULL && h.rightChild == NULL){//如果是叶子节点,输出器哈夫曼编码string s;Huffman temp = h;while(temp.parent != NULL){s = temp.s + s;temp = *temp.parent;}cout << h.elementChar << "的哈夫曼编码是:" << s << endl; return;}//左孩子huffmanCode(*h.leftChild);//右孩子huffmanCode(*h.rightChild);}int main(){int l,p=0;double q=0.0;clock_t start,end,over;start=clock();end=clock();over=end-start;start=clock();string huffmanStr;cout << "请输入一串字符序列:" << endl;cin >> huffmanStr;//得到字符串信息int i=0,j,n,m[100],h,k=0;char cha[100];n = huffmanStr.length();cout << "字符串总共有字符" << n << "个" << endl;for(int i = 0; i < n; i++){j = 0; h = 0;while(huffmanStr[i] != huffmanStr[j])j++;if(j == i){cha[k] = huffmanStr[i];cout << "字符" << cha[k] << "出现";}//如果j !=i 则略过此次循环elsecontinue;for(j = i; j < n; j++){if(huffmanStr[i] == huffmanStr[j])h++;}cout << h << "次" << endl;m[k] = h;k++;}//哈夫曼编码Huffman huffmanTemp;vector < Huffman > huffmanQueue;//初始化队列for(int i = 0; i < k; i++){huffmanTemp.elementChar = cha[i];huffmanTemp.weight = m[i];huffmanQueue.push_back(huffmanTemp);}//得到哈夫曼树所有节点int huffmanQueue_index = 0;sort(huffmanQueue.begin(), huffmanQueue.end());while(huffmanQueue.size() < 2 * k - 1){//合成最小两个节点的父节点huffmanTemp.weight = huffmanQueue[huffmanQueue_index].weight + huffmanQueue[huffmanQueue_index + 1].weight;huffmanQueue[huffmanQueue_index].s = '0';huffmanQueue[huffmanQueue_index + 1].s = '1';huffmanTemp.elementChar = '*';//将父节点加入队列huffmanQueue.push_back(huffmanTemp);sort(huffmanQueue.begin(), huffmanQueue.end());huffmanQueue_index += 2;}//把所有节点构造成哈夫曼树int step = 0;//步长while(step + 2 < 2 * k){for(int j = step + 1; j <= huffmanQueue.size(); j++){if(huffmanQueue[j].elementChar == '*' && huffmanQueue[j].leftChild == NULL && (huffmanQueue[j].weight == huffmanQueue[step].weight + huffmanQueue[step+1].weight)){huffmanQueue[j].leftChild = &huffmanQueue[step];huffmanQueue[j].rightChild = &huffmanQueue[step+1];huffmanQueue[step].parent = huffmanQueue[step+1].parent = &huffmanQueue[j]; break;}}step += 2;}//序列最后一个元素,即哈弗曼树最顶端的节点huffmanTemp = huffmanQueue.back();huffmanCode(huffmanTemp);for(l=0;l<1000000000;l++)p=p+l;end=clock();printf("The time is %6.3f",(double)(end-start-over)/CLK_TCK);return 0;}。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
实验四 哈夫曼编码的贪心算法设计(4学时)
[实验目的]
1. 根据算法设计需要,掌握哈夫曼编码的二叉树结构表示方法;
2. 编程实现哈夫曼编译码器;
3. 掌握贪心算法的一般设计方法。
实验目的和要求
(1)了解前缀编码的概念,理解数据压缩的基本方法;
(2)掌握最优子结构性质的证明方法;
(3)掌握贪心法的设计思想并能熟练运用
(4)证明哈夫曼树满足最优子结构性质;
(5)设计贪心算法求解哈夫曼编码方案;
(6)设计测试数据,写出程序文档。
实验内容
设需要编码的字符集为{d 1, d 2, …, dn },它们出现的频率为 {w 1, w 2, …, wn },应用哈夫曼树构造最短的不等长编码方案。
核心源代码
#include <stdio.h>
#include <stdlib.h>
#include <string.h>
typedef struct
{
unsigned int weight; //用来存放各个结点的权值
unsigned int parent,LChild,RChild; //指向双亲、孩子结点的指针
} HTNode, *HuffmanTree; //动态分配数组,存储哈夫曼树
∑=j
i k k a
typedef char *HuffmanCode; //动态分配数组,存储哈夫曼编码//选择两个parent为0,且weight最小的结点s1和s2
void Select(HuffmanTree *ht,int n,int *s1,int *s2)
{
int i,min;
for(i=1; i<=n; i++)
{
if((*ht)[i].parent==0)
{
min=i;
break;
}
}
for(i=1; i<=n; i++)
{
if((*ht)[i].parent==0)
{
if((*ht)[i].weight<(*ht)[min].weight)
min=i;
}
}
*s1=min;
for(i=1; i<=n; i++)
{
if((*ht)[i].parent==0 && i!=(*s1))
{
min=i;
break;
}
}
for(i=1; i<=n; i++)
{
if((*ht)[i].parent==0 && i!=(*s1))
{
if((*ht)[i].weight<(*ht)[min].weight)
min=i;
}
}
*s2=min;
}
//构造哈夫曼树ht,w存放已知的n个权值
void CrtHuffmanTree(HuffmanTree *ht,int *w,int n) {
int m,i,s1,s2;
m=2*n-1; //总共的结点数
*ht=(HuffmanTree)malloc((m+1)*sizeof(HTNode));
for(i=1; i<=n; i++) //1--n号存放叶子结点,初始化
{
(*ht)[i].weight=w[i];
(*ht)[i].LChild=0;
(*ht)[i].parent=0;
(*ht)[i].RChild=0;
}
for(i=n+1; i<=m; i++) //非叶子结点的初始化
{
(*ht)[i].weight=0;
(*ht)[i].LChild=0;
(*ht)[i].parent=0;
(*ht)[i].RChild=0;
}
printf("\n哈夫曼树为: \n");
for(i=n+1; i<=m; i++) //创建非叶子结点,建哈夫曼树
{ //在(*ht)[1]~(*ht)[i-1]的范围内选择两个parent为0且weight最小的结点,其序号分别赋值给s1、s2 Select(ht,i-1,&s1,&s2);
(*ht)[s1].parent=i;
(*ht)[s2].parent=i;
(*ht)[i].LChild=s1;
(*ht)[i].RChild=s2;
(*ht)[i].weight=(*ht)[s1].weight+(*ht)[s2].weight;
printf("%d (%d, %d)\n",(*ht)[i].weight,(*ht)[s1].weight,(*ht)[s2].weight); }
printf("\n");
}
//从叶子结点到根,逆向求每个叶子结点对应的哈夫曼编码
void CrtHuffmanCode(HuffmanTree *ht, HuffmanCode *hc, int n)
{
char *cd; //定义的存放编码的空间
int a[100];
int i,start,p,w=0;
unsigned int c;
hc=(HuffmanCode *)malloc((n+1)*sizeof(char *)); //分配n个编码的头指针cd=(char *)malloc(n*sizeof(char)); //分配求当前编码的工作空间
cd[n-1]='\0'; //从右向左逐位存放编码,首先存放编码结束符
for(i=1; i<=n; i++) //求n个叶子结点对应的哈夫曼编码
{
a[i]=0;
start=n-1; //起始指针位置在最右边
for(c=i,p=(*ht)[i].parent; p!=0; c=p,p=(*ht)[p].parent) //从叶子到根结点求编码{
if( (*ht)[p].LChild==c)
{
cd[--start]='1'; //左分支标1
a[i]++;
}
else
{
cd[--start]='0'; //右分支标0
a[i]++;
}
}
hc[i]=(char *)malloc((n-start)*sizeof(char)); //为第i个编码分配空间
strcpy(hc[i],&cd[start]); //将cd复制编码到hc
}
free(cd);
for(i=1; i<=n; i++)
printf(" 权值为%d的哈夫曼编码为:%s\n",(*ht)[i].weight,hc[i]);
for(i=1; i<=n; i++)
w+=(*ht)[i].weight*a[i];
printf(" 带权路径为:%d\n",w);
. }
void main()
{
HuffmanTree HT;
HuffmanCode HC;
int *w,i,n,wei;
printf("**哈夫曼编码**\n" );
printf("请输入结点个数:" );
scanf("%d",&n);
w=(int *)malloc((n+1)*sizeof(int));
printf("\n输入这%d个元素的权值:\n",n);
for(i=1; i<=n; i++)
{
printf("%d: ",i);
fflush(stdin);
scanf("%d",&wei);
w[i]=wei;
}
CrtHuffmanTree(&HT,w,n);
CrtHuffmanCode(&HT,&HC,n);
}
实验结果
.
实验体会
哈夫曼编码算法:每次将集合中两个权值最小的二叉树合并成一棵新二叉树,n-1次合并后,成为最终的一棵哈夫曼树。
这既是贪心法的思想:从某一个最初状态出发,根据当前的局部最优策略,以满足约束方程为条件,以使目标函数最快(或最慢)为原则,在候选集合中进行一系列的选择,以便尽快构成问题的可行解。
每次选择两个权值最小的二叉树时,规定了较小的为左子树。
