时间序列分析模拟试题3
中级经济师-经济基础知识-基础练习题-第二十七章时间序列分析-三、时间序列的速度分析

中级经济师-经济基础知识-基础练习题-第二十七章时间序列分析-三、时间序列的速度分析[单选题]1.以2005年为基期,2011年和2012年我国国有农场的粮食总产量定基增长速度分别为72.1%和(江南博哥)81.3%。
2012年对2011年的环比发展速度为()。
A.5.3%B.14.1%C.105.3%D.114.1%正确答案:C参考解析:本题考查环比发展速度的计算。
定基发展速度=定基增长速度+1,且两个相邻时期定基发展速度的比率等于相应时期的环比发展速度。
所以本题中,环比发展速度=(81.3%+1)/(72.1%+1)=105.3%。
点赞(1)【环比。
比】所以164.3/137.4 结果大于1 只有D符合点赞(3)发展速度直接除,增长速度要加一点赞(0)发展速度不加一,直接不,增长速度加一后再比[单选题]4.某城市中家庭在2008年的收入为10万元,2016年的收入为36万元,以2008年为基期,则这个家庭收入的平均发展速度是()。
A.102%B.106%C.115%D.117%正确答案:D参考解析:平均发展速度是一定时期内各期环比发展速度的序时平均数,目前计算平均发展速度通常采用几何平均法。
[单选题]5.在时间序列的速度分析中,增长速度可以表示为()。
A.报告期增长量与基期水平的差B.发展水平之比C.报告期增长量与基期水平的比值D.发展速度+1正确答案:C参考解析:此题考查发展速度与增长速度。
增长速度是报告期增长量与基期水平的比值。
[单选题]6.已知某地区近三年社会消费品零售总额的环比增长速度分别为4%、6%、9%,则这时期该地区社会消费品零售总额的定基增长速度为()。
A.4%×6%×9%B.(4%×6%×9%)+1C.(104%×106%×109%)-1D.104%×106%×109%正确答案:C参考解析:此题考查发展速度与增长速度。
时间序列分析试题

2.设时间序列{X t}满足 ARMA(2,1)
(1 − B + 0.5B2 ) Xt = (1 + 0.4B)εt ,
(1)试分析序列{X t}的平稳性,(2)计算前 3 个 Green 函数 G0 、 G1 、 G2 。
(1)此时特征方程为: λ2 − λ + 0.5 = 0 ,特征根满足| λ1,2 |= 2 2 < 1,序列{Xt}平稳。
Xˆ t (1) = EX t +1 = E(0.6 X t + εt − 0.5εt−1) = 0.6 X t + εt − 0.5εt−1 ,
Xˆ t (2) = EX t+2 = E(0.6 X t +1 + εt+1 − 0.5εt )
= 0.6EX t+1 − 0.5εt = 0.36X t + 0.1εt − 0.3εt−1 ;
界平稳。
(4) X t − 1.1X t −1 = εt ;
此时的特征方程为 λ2 − 1.1λ = 0 ,解得 λ1 = 1.1, λ2 = 0 ;模型序列不平稳。 (5) (1 − B)2 X t = εt ;
此时的特征方程为 (λ − 1)2 = 0 ,解得 λ1 = λ2 = 1 ;模型序列不平稳,但是临界平稳。
− θ1εt −2 )
=
−θ1σ
2 ε
;
ρ (1)
=
γ (1) γ (0)
=
− θ1 1 + θ12
,即
ρ (1)θ12
+ θ1
+
ρ (1)
=
0 ,根据可逆性要求,解得θ1
=
0.70
时间序列分析-模拟试卷2套及答案

《时间序列分析》 期中考试模拟试卷(A )1.问答题(1) 常见的数据有哪些种类? (2) 什么是时间序列数据?(3) 常见的时间序列数据有哪些典型特征? (4)如何度量序列相依性?2.名词解释 (1) 平稳性 (2) 遍历性 (3) ACF(4) 长期协方差 (5) 白噪声3.下列自回归过程是否平稳? 若平稳,计算其均值和方差、以及自相关函数。
(1)r t =3+0.95r t−1+a t . (2)r t =1+1.05r t−1+a t .4.下列滑动平均过程是否可逆? (a )若可逆,求出其可逆表示;(b )计算其均值和方差、以及自相关函数。
(1)r t =3+0.95a t−1+a t . (2)r t =1+1.05a t−1+a t .5.证明:若y t =y t−1+u t ,u t 为i.i.d.N(0,σ2),则有T−2∑y t−12d →Tt=1σ2⋅∫[W (r )]2dr 1,T −1∑y t−1u t Tt=1d→σ22{[W (1)]2 −1}.参考答案1. (1)横截面数据、时间序列数据和面板数据;(2)时间序列数据是指同一个个体的一个或者多个特征在一系列时间观测点上的数据;(3) 序列平稳、非平稳、差分平稳、结构变化、季节性、协整、波动率聚集等;(4) 可以使用Pearson 相关系数度量变量之间的线性相关性,以及非线性相关系数,例如Spearman 秩相关系数和Kendall τ相关系数,来度量变量之间的非线性相关关系。
以上度量的共同点在于均为数据之间相依性的度量,并对样本数据得到相应的统计量,进行假设检验;但当时间序列数据之间存在非线性关系时,线性相关度量可能无法反应变量之间的相依性。
2. (1)平稳性分为严平稳和弱平稳,参考定义1.1和定义1.2;(2)遍历性刻画的是时间序列数据之间的相依程度随着数据之间时间间隔的增加而逐渐减弱的特征; (3)序列自相关系数关于阶数的变化的函数即为自相关函数,记为ACF ; (4)长期协方差为平稳时间序列的样本均值乘以√T (即√Ty ̅=√Ty t T t=1)的方差的极限; (5)白噪声是指均值为0、方差有限、且不存在时间维度上的相关性的平稳时间序列;。
时间序列分析练习题

17. 在趋势性检验中,进行单位根检验的意义是什么?
单位根检验就是根据已观测到的时间序列,检验产生这个时间序列的随机过程中的一阶 自回归系数是否为一,这个检验实际上就是对时间序列是否为一个趋势平稳过程的检验,如 果检验表明没有单位根,则它是一个趋势平稳过程,否则,它是一个带趋势的单位根过程。
①( 均值为常数 ) ②( 协方差为时间间隔 的函数 )
则称该序列为宽平稳时间序列,也叫广义平稳时间序列。 8. 对于一个纯随机过程来说,若其期望和方差(均为常数),则称之为白噪声过程。白 噪声过程是一个(宽平稳)过程。 9. 时间序列分析方法按其采用的手段不同可概括为数据图法,指标法和(模型法)
19. 线性趋势平稳的特点:当我们将时间序列中的完全确定的线性趋势去掉以后,所形 成的时间序列就是一个平稳的时间序列。
20. 如何以系统的观点看待时间序列的动态性? 系统的动态性就是在某一时刻进入系统的输入对系统后继行为的影响,也就是系统的记 忆性,描述记忆性的函数称为记忆函数。
三、证明题
1. AR(1)模型: X t 1 X t1 at ,其中 at 是白噪声,且 E at2
37. ARMA(n,m) 的逆转形式 X t I j X t j at 。 j 1
38.
模型适应性检验的相关函数法,在显著性水平
0.05 下,若
k
1.96 /
N,
则接受 k 0 的假设,认为 at 是独立的。
39. 模型适应性检验的 2 检验法,在显著性水平 下,若统计量
G12
G22
时间序列分析模拟试卷3

一、 填空题1. ARMA(p, q)模型_________________________________,其中模型参数为____________________。
2. 设时间序列{}t X ,则其一阶差分为_________________________。
3. 设ARMA (2, 1):1210.50.40.3t t t t t X X X εε---=++-则所对应的特征方程为_______________________。
4. 对于一阶自回归模型AR(1): 110t t t X X φε-=++,其特征根为_________,平稳域是_______________________。
5. 设ARMA(2, 1):1210.50.1t t t t t X X aX εε---=++-,当a 满足_________时,模型平稳。
6. 对于一阶自回归模型MA(1):10.3t t t X εε-=-,其自相关函数为______________________。
7. 对于二阶自回归模型AR(2):120.50.2t t t t X X X ε--=++则模型所满足的Yule-Walker 方程是______________________。
8. 设时间序列{}t X 为来自ARMA(p,q)模型:1111t t p t p t t q t q X X X φφεθεθε----=++++++则预测方差为___________________。
9. 对于时间序列{}t X ,如果___________________,则()~t X I d 。
10. 设时间序列{}t X 为来自GARCH(p ,q)模型,则其模型结构可写为_____________。
如果没有特别说明,在本练习中~,,t i i d ε,()()()2t t 0,,0,t E Var E t τεεσεετ===≠ 11.时间序列{}2,5,9的二阶差分为_________.12.时间序列{}t ε经过一阶差分后序列均值为_________,方差为_________________13.对于时间序列t X ,∆表示差分运算,则111d d d t t t X X X ---∆=∆-∆表示_____阶差分。
时间序列分析考试卷及答案
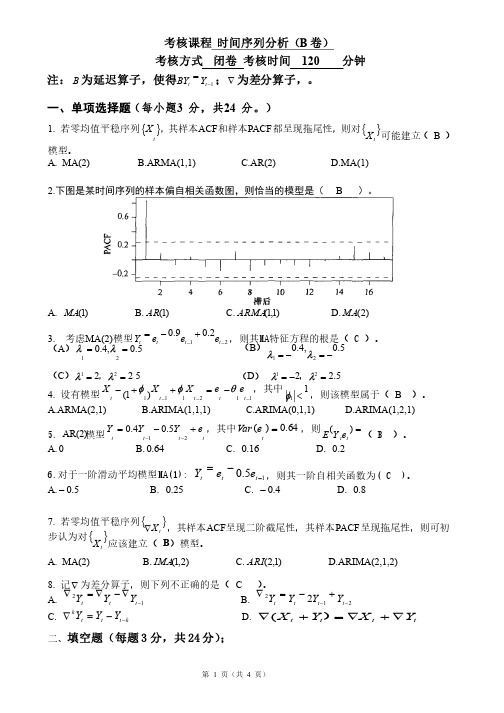
考核课程 时间序列分析(B 卷) 考核方式 闭卷 考核时间 120 分钟注:B 为延迟算子,使得1-=t t Y BY ;Ñ为差分算子,。
一、单项选择题(每小题3 分,共24 分。
)1. 若零均值平稳序列{}tX ,其样本ACF 和样本PACF 都呈现拖尾性,则对{}t X 可能建立( B )模型。
模型。
A. MA(2) B.ARMA(1,1) C.AR(2) D.MA(1) 2.下图是某时间序列的样本偏自相关函数图,则恰当的模型是( B )。
A. )1(MAB.)1(ARC.)1,1(ARMAD.)2(MA3. 考虑MA(2)模型212.09.0--+-=t t t t e e e Y ,则其,则其MA MA MA特征方程的根是(特征方程的根是(特征方程的根是( C C C )。
)。
)。
(A )5.0,4.021==l l (B )5.0,4.021-=-=l l (C )52221==l l , (D ) 5.2221=-=l l ,4. 设有模型112111)1(----=++-t t t t t e e X X X q f f ,其中11<f ,则该模型属于(,则该模型属于( B )。
)。
A.ARMA(2,1) B.ARIMA(1,1,1) C.ARIMA(0,1,1) D.ARIMA(1,2,1) 5. AR(2)模型tt t t e Y Y Y +-=--215.04.0,其中64.0)(=t e V ar ,则=)(t t e Y E ( B B )。
)。
)。
A.0 B.64.0 C. 16.0 D. 2.06.6.对于一阶滑动平均模型对于一阶滑动平均模型对于一阶滑动平均模型MA(1): MA(1): 15.0--=t t t e e Y ,则其一阶自相关函数为,则其一阶自相关函数为( C )( C )( C )。
A.5.0- B. 25.0 C. 4.0- D. 8.07. 若零均值平稳序列{}t X Ñ,其样本ACF 呈现二阶截尾性,其样本PACF 呈现拖尾性,则可初步认为对{}t X 应该建立(应该建立( B )模型。
金融时间序列分析英文试题(芝加哥大学) (3)

Graduate School of Business,University of ChicagoBusiness41202,Spring Quarter2005,Mr.Ruey S.TsaySolutions to MidtermAll tests are based on the5%significance level.Problem A:(30pts)Answer briefly the following questions.1.For problems1to6,consider the daily log return,in percentages,of the S&P500composite index from January1996to December31,2004for2267data points.Sum-mary statistics of the percentage log returns from SCA and Splus are attached.See Page 1of the attached output.Is the mean of percentage log returns significantly different from zero?Why?A:t-ratio=1.187<1.96.Thus,the mean return is not significantly different from zero.2.Suppose one invested$1dollar on the S&P500index at the very beginning of1996andheld the investment until the end of2004.What was the value of the investment at the market closing on December31,2004?A:total log return=0.0299*2267/100=0.67783so that the value=exp(0.67783)≈1.97.3.Test the null hypothesis that the skewness of the log returns is zero.Draw your conclu-sion.A:t-ratio=−0.0939/0.0514=−1.83<1.96so that the skewness is no signifiacnt different from zero.4.Given that the last percentage log return was−0.134(i.e.T=December31,2004),which is the corresponding simple return?A:R t=exp(−0.134/100)−1=−0.001339=.1339%.5.Are the log return serially correlated?You may use Q(10)of the series to answer thequestion.A:Q(10)=13.9with p-value0.178so that there is no serial correlation.6.Is there any ARCH effect in the log return series?You may use Q(12)of the squaredseries to answer the question.A:Yes,Q(12)of squared return is large at490(in SCA)and has a p-value of0.0from Splus.7.Give two empirical features of daily log returns of afinancial asset.A:Any two of(a)high kurtosis,(b)volatility clustering,(3)skew to left.18.What is the purpose of studying kurtosis of an asset return series?A:Understanding the tail behavior(or risk)of the return.9.Describe two applications of studying sample autocorrelation function(ACF)of an assetreturn series.A:(a)To test serial correlations in the return series,(b)to identify MA order.10.Describe two methods that can be used to identify the order of an AR model.A:(a)PACF,(b)Criterion functions such as AIC or BIC.11.Consider the AR(1)model(1−0.9B)r t=0.2+a t,where{a t}is an independent andidentically distributed random variables with mean zero and variance1.0.What is the half-life of the series?A:Half-life=ln(0.5)/ln(0.9)=6.58time units.12.Suppose that the log return r t of an asset follows the model below:r t=0.02+a t,a t=σt t,σ2t=0.116+0.42a2t−1.Let p t be the log price of the asset at time t and p T( )be the -step ahead forecast of the log price at the forecast origin T.Then,what is the value of p T( )as increases?A:0.02is the slope of time trend so that p T( )→∞as increases.13.For problems13-14,consider quarterly series of U.S.unit labor cost from1947to2004.The data were seasonally adjusted and obtained from the Federal Reserve Bank of St Louis.Let x t=(1−B)ULC t be thefirst-differenced series of the data at time t.The model(1−0.371B2)x t=0.265+(1+0.171B4)a t,σa=0.5998,fits the data reasonably well.Under thefitted model,what is the mean of x t,i.e.E(x t) =?A:E(x t)=0.265/(1−0.371)=0.421.14.Does the model imply that there exist business cycles in the unit labor cost?Why? A:No,because the two roots are real.From1−0.371x2,we have x=±1/ (0.371).15.Give two weaknesses of the GARCH-type of models for modeling asset volatility.A:Any two of(a)symmetric response to past positive and negative returns,(b)restric-tive,(c)providing no explanation of volatility clustering,(d)no adaptive in forecasting.2Problem B.(20pts)This problem is concerned with the analysis of quarterly earnings per share of the Procter&Gamble(PG)Company from1992to thefirst quarter of2003 for45data points.The data were obtained from First Call.Log transformation was taken to stabilize the variability of the puter output is attached;p.2-6of output. Due to strong seasonal pattern,which results in models that are close to being non-invertible, we analyze the seasonally differenced series in Splus.Let x t be the logarithm of quarterly earnings per share and w t=(1−B4)x t.Thus,SCA uses x t and Splus uses w t.1.(5points)Because ACF of the log earnings shows strong seasonal pattern,the seasonaldifference(1−B4)is taken.The ACF of the seasonally differenced data indicates no further differencing is necessary.Write down thefitted model for the x t series(not the differenced w t).A:(1−0.47B)(1−B4)x t=0.0508+(1−0.307B4)a t,σa=0.0502.2.(4points)Is the AR coefficient of thefitted model statistically significant?Why?A:Yes,the t-ratio is3.26,which is greater than the critical value1.96.3.(4points)Is there any serial correlation in the residuals of thefitted model?Use Q(12)of the ACF of residuals to answer the question.[Hint:for a chi-square distribution with m degrees of freedom,the expected value is m.]A:Q(12)=8.8which is less than E(χ210)=10so that p-value>0.05.4.(4points)Let T=45be the forecast origin.What are the1-step and2-step aheadforecasts of thefitted model(after taking anti-log transformation)?A:x45(1)=0.912and x45(2)=3.95(from SCA).For Splus,x45(1)=exp(0.0087−.1743)=0.847,x45(2)=exp(−.012479+1.278152)=3.55.5.(3points)Give an interpretation of the estimated constant0.0508of thefitted modelfor x t.A;Slope of time trend.3and the S&P500index from January1999to December2004with sample size T=1508.We employ the market model:r t=α+βr m,t+e t,where r t and r m,t are Wal-Mart stock return and S&P500index return,respectively.Splus output is attached;page6of output.Answer the following questions.1.(4points)Write down thefitted market model.A:r t=0.0205+0.9606r m,t+e t.2.(4points)The Ljung-Box statistics of the ACF of residuals show some minor serialcorrelations,but the ACFs are relatively small so we ignore the serial correlations and perform the ARCH effect test.Is there an ARCH effect in the residuals of thefitted market model?A:Yes,archTest gives a p-value about0.0.3.(4points)We employ a GARCH(1,1)model(called“m2”in the output).Write downthefitted ment on thefitted model.A:Let r t and r m,t be the Wal-Mart stock return and S&P500index return,respectively.The model isr t=0.9386r m,t+a t=0.9386+σt t, t∼t6.41σ2t=−4.29×10−5+0.0377a2t−1+0.963σ2t−1.The negative estimate ofα0does not make sense,but it is statistically not different from0.Also,theˆα1+ˆβ1≈1so that thefitted model indicates an IGARCH(1,1)model without the constant.4.(6points)Further analysis indicates that an EGARCH(2,1)modelfits the data better.There are two leverage effect parameters.Are these two effects statistically significant?Why?You may test the effect individually.A:Examine the t-ratio of the two leverage parameter estimates.For Lev(1),the t-atio is−2.129.For Lev(2),the t-ratio is−1.847.In both cases,the p-values are less tha0.05so that they are both significant.[It you use two-sided tests,then Lev(2)is notsignificant.]5.(2points)What are the1-step ahead forecasts for the return and its volatility of Wal-Mart stock at the forecast origin T=1508using the EGARCH model.A:zero for return and0.7082for volatility.4from January1995to December2004with2519observations.Splus output is attached;page 8of output.Answer the following questions.The ACFs of the returns are small so that the mean equation consists of a constant term only.1.(5points)Consider thefitted GARCH(1,1)model.The volatility equation isσ2t=0.042+0.049a2t−1+0.944σ2t−1.Letηt=a2t−σ2t.Rewrite the prior volatility equation in an ARMA form for the{a2t} series.A:a2t=0.042+0.993a2t−1+ηt−0.944ηt−1.2.(5points)Write down thefitted EGARCH(1,1)model with leverage effect(both meanand volatility equations and the parameter of the conditional distribution used).A:The model isr t=0.04918+a t=0.04918+σt t, t∼t6.68ln(σ2t)=−0.06316+0.1033(|a t−1|−0.5464a t−1σt−1)+0.989ln(σ2t−1).3.(4points)Test the hypotheses H o:v=5vs H a:v=5,where v is the degrees offreedom of the conditional Student t distribution.Draw your conclusion.[Hint:you may use the usual t-ratio test.]A;t-ratio=6.684−5=2.62>1.96.Thus,reject H o:v=5.4.(5points)To better understand the leverage effect,use thefitted EGARCH(1,1)modelto calculate the ratioσ2t( =−3)2t ,where t denotes the iid sequence of the innovationsdefined in class.A:From thefitetd volatility equation,we haveσ2t=exp(−0.06316)(σ2t−1).989exp(0.1033| t−1|−0.0564 t−1). Therefore,σ2t( =−3)σ2t( =3)=exp(−0.0564(−3))exp(−0.0564(3))=exp(0.0564×6)=1.403.5.(4points)Used thefitted EGARCH model and T=2519as the forecast origin.Whatare the1-step ahead forecasts of log return and volatility?A:Forecast of log return is0.0492and forecast of volatility is1.184.6.(4points)Write down the mean equation of thefitted GARCH-M model for the data.A:r t=0.058+0.00629σ2t+a t.7.(3points)Based on the GARCH-M model,is the risk premium statistically significant?Why?A:No,the t-ratio is0.453with p-value=0.65(two-sided).5。
(时间管理)第章时间序列分析习题

(时间管理)第章时间序列分析习题第8 章时间序列分析壹、填空题:1.平稳性检验的方法有、和。
2.单位根检验的方法有:和。
3.当随机误差项不存于自关联时,用进行单位根检验;当随机误差项存于自关联时,用进行单位根检验。
4.EG 检验拒绝零假设说明。
5.DF 检验的零假设是说被检验时间序列。
6.协整性检验的方法有和。
7.于用壹个时间序列对另壹个时间序列做回归时,虽然俩者之间且无任何有意义的关系,但经常会得到壹个很高的的值,这种情况说明存于问题。
8.结构法建模主要是以来确定计量经济模型的理论关系形式。
9.数据驱动建模以作为建模的主要准则。
10.建立误差校正模型的步骤为壹般采用俩步:第壹步,;第二步, 。
二、单项选择题:1.某壹时间序列经壹次差分变换成平稳时间序列,此时间序列称为()。
A.1 阶单整 B.2 阶单整C.K 阶单整 D.之上参考答案均不正确2.如果俩个变量均是壹阶单整的,则()。
A.这俩个变量壹定存于协整关系B.这俩个变量壹定不存于协整关系C.相应的误差修正模型壹定成立D.仍需对误差项进行检验3.当随机误差项存于自关联时,进行单位根检验是由()来实现。
ADF 检验 B.ADF 检验C.EG 检验 D.DW 检验4.有关 EG 检验的说法正确的是()。
A.拒绝零假设说明被检验变量之间存于协整关系B.接受零假设说明被检验变量之间存于协整关系C.拒绝零假设说明被检验变量之间不存于协整关系D.接受零假设说明被检验变量之间不存于协整关系三、多项选择题:1.平稳性检验的方法有()。
A.散点图B.自关联函数检验C.单位根检验D.ADF 检验2.当时间序列是非平稳的时候()。
A.均值函数不再是常数B.方差函数不再是常数C.自协方差函数不再是常数D.时间序列的统计规律随时间的位移而发生变化3.随机游走序列是()序列。
A.平稳序列B.非平稳序列C.统计规律不随时间的位移而发生变化的序列D.统计规律随时间的位移而发生变化的序列4.下面能够做协整性检验的有()。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
诚实考试吾心不虚 ,公平竞争方显实力,
考试失败尚有机会 ,考试舞弊前功尽弃。
上海财经大学《时间序列分析》课程考试卷
课程代码 课程序号
20 —20 学年第一学期
姓名 学号 班级
一、 单项选择题(每小题4分,共计20分) 1. t X 的d 阶差分为 (a )=d t t t k X X X -∇- (b )11=d d d t t t k X X X ---∇∇-∇
(c )111=d d d t t t X X X ---∇∇-∇ (d )11-12=d d d t t t X X X ---∇∇-∇ 2. 记B 是延迟算子,则下列错误的是
(a )01B = (b )()1=t t t B c X c BX c X -⋅⋅=⋅ (c )()11=t t t t B X Y X Y --±± (d )()=1d d t t d t X X B X -∇-=- 3. 关于差分方程1244t t t X X X --=-,其通解形式为 (a )1222t t c c + (b )()122t c c t + (c )()122t c c - (d )2t c ⋅
4. 下列哪些不是MA 模型的统计性质 (a )()t E X μ= (b )()()22111q t Var X θθσ=+++L
(c )()(),,0t t t E X E με∀≠≠ (d )1,,0q θθ≠K
……………………………………………………………装
订
线
…………………………………………………
5.上面左图为自相关系数,右图为偏自相关系数,由此给出初步的模型识别
(a)MA(1)(b)ARMA(1, 1)
(c)AR(2)(d)ARMA(2, 1)
二、填空题(每小题2分,共计20分)
1.在下列表中填上选择的的模型类别
2.时间序列模型建立后,将要对模型进行显著性检验,那么检验的对象为___________,
检验的假设是___________。
3.时间序列模型参数的显著性检验的目的是____________________。
4.根据下表,利用AIC和BIC准则评判两个模型的相对优劣,你认为______模型优于______
模型。
5.时间序列预处理常进行两种检验,即为_______检验和_______检验。
三、(10分)设{}
t
ε为正态白噪声序列,()()2
t t
0,
E Var
εεσ
==,时间序列}
{
t
X来自
11
0.8
t t t t
X Xεε
--
=+-
问模型是否平稳为什么
得
分
得
分
四、(20分)设}{t X 服从ARMA(1, 1)模型: 110.80.6t t t t
X X εε--=+-
其中1001000.3,0.01X ε==。
(
1)
给出未来3期的预测值;(10分) (2)
给出未来3期的预测值的95%的预测区间(0.975 1.96u =)。
(10分)
五、 (20分)下列样本的自相关系数和偏自相关系数是基于零均值的平稳序列样本量为500计算得到的(样本方差为)
ACF: 0:340; 0:321; 0:370; 0:106; 0:139; 0:171; 0:081; 0:049; 0:124; 0:088; 0:009; 0:077
PACF: 0:340; 0:494; 0:058; 0:086; 0:040; 0:008; 0:063; 0:025; 0:030; 0:032; 0:038; 0:030
根据所给的信息,给出模型的初步确定,并且根据自己得到的模型给出相应的参数估计,要求写出计算过程。
六、(10分)设}{t X 服从AR (2)模型: 1121t t t t X X X ααε--=++
其中{}t ε为正态白噪声序列,()()2t t 0,E Var εεσ==,假设模型是平稳的,证明其偏自
相关系数满足
2
203
kk k k αφ=⎧=⎨≥⎩。
