信号与系统双语课件chapter1.3
合集下载
信号与系统第一章课件
2. Discrete-Time Signals
—— The independent variable is discrete
11 xn
10 8
5
4
1
1
01 2 34 5 6 7
n is integer number
n
Continuous-time signals
Discrete-time signals
R
R i(t)
+ v(t) -
① t1 t t2
E t2 pt dt 1 t2 v2 t dt
t1
R t1
n1 n n2
n2
E x2 n
nn1
② t
E
ptdt 1
R
v2 t dt
§ 1.1.2 Signal Energy and Power v( t) —— voltage i( t) —— current
7
Chapter 1
Signals and Systems
1. Instantaneous power
瞬时功率 2. Total energy
pt vtit 1 v2t
Chapter 1
Signals and Systems
Chapter 1 Signals and Systems
• The mathematical description and representations of signals and systems.
• Signals and Systems arise in a broad array of application.
信号与系统(英文)chapter 1-信号与系统
1.5.2 Discrete-Time Complex Exponential and Sinusoidal signals(sequences)（序列）: n
x[n] = Cα
Ⅰ.Real Exponential Signals: if C﹑a are real Ⅱ. General Complex Exponential Signals * both C and a are complex numbers
x[n] = x[n + N] for all values of n. In this case, we say that x(t) (x[n]) is periodic with period T(N).
Example 1.1 Determine the fundamental period of the signal x(t) = 2cos(10πt+1)-sin(4πt-1).
1.5 SEVERAL BASIC SIGNALS
1.5.1 Continuous-time Complex Exponential （复 指数函数） and Sinusoidal Signals（正弦信号） at complex exponential signals:
x(t ) = Ce
Ⅰ.Real Exponential Signals: if C﹑a are real Ⅱ. Periodic Complex Exponential and Sinusoidal Signals : * if C is real and a purely imaginary jω 0 t periodic complex exponential
∫
T
−T
英文版《信号与系统》第3章讲义
Fourier Series
xt ake
k
jk 0t
h t
jk t 0 y t a H jk e k 0 k
j t j H h t e dt
Filter
Frequency-Shaping Filter 频率成形滤波器 Frequency-Selective Filter 频率选择性滤波器
a k real even a k Purely imaginary odd
10
Chapter 3 §3.5.7 Parseval’s Relation（帕兹瓦尔关系式）
1 2 2 x t dt a k T T k
Fourier Series
Average Power of x t
Fourier Series
-4 -2 0
1
FS x t c k
2
4
t
T 4 0
2
-4 -2
1 2 1 ① c tdt 0 x T T 4 2
jk 2
1
1
0
t d2x FS d k 2 dt
2 4 t
11 2 1 k 1 ② d e 1 1 k 2 44 4 1 ck 2 2 d jk c 2 jk / 2 k 0 k 0
1 jk t 0 a x t e dt k T 0 T 0
1 T0 Specially a0 xtdt ——Average value 0 T 0 Example 1 1 a1 j a-1 j 1j 1 t j t 0 0 2 2 1 sin t e e 0 2 j 2 j ak 0 k 1
Signals and Systems 信号与系统英文版第一节
vs, vc
Continuous & Discrete-Time Signals
Continuous-Time Signals
Most signals in the real world are continuous time, as the scale is infinitesimally（无穷小） fine. Eg voltage, velocity, Denote by x(t), where the time interval（间隔） may be bounded (finite) or infinite
– Voltages and currents in a circuit
Acoustic signals
– Acoustic pressure (sound) over time
Mechanical signals
– Velocity of a car over time
Video signals
– Intensity level of a pixel (camera, video) over time
How is a Signal Represented?
Mathematically, signals are represented as a function of one or more independent variables. For instance a black & white video signal intensity(亮度) is dependent on x, y coordinates and time t f(x,y,t) On this course, we shall be exclusively concerned with signals that are a function of a single variable: time
信号与系统 第一章课件
Ch1. Signals and Systems SIGNALS and SYSTEMS信号与系统任课老师：罗伟E-mail: teacherluowei@Ch1. Signals and Systems •本“信号与系统”课程所讨论的主要内容是：描述确定信号与线性时不变系统的基本数学方法和分析确定信号通过线性时不变系统的基本数学方法。
信号与系统四川大学电气信息工程学院201X 年春（64学时）序言•要求本课程注册学生应具备：1.进行复数运算和多项式运算的能力。
2.微积分学和求解常系数常微分方程的基础知识。
3.电路、电子电路、电工测量技术的基本理论与实践。
Ch1. Signals and Systems1SIGNALS AND SYSTEMS信号与系统Ch1. Signals and Systems Main content ：1.Continuous-Time and Discrete-Time Signals（连续时间与离散时间信号）2.Transformations of the IndependentVariable（自变量的变换）3.Exponential and Sinusoidal Signals（指数信号与正弦信号）4.The Unit Impulse and Unit StepFunctions（单位冲激与单位阶跃函数）5.Continuous-Time and Discrete-TimeSystems (连续时间与离散时间系统)6.Basic System Properties(基本系统性质)1.1 CONTINUOUS-TIME AND DISCRETE-TIME SIGNALS (p1)1.1.1 Examples and Mathematical Representation (p1) (举例与数学表示)•Signals: physical phenomena or physicalquantities, which change with time or space.•Functions of one or more independent variables. example: x(t)Figure 1.1 (p2)A simple RC circuit.A speech signalFigure 1.3 (p2) “Should we chase” 这句话的鸟鸣声的时域波形，其幅值是时间的一元函数心电图——幅值是时间的一元函数A pictureFigure 1.4 (p3) 一幅黑白(monochrome)照片可的函数是是像素的位置 :图片上 B},G ,R { ),( C n m ),(n m CContinuous-time and Discrete-time Signals（连续时间与离散时间信号）•continuous-time signals’ independent variable is continuous : x(t)(p3)•对一切时间t (除有限个不连续点外) 都有确定的函数值，这类信号就称为连续时间信号，简称连续信号。
信号与系统课件(英文)讲解
balance --- y[n] net deposit --- x[n] interest --- 1% so y[n]=y[n-1]+1%y[n-1]+x[n] or y[n]-1.01y[n-1]=x[n]
x[n] Balance in bank y[n]
(sytem
x(t)
t1 y(t)
t2
1 Signal and System
1.6.4 Stability
x[n]
Discrete-time y[n]
System
SISO system
MIMO system?
1 Signal and System
1.5.1 Simple Example of systems
Example 1.8:
RC Circuit in Figure 1.1 : Vc(t) Vs(t)
Memoryless system: It’s output is dependent only on the input at the same time. Features: No capacitor, no conductor, no delayer.
Examples of memoryless system: y(t) = C x(t) or y[n] = C x[n]
Representation of System: (1) Relation by the notation
x(t) L y(t)
x[n] L y[n]
1 Signal and System
(2) Pictorial Representation
x(t) Continous-time
System
x[n] Balance in bank y[n]
(sytem
x(t)
t1 y(t)
t2
1 Signal and System
1.6.4 Stability
x[n]
Discrete-time y[n]
System
SISO system
MIMO system?
1 Signal and System
1.5.1 Simple Example of systems
Example 1.8:
RC Circuit in Figure 1.1 : Vc(t) Vs(t)
Memoryless system: It’s output is dependent only on the input at the same time. Features: No capacitor, no conductor, no delayer.
Examples of memoryless system: y(t) = C x(t) or y[n] = C x[n]
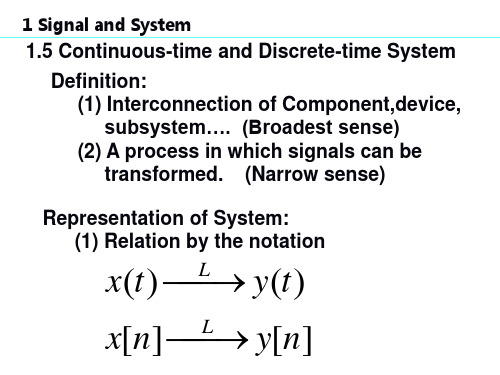
Representation of System: (1) Relation by the notation
x(t) L y(t)
x[n] L y[n]
1 Signal and System
(2) Pictorial Representation
x(t) Continous-time
System
信号与系统SignalsandSystemsppt课件
0.5
0.4
0.3
0.2
0.1
0
0
1
2
3
4
5
6
7
8
9
10
1
0.9
0.8
0.7
0.6
0.5
0.4
0.3
0.2
0.1
0
0
1
2
3
4
5
6
7
8
9
10
一、基本信号的MATLAB表示
% rectpuls
t=0:0.001:4; T=1; ft=rectpuls(t-2*T,T); plot(t,ft) axis([0,4,-0.5,1.5])
rand
产生(0,1)均匀分布随机数矩阵
randn 产生正态分布随机数矩阵
四、数组
2. 数组的运算
数组和一个标量相加或相乘 例 y=x-1 z=3*x
2个数组的对应元素相乘除 .* ./ 例 z=x.*y
确定数组大小的函数 size(A) 返回值数组A的行数和列数（二维） length(B) 确定数组B的元素个数（一维）
0.3
0.2
0.1
function [f,k]=impseq(k0,k1,k2) 0
-50 -40 -30 -20 -10
0
10 20 30 40 50
%产生 f[k]=delta(k-k0)；k1<=k<=k2
k=[k1:k2];f=[(k-k0)==0];
k0=0;k1=-50;k2=50;
[f,k]=impseq(k0,k1,k2);
已知三角波f(t)，用MATLAB画出的f(2t)和f(2-2t) 波形
信号与系统-课件-郑君里
School of Computer Science and Information
1.1 Signals
Signals are functions of independent variables that carry information. The independent variables can be continuous or discrete. The independent variables can be 1-D, 2-D, ••• , n-D. For this course: Focus on a single (1-D) independent variable which we call “time”. Continuous-Time signals: x(t), t-continuous values. Discrete-Time signals: x(n), n-integer values only.
School of Computer Science and Information
Examples
Electrical signals — voltages and currents in a circuit. Acoustic signals — audio or speech signals. Video signals — intensity variations in an image. Biological signals — sequence of bases in a gene.
School of Computer Science and Information
1.3 Types of Signals
1. Certain Signal and Random Signal
1.1 Signals
Signals are functions of independent variables that carry information. The independent variables can be continuous or discrete. The independent variables can be 1-D, 2-D, ••• , n-D. For this course: Focus on a single (1-D) independent variable which we call “time”. Continuous-Time signals: x(t), t-continuous values. Discrete-Time signals: x(n), n-integer values only.
School of Computer Science and Information
Examples
Electrical signals — voltages and currents in a circuit. Acoustic signals — audio or speech signals. Video signals — intensity variations in an image. Biological signals — sequence of bases in a gene.
School of Computer Science and Information
1.3 Types of Signals
1. Certain Signal and Random Signal
- 1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。
x[n] y[n]
Simple example of system:
vs (t ) vc (t ) i (t ) R dv c (t ) i (t ) C dt
(1) (2)
Finally ,we obtain a differential equation describing the relationship between the input vs (t ) and output vc (t ) :
u (t )
( ) d ( t s ) d s
0
t
Therefore
k ( )d ku(t )
t
Unit impulse can be thought of as the first derivative of the continuous-time unit step:
We also have an analogous expression for an impulse concentrated at an arbitrary point,t0,that is:
x(t ) (t t0 ) x(t0 ) (t t0 )
1.5 Continuous-time and discrete-time system
1.6.2 Invertibility and inverse system
y[n]
k
x[k ]
And a second example is a delay:
y[n] x[n 1]
A capacitor is an example of a continuous-time system with memory: t 1 y(t ) x( )d C
x[n] [n] x[0] [n]
More generally, if we consider a unit impulse [n n0 ] at n n0
x[n] [n n0 ] x[n0 ] [n n0 ]
Note :The sampling property of unit impulse is very important.
y (t ) x(t )
Or
y(n) x(n)
2. A system with memory if the current output is dependent on past value or the future value of the input and output. An example of the discrete-time system with is an accumulator or summer: n
Ch1.6 Basic system properties
1. System with and without memory 2. Invertibility and inverse system 3.Causality
4. Stability
5.Time-Invariance 6. linearity
System 2
output addition
Here , the same input signals is apply to system 1 and system 2.
We can combine both series and parallel interconnections to obtain more complicated interconnections. An example is as follow:
notation for it shown in figure before, where the arrow at t=0 indicates that the area of the pulse is concentrate at t=0 and the height of the arrow and the “1” next to the arrow are used to represent the area of the impulse.
y[n] (2x[n] x 2[n])2
memoryles s
Similarly ,a resistor is a memoryless system Voltage
y(t ) Rx(t )
Current
One particular simple memoryless system is the identity system, whose output is identical to input. That is
1.6.1 System with and without memory
1. A system is said to be memoryless if its input for each value of the independent variable at a given time is depended only on the input at that time. For example:
Physical system in the broadest sense are an interconnection of components, devices, or subsystem. A system can be viewed as a process in which input signals are transformed by the system or cause the system to respond in some way. input
du (t ) (t ) dt
4 Sampling property
As with the discrete-time impulse, the continuous-time impulse has a very important sampling property.
x(t ) (t ) x(0) (t )
output
vs
RC circuit
vc
syste
A continuous-time system is a system in which continuoustime input signals are applied and result in continuous-time output signals.
x(t )
Continuous-time system
y(t )
We often represent the input-output relation by the notion:
x(t ) y(t )
Discrete-time system:
x[n]
Discrete-time system
y[n]
1.4 The unit impulse and unit step function
1.4.1 The discrete-time unit impulse and unit step sequence
1 Unit impulse:
0 [ n] 1
n0 n0
Throughout the book, we refer [n] interchangeably as the unit impulse or unit sample.
2 Unit step: u[n]
0 u[n] 1
n0 n0
3 Relationship between the discrete-time unit impulse and unit step
(1) The unit impulse is the first difference of the step:
System 1
System 2
Input
System 3
System 4
output
3 Feedback interconnection :
Here ,the output of system 1 is the input to system 2,while the output of system 2 is fed back and addeduce the actual input to system 1.
1.4.2 The continuous-time unit step and unit impulse function 1 Unit step:
0 u (t ) 1
t0 t 0
2 Unit impulse:
(t )
1
0
t
(t ) has no duration but unit area, we adopt the graphical
dvc (t ) 1 1 vc (t ) vs (t ) dt RC RC
1.5.2 Interconnections of system
Many real systems are built as interconnections of several subsystems. By viewing system as an interconnection of its components, we can use our understanding of the component systems and of how they are interconnected in order to analyze the operation and behavior of the overall system. In addition, by describing a system in terms of an interconnection of simpler subsystem, we may in fact be able to define useful ways in which to synthesize complex systems out of simpler, basic building blocks.