GF(p)中高阶同余方程 x^ n = a (mod p)的平凡解
初等数论闵嗣鹤第四版答案

初等数论闵嗣鹤第四版答案介绍《初等数论闵嗣鹤第四版答案》是对闵嗣鹤所著《初等数论》第四版的习题答案进行了整理和解析。
《初等数论》是普通高校数学系本科生的一门基础课程,有助于培养学生的数学思维和推理能力。
通过学习该答案,学生可以更好地理解和掌握《初等数论》中的知识点,并提高解题能力。
目录1.第一章素数2.第二章同余3.第三章数论函数4.第四章域上的多项式5.第五章幂的剩余与解方程6.第六章整数的几何性质第一章素数1.1 什么是素数?简要解答:素数指的是只能被1和自身整除的正整数。
详细解答:一个大于1的正整数如果只能被1和它本身整除,则称之为素数,也叫质数。
反之,如果大于1的正整数可以被其他正整数整除,则称之为合数。
最小的素数是2。
1.2 素数的性质简要解答:素数有无限多个,并且一个数是否是素数可以通过试除法判断。
详细解答:欧几里得证明了素数有无限多个的结论。
对于给定的一个正整数n,如果在2到√n之间找不到小于n的因数,那么n就是素数。
这就是试除法。
试除法是素数判断的基础,但它的效率不高,因为需要逐个试除所有小于n的数。
1.3 素数的应用简要解答:素数在密码学和随机数生成中经常被使用。
详细解答:由于素数具有唯一分解性质,使得许多密码学算法中的关键操作依赖于素数。
比如RSA算法中,公钥和私钥的生成需要使用两个大素数。
此外,素数还在随机数生成和随机性检验中发挥重要作用。
第二章同余2.1 什么是同余?简要解答:同余是数论中的一种等价关系。
详细解答:a和b对模m同余,记作a≡b(mod m),当且仅当a和b的差是m的倍数。
同余关系具有三个基本性质:反身性、对称性和传递性。
同余关系的性质使得其在数论中有广泛的应用。
2.2 同余定理简要解答:同余定理是一类用来计算同余的定理,包括欧拉定理、费马小定理等。
详细解答:欧拉定理是指当a和m互质时,a的φ(m)次方与1同余模m,其中φ(m)表示不大于m且与m互质的正整数的个数。
同余方程与模方程的解法

同余方程与模方程的解法一、同余方程在数论中,同余方程是指形如ax ≡ b (mod m) 的方程,其中 a、b、m 为整数。
解同余方程的方法有多种,下面将介绍两种常用的解法。
1. 穷举法:穷举法是最简单直观的解同余方程的方法之一。
具体步骤如下:(1)列出满足条件的整数集合。
根据同余的定义,我们知道 x 和 b 对 m 取余数是相同的,即 x 和 b 在模 m 意义上是相等的。
因此,我们可以列出一个整数集合 S,其中的元素 x 满足x ≡ b (mod m)。
(2)从集合中选出满足条件的解。
根据具体的题目要求,我们可以从集合 S 中选出满足方程的解。
2. 扩展欧几里得算法:扩展欧几里得算法是一种高效解同余方程的方法。
它利用了欧几里得算法的思想,通过递归求解,最终得到同余方程的解。
具体步骤如下:(1)求解递归基。
如果 b = 0,则方程变为ax ≡ 0 (mod m),此时方程的解为 x = m / (a, m),其中 (a, m) 表示 a 和 m 的最大公因数。
(2)求解通解。
如果b ≠ 0,则根据同余方程的性质可知,ax ≡ b (mod m) 的解与 ax ≡ 1 (mod m) 的解具有相同的形式。
因此,我们可以利用扩展欧几里得算法求解 ax + my = (a, m),其中 y 是方程ax ≡ 1 (mod m) 的一个解。
(3)求解特解。
根据通解的形式,我们可以求解出 ax + my = (a, m) 的一个特解 x0。
然后,利用 x = x0 * (b / (a, m)),即可求得同余方程的特解。
二、模方程模方程是指形如x² ≡ a (mod m) 的方程,其中 a、m 为整数。
解模方程的方法有多种,下面将介绍两种常用的解法。
1. 勒让德符号和二次互反律:勒让德符号是数论中的一个重要概念,它用来判断二次剩余和二次非剩余。
对于模方程x² ≡ a (mod p)(p 是奇素数),可以利用勒让德符号判断 a 是否是模 p 的二次剩余。
模为素数幂的同余式与hensel引理-概述说明以及解释

模为素数幂的同余式与hensel引理-概述说明以及解释1. 引言1.1 概述在数论领域,同余式和Hensel引理是两个重要的概念。
同余式是一种数学关系,表示两个数在给定的模数下具有相同的余数。
模为素数幂的同余式是指同余式中的模数为素数的幂次方。
本文的目的是探讨模为素数幂的同余式与Hensel引理之间的关系。
首先,我们会介绍同余式的定义及其在数论中的重要性。
接着,我们将详细研究模为素数幂的同余式的特性,包括它们的性质和应用。
然后,我们会引入Hensel引理,并深入了解它的原理、证明以及其在数论研究中的应用。
在文章的结论部分,我们将探讨模为素数幂的同余式与Hensel引理之间的关系,并总结本文的主要内容。
最后,我们将提出一些进一步研究的方向,以便读者可以在这一领域中进行深入探索。
通过本文的阅读,读者将能够对模为素数幂的同余式和Hensel引理有更深入的理解,进一步拓宽数论领域的知识面,并为未来的研究提供更多的思路与启发。
1.2 文章结构文章结构部分的内容如下:2. 正文2.1 模为素数幂的同余式同余式是数论中非常重要的概念,它涉及到数的整除关系。
在本节中,我们将介绍模为素数幂的同余式的定义、特性以及应用。
2.1.1 同余式的定义同余式是指在某个模下,两个数之间的差是该模的倍数。
形式上,如果两个整数a和b满足a≡b (mod m),那么a和b对于模m是同余的。
2.1.2 模为素数幂的特性在数论中,模为素数幂的同余式具有一些独特的性质。
我们将详细介绍模为素数幂的同余式的特性,包括同余关系的传递性、可逆性以及模运算的基本性质。
2.1.3 同余式的应用模为素数幂的同余式在密码学、编码理论和离散数学等领域有广泛的应用。
我们将讨论一些具体应用场景,如RSA加密算法中的同余式应用、纠错编码中的同余式运算等。
2.2 Hensel引理Hensel引理是一种重要的数论工具,它在解决一些关于同余式的问题时具有很大的帮助。
湖南省株洲市第二中学2023-2024学年高一下学期期中数学试题
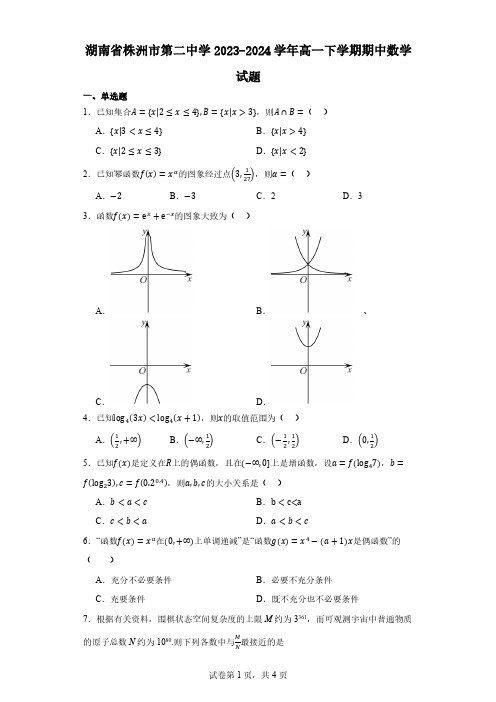
湖南省株洲市第二中学2023-2024学年高一下学期期中数学试题一、单选题1.已知集合A={x|2≤x≤4},B={x|x>3},则A∩B=()A.{x|3<x≤4}B.{x|x>4}C.{x|2≤x≤3}D.{x|x<2}A.−2B.−3C.2D.33.函数f(x)=e x+e−x的图象大致为()A.B.、C.D.4.已知log4(3x)<log4(x+1),则x的取值范围为()5.已知f(x)是定义在R上的偶函数,且在(−∞,0]上是增函数,设a=f(log47),b= f(log23),c=f(0.20.4),则a,b,c的大小关系是()A.b<a<c B.b<c<aC.c<b<a D.a<b<c6.“函数f(x)=x a在(0,+∞)上单调递减”是“函数g(x)=x4−(a+1)x是偶函数”的()A.充分不必要条件B.必要不充分条件C.充要条件D.既不充分也不必要条件7.根据有关资料,围棋状态空间复杂度的上限M约为3361,而可观测宇宙中普通物质的原子总数N约为1080.则下列各数中与M最接近的是(参考数据:lg3≈0.48)A.1033B.1053C.1073D.10938.定义域为R的函数f(x)满足:当x∈[0,1)时,f(x)=3x−x,且对任意的实数x,均则f(ab)+f(a)+f(2a)=()有f(x)+f(x+1)=1,记a=log32,b=log213二、多选题9.成人心率的正常范围为60~100次/分钟,超过100次/分钟为心率过速,观测并记录一名心率过速成人患者服用某种药物后心率,其随时间的变化如图所示,则该患者()A.服了药物后心率会马上恢复正常B.服药后初期药物起效速度会加快C.所服药物约15个小时后失效(服药后心率下降期间为有效期)D.欲控制心率在正常范围内,一天需服用该药2次10.下列不等式的解集为R的是()A.x2+6x+11>0B.x2−3x−3<0C.−x2+x−2<0D.x2+2√5x+5≥011.下面结论正确的是()曼函数R (x )(x ∈[0,1]),下列说法正确的是( )三、填空题四、解答题 17.求值:(1)80.25×√24+(6427)13−(−2021)0;(2)lg25+lg2⋅lg50+(lg2)2.18.已知函数y =b ⋅a x 是指数函数.(1)该指数函数的图象经过点(3,8),求函数的表达式; (2)解关于x 的不等式:a 3x−4>(1a )3;19.已知函数f (x )=(log 2x −2)(log 2x −1). (1)当x ∈[2,8]时,求该函数的值域;(2)若f (x )≥mlog 2x 对于x ∈[4,16]恒成立,求m 的取值范围.20.近年来,中国自主研发的长征系列运载火箭的频频发射成功,标志着中国在该领域已逐步达到世界一流水平.设火箭推进剂的质量为M(单位:t),去除推进剂后的火箭有效载荷质量为m(单位:t),火箭的飞行速度为v(单位:km s⁄),初始速度为v0(单位:km s⁄),已知其关系式为齐奥尔科夫斯基公式:v=v0+ω⋅ln(1+Mm),其中ω是火箭发动机喷流相对火箭的速度.假设v0=0km/s,m=25t.(参考数据:e 16.73≈261.56,ln80≈4.382).(1)若ω=3km/s,当火箭飞行速度达到第三宇宙速度(16.7km s⁄)时,求相应的M;(精确到小数点后一位)(2)如果希望火箭飞行速度达到16.7km s⁄,但火箭起飞质量的最大值为2000t,请问ω的最小值为多少?(精确到小数点后一位)21.设函数f(x)=ka x−a−x(a>0且a≠1,k∈R),f(x)是定义域为R的奇函数.(1)求k的值:(2)已知a=3,若∃x∈[log32,2],使f(2x)+2⋅3−2x≥λ⋅f(x)成立.请求出最大的整数λ.22.已知函数f(x)=a x(a>0且a≠1),其反函数为y=g(x).(1)若a=2,求g(x)的解析式;(2)若函数y=g[f(x)+3k−1]值域为R,求实数k的取值范围;(3)定义:若函数f(x)与g(x)在区间[a,b],(a<b)上均有定义,且∀x∈[a,b],恒有|f(x)−g(x)|≤1,则称函数f(x)与g(x)是[a,b]上的“粗略逼近函数”.若函数g(x−3a)和g(1x−a)是[a+2,a+3]上的“粗略逼近函数”,求实数a的最大值.参考答案:1.A【分析】应用集合的交运算求A ∩B 即可.【详解】由题设A ∩B = {x|2≤x ≤4}∩{x|x >3}={x|3<x ≤4}. 故选:A 2.B【分析】根据点的坐标满足幂函数方程,代入计算可得α=−3. 【详解】将点(3,127)代入可得3α=127,解得α=−3. 故选:B 3.D【分析】B 选项的不是函数图象,故排除,再结合特殊值排除AC 选项. 【详解】先排除B 选项,因为不是函数图象; f (0)=e 0+e −0=2,排除AC 选项. 故选:D 4.D【分析】根据对数函数单调性和定义域分析求解. 【详解】因为y =log 4x 在定义域(0,+∞)内单调递增,若log 4(3x )<log 4(x +1),则0<3x <x +1,解得0<x <12,所以x 的取值范围为(0,12).故选:D. 5.A【详解】根据指、对数函数单调性可得0<0.20.4<1<log 47<log 23,结合偶函数的性质分析判断.【分析】因为1<log 47=12log 27=log 2√7<log 23,即1<log 47<log 23,又因为0<0.20.4<0.20=1,即0<0.20.4<1, 可得0<0.20.4<1<log 47<log 23,由题意可知:f (x )在[0,+∞)上单调递减,所以b <a <c . 故选:A. 6.B【分析】通过求解函数f (x )和g (x )符合条件的a 的取值,即可得出结论. 【详解】由题意,在f (x )=x a 中, 当函数在(0,+∞)上单调递减时,a <0, 在g (x )=x 4−(a +1)x 中,函数是偶函数, ∴{g (−x )=(−x )4−(a +1)(−x )g (x )=x 4−(a +1)xg (x )=g (−x ),解得:a =−1, ∴“函数f (x )=x a 在(0,+∞)上单调递减”是“函数g (x )=x 4−(a +1)x 是偶函数”的必要不充分条件, 故选:B. 7.D【详解】试题分析:设MN =x =33611080 ,两边取对数,lgx =lg 33611080=lg3361−lg1080=361×lg3−80=93.28,所以x =1093.28,即MN 最接近1093,故选D.【名师点睛】本题考查了转化与化归能力,本题以实际问题的形式给出,但本质就是对数的运算关系,以及指数与对数运算的关系,难点是令x =33611080,并想到两边同时取对数进行求解,对数运算公式包含log a M +log a N =log a MN ,log a M −log a N =log a M N,log a M n =nlog a M . 8.D【分析】根据函数在[0,1)上的解析式以及f (x )+f (x +1)=1,将ab,a,2a 的范围利用表达式化到[0,1)上代入计算即可得出结果.【详解】由a =log 32,b =log 213=log 23−1=−log 23可得ab =log 32⋅(−log 23)=−1,所以f (ab )=f (−1),由f (x )+f (x +1)=1可得f (−1)+f (0)=1, 即f (−1)=1−f (0)=1−(30−0)=0,所以f (ab )=f (−1)=0; 易知log 31=0<a =log 32<log 33=1,可得a ∈[0,1), 所以f (a )=3a −a =3log 32−log 32=2−log 32;显然f (2a )=f (2log 32)=f (log 34)=f (log 33+log 343)=f (1+log 343), 又f (x )+f (x +1)=1可得f (1+log 343)=1−f (log 343);显然0<log 343<1,所以f (1+log 343)=1−f (log 343)=1−(3log 343−log 343)=1−43+log 343=−13+log 34−1=−43+log 34; 可得f (ab )+f (a )+f (2a )=0+2−log 32−43+log 34=23−log 32+2log 32=23+log 32.故选:D 9.BCD【分析】根据图象逐项分析判断.【详解】对于选项A :由图可知:服药2个小时后心率会恢复正常,故A 错误; 对于选项B :服药后初期心率下降速度增大,即药物起效速度会加快,故B 正确; 对于选项C :当t ∈[0,15]时,图象是下降的,所以所服药物约15个小时后失效,故C 正确; 对于选项D :因为心率在正常范围内的时长为22小时,所以欲控制心率在正常范围内,一天需服用该药2次,故D 正确; 故选:BCD. 10.ACD【分析】分别对不等式所对应的方程的判别式进行逐一判断,结合一元二次函数图象即可得出结论.【详解】对于A ,易知方程x 2+6x +11=0的判别式Δ=62−4×11<0, 即对应的整个二次函数图象都在x 轴上方,所以解集为R ,即A 正确; 对于B ,易知方程x 2−3x −3=0的判别式Δ=32+4×3>0, 由对应的二次函数图象可知其解集不可能为R ,即B 错误; 对于C ,易知方程−x 2+x −2=0的判别式Δ=12−4×2<0, 即对应的整个二次函数图象都在x 轴下方,所以解集为R ,即C 正确;对于D ,易知不等式x 2+2√5x +5≥0可化为(x +√5)2≥0,显然该不等式恒成立,即解集为R ,即D 正确; 故选:ACD 11.ACD【分析】对于A ,易知2x −1>0,利用基本不等式即可得x =1时2x +12x−1取到最小值为3,【详解】根据对数运算法则可知1log 2A +1log 3A +1log 4A +⋯+1log 2023A =log A 2+log A 3+log A 4+⋯+log A 2023 =log A (2×3×4×⋯×2023)=log A A =1; 故答案为:1 16.(−1,3)【分析】类比题目构造函数过程,对不等式x 6−2x −3<(2x +3)3−x 2进行整理变形为(x 2)3+x 2<(2x +3)3+(2x +3),由其结果特征,构造函数g (x )=x 3+x ,根据函数单调性,求解不等式.【详解】设g (x )=x 3+x ,易知函数g (x )在R 上是增函数,不等式x 6−2x −3<(2x +3)3−x 2变形为x 6+x 2<(2x +3)3+(2x +3), 即(x 2)3+x 2<(2x +3)3+(2x +3), 即g (x 2)<g (2x +3),所以x 2<2x +3即x 2−2x −3<0, 解得−1<x <3,所以原不等式的解集为(−1,3). 故答案为:(−1,3). 17.(1)73(2)2【分析】(1)根据根式与分数指数幂的转化以及指数的运算性质化简求值即可. (2)根据对数的运算性质化简求值即可【详解】(1)80.25×√24+(6427)13−(−2021)0=234×214+(43)3×13−1=2+43−1=73(2)lg25+lg2⋅lg50+(lg2)2=2lg5+lg2⋅(lg50+lg2)=2(lg5+lg2)=2 18.(1)y =2x(2)当0<a <1时,(−∞,13);当a >1时,(13,+∞)【分析】(1)由指数函数定义和所过点列方程组求出表达式. (2)分别讨论0<a <1和a >1,结合指数函数的单调性求解.【详解】(1)因为函数y =b ⋅a x 是指数函数,且图象经过点(3,8),所以{b =18=a 3,即a =2,b =1, 函数的解析式为y =2x ;(2)a 3x−4>(1a )3=a −3,当0<a <1时,y =a x 为减函数,则3x −4<−3,解得x <13,解集为(−∞,13)当a >1时,y =a x 为增函数,则3x −4>−3,解得x >13,解集为(13,+∞) 19.(1)[−14,2](2)(−∞,0]【分析】(1)由x ∈[2,8],可得log 2x ∈[1,3],利用换元法可转化为求f (t )=t 2−3t +2,t ∈[1,3]的值域,利用二次函数性质可得其值域为[−14,2]; (2)将原不等式转化成t −3+2t ≥m 对于t ∈[2,4]恒成立,利用对勾函数单调性即可得m ≤0.【详解】(1)由对数函数单调性可知,当x ∈[2,8]时,log 2x ∈[1,3],令log 2x =t,t ∈[1,3],即可得f (t )=t 2−3t +2,t ∈[1,3],由二次函数性质可知当t =32时,f (t )min =−14,当t =3时,f (t )max =2; 因此可得当x ∈[2,8]时,该函数的值域为[−14,2]. (2)当x ∈[4,16]时,可得log 2x ∈[2,4],原不等式可化为t 2−3t +2≥mt 对于t ∈[2,4]恒成立,即可得t −3+2t ≥m 对于t ∈[2,4]恒成立,易知函数y =t −3+2t 在t ∈[2,4]上单调递增, 所以y min =2−3+22=0,因此只需y min =0≥m 即可,得m ≤0;即m 的取值范围是(−∞,0].20.(1)6514.0t(2)3.8【分析】(1)根据题意可得v =3ln (1+M 25),令v =16.7运算求解;(2)根据题意可得v =ω⋅ln M+2525,令v =16.7整理可得ln (M +25)=16.7ω+ln25,解不等式ln (M +25)≤ln2000即可得结果.【详解】(1)由题意可得:v =3ln (1+M 25),令v =3ln (1+M 25)=16.7,则M =25(e 16.73−1)≈6514.0(t ),故当火箭飞行速度达到第三宇宙速度(16.7km s ⁄)时,相应的M 为6514.0t.(2)由题意可得:v =ω⋅ln (1+M 25)=ω⋅ln M+2525, 令v =ω⋅lnM+2525=16.7,则ln (M +25)=16.7ω+ln25≤ln2000, ∴ω≥16.7ln2000−ln25=16.8ln80≈3.8,故ω的最小值为3.8.【点睛】方法点睛:函数有关应用题的常见类型及解决问题的一般程序(1)常见类型:与函数有关的应用题,经常涉及物价、路程、产值、环保等实际问题,也可涉及角度、面积、体积、造价的最优化问题;(2)应用函数模型解决实际问题的一般程序读题(文字语言)⇒建模(数学语言)⇒求解(数学应用)⇒反馈(检验作答);(3)解题关键:解答这类问题的关键是确切地建立相关函数解析式,然后应用函数、方程、不等式和导数的有关知识加以综合解答.21.(1)k =1(2)9【分析】(1)利用奇函数性质可求得k =1;(2)由a =3可得f (x )=3x −3−x ,将不等式化简可得(3x −3−x )2+2≥λ⋅(3x −3−x ),利用换元法可得λ≤t +2t ,t ∈[32,809]能成立,利用函数单调性即可得出λ的最大整数取值为λ=9.【详解】(1)根据题意可知f (0)=k −1=0,解得k =1;此时f (x )=a x −a −x ,经检验f (x )满足f (−x )=a −x −a −(−x )=−(a x −a −x )=−f (x ),即f (x )为奇函数,所以k =1.(2)由a =3可得f (x )=3x −3−x ,则不等式f (2x )+2⋅3−2x ≥λ⋅f (x )可化为32x −3−2x +2⋅3−2x ≥λ⋅(3x −3−x ), 即32x +3−2x ≥λ⋅(3x −3−x ),可得(3x −3−x )2+2≥λ⋅(3x −3−x ),易知函数y =3x −3−x 在x ∈[log 32,2]单调递增,令t =3x −3−x ∈[32,809],所以λ≤t +2t ,易知t +2t 在t ∈[32,809]上单调递增,即可知t +2t ∈[176,3281360], 根据题意可知λ≤3281360≈9.11,即可知λ的最大整数取值为λ=9.22.(1)g (x )=log 2x(a >0且a ≠1).(2)(−∞,0](3)9−√5712【分析】(1)根据指、对数函数互为反函数分析求解;(2)根据题意可知y =f (x )+3k −1=a x +3k −1的值域包含(0,+∞),结合指数函数性质分析求解;(3)根据对数函数的真数大于0分析可得0<a <1,根据题意结合对数函数单调性可得a ≤(x −3a )(x −a )≤1a 在[a +2,a +3]上恒成立,结合二次函数性质分析求解. 【详解】(1)由题意可知:g (x )=log a x(a >0且a ≠1),若a =2,则g (x )=log 2x(a >0且a ≠1).(2)若函数y =g [f (x )+3k −1]值域为R ,可知y =f (x )+3k −1=a x +3k −1的值域包含(0,+∞),因为a x >0,则a x +3k −1>3k −1,即y =a x +3k −1的值域为(3k −1,+∞), 可得3k −1≤0,即3k ≤1,解得k ≤0,所以实数k 的取值范围实数k 的取值范围(−∞,0].(3)因为g (x )=log a x(a >0且a ≠1)的定义域为(0,+∞),且x ∈[a +2,a +3], 对于g (1x−a ),可知1x−a >0,成立,对于g (x −3a ),可知(a +2)−3a =2−2a >0,解得0<a <1,。
同余式xk≡a(modp)的解法

同余式xk≡a(modp)的解法
“同余式xk≡a(modp)”是在数论中比较重要的一类问题,问题的关键在于如
何解决这样的模线性方程,即:找X,使得Xk (mod p) = a (mod p)。
其解法可以简单描述如下:首先,要求出Xk(mod p)能构造出1,2,…,p-
1这p-1个不同的余数,其次,求出Xk(mod p)中a(modp)所对应的小数X,这
就是模线性方程的解。
那么,如何求得这些小数X呢?实质上,就是利用中国余数定理,它将形如
X0,X1,...Xr 的r个余数或者余式组合,归纳出一种统一的形式比如说:
X0+X1X+…+XrXr(mod p),其次,又引入了欧几里的按余分解定理,即它可以将
X0+X1X+…+XrXr(mod p)分解为X0+X1X1+…+XrXr(mod p),至此,所求的X就
可以求出了。
由此可知,解决模线性方程的非常依赖中国余数定理和欧几里得按余分解定理,它们构成了一套完整可逆的推导过程,通过它也许可以找到解决模线性方程的解,从而减少更多题目的答案推导时间,变得更加有趣、有趣起来,有利于学习者增强智力。
同余方程x2=a(modp2)的公式解法

同余方程x2=a(modp2)的公式解法
模恰好计算机有着许多简单的求解方法,其中一种求解方法就是求解同余方程。
同余方程是一类常见的代数方程,它是模糊性数学中最有用的工具,广泛应用于密码学和信息安全领域。
特别是对于二次模方程x^2=a(mod p^2),其为特殊的二次模同余方程。
我们可以使用以下步骤来求解二次模方程:
1)首先找出与所要求的模(即p2)互素的质数p1,且p1满足
p1=1(mod 4)
2)设a=b2 mod p2其中b2 mod p2的平方根为b1,那么
b2=b12(mod p2)和b2=b12(mod p1)
3)将幂p1记为P,b12=b1P (mod p2)
4)用欧拉函数找到一个整数x,使得xP=1 (mod p1), 那么假设记b12= c,则c1/x就是x2=a (mod p2)的一个解
上述就是求解同余方程x2=a(modp2)的公式解法,它提供了一种有效的方法来求解二次模方程。
通过求解该同余方程,我们可以实现多种复杂的计算,如生成指定长度的随机整数和求解特定长度密码中所存在的运算问题等。
由此可见,求解同余方程可以大大提高计算机的效率,节省算力,也大大方便了计算机科学家们开展相关研究。
因此,在计算机科学领域,求解同余方程扮演着重要的角色。
第四章 同余方程

第一节 同余方程的基本概念
因此,第一个结论可由第四章第一节定理 1得出。 若同余方程(2)有解x0 ,则存在y0 ,使得x0 与y0 是方程(3)的解,此时,方程(3)的全部解是
m t x x0 (a , m ) t Z. a y y t 0 (a , m )
则x0必是同余方程
g(x) 0 (mod m) 或 h(x) 0 (mod m)
的解. 证明 留做习题。 下面,我们来研究一次同余方程的解。
第一节 同余方程的基本概念
定理2 设a,b是整数, a 0 (mod m). 则同余方
程
ax b (mod m) (2)
有解的充要条件是(a, m)b。若有解,则恰有d
第一节 同余方程的基本概念
例2 解同余方程
325x 20 (mod 161)
解 同余方程(6)即是 3x 20 (mod 161)。 解同余方程 161y 20 (mod 3), 2y 1 (mod 3), 得到y 2 (mod 3),因此方程(6)的解是 x 20 2 161 = 114 (mod 161)。
例4 解同余方程6x 7 (mod 23)。 解 由例3,依次得到
6x 7 (mod 23) 5x 73 2 (mod 23) 3x 24 8 (mod 23) 2x 8(7) 10 (mod 23) x 5 (mod 23)。
第一节 同余方程的基本概念
x 0, x 0 m d , x0 2m d , , x0 (d 1)m d
第一节 同余方程的基本概念
闵嗣鹤、严士健,初等数论第三章习题解答

第三章 同余§1习题(P53)1. 证明定理2及性质庚、壬 01定理2 若11(mod )k k A B m αααα≡(mod )i i x y m ≡ ,1,2,,i k =则1111k k kk A x x αααααα≡∑ 1111(mod )k k kk B y y m αααααα∑证:由(mod )i i x y m ≡ ⇒戊(mod )ii ii x y m αα≡11kkx x αα⇒≡戊11(mod )k k y y m αα111kk k A x x αααα⇒≡ 戊111(mod )k kk B y y m αααα1111kk kkA x x αααααα⇒∑≡ 丁1111(mod )k k kk B y y m αααααα∑02庚证:(i )(mod )a b m ≡∵ 由P48定理1m a b km ka kb ⇒−⇒−,0(mod )km ak bk mk >⇒≡ (ii )设1a a d =,1b b d =,1m m d =0m >∵,100d m >⇒>(mod )a b m ≡∵ 111()m a b dm d a b ⇒−⇒−111111(mod )(mod a b mm a b a b m d d d⇒−⇒≡⇒≡2. 设正整数101010nn a a a a =+++ 010i a <-,试证11/a 的充要条件是011(1)ni i i a =−∑。
证:由101(mod 11)10(1)(mod 11)i i ≡−⇒≡−10(1)(mod 11)10(1)(mod 11)nni iii i i i i i i a a a a ==⇒≡−⇒≡−∑∑01110(1)nnii i i i i a a ==⇒−−∑∑于是11a 011(1)ni i i a =⇔−∑3. 找出整数能被37,101整除的判别条件来。
01 由10001(mod 37)≡ 及1010001000n n a a a a =+++ ,01000i a <-,由上面证明之方法得3737ni i a a =⇔∑02 由1001(mod 101)≡− 及10100100n n a a a a =+++ 0100i a <- 由上面证明之方法可得:101101(1)ni i i a a =⇔−∑4. 证明3264121+证:由7640251(mod 641)=×≡− 及4456252(mod 641)−=−≡3272577252122252(25)∴+≡×−×=−742173212(525)2(5)(521)≡−×−≡×−×+32173(521)(25)1≡×+≡×= 3(1)10(mod 641)≡−+≡3264121∴+5. 若a 是任一单数,则221(mod 2)nn a +≡(1)n . 证明:当n =1时,322/1a − 2(21)14(1)k k k +−=+∵ 假定2221nn a +−,则有1222222211()1(1)(1)n nn n na a a a a +⋅−=−=−=−+由2221nn a +−,221na +(∵a 是单数,∴21na +是双数)∴1321n n a a ++−,即1221(mod 2)n n a ++≡6. 应用检查因数的方法求出下列各数的标准分解式(i )1535625 (ii )1158066 解:(i )由215356252561425252457=×=×由3245718+++=,324573819391=×=× 由91713=×43153562553713∴=⋅⋅⋅(ii )由311586627+++++=,11580663386022=×33862221++++=,3860223128674=×由7128674546−+=,128674718382=×718382364−+=,1838272626=×262621313213101=×=×× 22115806637131012∴=⋅⋅⋅⋅§2习题(P57)1. 证明s t x u p v −=+,u =0,1,…,1s t p −−,v =0,1,…,1t p −,t s -,是模s p 的一个 完全剩余系。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
The Trivial Solution to the High Degree Congruence x n≡a(mod p) in (p)Shenghui SuSchool of Information EngineeringUniversity of Science & Technology BeijingBeijing 100083 P. R. Chinasheenway@AbstractThis paper gives the definition of the trivial solution to the high degree congruence x n≡a(mod p), presents one sufficient and necessary condition for the congruence to havesolutions, derives and proves one new judgment condition complementarily. Elaboratestwo methods for computing the trivial solution to the congruence in determinatepolynomial time, argues non-trivial solutions to the congruence can not be obtainedcyclically from the trivial solution, infers and demonstrates the two new methods forseeking the trivial solution. In summary, resolves the root existing problem and the trivialroot computing problem for x n≡a (mod p) with an arbitrary n. At last, points out thateven through a probabilistic polynomial time algorithm, seeking a specific non-trivialsolution is still infeasible when the number of the solutions is large enough.Keywords: High degree congruence, Cryptosystem, Trivial solution, Polynomial timealgorithm.1 IntroductionThe discrete logarithm problem and the root finding problem are two closely related problems in computational number theory.The discrete logarithm problem, shortly the DLP, is to seek an exponent n such that x n≡a (mod u), given the triple <x, a, u>. This problem is intractable because no polynomial time algorithm for it has been found yet [1]. The famous public-key cryptosystem ElGamal is built on this hardness [2].The root finding problem is to seek a root x such that x n≡a (mod u), given the triple <n, a, u>. This problem is slightly easier than the DLP since there are polynomial time algorithms for it, provided u is a prime power. However, when φ(u) is unknown, even seeking square roots is equivalent to the integer factorization hardness on which the security of RSA is based [3], where φ(u) is the Euler’s phi function which represents the number of those positive integers coprime to and less than u.When u is equal to a prime p (if p is a prime power, the discussion is similar), the polynomial time algorithms for solving x n≡a (mod p) may be partitioned into the probabilistic type [4] and the determinate type. The former is employed for any random solution to x n≡a (mod p), and the latter is employed only for the trivial solution to x n≡a (mod p) if it exists.[Definition 1] Under some special conditions, a solution to the high degree congruence x n≡a (mod p) can be found in determinate polynomial time. This solution may be written as a to a certain power modulo p, and hence, is called the trivial solution or the trivial root.Through this paper, unless otherwise specified, n≥ 3, p is an odd prime, and a≤p or pł a, that is, a is notexactly divisible by p. The sign ‘gcd’ denotes the greatest common divisor.In the paper, we present theorem 1, derive and prove corollary 1.1. They serve as the discriminants for solutions to the congruence x n≡a (mod p) to exist. We expound theorem 2 and 3, infer and prove corollary 3.1, 3.2, 3.3 and 3.4. By theorem 2, 3, corollary 3.3 and 3.4, we can find out the trivial solution to x n≡a (mod p) in determinate polynomial time.2 Sufficient and Necessary Conditions for the Congruence x n≡a (mod p) to Have SolutionsTo judge whether the congruence x n≡a (mod p) has solutions is much easier than to seek its solutions. [Theorem 1] If n | (p – 1), the sufficient and necessary condition for the congruence x n≡a (mod p) to have solutions isa (p – 1) / n≡ 1 (mod p),and the number of the solutions is n when they exist.The proof of theorem 1 is referred to subsection 1.6.8 of chapter 1 of [5].[Corollary 1.1] If nł (p – 1), let k = gcd(n, p –1), m = n / k and µ satisfy µm≡ 1 (mod (p –1) / k), then x n≡a (mod p) is equivalent tox k≡aµ (mod p),that is, the two congruences have the same set of solutions. Furthermore, the sufficient and necessary condition for either congruence to have solutions is a (p – 1) / k≡ 1 (mod p).Proof: The original congruence may be written as (x k)m≡a (mod p). Let µm = 1 + h (p – 1) / k, where h is a positive integer.Suppose that (x k)m≡a (mod p) and x k≡aµ (mod p) both have solutions, and the solution sets are respectively X1 and X2.∀x1∈X1, then (x1k)m≡a (mod p), and by the properties [6] of the group* p, we have(x1k)mµ≡aµ (mod p)(x1k)1 + h (p – 1) / k≡aµ (mod p)x1k≡aµ (mod p),which indicates that x1∈X2. Considering the arbitrariness of x1, X1 ⊆ X2. Note that by the Fermat’s little theorem [1], x1p – 1≡ 1 (mod p).Clearly, substituting x1k≡aµ (mod p) for x1k of (x1k)m≡a (mod p) yields aµm≡a (mod p).∀x2∈X2, then x2k≡aµ (mod p), and by the properties [6] of the group* p, we have(x2k)m≡aµm≡a (mod p), namely x2n≡a (mod p),which indicates that x2∈X1. Considering the arbitrariness of x2, X2 ⊆ X1.Therefore, X1 = X2. Since both the sets are finite, |X1| =|X2|.By theorem 1, the sufficient and necessary condition for the congruence x k≡aµ (mod p) have solutions is (aµ) (p – 1) / k≡ 1 (mod p), and the number of the solutions is k when they exist.Due to aµm≡a (mod p), there is aµm – 1≡ 1 (mod p).Let the order of a be d, then d | (µm – 1), i.e. µm – 1 = v d, where v is a positive integer.Presume that gcd(d, µ) = t≥ 2, and let d = t i and µ = t j. Substituting t i and t j for µ and d above yieldst j m – 1 = v t it (j m – v i) = 1.Because (j m – v i) is an integer and t≥ 2, the equation t (j m – v i) = 1 brings on a contradiction, which means that gcd(d, µ) = t < 2, namely gcd(d, µ) = 1.By the group theories [6], aµ and a have the same order, and thus d | ((p – 1) / k). That is, the sufficient and necessary condition for x k≡aµ (mod p) to have solutions may be expressed as a (p – 1) / k≡ 1 (mod p). Furthermore, |X1| =|X2| = k when the solutions exist, or |X1| =|X2| = 0 when there exists no solution.3 Seeking Polynomial Time Solutions to the Congruence x n≡a (mod p)[Theorem 2] [7] For the congruence x n≡a (mod p), if gcd(n, p – 1) = 1, every a has just one n-th root modulo p. Especially, let µ be the multiplicative inverse of n modulo p – 1, then aµ mod p is one n-th root to the congruence.It is known from corollary 1.1 that x n≡a (mod p) has just a solution when gcd(n, p –1) = 1.[Theorem 3] [7] For the congruence x n≡a (mod p), if n | (p – 1) and gcd(n, (p – 1) / n) = 1, then when a is one n-th power residue modulo p, aµ mod p is one n-th root, where µ is the multiplicative inverse of n modulo (p – 1) / n.The proofs of theorem 2 and 3 are similar, and thus only theorem 3 is proved below.In fact, what we need to do is only to verify (aµ)n≡a (mod p).If p | a, then there is a≡ 0 (mod p), and obviously (aµ)n≡a (mod p) holds.If pł a, according to µn≡ 1 (mod (p – 1) / n), there always exists a positive integer h such thatµn = 1 + h (p – 1) / n.Because a is known to have one n-th root x, a can be described as a≡x n (mod p). Then(aµ)n≡ ((x n)µ)n≡x nµn≡x n (1 + h (p – 1) / n )≡x n x h (p – 1)≡x n(x p – 1)h≡x n1h≡x n≡a (mod p).In the above deduction, the Fermat’s little theorem x p – 1≡ 1 (mod p) [1] is employed.Notice that theorem 3 cannot ensure that aµ mod p is exactly one n-th root of a─ thus it is necessary to verify at last whether aµ to the n-th power is equals to a or not, and furthermore, aµ is only the trivial root to x n≡a (mod p).From theorem 3, we can infer the following four important corollaries.[Corollary 3.1] For the congruence x n≡a (mod p), if n | (p – 1), gcd(n, (p – 1) / n) = 1, and let d be the order of the trivial solution aµ with µn≡ 1 (mod (p – 1) / n), then gcd(n, d) = 1 and d | ((p – 1) / n). Proof: Substituting aµ for x of x n≡a (mod p) yields (aµ)n≡a (mod p), namelyaµn – 1≡ 1 (mod p).Let ď be the order of a∈* p, then aď≡ 1 (mod p).Presume that gcd(n, ď ) = k≥ 2. Let n = i k and ď = j k, thenµn – 1 = hďµi k – 1 = h j k(µi – h j) k = 1.Because (µi – h j) is an integer and k≥ 2, the equation (µi – h j) k = 1 brings on a contradiction, which means that gcd(n, ď ) = k < 2, namely gcd(n, ď ) = 1.Additionally, due to gcd(µ, (p – 1) / n) = 1 and ď | (p – 1), gcd(µ, ď ) = 1. According to the group theory [6], the order d of aµ mod p is equal to the order ď of a, and so gcd(n, d) = 1. Further, considering d | (p – 1), we have d | ((p – 1) / n).[Corollary 3.2] For the congruence x n≡a (mod p), if n | (p – 1) and gcd(n, (p – 1) / n) = 1, then the congruence has only a trivial root, and any non-trivial root cannot be obtained cyclically from the trivial root aµ, where µ satisfies µn≡ 1 (mod (p – 1) / n).Proof: It is known from the proof of corollary 3.1 that a and aµ∈* p have the same order.We might as well let the order of a be d.Assume that another solution y can be written as a kµ mod p with k < d. Substituting it for x of the congruence yields(a k µ)n≡a (mod p)a kµn – 1≡ 1 (mod p),which indicates that kµn – 1 = ĥd, namely k (µn) – ĥd = 1.It is known from corollary 3.1 that gcd(µn, (p –1) / n) = 1 and d | ((p –1) / n), so gcd(µn, d) = 1.By the greatest common divisor theorem [5], there exists only one positive integer k < d which meets the equation k (µn) – ĥd = 1.Additionally, due to aµn – 1≡ 1 (mod p), µn – 1 =h d, i.e. µn – h d = 1 holds. Comparing the two difference equalities gives k = 1 and ĥ = h.Hence, there exists only the trivial root, and cycling the trivial root cannot generate any non-trivial root. [Corollary 3.3] For the congruence x n≡a (mod p), let k = gcd(n, p – 1) with 1 < k < n. If gcd(k, (p – 1) / k) = 1 and a is one n-th power residue modulo p, then aµη is the trivial root, where µ satisfies µ (n / k) ≡ 1 (mod (p – 1) / k) and η doesηk≡ 1 (mod (p – 1) / k).Proof: Let n = k m, then the congruence can be expressed as(x k)m≡a (mod p).Due to gcd(m, (p – 1) / k) = 1, it is known from corollary 1.1 that the congruencex k≡aµ (mod p),where µ satisfies µm≡ 1 (mod (p – 1) / k), and the congruence (x k)m≡a (mod p) have the same set and number of solutions.Since a is one n-th power residue modulo p, by corollary 1.1, aµ must be one k-th power residue. Further, by gcd(k, (p – 1) / k) = 1 and theorem 3, we can infer that the trivial root to the original congruence is aµη mod p, where η satisfies ηk≡ 1 (mod (p – 1) / k). Thus, corollary 3.3 holds.[Corollary 3.4] For the congruence x n≡a (mod p), if n2 | (p – 1) and gcd(n2, (p – 1) / n2) = 1, then when the trivial root exists in 1 / n probability, it is aµ n mod p, where µmeets µn 2≡ 1 (mod (p – 1) / n2). Proof: Assume that a is one n-th power residue modulo p.Due to x n≡a (mod p), raising either side of the congruence to the n-th power yieldsx n2≡a n (mod p).Let the solution sets of x n≡a (mod p) and x n2≡a n (mod p) be respectively X1 and X2, then |X1| = n, |X2| = n2. Because every solution satisfying the original congruence x n≡a (mod p) is sure to satisfy the new congruence x n2≡a n (mod p), we have X1 ⊂ X2.Additionally, thanks to gcd(n2, (p – 1) / n2) = 1, there exists µ which satisfies µn2≡ 1 (mod (p – 1) / n2). By theorem 3, aµ n mod p is the trivial root to the new congruence.Further, we can verify whether aµ n mod p is the trivial root to x n≡a (mod p) or not.If is, the condition a (p – 1) / n2≡ 1 (mod p) holds affirmatively. That is, a belongs to the sub-group of order (p –1) / n2. Naturally, a also belongs to the sub-group of order (p –1) / n. Therefore, the probability that aµn is the trivial root to x n≡a (mod p) is((p – 1) / n2) / ((p – 1) / n) = n / n2 = 1 / n.If not, then x n≡a (mod p) has only non-trivial roots, or no solution at all when a is not a true residue.4 ConclusionsFor the congruence x n≡a (mod p) with the true residue a, if gcd(n, p – 1) = 1, it has only a solution, namely the trivial solution. If n | (p – 1) and gcd(n, (p – 1) / n) = 1, it has the trivial solution and non-trivial solutions. If n | (p – 1) and gcd(n, (p – 1) / n) > 1, it has likely only non-trivial solutions. By theorem 2, 3, corollary 3.3 and 3.4, we can figure out the trivial solution in determinate polynomial time. Through the algorithm in section 1.6 of [4], we can seek random non-trivial solutions in probabilistic polynomial time. However, to seek a specific non-trivial solution is still infeasible in polynomial time when the number of the solutions is large enough.We can not obtain the non-trivial solutions from the trivial solution, that is, a non-trivial solution can not be expressed with the trivial solution.Let g be a generator, then gφ(p)≡ 1 (mod p), and all the elements of * p may be described as g1, g2, …, gφ(p). Further, let a≡gτ (a) (mod p), and x≡gτ (x) (mod p), then all the solutions to x n≡a (mod p) can be written as x≡gτ (x) + k (p – 1) / gcd(p – 1, n) (mod p), where k = 0, 1, …, (n – 1) [5]. However, to compute τ(a) is the DLP, that is, to seek the general expression of the solutions to x n≡a (mod p) is intractable.References[1] A. J. Menezes, P. van Oorschot, and S. Vanstone, Handbook of Applied Cryptography, London: CRC Press, 1997,ch.2.[2] T. ElGamal, “A Public-key Cryptosystem and a Signature Scheme Based on Discrete Logarithms,” IEEETransactions on Information Theory, v. 31, n. 4, 1985, pp. 469-472.[3] R. L. Rivest, A. Shamir and L. M. Adleman, “A Method for Obtaining Digital Signatures and Public-keyCryptosystems,” Communications of the ACM, 21(2), 1978, pp.120-126.[4] Henri Cohen, A Course in Computational Algebraic Number Theory, Berlin: Springer-Verlag, 2000, ch. 1, 3.[5] Song Y. Yan, Number Theory for Computing, 2nd ed., Berlin: Springer-Verlag, 2002, ch. 1.[6] Thomas W. Hungerford, Algebra, New York: Springer-Verlag, 2004, ch. 1–3.[7] Paul Garrett, Making, Breaking Codes: An Introduction to Cryptology, New Jersey: Prentice-Hall, 2001, ch. 12.About AuthorShenghui Su, professor and doctorand, obtained bachelor and master degrees respectively from National University of Defence Technology and Peking University, and now is engaged in researches on cryptographic algorithms, information securities and decision support systems.。
