量子力学习题课2
量子力学教程课后习题答案
量子力学教程课后习题答案量子力学习题及解答第一章量子理论基础1．1 由黑体辐射公式导出维恩位移定律：能量密度极大值所对应的波长与温度T成反比，即T=b（常量）；并近似计算b的数值，准确到二位有效数字。
解根据普朗克的黑体辐射公式，（1）以及，（2），（3）有这里的的物理意义是黑体内波长介于λ与λ+dλ之间的辐射能量密度。
本题关注的是λ取何值时，取得极大值，因此，就得要求对λ的一阶导数为零，由此可求得相应的λ的值，记作。
但要注意的是，还需要验证对λ的二阶导数在处的取值是否小于零，如果小于零，那么前面求得的就是要求的，具体如下：如果令x= ，则上述方程为这是一个超越方程。
首先，易知此方程有解：x=0，但经过验证，此解是平庸的；另外的一个解可以通过逐步近似法或者数值计算法获得：x=4.97，经过验证，此解正是所要求的，这样则有把x以及三个物理常量代入到上式便知这便是维恩位移定律。
据此，我们知识物体温度升高的话，辐射的能量分布的峰值向较短波长方面移动，这样便会根据热物体（如遥远星体）的发光颜色来判定温度的高低。
1．2 在0K附近，钠的价电子能量约为3eV，求其德布罗意波长。
解根据德布罗意波粒二象性的关系，可知E=h，如果所考虑的粒子是非相对论性的电子（），那么如果我们考察的是相对性的光子，那么E=pc 注意到本题所考虑的钠的价电子的动能仅为3eV，远远小于电子的质量与光速平方的乘积，即，因此利用非相对论性的电子的能量——动量关系式，这样，便有在这里，利用了以及最后，对作一点讨论，从上式可以看出，当粒子的质量越大时，这个粒子的波长就越短，因而这个粒子的波动性较弱，而粒子性较强；同样的，当粒子的动能越大时，这个粒子的波长就越短，因而这个粒子的波动性较弱，而粒子性较强，由于宏观世界的物体质量普遍很大，因而波动性极弱，显现出来的都是粒子性，这种波粒二象性，从某种子意义来说，只有在微观世界才能显现。
1．3 氦原子的动能是（k为玻耳兹曼常数），求T=1K时，氦原子的德布罗意波长。
量子力学(二)习题参考答案
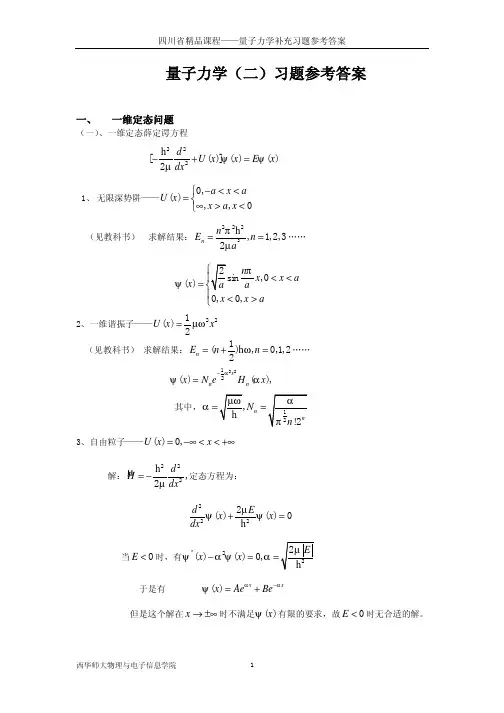
2µ (U1 − E ) h2 2µ E h2
ψ 2 '' ( x) + k 2ψ 2 ( x ) = 0, k =
西华师大物理与电子信息学院
4
四川省精品课程——量子力学补充习题参考答案
ψ 3'' ( x) − β 2ψ 3 ( x) = 0, β =
其解分别为：
2µ (U 2 − E ) h2
ψ 1 ( x) = A1eα x + B1e −α x ψ 2 ( x) = C sin(kx + δ ) ψ 3 ( x ) = A2e β x + B2 e− β x
2
2
⑤
而透射系数
⑥
2） 、当 E＜U0 时，有ψ 2 '' ( x ) − k3 2ψ 2 ( x ) = 0 ， k3 = 其解为：ψ 2 ( x ) = Ce
− k3 x
+ De k3 x = Ce − k3 x （ψ 2 有限条件）
⑦
以下可以重复前面的求解过程。 不过， 为了简单我们亦可以在前面得到的结果⑤中做代 换 k2 ＝i k3 ，得到
由（18）式， （16） 、 （17）变成 或由 （19） 式， （16） 、 （17） 变成
（20）或（21）式就是讲义上习题 2．7 的结果。 a) 将 δ = 0 代入ψ 2 ( x) 中有：ψ 2 ( x) = C sin kx 由连续性条件：ψ 2 ( a) = ψ 3 ( a ) → C sin( ka ) = B2 e − β a
ψ m (ϕ ) =
除了 m=0 的态之外， E m 圴是二重简并的。 5、梯形式——— U ( x ) =
0, x < 0 U 0 , x > 0
量子力学——第二章作业参考答案
+
⎛ ⎜ ⎝
∂ψ ∂t
*
Vψ
+
∂ψ ∂t
Vψ
*
⎞ ⎟
，
⎠
（2）
ψ 、ψ * 满足薛定谔方程
i
∂ψ ∂t
=
⎛ ⎜ ⎝
−
2
2m
∇2
+V
⎞⎟ψ ⎠
，
−i
∂ψ * ∂t
=
⎛ ⎜
−
⎝
2
∇2 2m
+V
⎞⎟ψ * ， ⎠
（3） （4）
用 ∂ψ * 乘以（3）式加上用 ∂ψ 乘以（4）式得
∂t
∂t
∂ψ ∂t
Vψ *
dt
s
通常 < 2V2 >≠ 0 ，也就是说在整个区域找到粒子的概率随时间发生变化，概率守恒破缺；
即使 < 2V2 >= 0 ，由（8）式知概率守恒也存在局域破缺除非V2 (r ) = 0
（b）证明如下： 由（a）得
d dt
∫∫∫ d 3rψ τ
*ψ
=
−∫∫ dsi s
j
+
∫∫∫ d 3rψ τ
*
2V2 ψ
第二章作业参考答案
(曾谨言著《量子力学教程》(第二版) 习题 1 P24-P26)
∫ 1.1 证明：（a）能量的平均值 < E >= d 3rψ *Hˆψ ，
哈密顿量 Hˆ = Pˆ 2 2m +V (r ) ，波函数ψ =ψ (r ,t ) ，（1）式变为
（1）
∫ < E >=
d 3r
⎛ ⎜ψ
*
Pˆ 2
+
∂ψ ∂t
外国教材量子力学概论2ndedition课后练习题含答案
Introduction to Quantum MechanicsOverviewQuantum Mechanics is a branch of Physics that describes the behavior of matter and energy at a microscopic level. This discipline has had a significant impact on modern science and technology, and its principles have been applied to the development of various fields, such as computing, cryptography and medicine. The study of Quantum Mechanics requires a basic understanding of the principles of Mathematics and Physics. The m of this document is to provide an introduction to Quantum Mechanics and to provide a set of practice exercises with answers that will allow students to test their knowledge and understanding of the subject.Fundamental PrinciplesThe fundamental principles of Quantum Mechanics are based on the concept of a wave-particle duality, which means that particles can behave as both waves and particles simultaneously. The behavior of particles at the microscopic level is probabilistic, and it is described by a wave function. A wave function is a complex function that describes the probability of finding a particle at a givenlocation. The square of the amplitude of the wave function gives the probability density of finding the particle at that point in space. The wave function can be used to calculate various physical quantities, such as the position, momentum and energy of a particle.Operators and ObservablesIn Quantum Mechanics, physical quantities are represented by operators. An operator is a mathematical function that acts on a wave function and generates a new wave function as a result. Operators are used to represent physical observables, such as the position, momentum and energy of a particle. The eigenvalues of an operator correspond to the possible results of a measurement of the corresponding observable. The eigenvectors of an operator correspond to the possible states of a particle. The state of a particle is described by a linear combination of its eigenvectors, which is called a superposition.Schrödinger EquationThe Schrödinger Equation is a mathematical equation that describes the time evolution of a wave function. It is based on the principle of conservation of energy, and it representsthe motion of a quantum system in terms of its wave function. The equation is given by:$$\\hat{H}\\Psi=E\\Psi$$where $\\hat{H}$ is the Hamiltonian operator, $\\Psi$ is the wave function, and E is the energy of the system. The Schrödinger Equation is the foundation of Quantum Mechanics, and it is used to calculate various physical properties of a particle, such as its energy and momentum.Practice Exercises1.Calculate the wave function for a particle that isin a 1D box of length L.–Answer: The wave function for a particle in a 1D box is given by:$$\\Psi(x)=\\sqrt{\\frac{2}{L}}\\sin{\\frac{n\\pi x}{L}}$$where n is a positive integer.2.Derive the time-dependent Schrödinger Equation.–Answer: The time-dependent SchrödingerEquation is given by:$$i\\hbar\\frac{\\partial\\Psi}{\\partialt}=\\hat{H}\\Psi$$3.Calculate the momentum operator for a particle in1D.–Answer: The momentum operator for a particle in 1D is given by:$$\\hat{p_x}=-i\\hbar\\frac{\\partial}{\\partial x}$$4.What is the uncertnty principle?–Answer: The uncertnty principle is afundamental principle of Quantum Mechanics thatstates that the position and momentum of a particlecannot be measured simultaneously with arbitraryprecision. Mathematically, it is given by: $$\\Delta x\\Delta p_x\\geq\\frac{\\hbar}{2}$$5.Calculate the energy of a particle in a 1D box oflength L with quantum number n.–Answer: The energy of a particle in a 1D box is given by:$$E_n=\\frac{n^2\\pi^2\\hbar^2}{2mL^2}$$ConclusionQuantum Mechanics is a fascinating and challenging fieldof study that has provided a deeper understanding of the behavior of matter and energy at the microscopic level. Theprinciples of Quantum Mechanics have been applied to various fields of study, including computing, cryptography and medicine, and they have contributed to significant advances in these fields. The practice exercises provided in this document are intended as a tool for students to test their knowledge and understanding of Quantum Mechanics. By solving these exercises, students will gn a deeper understanding of the fundamental principles of Quantum Mechanics and strengthen their problem-solving skills in this exciting field of study.。
量子力学习题课
习 题 课例：求下列各粒子相关的de Broglie 波的波长： （1） 能量为100ev 的自由电子； （2） 能量为0.1ev 的自由中子； （3） 能量为0.1ev,m=1g 的质点；（4） T =1K 时，具有动能E=KT3/2(K Bolzman 常数)的氮原子。
解：在V «C 的情况下，mE h p h mE P 2//,2===λ (1) E=100ev=1.6×10-10erg,因为m=9×10-28g h=6.6×10-27erg.s 所以 2.1102.1106.11092106.68102827=⨯=⨯⨯⨯⨯⨯=----cm λÅ（2） E=0.1ev = 1.6×10-13erg, m=1.675×10-24g9.0109.0106.11068.12106.68132427=⨯=⨯⨯⨯⨯⨯=----cm λÅ(3) E=0.1ev = 1.610-13erg, m=1g121327107.1106.12106.6---⨯=⨯⨯⨯=λ Å（4） E =1.5×1.38×10-16erg, m=4×1.67×10-24=6.68×10-24g6.121038.15.11068.62106.6162427=⨯⨯⨯⨯⨯⨯=---λ Å星体光谱的成分与绝对黑体光谱十分相近。
估计黑体表面温度的方法之一，在于确定黑体光谱中辐射强度λU 的最大值所对应的波长。
太阳的这2个波长等于0.5μm,北极星的波长等于0.35μm,天狼星的等于0.29μm 。
试计算这些星体的温度。
解：根据黑体辐射的Plank 公式，物体在温度为T 的热平衡态下，辐射的能谱密度λU 与热辐射频率ν之间的关系为)1(1)/exp(833-=kT h C h U ννπλ将（1）式中的辐射频率ν换成辐射波长λ表示，)2(1)/exp(181,52-=-==kT hc hCU d Cd Cλλπλλνλνλ）改写成将（由d λU /d λ=0,可得λU （max ）所应的波长λ：)3(]1)/[exp()/exp(50)1)/exp(18(5-==-=kT hc kT kT hc hc kT hc hc d d d dU λλλλλπλλλ得若令x=hc/λkT, 则（3）改写成 15-=x xe xeXe x =5e x -5, e -x +(x/5)-1=0, e -x =1-(x/5) (4) (4)式是一个超越方程，做图法求解，⎪⎩⎪⎨⎧=-=)6()5(51xe y x y 曲线（5）和（6）的交点的横坐标便是所求的解，如图： 求得 x=4.97, (7) 既hc/kT (8) 或T λmax=hc/4.97k=b (9)次式为Wien 位移公式（wien formula ），其b=hc/4.97k=2.9×10-3m.℃ 由（9）式可估计星体表面温度如下： （a ） 太 阳：λmax=0.55μm=0.55×10-6m, T max =b/λmax=5.27×103℃（b ） 北极星：λmax=0.35μm=0.35×10-6m, T max =b/λmax=8.27×103℃ （c ） 天狼星：λmax =0.29μm=0.29×10-6m, T max =b/λmax=10×103℃例：如果我们观测一个大小为2.5Å的物体，可用的光子的最小能量是多少？若把光子改成电子呢？解：为了发生散射，光波的波长必须与所观测物体的大小同数量级或更小。
量子力学习题以及课堂练习答案
一.微观粒子的波粒二象性1、在温度下T=0k 附近，钠的价电子能量约为3电子伏特，求其德布罗意波长。
2、求与下列各粒子相关的德布罗意波长。
（1）能量为100电子伏特的自由电子；（2）能量为0.1电子伏特的自由中子；（3）能量为0.1电子伏特，质量为1克的自由粒子； （4）温度T=1k 时，具有动能kTE 23=的氦原子，其中k 为玻尔兹曼常数。
3、若电子和中子的德布罗意波长等于oA 1，试求它们的速度、动量和动能。
4、两个光子在一定条件下可以转化为正负电子对，如果两电子的能量相等，问要实现这种转化，光子的波长最大是多少？5、设一电子为电势差U 所加速，最后打在靶上，若电子的动能转化为一光子，求当这光子相应的光波波长分别为5000oA （可见光）o A 1（x 射线），oA001.0（γ射线）时，加速电子所需的电势差各是多少？二.波函数与薛定谔方程1、设粒子的归一化波函数为 ),,(z y x ϕ，求 （1）在),(dx xx +范围内找到粒子的几率；（2）在),(21y y 范围内找到粒子的几率； （3）在),(21x x 及),(21z z 范围内找到粒子的几率。
2、设粒子的归一化波函数为 ),,(ϕθψr ，求：（1）在球壳),(dr rr +内找到粒子的几率；（2）在),(ϕθ方向的立体角Ωd 内找到粒子的几率； 3、下列波函数所描述的状态是否为定态？为什么？（1）Eti ix Eti ix ex ex t x---+=ψ)()(),(211ψψ[])()(21x x ψψ≠（2）tE i t E i ex ex t x 21)()(),(2--+=ψψψ)(21E E ≠（3）EtiEti ex ex t x)()(),(3ψψ+=ψ-4、对于一维粒子，设 xp i o e xπψ21)0,(=，求 ),(t x ψ。
5、证明在定态中，几率密度和几率流密度均与时间无关。
6、由下列两个定态波函数计算几率流密度。
陈鄂生《量子力学教程》习题答案
第二章 力学量算符2.1 证明空间反演算符ˆˆ(()())x x ψψ∏∏=-是厄米算符。
指出在什么条件下，ˆd p i dx =- 是厄米算符。
2.2 动量在径向方向的分量定义为1ˆˆˆ2r p r r ⎛⎫=⋅+⋅ ⎪⎝⎭r r p p ，求出ˆr p 在球坐标系中的表示式。
2.3 证明[][]ˆˆˆ,()();,()()ˆx x x x p f x i f x x f p i f p x p∂∂=-=∂∂ 2.4 设算符ˆA满足条件2ˆ1A =，证明ˆˆcos sin i A e i A ααα=+，其中α为实常数. 2.5 设算符ˆˆˆˆˆˆˆ,1KLM LM ML =-=,又设ϕ为ˆK 的本征矢,相应本征值为λ.求证ˆˆu L v M ϕϕ≡≡和也是ˆK 的本征矢,并求出相应的本征值.2.6 粒子作一维运动,2ˆˆ()2p H V x μ=+,定态波函数为n ,ˆ,1,2,3,n H n E n n == (1)证明ˆnm n pm a n x m =,并求出系数nm a . (2)利用(1)式推导求和公式()22222ˆn m nEE n x m m p m μ-=∑ (3)证明()222n m n EE n x m μ-=∑ 2.7 设ˆF为厄米算符,证明在能量表象中下式成立:()21ˆˆˆ,,2n m nk n E E F k F F H k ⎡⎤⎡⎤-=⎣⎦⎣⎦∑ 2.8 已知(,)lm Y θϕ是2ˆˆZL L 和的共同本征函数，本征值分别为2(1)l l m + 和。
令ˆˆˆx y L L L ±=±. (1)证明ˆ(,)lm L Y θϕ±仍是2ˆˆZ L L 和的共同本征函数,求出他们的本征值.(2)推导公式1ˆ(,)(,)lm lm L Y Y θϕθϕ±± 2.9 证明ˆˆ11ˆˆˆˆˆˆˆˆˆˆˆ,,,,,,2!3!A A e Be B A B A A B A A A B -⎡⎤⎡⎤⎡⎤⎡⎤⎡⎤⎡⎤=++++⎢⎥⎣⎦⎣⎦⎣⎦⎣⎦⎣⎦⎣⎦2.10 设算符ˆA 与ˆB 同它们的对易关系式ˆˆ,A B ⎡⎤⎣⎦都对易，证明1ˆˆˆˆˆ,,n n A B nB A B -⎡⎤⎡⎤=⎣⎦⎣⎦ 1122ˆˆˆˆˆˆ,,ˆˆˆˆˆˆA B A B A B A B A B A B e e e e e e e ⎡⎤⎡⎤-+++⎣⎦⎣⎦==或2.11 设ˆL 为轨道角动量算符。
量子力学教程（二版）习题答案
第一章 绪论1.1．由黑体辐射公式导出维恩位移定律：C m b bTm3109.2 ,×´==-l 。
证明：由普朗克黑体辐射公式：由普朗克黑体辐射公式：n n p nr n nd ec hd kTh 11833-=， 及ln c=、l ln d c d 2-=得1185-=kThcehc l l l p r ，令kT hc x l =，再由0=l r l d d ，得l .所满足的超越方程为所满足的超越方程为15-=x x e xe用图解法求得97.4=x ，即得97.4=kT hc m l ，将数据代入求得C m 109.2 ,03×´==-b b T ml 1.2．在0K 附近，钠的价电子能量约为3eV ,求de Broglie 波长. 解：010A 7.09m 1009.72=´»==-mEh p h l # 1.3. 氦原子的动能为kT E 23=，求K T 1=时氦原子的de Broglie 波长。
波长。
解：010A 63.12m 1063.1232=´»===-mkT h mE h p h l其中kg 1066.1003.427-´´=m ，123K J 1038.1--×´=k # 1.4利用玻尔—索末菲量子化条件，求：利用玻尔—索末菲量子化条件，求： （1）一维谐振子的能量。
）一维谐振子的能量。
（2）在均匀磁场中作圆周运动的电子的轨道半径。
）在均匀磁场中作圆周运动的电子的轨道半径。
已知外磁场T 10=B ，玻尔磁子123T J 10923.0--×´=B m ，求动能的量子化间隔E D ，并与K 4=T 及K 100=T 的热运动能量相比较。
的热运动能量相比较。
解：（1）方法1：谐振子的能量222212q p E mw m +=可以化为()12222222=÷÷øöççèæ+mw m E q Ep的平面运动，轨道为椭圆，两半轴分别为22,2mw m Eb E a ==，相空间面积为，相空间面积为,2,1,0,2=====òn nh EE ab pdq nw pp 所以，能量 ,2,1,0,==n nh E n方法2：一维谐振子的运动方程为02=+¢¢q q w ，其解为，其解为()j w +=t A q sin速度为速度为 ()j w w +=¢t A q c o s ，动量为()j w mw m +=¢=t A q p cos ，则相积分为，则相积分为 ()()nh T A dt t A dt t A pdq T T ==++=+=òòò2)cos 1(2cos 220220222mw j w mw j w mw ， ,2,1,0=n nmw nh T nh A E ===222， ,2,1,0=n （2）设磁场垂直于电子运动方向，受洛仑兹力作用作匀速圆周运动。
量子力学习题课
数 (x) 4 sin xcos2 x 描写，求粒子能量的可能值和相应
aa
a
的几率。
8.在一维无限深势阱中运动的粒子，势阱的宽度为a，如 果粒子的状态由波函数 (x) Ax(a x) 描写，A为归一
化常数，求粒子能量的几率分布和能量的平均值。
9.荷电q的谐振子，受到外电场 的作用，V (x) 1 m 2 x2 q x
量子力学习题课（1）
，x 0
1.一粒子在一维势场 U (x) 0， 0 x a 中运动，求粒子的 能级和对应的波函数。 ，x a
2.证明（2.6-14）式中的归一化常数是
A 1 a
3.求一维谐振子处在激发态时几率最大的位置。
4.设
(x)
1 2x2
Ae 2
2
求能量本征值和本征函数。
10.设粒子（能量 E 0 ）从左入射，碰到下列势阱 0 （图），求阱壁处的反射系数。
-U
(为常数 )
，求A
=
？
5.试证明 (x)
1 2x2
e2
(2
3x
3
3
x)是线性谐振子的波函数，并
3
求此波函数对应的能量。
6.一维运动的粒子处在
Axe x ,
(x)
0,
其中 0 ，
求：粒子动量的几率分布函数。
当x 0 当x 0
的状态，
7.设粒子在一维无限深阱中运动，如果粒子的状态由波函
量子力学中的量子力学力学量守恒练习题及
量子力学中的量子力学力学量守恒练习题及解答量子力学中的量子力学力学量守恒练习题及解答量子力学作为现代物理学的重要分支，探讨了微观粒子的行为和性质。
其中，量子力学力学量守恒是研究的重要内容之一。
本文将为您提供一些量子力学中力学量守恒方面的练习题和相应的解答，帮助您加深对该领域的理解。
练习题一：考虑一个质量为m的自由粒子，其波函数表示为ψ(x,t)。
在不考虑外力作用的情况下，该自由粒子的波函数满足薛定谔方程iħ∂ψ(x,t)/∂t = -ħ²/(2m)∂²ψ(x,t)/∂x²。
请证明在这种情况下，动量算符p = -iħ∂/∂x是守恒量。
解答一：为了证明动量算符p是守恒量，我们需要证明其对时间的导数为零，即d⟨p⟩/dt = 0。
根据动量算符的定义，p = -iħ∂/∂x。
因此，我们需要计算其对时间的导数，即d⟨p⟩/dt。
首先，计算出算符 p 在波函数ψ(x,t) 上的期望值⟨p⟩，即⟨p⟩= ∫ψ*(x,t)·p·ψ(x,t) dx将动量算符 p 的定义代入上式，得到⟨p⟩ = -iħ∫ψ*(x,t)·(∂/∂x)·ψ(x,t) dx接下来，对上式右侧的积分进行分部积分。
令f(x) = ψ*(x,t)，g'(x) = (∂/∂x)·ψ(x,t)。
根据分部积分的公式，我们有∫f(x)·g'(x) dx = [f(x)·g(x)] - ∫f'(x)·g(x) dx将 f(x) 和 g'(x) 代入上式，得到∫ψ*(x,t)·(∂/∂x)·ψ(x,t) dx = [ψ*(x,t)·∂ψ(x,t)/∂x] -∫(∂/∂x)·[ψ*(x,t)·∂ψ(x,t)/∂x] dx将以上结果代入到⟨p⟩的表达式中，得到⟨p⟩ = -iħ·[ψ*(x,t)·∂ψ(x,t)/∂x] + iħ∫(∂/∂x)·[ψ*(x,t)·∂ψ(x,t)/∂x] dx我们可以观察到，上式右侧的两项是一个净的积分。
第一章 量子力学基础习题20111019
E
0 a 0
a
Hdx
(4 E1 9 E 2 ) 1 (4 E1 9 E 2 ) 2 49 13 dx
1 h2 4h 2 (4 9 ) 2 2 13 8m a 8m a 5h 2 13m a2
习题
1.49-51 处于状态的一维箱中的粒子的动量和动量平方有无 确定值，若有，求确定值；若没有，求平均值。
基本知识
5.态叠加原理
若Ψ1、 Ψ2、••• Ψi、••• Ψn为某一微观体系的可能状态，由 它们线性组合也是该体系的可能状态。
c1 1 c2 2 cn n ci i
i 1
n
式中Ci是任意常数，数值的大小反应了Ψi对Ψ的贡献 的大小。
ˆ A i ai i a
基本知识
4.Schrodinger方程
在量子力学中，决定微观体系运动状态的是定态Schrodinger 方程:
ˆ H (r) E (r )
2 2 [ V (r )] (r ) E (r ) 2m
实质是能量算符的本征方程。 解法：一维箱 精确求解 三维箱 分离变量法 平面刚性转子
Ci ai
2
C
2 i
ci ai
i 1
n
2
基本知识
三.简单应用
1.一维箱中粒子
n 2 x sin x x a a
h2 2 E nx 8m a2
2.三维箱中粒子 三个方向一维箱的叠加。
n y nx 8 n ( xyz) sin x sin y sin z z abc a b c
n 解： ＝ 83 sin n x x sin yx y sin nz z a a a a
量子力学(第二版)答案 苏汝铿 第二章课后答案2.10-2#12
1 4 2 x 2 2 2 2 m 2 x 2 , 2 2m 2m
2 2 2
m
, x
Vn n x Vdx
2
N n H n e
2 2 2
2m
d
又由厄秘多项式 H n 的递推式 2 H n H 2 n1 2nH n1
2
a 0
2
于是 A 30a
5
下面考虑粒子能量的概率分布和能量的平均值：
2 n sin x 对一个无限深的势阱，其能级为 En 的波函数为： n x a a
0
a
x 是各个能级的波函数的叠加，故设
x cn n x cn
n n
2 n sin x a a
2 D exp 2m U 0 E b a
U1
又
a
U2
b
D R 1 ，D 是贯穿系数，R 是反射系数，
所以 D 是粒子透过势垒的概率，而由概率论知识， 粒子连续贯穿两个方势垒的概率等于 分别单独贯穿这两个势垒的概率之积。 于是
E
图 2
a b
a
0
5 2 E x dx 2 ma
2
2.10
设两个方势垒的形状分别是：
0 U x U1
x 0 , 0 x a
0 U x U 2
a x b, x c b x c
U
E
图1
求粒子连续贯穿两个方势垒的贯穿系数。 解：如图（1） ，对低能入射，贯穿一个方势垒的贯穿系数为
曾谨言《量子力学教程》(第3版)配套题库【课后习题-一维势场中的粒子】
第2章一维势场中的粒子2.1 设粒子限制在矩形匣子中运动，即求粒子的能量本征值和本征波函数，如a=b=c，讨论能级的简并度。
解：在匣子内即其中采用直角坐标系，方程的解可以分离变量。
再考虑到边条件能量本征函数可表示为再考虑到可以求出粒子的能量本征值为而归一化的能量本征函数为对于方匣子a=b=c，能级的简并度为满足条件的正整数解的个数。
【参阅：《量子力学》，卷Ⅱ，PP．420～421，练习2】2.2 设粒子处于一维无限深方势阱中，证明处于能量本征态的粒子，讨论的情况，并与经典力学计算结果比较．证明：设粒子处于第n个本征态，其本征函数为在经典情况下，在区域（0，a）中粒子处于dx范围中的概率为，所以当，量子力学的结果与经典力学计算值一致．2.3 设粒子处于一维无限深方势阱中处于基态（n=1，见2．2节式（12）），求粒子的动量分布．解：基态波函数测量粒子的动量的概率分布为。
【参阅：《量子力学》，卷I，PP．87～88，练习4和练习5】2.4 设粒子处于无限深方势阱中，粒子波函数为A为归一化常数，（a）求A；（b）求测得粒子处于能量本征态的概率特别是作图，比较与曲线．从来说明两条曲线非常相似，即几乎与基态完全相同，解：（a）根据归一化条件可得，所以（b）用展开，，只当n=1，3，5，…时，才不为0，特别是，非常接近于1．考虑到归一化条件，，可知概率几乎为0，即与概率几乎完全相同．（c）图2-1（实线）（虚线）2.5 同上题，设粒子处于基态（n=1），．设t=0时刻阱宽突然变为2a，粒子波函数来不及改变，即试问：对于加宽了的无限深方势阱是否还是能量本征态?求测得粒子处于能量本征值的概率．解：对于加宽了的无限深方势阱，能量本征值和能量本征态分别为可见不再是它的能量本征态，．由于势阱突然变宽，粒子波函数和能量来不及改变，粒子能量仍保持为，而可以按展开，经过计算可得所以粒子处于，即能量仍为的概率为．2.6 设粒子（能量E＞0）从左入射，碰到下图所示的势阱，求透射系数与反射系数．图2-2解：考虑上图所示势阱中粒子，可证明粒子碰到侧壁的透射系数为其中反射系数为其中不难验证概率守恒关系式2.7 利用Hermite多项式的递推关系（附录A3，式（13）），证明谐振子波函数满足下列关系：并由此证明，在态下证明：已知所以利用本征函数的正交性，可得．。
量子力学第一、二章习题课
第一部分 状态与波函数
1、量子力学中用波函数描写微观体系的状态 、 2、 态叠加原理：设 ψ 1 ,ψ 2 ,ψ 3 ,⋯ψ n ⋯是体系的可能状态，那么， 、 态叠加原理： 是体系的可能状态，那么， 这些态的线性叠加 状态。 状态。
ψ = ∑ cnψ n
n
也是体系的一个可能
3、波函数随时间的变化规律由薛定谔方程给出： 、波函数随时间的变化规律由薛定谔方程给出：
j= iℏ (ψ∇ψ ∗ −ψ ∗∇ψ ) 2µ
ρ = ψ ∗ψ 与几率密度
∂ρ +∇⋅ j = 0 满足连续性方程 ∂t
第二部分 一维运动
1、一维无限深势阱 、 本征值 本征函数
0 V ( x) = ∞ 0<x<a x ≤ 0或者x ≥ a
n 2π 2 ℏ 2 En = , n = 1,2,3, ⋯ 2 2 µa
dm p = dp mc 2
dm p υg = c = =υ dp m
2
的物体， 三、如果我们需要观测一个大小为 2.5 Α 的物体，可用的光子的 最小能量是多少？若把光子改为电子呢？（ ？（要 最小能量是多少？若把光子改为电子呢？（要） 解：为了发生散射，光波的波长必须与所观测物体的大小同 为了发生散射， 数量级或者更小。 数量级或者更小。故在本问题中能够采用的光的最大波长 ，这样相应的光子的最小能量为： 这样相应的光子的最小能量为：
ℏ2 2 ∂Ψ ih =− ∇ Ψ + V (r , t )Ψ ∂t 2µ
当势场
V (r ) 不显含时间
t
时，其解是定态解
Ψ (r , t ) = ψ (r )e −iEt / ℏ
ψ (r ) 满足定态薛定谔方程
量子力学科恩课后习题答案
量子力学科恩课后习题答案量子力学科恩课后习题答案量子力学是现代物理学的重要分支，研究微观粒子的行为和性质。
科恩是一位著名的量子力学教授，他的课程内容深入浅出，为学生提供了丰富的知识和习题。
本文将为读者解答一些量子力学科恩课后习题，帮助读者更好地理解量子力学的概念和原理。
1. 习题：描述薛定谔方程的基本原理是什么？答案：薛定谔方程是量子力学的基本方程，描述了微观粒子的波函数随时间的演化。
薛定谔方程的基本原理是根据哈密顿量和波函数的关系，通过解薛定谔方程得到粒子的波函数随时间的变化规律。
薛定谔方程的一般形式为：iħ∂ψ/∂t = Hψ，其中i是虚数单位，ħ是约化普朗克常数，∂/∂t表示对时间的偏导数，H是系统的哈密顿量。
2. 习题：什么是量子力学中的叠加态？答案：量子力学中的叠加态是指粒子处于多个可能的状态之间的叠加状态。
根据量子力学的叠加原理，一个粒子可以同时处于多个状态之间，直到被测量时才会坍缩到其中一个确定的状态。
叠加态可以用数学上的线性组合表示，例如：|ψ⟩= α|0⟩+ β|1⟩，其中|0⟩和|1⟩是两个可能的状态，α和β是复数系数。
3. 习题：什么是量子纠缠？答案：量子纠缠是指两个或多个粒子之间存在一种特殊的关联关系，无论它们之间有多远，它们的状态仍然是相互依赖的。
量子纠缠是量子力学的重要特性，它违背了经典物理学的局域性原理。
例如，当两个纠缠态的粒子之间进行测量时，它们的结果是彼此相关的，无论它们之间的距离有多远。
4. 习题：什么是量子隧穿效应？答案：量子隧穿效应是指粒子在经典力学下无法通过势垒的情况下，通过量子力学的特性，在势垒中出现的概率。
根据量子力学的波粒二象性，粒子不仅可以被视为粒子，还可以被视为波动。
当粒子遇到势垒时，根据波动性质，它有一定的概率穿过势垒并出现在势垒的另一侧。
这种现象在纳米技术和核物理学中具有重要应用。
5. 习题：什么是量子力学中的不确定性原理？答案：量子力学中的不确定性原理是由海森堡提出的，指出在某些物理量的测量中，无法同时准确测量这些物理量的取值。
陈鄂生《量子力学教程》习题答案第二章力学量算符
陈鄂生《量子力学教程》习题答案第二章_力学量算符陈鄂生《量子力学教程》习题答案第二章_力学量算符含答案第一节算符理论基础1.量子力学中的基本假设包括哪些？它们各自的物理意义是什么？答：量子力学中的基本假设包括：(1) 波函数假设：用波函数Ψ(x)描述微观粒子的运动状态，波函数的模的平方表示找到粒子在空间中某一点的概率。
(2) 物理量算符假设：每个物理量都对应一个算符，而对应的测量值是算符的本征值。
(3) 波函数演化假设：波函数随时间的演化遵循薛定谔方程。
(4) 基态能量假设：系统的最低能量对应于基态，且能量是量子化的。
这些基本假设反映了量子力学的基本原理和规律。
2.什么是算符的本征值和本征函数？答：算符的本征值是指对应于某个物理量的算符的一个特征值，它代表了该物理量的一个可能的测量结果。
本征函数是对应于某个物理量的算符的一个特征函数，它表示的是该物理量的一个可能的状态。
3.什么是算符的厄米性？答：算符的厄米性是指一个算符与其共轭转置算符相等。
对于一个算符A，如果满足A†=A，则称该算符是厄米算符。
4.什么是算符的厄米共轭？答：算符的厄米共轭是指将算符的每一项的系数取复共轭得到的新算符。
对于一个算符A，它的厄米共轭算符A†可以通过将A的每一项的系数取复共轭得到。
5.什么是算符的共同本征函数？答：算符的共同本征函数是指对于两个或多个算符A和B，存在一组波函数Ψ(x)使得同时满足AΨ(x)=aΨ(x)和BΨ(x)=bΨ(x)。
其中a和b分别是A和B的本征值。
6.什么是算符的对易性？答：算符的对易性是指两个算符之间的交换顺序不改变它们的结果。
如果两个算符A和B满足[A,B]=AB-BA=0，则称它们对易。
第二节动量算符1.什么是动量算符？它的本征值和本征函数分别是什么？答：动量算符是描述粒子动量的算符，用符号p表示。
动量算符的本征值是粒子的可能动量值，本征函数则是对应于这些可能动量的波函数。
动量算符的本征函数是平面波函数，即Ψp(x)=Nexp(ipx/ħ)，其中N是归一化常数，p是动量的本征值。
曾谨严量子力学习题第二章
= = 用高斯定理: = 中间一式的第一项是零,因为假定满足平方可积条件,因而因此 ,能让能量 平均值 因此令(本征态)则而
得证 # [9]设粒子在势场中运动 (1)证明其能量的平均值是: (1) 其中W是能量密度 (2)证明能量守恒公式
利用积分 ：
写出共轭函数（前一式变号）：
本题也可以用Fresnel积分表示，为此可将（6）式积分改为： 用课本公式得，两者相乘，可得相同的结果。 #
[14]在非定域势中粒子的薛定谔方程式是： （1）
求几率守恒对非定域势的要求。此时，只依赖于波函数在空间一点的几 率波是否存在？
[解]按题意，是要求写出几率守恒的条件，从这个条件寻出应当遵 守的要求。几率守恒的条件是：
（6） 故相速度（物质波的）应当超过光速。 最后找出和的关系，将（1）（2）相除，再运用德氏波假设：
， （7） # [6]（1）试用Fermat最小光程原理导出光的折射定律
（2）光的波动论的拥护者曾向光的微粒论者提出下述非难： 如认为光是粒子，则其运动遵守最小作用量原理 认为则这将导得下 述折射定律 这明显违反实验事实，即使考虑相对论效应，则对自由粒子：仍就 成立，E是粒子能量，从一种媒质到另一种媒质E仍不变，仍有，你怎 样解决矛盾？
（解）甲法：光线在同一均匀媒质中依直线传播，因此自定点A到定 点B的路径是两段直线：光程
设A，B到界面距离是a,b(都是常量)有 又AB沿界面的投影c也是常数，因而，存在约束条件：
（2） 求(1)的变分,而将,看作能独立变化的,有以下极值条件 (3) 再求（2）的变分
(3)与(4)消去和得 (5)
(1)
量子力学教程(第二版)周世勋习题解答
A2 2 T A2 2T pdq A 0 cos t dt 2 0 (1 cost )dt 2 nh ， n 0,1,2,
2 2 T 2
A2 2 nh E nh ， n 0,1,2, 2 T
6
v 2 v （2）设磁场垂直于电子运动方向，受洛仑兹力作用作匀速圆周运动。由 evB ，得 R R eB
《量子力学教程》 习题解答
1
《量子力学教程》
习题解答说明
• 为了满足量子力学教学和学生自学的需要，完 善精品课程建设，我们编写了周世勋先生编写 的《量子力学教程》的课后习题解答。本解答 共分七章，其中第六章为选学内容。 • 第一章 第二章 第三章 第四章 第五章 第六章 第七章
2
目录
• • • • • • • 第一章 绪论 第二章 波函数和薛定谔方程 第三章 力学量的算符表示 第四章 态和力学量的表象 第五章 微扰理论 第六章 弹性散射 第七章 自旋和全同粒子
2mE 2
2 2 2 En n 2ma 2
(n 1,2,3,) 可见 E 是量子化的。
对应于 E n 的归一化的定态波函数为
2 n i Ent sin xe , 0 x a n ( x, t ) a a 0, x a, x a
2
11
2.3
一粒子在一维势场
，x 0 U ( x) 0， 0 x a ，x a
中运动，求粒子的能级和对应的波函数。 解： U ( x)与t 无关，是定态问题。其定态 S—方程
2 d 2 ( x) U ( x) ( x) E ( x) 2m dx 2
可见， J 2与r 反向。表示向内(即向原点) 传播的球面波。
量子力学周世勋第二版课后习题解答第2章
2.1.证明在定态中，几率流与时间无关。
证：对于定态，可令)]r ()r ()r ()r ([m 2i ]e )r (e )r (e )r (e )r ([m2i )(m 2i J e)r ( )t (f )r ()t r (**Et iEt i **Et i Et i **Etiψψψψψψψψψψψψψψψ∇-∇=∇-∇=∇-∇===-----）（）（，可见t J 与无关。
2.2 由下列定态波函数计算几率流密度： ikr ikr e re r -==1)2( 1)1(21ψψ 从所得结果说明1ψ表示向外传播的球面波，2ψ表示向内(即向原点) 传播的球面波。
解：分量只有和r J J 21在球坐标中 ϕθθϕθ∂∂+∂∂+∂∂=∇s i n r 1e r 1e r r 0 r mrk r mr k r r ik r r r ik r r m i r e rr e r e r r e r m i mi J ikr ikr ikr ikr30202201*1*111 )]11(1)11(1[2 )]1(1)1(1[2 )(2 )1(==+----=∂∂-∂∂=∇-∇=--ψψψψ r J 1与同向。
表示向外传播的球面波。
rmr k r mr k r r ik r r r ik r r m i r e r r e r e r r e r m i mi J ikr ikr ikr ikr3020220*2*222 )]11(1)11(1[2 )]1(1)1(1[2 )(2 )2(-=-=---+-=∂∂-∂∂=∇-∇=--ψψψψ可见，r J与2反向。
表示向内(即向原点) 传播的球面波。
补充：设ikxex =)(ψ，粒子的位置几率分布如何？这个波函数能否归一化？∞==⎰⎰∞∞dx dx ψψ*∴波函数不能按1)(2=⎰∞dx x ψ方式归一化。
其相对位置几率分布函数为12==ψω表示粒子在空间各处出现的几率相同。
- 1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。
(
)
(
)
l=F l + + iF l− ， （2）证明任何一个算符均可分解为 F l+ = 1 F l+F l† l− = 1 F l−F l† ， 其中 F ，F 2 2i l l F + 与 F − 均为厄米算符.
l† F − =
(
)
−1 l † l l− F −F = F 2i
(
)
(
)
因此,这样利用上述方法,任意一个算符都可以表示为:
R > 0 是一个给定的半径，
2 2 2 ˆ ψ = − = ∇ 2ψ = = π ψ H 2μ 2 μR 2
,
0 ≤ r ≤ R, r > R.
ˆ 的本征函数,本征值是 这表明 ψ 是 H
= 2π 2 2 μR 2
.
（注：这个问题叫做球形无限深势阱，在核物理中有用.） 【讨论题 2】一条长度为 l 的细杆（它的质量不计）两端各有一个质 量为 μ 的粒子，绕着杆的固定中点在一个平面内转动，这个系统的 哈密顿算符是
2
2 ˆ = − = ∇ 2 .归一化常数 C 的值不影 只需要考虑 0 ≤ r ≤ R 的区间和 H 2μ
响答案，不必算出.
体系的哈密顿算符为 2 ˆ2 ˆ = L =−= H 2I 2I
⎡ 1 ∂ ⎛ 1 ∂ ⎞ ∂2 ⎤ sin θ + ⎟ ⎢ sin θ ∂θ ⎜ . ∂θ ⎠ sin 2 θ ∂ϕ 2 ⎥ ⎝ ⎣ ⎦
2
,
其中 ϕ 为杆的转动角.
求它的能级和对应的归一化能量本征函数.各能级的简并度都是多 大？ 【解答】 转动惯量为 I = μ l 2 的刚性转子，它的能量的经典表示式是
H= L2 ， （ L 是角动量） ， 2I
1 2
在球坐标中，有
1 ∂ ⎛ ∂ ⎞ 1 ∂ ∂ 1 ∂2 ∇ = 2 ⎜ r2 ⎟ + 2 ( sin θ ) + 2 2 ∂θ r sin θ ∂ϕ 2 r ∂r ⎝ ∂r ⎠ r sin θ ∂θ
（10）
l 为 Hermite（厄米）算符.即若 F l 为厄米算符. l+ = F l ，则称 F 则称 F
【证明】 l 1 ll ll l= 1 l l−B ll A ) ，有 （1）令 C = AB + B A ， D ( AB 2 2i † 1 l† † l† l† l+B ll l† = 1 l l AB A = (B C A +A B ) 2 2 1 ll ll l = B A + AB = C 2
ii
i
对于定轴转动， 即转子被约束在某个平面内的圆周上运动， 可以设 θ = π .
2
由上式可得到平面转子的哈密顿算符：
2 2 ˆ =−= d H 2 I dϕ 2
.
波函数也与 θ 无关，只取决于 Ylm = Pl m (θ ) Φ (ϕ ) 中的 Φ m (ϕ ) ，即
ψ (ϕ ) = Φ m (ϕ ) =
（7） （8）
众所周知，自由振子的能级 1⎞ ( 0) ⎛ En = ⎜ n + ⎟ =ω ， n = 0,1, 2," 2⎠ ⎝ 因此
iv
（9）
iii
1⎞ 1 1⎞ q 2ε 2 ⎛ ⎛ 2 En = ⎜ n + ⎟ =ω − mω 2 x0 = ⎜ n + ⎟ =ω − 2⎠ 2 2⎠ 2mω 2 ⎝ ⎝ ϕn ( x ) = ψ n ( x − x0 )
†
=
1 2 l 2 l + pr r2
l (b) pr = −i= ⎜
r, l p ⎤ = i=; (c) ⎡ ⎣ r⎦
2 2 2 l (d) pr = −= ⎜ ∂r 2 + r ∂r ⎟ = −= r 2 ∂r r ∂r ; ⎝ ⎠ 2
⎛ ∂2
2 ∂ ⎞
1 ∂
∂
2 1 2 l2 l p = l + pr . (e) r2
(m = 0, ±1, ±2,")
1 imϕ e . 2π
或者， ψ 0 (ϕ ) =
1 2π
， ψ m (ϕ ) =
1 2π
1
π
sin mϕ 或
1
π
cos mϕ (m = 1, 2, ") .
所以 E0 不简并， Em (m ≠ 0) 二重简并. 基态波函数是 ψ 0 = .
2 l p' 1 1 2 l H= + mω 2 x '2 − mω 2 x0 （6） 2m 2 2 l 和H m 的差别在于变量由 x 换成 x ' ，并 比较式（1）和（6） ，易见 H 0
(
)
† † † l l) A −l A B
(
)
(
)
（2）对于一个给定的算符 F ，可以将其分成两部分
l
1 l l† 1 l l† l l F−F 其中 F + = F + F ， F − = 2i 2 有 1 l† l l+ l† F +F =F F + = ，
2
(
)
l=F l + + iF l− F
∫ψ
∗
∗ lφ )dτ = ( F (F ∫ lψ ) φ dτ ,
l x ( x ) = e − i x0 p = = e D 【说明】如引入坐标平移算符 0 l D x ( x )ψ ( x ) = ψ ( x − x ) 它对波函数的作用是
0 0
− x0
d dx
(
)
则 H 和 H 0 的本征函数可用平移算符联系起来： l x ( x )ψ ( x ) ϕ ( x) =ψ ( x − x ) = D
并利用单值条件，也能得到波函数及能量的本征值. 因而平面转子的能级及波函数分别为：
Em
( 0)
（3） （4） （5）
如作坐标平移，令
m2 =2 , = 2I
( 0) ψm (ϕ ) =
ห้องสมุดไป่ตู้
x ' = x − x0
由于
d d l p = −i = = − i = =l p' dx dx ' H 可以表示成
习题讨论课二
第一部分 波函数与薛定鄂方程
重点掌握的模型: [1] [2] [3] [4] 自由粒子模型 无限深势阱模型 定点转动模型及定轴转动模型 线性谐振子模型 所以
∇ 2ψ = =
1 ∂ ⎛ 2 ∂ ⎞ ⎧C ⎛ π r ⎞⎫ ⎜r ⎟ ⎨ sin ⎜ ⎟⎬ r 2 ∂r ⎝ ∂r ⎠ ⎩ r ⎝ R ⎠⎭
【证明】
G G ∂ G 1 ∂ G r 2 1 ∂ ∇ ⋅ = ∇ = + + e e e r θ ϕ (注: ; ) ∂r r ∂θ r sin θ ∂ϕ r r
r, l p ⎤ = rl pr − l p r r = −i = ⎜ r （c） ⎡ ⎣ r⎦ ∂ ∂ ∂r ⎞ ⎛ ∂ ⎞ ⎛ ∂ + 1 − r − 1 ⎟ = −i = ⎜ r − r − ⎟ = i = ∂r ∂r ∂r ⎠ ⎝ ∂r ⎠ ⎝ ∂r
G G + G + G + + † 1 ⎡⎛ r G ⎞ ⎛ G r ⎞ ⎤ 1 ⎡ G + ⎛ r ⎞ ⎛ r ⎞ G ⎤ l p p p p = ⋅ + ⋅ = ⋅ + ⎢⎜ ⎢ ⎟ ⎜ ⎟ ⎥ ⎜ ⎟ ⎜ ⎟ ⋅ p⎥ （a） r r r 2⎢ 2 ⎝ ⎠ ⎝ ⎠ ⎝r⎠ ⎝r⎠ ⎥ ⎢ ⎥ ⎣ ⎦ ⎣ ⎦ G G 1⎛ G r r G⎞ l = ⎜ p ⋅ + ⋅ p ⎟ = pr 2⎝ r r ⎠
2 2 ˆ =− = ∂ H 2 μ l ∂ϕ 2
c 是归一化因子.势能函数是
(0 ≤ r ≤ R) ⎧0, U (r ) = ⎨ +∞ , (r > R) ⎩ ˆ 的本征函数？如果是，对应的 问：这个波函数是不是哈密顿算符 H
本征值是多大？ 【解答】 单粒子的哈密顿算符：
ˆ = − = ∇2 + U ， H 2μ
C ∂ ⎛ 2⎧π ⎛πr ⎞ 1 ⎛ π r ⎞⎫ ⎞ ⎜ r ⎨ cos ⎜ ⎟ − 2 sin ⎜ ⎟⎬ ⎟ r 2 ∂r ⎝ ⎩ Rr ⎝ R ⎠ r ⎝ R ⎠⎭ ⎠ C ∂ ⎡π r ⎛πr ⎞ ⎛ π r ⎞⎤ = 2 ⎢ cos ⎜ ⎟ − sin ⎜ ⎟⎥ r ∂r ⎣ R ⎝ R⎠ ⎝ R ⎠⎦
1 imϕ e ， 2π
将哈密顿算符作用于波函数，得能量的本征值：
Em = m2 =2 , 2I (m = 0, ±1, ±2,")
能量本征函数记为ψ n ( x ) ，能级记为 En ，外加均匀电场 ε 使振子额外受力 f = qε ，总能量算符变成 2 l p 1 l + mω 2 x 2 − qε x H= （2） 2m 2 新的能级记为 En ，本征函数记为 ϕn ( x ) .
∫
+∞
−∞
C 2 e −α | x| dx = 2 C 2 ∫ e −2α x dx =
0
2
+∞
C2
α
,
C= α
.
⎛ i G G⎞ exp ⎜ p ⋅ r ⎟ ⎝= ⎠
（2）动量的本征函数
G (r ) = ψp
G
1
( 2π = )
3/ 2
将粒子的波函数按动量的本征函数展开，有 ψ ( x ) = ∫ c ( p )ψ p ( x ) d p . 动量测量的概率振幅是
l=F l + + iF l− F
【讨论题 5】定义径向动量算符
l 满足下述关系 定义：对于任意波函数ψ , φ ,若算符 F