第十二章琼斯模型教程文件

合集下载

琼斯模型第二部分(涉及琼斯模型的假设检验及修正)资料

琼斯模型第二部分5.假设检验5.1应计利润模型1节4中显示的描述性统计值能够解释成支持盈余管理假设，但是只有在假定当年与前年应计利润的差值只是源于操作性应计利润的变化值的时候才成立，因为非操作性应计利润假定每期不变。

2为了释放该假设，我使用下面的用于总应计利润的期望模型来控制公司经济环境的变化：TAit/Ait-1=αi[1/Ait-1]+β1i[ΔREVit/Ait-1]+ β2i[PPEit/Ait-1]+ξit （2）其中：TAit=第t年公司i总应计利润；ΔREVit＝公司i第t年收入减去第t－1年收入的差值；PPEit＝第t年公司i原始不动产、厂房和设备；Ait-1＝公司i第t－1年的总资产；ξit＝公司i第t年的误差项；i＝1，…，N公司编号（N＝23）；t＝1，…，Ti，公司i估计期间内的年份的编号（Ti范围是14年到32年）。

1为了提供一个较长时间序列的观察值，本节报告的盈余管理假设检验中使用的总应计利润的定义是由节4中使用的定义修改过的。

2本节中使用的总应计利润的测度值没有调整长期债务到期部分和应付所得税，因为有些早期的观察值在Comprstat tapes中找不到。

3剔除长期债务到期部分和应付所得税调整部分使每个公司观察值的平均数量从12.9提高到了25.2.1在方程（2）中，原始不动产、厂房、设备和收入的变化值被包含在模型中用来控制条件变化引起的非操作性应计利润的变化值。

2总应计利润（TA）包括运营资本帐户的变化值，比如应收账款、存货和应付账款，它们在某种程度上取决于收入的变化值。

3 收入被用来控制公司的经济环境，因为他们是经理人员操纵之前公司运营的客观测度变量，但是它并不是完全外生的变量。

4原始不动产、厂房、设备被包括进来控制总应计利润中与非操作性折旧费用相关的部分。

5原始不动产、厂房、设备被包括在期望模型中，而不是这些帐户的变化值被包含在模型中，因为总折旧费用（与折旧费用变化值对照）被包含在了总应计利润的测度变量中。

Earns Management during Import Relief investigations 琼斯模型

2
Role of Accounting Numbers in Foreign Trade Regulation
2.2 Use of Accounting Numbers by the ITC
Petitions file for import relief investigations. Managers do have incentives to manage earnings in order to increase the apparent injury to the firm, and thereby, to the industry.
Tests of hypothesis
• Year 0 : the year the investigation completes • Year -1 : the prior year the investigation completes
Earnings Management During Import Relief Investigations
LIMITATIONS 3. Managers may rely on cost allocations rather than accruals to manage earnings for the product line investigated by the ITC. 4. The power of the tests may not be sufficient to detect managers’ incomedecreasing accounting choices.
Earnings Management During Import Relief Investigations

范经华使用的琼斯模型公式

范经华使用的琼斯模型公式
传统的琼斯类模型。

这一类模型的基本思想认为非操纵性应计主要受两个因素影响，销售收入变动和固定资产水平，主张通过回归方法来分离总应计中的操纵性成分，具体包括基本琼斯模型、修正琼斯模型等。

扩展的琼斯类模型。

该类模型的提出者认为传统的琼斯模型忽略了许多与盈余管理无关，但会影响正常应计利润水平的其他变量，会导致计量偏差，主张把其它控制变量引入传统的琼斯模型，从而形成无形资产琼斯模型、现金流量琼斯模型、前瞻性修正琼斯模型和收益匹配琼斯模型等。

非线性的琼斯模型。

这类模型的提出者认为会计谨慎性对于利得和损失确认的不对称性，导致应计和企业业绩之间存在非线性相关性，而传统以及扩展的琼斯模型均采取的是线性形式，会引起计量偏差。

主张把非线性关系引入传统及扩展的琼斯模型，形成非线性琼斯模型、非线性修正的琼斯模型等。

非琼斯类模型。

文献中虽然以琼斯模型及其扩展模型为主体，但是有些研究者提出其它一些计量方法，主要包括Healy模型、DeAngelo模型和KS模型。

TheFreeWillTheorem

University of Ljubljana FACULTY OF MATHEMATICS AND PHYSICSDepartment of physicsseminar2008/09The Free Will TheoremTimon MEDEmentor:prof.Rudolf PodgornikLjubljana,5Nov2008AbstractI begin with description of quantum entanglement and its relevance to the EPR-type (thought and real)experiments which played a decisive role in rejecting determinism and hidden-variable theories.Then I proceed with deriving the main object of my paper,the recently published Conway and Kochen’s Free Will Theorem(byﬁrst proving the Kochen-Specker paradox, on which it is founded).There it is being shown on the basis of three physical axioms, following from the special theory of relativity and quantum mechanics,that if the choice for a particular type of spin1experiment is not a function of the information accessible to the experimenters,then its outcome is equally not a function of the information accessible to the particles.Therefor the response of the particles can be seen as free.In conclusion I review some signiﬁcant remarks that followed in responses on this arti-cle and mention the relevant points from the subsequent discussion and the philosophical aspects and implications of this theme.Contents1Introduction2 2Entangled states32.1Joint state space for two subsystems (3)2.2Entanglement (3)2.3Singlet state of two spin1particles (5)3EPR paradox and the hidden variable theories63.1EPR paradox (6)3.2Hidden variable theories and no-go theorems (9)3.3Kochen-Specker paradox (10)3.3.1K-S paradox for Peres’33-direction conﬁguration (12)4The Free Will Theorem144.1Introduction to The Free Will Theorem (14)4.2Philosophical thoughts on the question of Free Will (15)4.3Stating the theorem (15)4.4The proof of the Free Will Theorem (17)5Conclusion21 6Appendix241Chapter1IntroductionI would like to begin by presenting some of the reasons that motivated me for choosing this topic and point out some of the goals of this paper.First of all,there was this rather intriguing title,that sounded very promising in a way that allowed me to combine my interest in physical theory(quantum mechanics)and philosophy and to conﬁrm myﬁrm belief that at the forefront of every science they go hand-in-hand with eachother and become inseparable,perhaps even indistinguishable.Besides that I was given an excellent opportunity to learn more about the foundations of the quantum mechanical theory and some of its most controversial conceptual yet unsolved problems that are usually the main reason why this theory strikes most of the people,even physicists,as odd.Even Richard Feynman once remarked:”I think I can safely say that nobody understands quantum mechanics.”The main problem is,that quantum mechanics contrary to our intuition shows that the world is objectivelly random,not determenistic.This is closely linked with the in-famous measurement problem i.e.the problem of the wave function collapse,better known as the paradox of Schrodinger’s cat.Heisenberg’s uncertainty principle(comple-mentarity)seems to lie in the heart of this matter,and not just being a consequence of our imperfect experimental methods.Andﬁnally,how to understand objective random-ness of the single event in connection with entangled states in e.g.EPR-setup,where the result of measurement of a random event on one end is determined by the result of preceding measurement of another random event on other’s end of the experimental setup,Einstein’s relativity of time making it even stranger,because the direction of the inﬂuence is completely(reference)frame dependent.Next I wish to mention two very inﬂuential gedanken-experiments that greatly changed our understanding of physics:Bell inequality and the Kochen-Specker paradox showed a way how to experimentally resolve some age-old philosophical dilemmas.And last but not least,I also wanted to present an excerpt from contemporary debate about the foundations of quantum theory,to show the course it’s taking and to empha-size that this quest is far from over.This paper is mainly based upon the recent work by Conway and Kochen in their2006 article”The Free Will Theorem”and the subsequent replies by Bassi,Ghirardi,Tumulka, Adler,...2Chapter2Entangled states2.1Joint state space for two subsystems[3]The representation of physical states of two independent(noninteracting)quantum sys-tems,labelled A and B,can be considered in two independent Hilbert spaces by choosing their state vectors:|ΨA ∈H Aand|ΨB ∈H BWhen we bring these two systems together and let them interact,the joint state space (the Hilbert space of the composite system)for systems A and B corresponds to the tensor product of H A and H B,denotedH AB=H A⊗H BLet N A be the dimension of H A,and N B the dimension of H B.If{|1 A,|2 A,|3 A, (i)a complete orthonormal basis for H A and{|1 B,|2 B,|3 B,···}is a complete orthonormal basis for H B,then H A⊗H B is the Hilbert space of dimension N AB=N A N B,spanned by the vectors of the form|i A⊗|j B.Hence arbitrary states in H AB have the form|ΨAB =N Ai=1N Bj=1c ij|i A⊗|j BAs long as weﬁx an ordering for the new basis states|i A⊗|j B,the set of N A N B complex coeﬃcients c ij can be used as a vector representation for kets in H AB.2.2Entanglement[4]As a consequence of this mathematical rule for representation of joint states,there exist beside separable i.e.product states of composite system also‘nonfactorizable’states |ΨAB ∈H AB that cannot be expressed as the tensor product of a state|ΨA ∈H A with3a state|ΨB ∈H B.Such states are said to be entangled.For example,let’s consider two three-dimensional(e.g.spin1)systems.Say we have chosen orthonormal bases{|−1 A,|0 A,|1 A}for H A and{|−1 B,|0 B,|1 B}for H B.Then H AB is spanned by the nine states:{|−1 A⊗|−1 B,|−1 A⊗|0 B,|−1 A⊗|1 B,|0 A⊗|−1 B,|0 A⊗|0 B,|0 A⊗|1 B,|1 A⊗|−1 B,|1 A⊗|0 B,|1 A⊗|1 B}Factorizable(non-entangled)states in H AB are all of the form|ΨfacA =(c A−1|−1 A+c A|0 A+c A1|1 A)⊗(c B−1|−1 B+c B|0 B+c B1|1 B)==c A−1c B−1|−1 A⊗|−1 B+c A−1c B|−1 A⊗|0 B+c A−1c B1|−1 A⊗|1 B++c Ac B−1|0 A⊗|−1 B+c Ac B|0 A⊗|0 B+c Ac B1|0 A⊗|1 B++c A1c B−1|1 A⊗|−1 B+c A1c B|1 A⊗|0 B+c A1c B1|1 A⊗|1 BThat is,a certain relationship exists between the coeﬃcients of the nine basis states in H AB.An example of an entangled state,whose coeﬃcients do not exhibit the above relationship,is|ΨAB =1√3|1 A⊗|−1 B+1√3|−1 A⊗|1 B−1√3|0 A⊗|0 B=|ΨA ⊗|ΨB ;|ΨA ∈H A,|ΨB ∈H Bwhich can easily be seen if we look at the equations:c A 1c B−1=1√3=0c A −1c B1=1√3=0c A 0c B=−1√3=0that show that none of the coeﬃcients c A−1,c A,c A1,c B−1,c B,c B1is equal to zero,so if|ΨABwas factorizable,it should also contain the terms|−1 A⊗|−1 B,|−1 A⊗|0 B,|0 A⊗|−1 B,|0 A⊗|1 B,|1 A⊗|0 B,|1 A⊗|1 B which it doesn’t.When the joint state of two subsystems is entangled,there is no way to assign a deﬁnite pure quantum state to either subsystem alone-the entangled states of both subsystems are inseparable.Instead,they are superposed with one another.This interconnection leads to correlations between observable physical properties(e.g.spin or charge)of remote systems,where the spa-tial separation between the two individual objects that represent those two subsystems (e.g.two particles)is being irrelevant.That bothered Albert Einstein so much that he denoted entanglement as”spukhafte Fernwirkung”or”spooky action at a distance”,4but every subsequent experiment conﬁrmed in every detail the idea of quantum entan-glement.The current state of belief is that although two entangled systems appear to interact across large distances instantaneously,no useful information can be transmitted in this way,meaning that causality cannot be violated through entanglement.This is the statement of the no-communication theorem[2]-quantum entanglement does not enable the transmission of classical information faster than the speed of light because a classical information channel is required to complete the process.Pairs of entangled particles are generated by the decay of other particles,naturally or through induced collision and this quantum entanglement has applications in the emerging technologies of quantum computing and quantum cryptography(superdense coding),and has been used to realize quantum teleportation experimentally.At the same time,it prompts some of the more philosophically oriented discussions concerning quantum theory.2.3Singlet state of two spin1particlesAn example of a spin1system is an atom of orthohelium[8]-the form of the helium atom in which the spins of the two electrons are parallel(S=1,triplet state)which implies lower energies than for the form with antiparallel spins(S=0,singlet state) called parahelium.We use Clebsch-Gordan’s coeﬃcients to write down the singlet state of a twinned pair of such interacting spin1particles i.e.the state with total spin0corresponding therefor to quantum numbers S=0and M=0and we get exactly the state already mentioned above as an example of an entangled state:|S ab=0,M abw =0 =1√3[|M aw=1 |M bw=−1 +|M aw=−1 |M bw=1 −|M aw=0 |M bw=0 ]This state is independent of the direction w,because S aw (=S w⊗I)and S bw(=I⊗S w )act on diﬀerent Hilbert spaces and are therefor commuting operators for any directions w and w .This means,that singlet state looks the same for every basis-we can for example write it down using eigenstates of spin along x-or z-axis and the form stays the same.Components of spin for each entangled particle are indeterminate until some physical intervention is made to measure them.Then the wavefunction collapse causes the system to leap into one of superposed basic states and acquire the coresponding values of the measured property.When the two members of a singlet pair are measured,they will always be found in opposite states.The distance between the two particles is irrelevant.Singlet states have been experimentally achieved for two spin1/2particles separated by more than10km.Presumably a similar singlet state for distantly separated spin1 particles will be attained with suﬃcient technology.5Chapter3EPR paradox and the hidden variable theories3.1EPR paradox[9]In quantum mechanics,the EPR paradox is a thought experiment introduced in1935 by Einstein,Podolsky,and Rosen[5]to argue that quantum mechanics is not a complete physical theory.Quantum theory and quantum mechanics do not account for single measurement outcomes in a deterministic way.According to an accepted interpretation of quantum mechanics known as the Copenhagen interpretation,a measurement causes an instanta-neous collapse of the wave function describing the quantum system,and the system after the collapse appears in a random state.Einstein did not believe in the idea of genuine randomness in nature.In his view,quantum mechanics was incomplete and suggested that there had to be’hidden’variables responsible for random measurement results.In their paper,mentioned above,they turned this philosophical discussion into a physical argument.They claimed that given a speciﬁc experiment(like the one described below), in which the outcome of a measurement could be known before the measurement actually takes place,there must exist something in the real world,an”element of reality”,which determines the measurement outcome.They postulated that these elements of reality are local,in the sense that they belong to a certain point in spacetime.This element may only be inﬂuenced by events which are located in the backward light cone of this point in spacetime.Even though these claims sound reasonable and convincing,they are founded on assumptions about nature which constitute what is now known as local realism and has been later experimentally proven wrong.Locality seems to be a consequence of special relativity,which states that information can never be transmitted faster than the speed of light without violating causality.It is generally believed that any theory which violates causality would also be internally inconsistent,and thus deeply unsatisfactory.It turns out that quantum mechanics only violates the principle of locality without violating causality.6The EPR paradox draws on quantum entanglement,to show that measurements performed on spatially separated parts of a quantum system can apparently have an instantaneous inﬂuence on one another.This eﬀect is now known as ”nonlocal behavior”(or ”spooky action at a distance”).In order to illustrate this,let us consider a simpliﬁed version of the EPR thought experiment put forth by David Bohm.Figure 1.The EPR experimental setupWe have a source that emits pairs of spin 1/2particles (e.g.electrons),with one particle sent to destination A ,where there is an observer named Alice,and another sent to destination B ,where there is an observer named Bob.Our source is such that each emitted electron pair is in an entangled quantum state called a spin singlet:|S ab =0,M ab w =0 =1√2[|M a w =12 |M b w =−12 −|M a w =−12 |M b w =12 ]==1√2[|↑↓ −|↓↑ ]which can be viewed as a quantum superposition of two states,one with electron A having spin up along the z -axis (+z )and electron B having spin down along the z -axis (−z )and another with opposite orientations.Therefore,it is impossible to associate either electron in the spin singlet with a state of deﬁnite spin until one of them is being measured and the quantum state of the system collapsed into one of the two superposed states.Since the quantum state determines the probable outcomes of any measurement per-formed on the system,if Alice measured spin along the z -axis and obtained the outcome +z ,Bob would subsequently obtain −z measuring in the same direction with 100%probability and similarly if Alice obtained −z ,Bob would get +z .And because the singlet state is symetrical with respect to rotation,the same is true for any choice of direction of spin measurement.11modest suggestion for proper expressions by Conway and Kochen to avoid the conceptual problems7The problem now comes down to this:because the spin along x-axis and spin along z-axis are”incompatible observables”,subjected to Heisenberg uncertainty principle,a quantum state cannot possess a deﬁnite value for both variables.So how does Bob’s electron’s spin,measured in x-direction,instantaneously know,which way to point,if it is not allowed to know(locality)whether Alice decided to measure spin along x-axis (Bob’s x-spin measurement will with certainty produce opposite result)or z-axis(Bob’s x-spin measurement will have a50%probability of producing+x and a50%probabil-ity of−x)?Using the usual Copenhagen interpretation rules that say the wave function ”collapses”at the time of measurement,there must be action at a distance or the electron must know more than it is supposed to.According to its authors the EPR experiment yields a dichotomy.Either1.The result of a measurement performed on one part A of a quantum system has anon-local eﬀect on the physical reality of another distant part B,in the sense that quantum mechanics can predict outcomes of some measurements carried out at B;or...2.Quantum mechanics is incomplete in the sense that some element of physical realitycorresponding to B cannot be accounted for by quantum mechanics(that is,some extra variable is needed to account for it.)Incidentally,although we have used spin as an example,many types of physical quan-tities—what quantum mechanics refers to as”observables”—can be used to produce quantum entanglement.The original EPR paper used momentum for the observable. Experimental realizations of the EPR scenario often use photon polarization,because polarized photons are easy to prepare and measure.Figure2.Measurements on a pair of entangled photonsThe EPR paradox is a paradox in the following sense:if one takes quantum mechanics and adds some seemingly reasonable(but actually wrong,or questionable as a whole) conditions(referred to as locality,realism,counter factual deﬁniteness2[10]and complete-ness),then one obtains a contradiction.However,quantum mechanics by itself does not 2counter factual deﬁniteness is the ability to speak meaningfully about the deﬁniteness of the results of measurements,even if they were not performed8appear to be internally inconsistent,nor—as it turns out—does it contradict relativity. As a result of further theoretical and experimental developments since the original EPR paper,most physicists today regard the EPR paradox as an illustration of how quantum mechanics violates classical intuitions.3.2Hidden variable theories and no-go theorems There are several ways to resolve the EPR paradox.The one suggested by EPR is that the statistical(probabilistic)nature of quantum mechanics indicates,despite its enor-mous success in experimental predictions,that quantum mechanics is an”incomplete”description of reality and there should exist some yet undiscovered more fundamental theory of nature,that can due to its dependence on some hidden factors that govern the behaviour of quantum world,always predict the outcome of each measurement with certainty.Such theories are called hidden variable theories.[11]Although determinism (Albert Einstein insisted that,”God does not play dice”)was initially a major motiva-tion for physicists looking for hidden variable theories,nondeterministic theories trying to explain what the supposed reality underlying the quantum mechanics formalism looks like,later also emerged.Bell,Kochen and Specker,and others then produced some powerful no-go theorems[12] that dispose of the most plausible hidden variable theories by showing that even though they may look attractive are in fact not possible.First in1964,John Bell showed through his famous theorem[6]that if local hidden variables exist,certain experiments could be performed where the result would have to satisfy a Bell inequality[7].If,on the other hand,quantum entanglement is correct the Bell inequality would be violated.Experi-ments have later been performed that have found violations of these inequalities up to 242standard deviations and thus with great certainty ruled out local hidden variable the-ories.Another no-go theorem concerning hidden variable theories is the Kochen-Specker theorem,described in detail below.No-go theorems together with experiments have shown a vast class of hidden vari-able theories to be incompatible with observations.However,there are a couple of ways around that.Theoretically,though highly improbable,there could be experimental de-ﬁciencies called loopholes,that aﬀect the validity of the experimentalﬁndings.Another way of blocking no-go theorems that hidden variable theories have proposed is“con-textuality”–that the outcome of an experiment depends upon hidden variables in the apparatus.A hidden-variable theory which is consistent with quantum mechanics would have to be non-local,maintaining the existence of instantaneous or faster than light nonlocal relations(correlations)between physically separated entities(information travel is still restricted to the speed of light).Theﬁrst hidden-variable theory was the pilot wave theory of Louis de Broglie,dating from the late1920s.The currently best-known hidden-variable theory is David Bohm’s Causal Interpretation of quantum mechanics,created in91952,that gives the same answers as quantum mechanics,thus invalidating the famous theorem by von Neumann that no hidden variable theory reproducing the statistical predictions of QM is possible.Bohm’s(nonlocal)hidden variable is called the quantum potential.3.3Kochen-Specker paradoxIn quantum mechanics,the Kochen-Specker theorem[14,13]is a certain”no go”theorem proved by Simon Kochen and Ernst Specker in1967.[30]It places certain constraints on the permissible types of hidden variable theories which try to explain the apparent randomness of quantum mechanics as a deterministic theory featuring hidden states, deﬁning deﬁnite values of observables at all times.The theorem is a complement to Bell’s inequality.The theorem excludes noncontextual hidden variable theories intending to repro-duce the results of quantum mechanics and requiring values of a quantum mechan-ical observables to be noncontextual(i.e.independent of the measurement arrange-ment).(However,note,that it remains possible,that the value attribution may be context-dependent,i.e.observables corresponding to equal vectors in diﬀerent measurement arrangements need not have equal values.)The Kochen-Specker theorem was an important step forward in the debate on the (in)completeness of quantum mechanics,since it demonstrated the impossibility of Ein-stein’s assumption,made in the famous Einstein-Podolsky-Rosen paper from1935(cre-ating the so-called EPR paradox),that quantum mechanical observables represent’ele-ments of physical reality’.Bohr[1]tried to overcome that problem by introducing con-textuality which in exchange implied nonlocality(or spooky action at a distance,as Einstein loved to call it).In the1950’s and60’s two lines of development were open for those being fond of metaphysics,both lines improving on a”no go”theorem presented by von Neumann,trying to prove the impossibility of hidden variable theories yielding the same results as does quantum mechanics.Bell assumed that quantum reality is nonlocal, and that probably only local hidden variable theories are in disagreement with quantum mechanics.More importantly did Bell manage to lift the problem from the metaphys-ical level to the physical one by deriving an inequality,the Bell inequality,that can be experimentally tested.A second line is the Kochen-Specker one.The essential diﬀerence with Bell’s ap-proach is that there is no implication of nonlocality because the proof refers to ob-servables belonging to one single object,to be measured in one and the same region of space.And while Bell inequality gives only statistical restrictions on the results of measurements(their statistical distributions diﬀer in both types of theory),the Kochen-Specker paradox states that certain sets of QM observables cannot be assaigned values at all.Contextuality is related here with incompatibility of quantum mechanical ob-servables,incompatibility being associated with mutual exclusiveness of measurement arrangement.By using the so-called yes-no observables,having only values0and1, corresponding to projection operators on the eigenvectors of a certain orthogonal basis in a three-dimensional Hilbert space,Kochen and Specker were able toﬁnd a set of11710such projection operators,not allowing to assign to each of them in an unambiguous way either value0or1.But here we reproduce one of the similar though much simpler proofs given much later by Asher Peres.original.jpgFigure3.The original Kochen-Specker’s117directions113.3.1Kochen-Specker paradox for Peres’33-direction conﬁguration Kochen-Specker paradox for Peres’33-direction conﬁguration:“There is no101-function for the±33directions of Figure4.”Figure4.The±33directions are deﬁned by the lines joining the center ofthe cube to the±6mid–points of the edges and the±3sets of9points of the3×3square arrays shown inscribed in the incircles of its faces.For the triple experiment3,noncontextuality doesn’t allow the particle’s spin in the z-direction(say)to depend upon the measurement frame(x,y,z).This means that we can assign deﬁnite values of squares of components of spin to every direction from Peres’set.For a spin1particle such a symmetrical function of direction is called“101-function”.One of its properties is that for three orthogonal directions we always get some permu-tation of a“canonical outcome”(1,0,1).That entails that we can not get the outcome(0,0)for two orthogonal directions.Proof.Assume that a101–functionθis deﬁned on these±33directions.Ifθ(W)=i,wewrite W→i.Altogether there are40diﬀerent orthogonal triples-16inside the Peresconﬁguration together with the24that are obtained by completing its24remaining or-thogonal pairs.The orthogonalities of the triples and pairs used below in the proof of a contradiction are easily seen geometrically.For instance,in Figure2,B and C subtendthe same angle at the center O of the cube as do U and V,and so are orthogonal.ThusA,B,C form an orthogonal triple.Again,since rotating the cube through a right angle(90◦)about OZ takes D and G to E and C,the plane orthogonal to D passes throughZ,C,E,so that C,D is an orthogonal pair and Z,D,E is an orthogonal triple.As usual,we write“wlog”to mean“without loss of generality”.3deﬁned in SPIN12The orthogonality of X,Y,Z implies X→0,Y→1,Z→1wlog(symmetry)The orthogonality of X,A and X,A implies A→1and A →1The orthogonality of A,B,C and A ,B ,C implies B→1,C→0wlog(symme-try with respect to rotation through an angle180◦about OX)and B →1,C →0wlog (symmetry with respect to rotation through an angle90◦about OX)The orthogonality of C,D and C ,D implies D→1and D →1The orthogonality of Z,D,E and Z,D ,E implies E→0and E →0The orthogonality of E,F and E,G and similarly E ,F and E ,G implies F→1, G→1and F →1,G →1The orthogonality of F,F ,U implies U→0The orthogonality of G,G ,V implies V→0and since U is orthogonal to V,this is a contradiction that proves the above asser-tion(Lemma).Figure5.Spin assignments for Peres’33directions13Chapter4The Free Will Theorem4.1Introduction to The Free Will Theorem[15]Do we really have free will,or is it all just an illusion?And what exactly is free will if it exists and how is it possible to reconcile it with determinism?What if everything is predetermined but we still can’t have the knowledge of the future even in theory?Fate, destiny?Since the dawn of time the question of our free will has been one of the most contro-versial ones and it still remains that way in present day physics which hasn’t yet found the proper place for it inside the theory.The Free Will Theorem of John Conway and Simon Kochen[23]doesn’t answer to this ancient riddle but merely states that,if we have a certain amount of”free will”,then so do some elementary particles.Thus from explicitly assumed small amount of human free will,which is only that we can freely choose to make any one of a small number of observations,it deduces free will of the particles all over the universe i.e.the particles’response to a certain type of experiment is not determined by the entire previous history of that part of the universe accessible to them,so we can describe that response as free.If the questions aren’t determined ahead of time,so are not the outcomes of measurement. But that does not mean that free will exists at all.A fully deterministic view of the universe could imply that both our questions about the particles and the answers to those questions are pre-ordained.What follows is,that if their physical axioms are even approximately true,together with the Free Will Assumption,they imply,that no theory,(whether it extends quantum mechanics or not),can correctly predict the results of future spin experiments,since these results involve free decisions that the universe has not yet made.Therefor this failure to predict them should no longer be regarded as a defect of theories extending quantum mechanics,but rather as their advantage.The strength of this no-go theorem lies in that it doesn’t presuppose any physical theory but refers actually only to the predicted macroscopic results of certain possible experiments.(The theorem refers to the world itself,rather than to some theory of the world.)14。

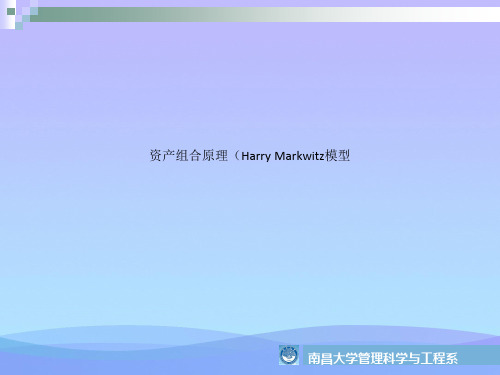
资产组合原理Harry Markwitz模型优选文档PPT

N
σ ＝〔（（i 1 HPRi－历史平均收益率）2 ／n〕1／2
预期收益率：
N
R = (概率)×（可能收益率） i 1
预期收益率的风险衡量：
N
σ =〔 (概率)×（可能收益率－期望收益率）2 i 1
〕1／2
南昌大学管理科学与工程系
2、资产组合的收益与风险
• 资产组合：也称投资组合，是一个资产集合P，这个集合P里包含N个资产，投资在第i个资产上资本量占总投资的比例为Xi ，i ＝1，2 … N
资产组合原理（Harry Markwitz模型南昌大学管理科学与工程系
6.1 Harry Markwitz模型及其基本假设
马柯维兹(Harry Markowitz)1952年在 Journal of Finance发表了论文《资产组合的选择》，标志着现代投资理论发展的开端。
马克维茨1927年8月出生于芝加哥一个店主家庭，大学在芝大读经济系。在研究生期间，他作为库普曼的助研，参加了计量经济学会的证券市场研究工作。他的导师是芝大商学院院长《财务学杂志》主编凯彻姆教授。凯要马克维茨去读威廉姆斯的《投资价值理论》一书。
– 证券组合期望回报率有几种计算方式，每种方式得到相同的结果。
南昌大学管理科学与工程系
（2）利用期末价格计算证券组合的期望回报率
证券在证券组合每股的期末
名称中的股数预期价值
总的期末预期价值
A
100
46.48元
46.48元 100=4,648元
B
200
43.61元
43.61元 200=8,722元
马的贡献是开创了在不确定性条件下理性投资者进行资产组合投资的理论和方法，第一次采用定量的方法证明了分散投资的优点。他用数学中的均值方差，使人们按照自己的偏好，精确地选择一个确定风险下能提供最大收益的资产组合。获1990年诺贝尔经济学奖。

GM模型

§10.2 GM(1,1)模型 1．设：()()()()()()()⎪⎭⎫ ⎝⎛=n x x xX0000,,2,1()()()()()()()⎪⎭⎫ ⎝⎛=n x x xX1111,,2,1称：()()()()b k a k x x =+10 为GM(1,1)模型的原始形式。

2．令：()()()()()()⎪⎭⎫ ⎝⎛-+=121111k k k xxz3．称：()()()()b k a k z x =+10为GM(1,1)模型的基本形式。

4．GM(1,1)模型求解过程的基本程序第一步，根据原始序列求()()k z 1。

第二步，利用最小二乘法求GM(1,1)模型基本形式的参数解。

（1）()()()()()()⎥⎥⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎢⎢⎣⎡=n Y xx x 00032，()()()()()()⎥⎥⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎢⎢⎣⎡---=11312111n B zz z（2）⎥⎦⎤⎢⎣⎡b a ＝()YBB B TT1-第三步，()()()()ab a b k exx at+⎥⎦⎤⎢⎣⎡-=+-1101ˆ，k ＝1，2，…，n 。

第四步，()()()()()()k k k xxx ˆˆˆ11011-+=+， k=1，2，…，n 。

5．一般输入输出建模称为黑箱建模，例如回归模型。

6．由GM(1,1)模型基本解法求出a ，b 后，对原始数据()()10+k x 的预测有三种表达方法。

（1）()()()()()()()()()()⎪⎪⎩⎪⎪⎨⎧-+=++⎥⎦⎤⎢⎣⎡-=+-k k k a ba b k xx x e x x atˆˆˆˆ11011111 （2）()()()()1ˆˆ10--=k k xx αβab5.01+=β，aa5.01+=α，规定()()()()1100ˆxx =（3）()()()()()exx k a k 2001ˆˆ--⎪⎭⎫ ⎝⎛-=αβ，规定()()()()1100ˆxx =，()()()()2200ˆxx =7．例10.2.1 GM 模型求解的规范要求（1）第一步，对原始数据做一次累加。

第十一章-布莱克-舒尔斯-默顿期权定价模型课件

模型
一、B-S-M期权定价的思路
三、强式效率市场假说 (Strong-Form Market
Efficiency)
强式效率市场假说认为价格已充分地反映了所有关
于公司营运的信息，这些信息包括已公开的或内部
未公开的信息。
推论三：在强式有效市场中，没有任何方法能帮助
投资者获得超额利润，即使基金和有内幕消息者也
dG (GaG12Gb2)dtGbdz
x t 2x2
x
其中，dz是一个标准布朗运动。这就是著名的伊藤
引理。
第十一章-布莱克-舒尔斯-默顿期权定价模型
三、伊藤过程与伊藤定理
在伊藤过程的基础上，数学家同普伊通全藤导数（K.Ito）
有什么区别
进一步推导出：若变量x遵循伊藤过程，则变量x和
t的函数G将遵循如下过程：
（一）对的理解 1、证券价格的年波动率，又是股票价格对数收益率的年标准差 2、一般从历史的证券价格数据中计算出样本对数收益率的标准差，再对时间标准化，得到年标准差，即为波动率的估计值。在计算中，一般来说时间距离计算时越近越好；时间窗口太短也不好；一般来说采用交易天数计第算十一章波-布莱动克-模舒率型尔斯-默而顿期不权定价采用日历天数。
x t
x
dG (GaG12Gb2)d tGbdz
x t 2x2
x
第十一章-布莱克-舒尔斯-默顿期权定价模型
三、伊藤过程与伊藤定理
案例11.1 假设变量S服从
dSSdtSdz
令G=lnS，a(x,t)S,b(x,t)=S则
dGdlnS(-2)dtSdz
2
第十一章-布莱克-舒尔斯-默顿期权定价模型
这就是伊藤过程（Ito Process）。其中，dz 是一个标准布朗运动，a、b是变量x和t的函数，变量x的漂移率为a，方差率为b 。 2

琼斯模型阅读笔记

目录1研究概述 (1)1.1研究背景 (1)1.2基本思路 (2)1.3创新点 (2)1.4研究贡献 (2)2制度背景 (3)3提出假说 (3)3.1盈余管理假设 (3)3.2调查期间 (4)3.3局限 (4)4样本选择 (4)4.1样本选择 (4)4.2变量选择 (5)5琼斯模型 (5)5.1基本原理 (5)5.2具体操作 (6)6研究结论 (7)7模型评价 (7)1 研究概述1.1 研究背景图1-1 研究背景1）进口援助进口援助的规则中公开使用的会计数字给经理人员提供了盈余管理的动机，目的是提高获得进口援助的可能性和或提高援助的数量。

进口援助调查提供了一个具体的盈余管理的动机,这是在其他的盈余管理研究中没有的。

ITC 没有根据使用的会计程序或者根据公司制定的关于应计利润的决策调整财务数据。

2）Heal 模型Heal(1985)假设预期非操纵性应计利润等于零，总应计的任何非零值归于管理层操纵。

3）DeAngelo 模型DeAngelo(1986)假设非操纵性应计利润服从随机游走过程。

也就是，对于一个稳定状态的企业，t 时的非操纵性应计利润被假设等于t 一1时的非操纵性应计，因而t 时与t 一1时总应计之间的差异被归于会计操纵。

1/-=it it it TA TA EDA 11/)(---=ititit it A TA TA EDA1.2图1-2 基本思路1.3创新点1）进口援助调查提供了一个具体的盈余管理的动机，这是在其他的盈余管理研究中没有的。

2）对总体应计利润中的操纵性的部分的估计被用作盈余管理的测度变量，而不是使用单一应计利润中的操纵性应计利润部分。

3）公司特征（firm-specific）期望模型被发展成为估计正常（非操纵性的）应计利润。

4）本文发展使用的方法扩展了其他盈余管理研究中的方法；尤其是，时间序列模型被发展用来估计总体非操纵性应计利润，盈余管理假设使用了截面检验。

1.4研究贡献琼斯（1991）发现，被调查的企业为了调减收益而调整应计项目。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

第十二章琼斯模型第十二章琼斯模型本章导读：本章对琼斯模型做了简单介绍，以及给出了琼斯模型的详细stata 程序及解释，使学生能够对琼斯模型在stata 应用有更清晰的认识。

11.1 琼斯模型简介琼斯模型主要认为公司主营业务收入的变动会带来营运资本变动导致企业应计利润的变动，固定资产会产生折旧从而带来应计利润的减少，因此Jones 模型用销售收入增量（△REV ）以及固定资产原值（PPE ）作为自变量，建立总应计的多元线性回归方程，通过参数估计，预测事件期的可操纵性应计。

具体的计算是分为两步：⑴首先利用估计期（P ）的时序数据，将总应计（TA ）回归到总应计的非操纵性成分决定因子（△REV 和PPT ）jt jt j jt j j jt PPE REV TA εββα++∆+=21式中 jt TA ——公司j 在t 年的应计项目总额；jt REV ∆——公司j 在t 年的收入与t-1年收入的差额；jt PPE ——公司j 在t 年的财产、厂房和设备总额；jt ε——反映除jt REV ∆与jt PPE 以外的参差项目对jt TA 所带来的影响；,j α,1j βj 2β——需要进行估计的常数。

选jt REV ∆为变量是为以公司经营活动收入变动额为基础，计算出流动资产和负债的非操纵性应计项目。

同理，jt PPE 是以公司的资本性资产投资额为基础，计算出折旧费用的非操纵性部分。

⑵利用上述模型，求出各参数的系数估计值（,j α,1j βj 2β），然后运用事件期（t ）的数据，计算出非操纵性应计的预期。

那么：)(21jt j jt j j jt jp PPE REV TA U ββα+∆+-=式中，p 为调查年份；jt TA 为公司j 在p 年的应计项目总额；括号内是根据回归模型预测出该年度非操纵性应计项目。

因此jp U 即为公司j 在p 年的操纵性应计项目的预测数。

11.2 琼斯模型的stata 程序和解释clear/*clear 这个命令，在Stata 9.2之前，表示清空掉内存中的所有数据，包括变量、矩阵等等；但Stata 10以后，矩阵就无法清空*/set memory 200m/*修改内存值为200兆，memory表示查看Stata所使用的内存大小以及改变Stata最大可以使用的内存。

如图12.1*/图12.1cd "d:\stata\"/*设置将所有的数据集保存在指定的路径d:/sas programe/data 里，前面三个命令一般可以认为是一个固定的格式 */use acc2006,clear/*打开d:/sas programe/data路径下的acc2006.dta数据集*/sort dm year/*按公司代码和年度排序*/xtset dm year/*定义面板数据，dm为公司代码，year为年份,回车后显示如下图12.2*/图12.2*define the total accrual/*定义应付项目总额*/gen ca=bs43/*bs43为流动资产*/gen lagca=l.ca/*定义lagca为流动资产的滞后一期,当数据集为面板数据时，可以直接用l.变量名表示其滞后一期的变量，滞后两期变量表示为l2.变量名*/gen chgca=ca-lagca/*chgca为流动资产差额*/gen cl=bs116/* bs116为流动负债*/gen lagcl=l.cl/*滞后一期的流动负债*/gen chgcl=cl-lagcl/*流动负债差额*/gen cash=bs5+max(bs6,bs10)/*bs5为货币资金，bs6,bs10分别为短期投资，短期投资净额*/gen lagcash=l.cash/*现金的滞后一期值*/gen chgcash=cash-lagcash/*现金差额*/gen stdebt=bs90+bs111/*bs90为短期借款，bs111为一年内到期的长期借款*/gen lagstdebt=l.stdebtgen chgstdebt=stdebt-lagstdebt/*短期有息负债差额*/gen accrual=(chgca-chgcash)-(chgcl-chgstdebt)/*应计项目*/gen ast=bs89/*bs89为总资产*/gen lagast=l.bs89/*上一期总资产*/*define the total accrual based on Jones(1991)/* 按Jones(1991)定义的应计项目总额*/gen ta=accrual/lagast/*公司的应计项目总额TA*/*define the independent variables based on Jones (1991)/* 按Jones(1991)定义的被解释变量*/gen sale=is5/*sale定义为为主营业务收入*/gen lagsale=l.is5/*定义上一期的主营业务收入*/gen dsale=sale-lagsale/*公司主营业务收入的差额*/gen chgsl=dsale/lagast/*用总资产调整的公司主营业务收入差额*/gen fix=bs71/lagast/*定义fix为固定资产除以总资产*/gen size=1/(lagast/1000000000)/*定义size，总资产以亿元为单位*/sort dm/*merge dm using "d:\stata\sic2.dta",uniqusing/*合并数据集，此数据集在"d:\stata\sic2.dta"路径下，uniqusing是sic2数据集中的每个公司的观测是唯一的，如果合并的两个数据集每个公司的观测都是唯一的，则后用unique，而如果是将按ac2006合并到sic2中，则用uniqmaster*/tab _merge/*列出_merge不同取值的频率，_merge =1、2、3 其中_merge=1表示此观测值只出现在原来的数据集中，_merge=2表示此观测值只出现在新加入的数据集中，_merge=3表示此观测值同时存在于两个数据集中，*/keep if _merge==3/*保留同时存在于两个数据集中中的观测值*/drop _merge/*去掉_merge变量*/drop if ta==. | chgsl==./*去掉应急项目总额和主营业务收入收入差额缺失的观测值*/sort sic2 year/*按行业和年份排序*/save jones1.dta,replace/*保存修改的数据集*/*approach1/*第一种方法*/*using the whole observations/*运用全样本*/use jones1,clear/*打开数据集jones1*/winsorizeJ ta chgsl fix size,suffix(w)/*对数据集中的变量ta chgsl fix size进行winsorize，默认是上下1%。

变量winsorize后生成新变量后缀为w */sum *w size/*对所有后缀为w的变量和size做描述性统计*/pwcorr *w size,sig/*求所有后缀为w的变量、size间的相关系数，并列出相关系数的显著性水平*/quietly by sic2 year:reg taw chgslw fixw sizew,noconstant/*分行业年度回归，不显示回归结果*/predict nda,xb/*根据以上回归得出因变量的拟合值nda*/predict da,resid/*根据以上回归方程，产生参差序列的变量*/sum taw nda da/*对taw，nda，da做描述性统计分析*/save da.dta,replace/*保存数据集为da*/*approach2/*方法二*/*using the sample with enough observations in industry-yearuse jones1,clear/*打开数据集*/by sic2 year:gen obs=_N/*按行业和年度对观测值编号*/drop if obs<15/*去掉观测值编号小于15*/winsorizeJ ta chgsl fix size,suffix(w)/*对数据集中的变量ta chgsl fix size进行winsorize，默认是上下1%。