一道美国数学竞赛题的解法新探
美国数学竞赛AMC8 -- 2010年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 -- 2010年真题解析(英文解析+中文解析)Problem 1Answer: CSolution:Given that these are the only math teachers at Euclid Middle School and we are told how many from each class are taking the AMC 8, we simply add the three numbers to find the total.11+8+9=28.中文解析:参加竞赛的学生总人数是:11+8+9=28. 答案是C。
Problem 2Answer: DSolution:Substitute a=5, b=10 into the expression for a@b to get: 5@10=(5*10)/(5+10)=50/15=10/3.中文解析:(5*10)/(5+10)=50/15=10/3. 答案是D。
Problem 3Answer: CSolution:The highest price was in Month 1, which was $17. The lowest price was in Month 3, which was $10. 17 is 17/10 =170% of 10, and is 170-100=70% more than 10. Therefore, the answer is 70. 中文解析:最高价是1月,17美元。
最低价格是3月10美元。
最高价比最低价多:(17-10)/10=70%。
答案是C。
Problem 4Answer: CSolution:Putting the numbers in numerical order we get the list 0,0,1,2,3,3,3,4 The mode is 3, The median is (2+3)/2=2.5. The average is 2. is The sum of all three is 3+2.5+2=7.5.中文解析:这组数按照从小到大的顺序排列是:0,0,1,2,3,3,3,4. 中位数Median是2.5;mode 是3,mean是16/8=2. 因此mean,median,mode的和是: 2.5+3+2=7.5. 答案是C。
AMC 美国数学竞赛 2002 AMC 10A 试题及答案解析

2002 AMC 10A1、The ratio is closest to which of the following numbers?SolutionWe factor as . As , ouranswer is .2、For the nonzero numbers , , , define.Find .Solution. Ouranswer is then .Alternate solution for the lazy: Without computing the answer exactly,we see that , , and . The sumis , and as all the options are integers, the correct one is obviously .3、According to the standard convention for exponentiation,.If the order in which the exponentiations are performed is changed, how many other values are possible?SolutionThe best way to solve this problem is by simple brute force.It is convenient to drop the usual way how exponentiation is denoted,and to write the formula as , where denotes exponentiation. We are now examining all ways to add parentheses to this expression. There are 5 ways to do so:1.2.3.4.5.We can note that . Therefore options 1 and 2 are equal, and options 3 and 4 are equal. Option 1 is the one given in the problem statement. Thus we only need to evaluate options 3 and 5.Thus the only other result is , and our answer is .4、For how many positive integers does there exist at least one positive integer such that ?infinitely manySolutionSolution 1For any we can pick , we get , therefore theanswer is .Solution 2Another solution, slightly similar to this first one would be using Simon's Favorite Factoring Trick.Let , thenThis means that there are infinitely many numbers that can satisfythe inequality. So the answer is .5、Each of the small circles in the figure has radius one. The innermost circle is tangent to the six circles that surround it, and each of those circles is tangent to the large circle and to its small-circle neighbors. Find the area of the shaded region.SolutionThe outer circle has radius , and thus area . The littlecircles have area each; since there are 7, their total area is . Thus,our answer is .6、Cindy was asked by her teacher to subtract from a certain numberand then divide the result by . Instead, she subtracted and thendivided the result by , giving an answer of . What would heranswer have been had she worked the problem correctly?SolutionWe work backwards; the number that Cindy started with is. Now, the correct result is . Ouranswer is .7、If an arc of on circle has the same length as an arc of oncircle , then the ratio of the area of circle to the area of circle isSolutionLet and be the radii of circles A and B, respectively.It is well known that in a circle with radius r, a subtended arc oppositean angle of degrees has length .Using that here, the arc of circle A has length . The arcof circle B has length . We know that they are equal,so , so we multiply through and simplify to get . As all circles are similar to one another, the ratio of the areas is just thesquare of the ratios of the radii, so our answer is .8、Betsy designed a flag using blue triangles, small white squares, anda red center square, as shown. Let be the total area of the bluetriangles, the total area of the white squares, and the area of thered square. Which of the following is correct?SolutionThe blue that's touching the center red square makes up 8 triangles, or 4 squares. Each of the corners is 2 squares and each of the edges is 1, totaling 12 squares. There are 12 white squares, thus we have.9、There are 3 numbers A, B, and C, such that ,and . What is the average of A, B, and C?More than 1SolutionNotice that we don't need to find what A, B, and C actually are, just their average. In other words, if we can find A+B+C, we will be done.Adding up the equations gives soand the average is . Our answer is .10、Compute the sum of all the roots of.SolutionSolution 1We expand to get which isafter combining like terms. Using the quadratic partof Vieta's Formulas, we find the sum of the roots is . Solution 2Combine terms to get, hence the rootsare and , thus our answer is .11、Jamal wants to store computer files on floppy disks, each ofwhich has a capacity of megabytes (MB). Three of his files requireMB of memory each, more require MB each, and theremaining require MB each. No file can be split between floppydisks. What is the minimal number of floppy disks that will hold all the files?SolutionA 0.8 MB file can either be on its own disk, or share it with a 0.4 MB. Clearly it is not worse to pick the second possibility. Thus we will have 3 disks, each with one 0.8 MB file and one 0.4 MB file.We are left with 12 files of 0.7 MB each, and 12 files of 0.4 MB each.Their total size is MB. The total capacity of 9 disks is MB, hence we need at least 10 more disks. And wecan easily verify that 10 disks are indeed enough: six of them will carry two 0.7 MB files each, and four will carry three 0.4 MB files each.Thus our answer is .12、Mr. Earl E. Bird leaves his house for work at exactly 8:00 A.M. every morning. When he averages miles per hour, he arrives at hisworkplace three minutes late. When he averages miles per hour, hearrives three minutes early. At what average speed, in miles per hour, should Mr. Bird drive to arrive at his workplace precisely on time?SolutionSolution 1Let the time he needs to get there in be t and the distance he travelsbe d. From the given equations, we know that and. Setting the two equal, we have andwe find of an hour. Substituting t back in, we find . From, we find that r, and our answer, is .Solution 2Since either time he arrives at is 3 minutes from the desired time, the answer is merely the harmonic mean of 40 and 60. The harmonicmean of a and b is . In this case, a and b are 40 and 60,so our answer is , so .Solution 3A more general form of the argument in Solution 2, with proof:Let be the distance to work, and let be the correct average speed.Then the time needed to get to work is .We know that and . Summing these twoequations, we get: .Substituting and dividing both sides by , we get ,hence .(Note that this approach would work even if the time by which he is late was different from the time by which he is early in the other case - we would simply take a weighed sum in step two, and hence obtaina weighed harmonic mean in step three.)13、Give a triangle with side lengths 15, 20, and 25, find the triangle's smallest height.SolutionSolution 1This is a Pythagorean triple (a 3-4-5 actually) with legs 15 and 20. Thearea is then . Now, consider an altitude drawn to anyside. Since the area remains constant, the altitude and side to which it is drawn are inversely proportional. To get the smallest altitude, it must be drawn to the hypotenuse. Let the length be x; we have, so and x is 12. Our answer is then.Solution 2By Heron's formula, the area is , hence the shortest altitude'slength is .14、Both roots of the quadratic equation are prime numbers. The number of possible values of isSolutionConsider a general quadratic with the coefficient of being and theroots being and . It can be factored as which is just. Thus, the sum of the roots is the negative of the coefficient of and the product is the constant term. (In general, this leads to Vieta's Formulas).We now have that the sum of the two roots is while the product is. Since both roots are primes, one must be , otherwise the sumwould be even. That means the other root is and the product mustbe . Hence, our answer is .15、Using the digits 1, 2, 3, 4, 5, 6, 7, and 9, form 4 two-digit prime numbers, using each digit only once. What is the sum of the 4 prime numbers?SolutionOnly odd numbers can finish a two-digit prime number, and a two-digit number ending in 5 is divisible by 5 and thus composite,hence our answer is .(Note that we did not need to actually construct the primes. If we had to, one way to match the tens and ones digits to form four primes is , , , and .)16、Let . What is?SolutionLet . Since one ofthe sums involves a, b, c, and d, it makes sense to consider 4x. We have. Rearranging, we have , so .Thus, our answer is .17、Sarah pours four ounces of coffee into an eight-ounce cup and fourounces of cream into a second cup of the same size. She then transfers half the coffee from the first cup to the second and, after stirring thoroughly, transfers half the liquid in the second cup back to the first. What fraction of the liquid in the first cup is now cream?SolutionWe will simulate the process in steps.In the beginning, we have:▪ounces of coffee in cup▪ounces of cream in cupIn the first step we pour ounces of coffee from cup to cup ,getting:▪ounces of coffee in cup▪ounces of coffee and ounces of cream in cupIn the second step we pour ounce of coffee and ounces of cream from cup to cup , getting:▪ounces of coffee and ounces of cream in cup▪the rest in cupHence at the end we have ounces of liquid in cup , and outof these ounces is cream. Thus the answer is .18、A cube is formed by gluing together 27 standard cubicaldice. (On a standard die, the sum of the numbers on any pair of opposite faces is 7.) The smallest possible sum of all the numbers showing on the surface of the cube isSolutionIn a 3x3x3 cube, there are 8 cubes with three faces showing, 12 with two faces showing and 6 with one face showing. The smallest sum with three faces showing is 1+2+3=6, with two faces showing is 1+2=3, and with one face showing is 1. Hence, the smallest possiblesum is . Our answer is thus.19、Spot's doghouse has a regular hexagonal base that measures oneyard on each side. He is tethered to a vertex with a two-yard rope.What is the area, in square yards, of the region outside of the doghouse that Spot can reach?SolutionPart of what Spot can reach is of a circle with radius 2, whichgives him . He can also reach two parts of a unit circle, whichcombines to give . The total area is then , which gives .20、Points and lie, in that order, on , dividing it intofive segments, each of length 1. Point is not on line . Point lieson , and point lies on . The line segments andare parallel. Find .SolutionAs is parallel to , angles FHD and FGA are congruent. Also,angle F is clearly congruent to itself. From SSS similarity,; hence . Similarly, . Thus,.21、The mean, median, unique mode, and range of a collection of eight integers are all equal to 8. The largest integer that can be an element of this collection isSolutionAs the unique mode is , there are at least two s.As the range is and one of the numbers is , the largest one can beat most .If the largest one is , then the smallest one is , and thus the meanis strictly larger than , which is a contradiction.If the largest one is , then the smallest one is . This means that wealready know four of the values: , , , . Since the mean of all thenumbers is , their sum must be . Thus the sum of the missing fournumbers is . But if is the smallest number,then the sum of the missing numbers must be at least ,which is again a contradiction.If the largest number is , we can easily find the solution. Hence, our answer is .NoteThe solution for is, in fact, unique. As the median must be , thismeans that both the and the number, when ordered by size,must be s. This gives the partial solution . For themean to be each missing variable must be replaced by the smallestallowed value.22、A sit of tiles numbered 1 through 100 is modified repeatedly by the following operation: remove all tiles numbered with a perfect square, and renumber the remaining tiles consecutively starting with 1. How many times must the operation be performed to reduce the number of tiles in the set to one?SolutionSolution 1The pattern is quite simple to see after listing a couple of terms.Solution 2Given tiles, a step removes tiles, leaving tiles behind. Now,, so in the next step tilesare removed. This gives , another perfect square.Thus each two steps we cycle down a perfect square, and insteps, we are left with tile, hence our answer is.23、Points and lie on a line, in that order, with and. Point is not on the line, and . The perimeterof is twice the perimeter of . Find .SolutionFirst, we draw an altitude to BC from E.Let it intersect at M. As triangle BEC is isosceles, we immediately get MB=MC=6, so the altitude is 8. Now, let . Using the Pythagorean Theorem on triangleEMA, we find . From symmetry,as well. Now, we use the fact that the perimeter of is twice the perimeter of .We have so. Squaring both sides, we havewhich nicely rearranges into. Hence, AB is 9 so our answer is .24、Tina randomly selects two distinct numbers from the setand Sergio randomly selects a number from the set. The probability that Sergio's number is larger than the sum of the two numbers chosen by Tina isSolutionThis is not too bad using casework.Tina gets a sum of 3: This happens in only one way (1,2) and Sergio can choose a number from 4 to 10, inclusive. There are 7 ways that Sergio gets a desirable number here.Tina gets a sum of 4: This once again happens in only one way (1,3). Sergio can choose a number from 5 to 10, so 6 ways here.Tina gets a sum of 5: This can happen in two ways (1,4) and (2,3). Sergio can choose a number from 6 to 10, so 2*5=10 ways here.Tina gets a sum of 6: Two ways here (1,5) and (2,4). Sergio can choose a number from 7 to 10, so 2*4=8 here.Tina gets a sum of 7: Two ways here (2,5) and (3,4). Sergio can choose from 8 to 10, so 2*3=6 ways here.Tina gets a sum of 8: Only one way possible (3,5). Sergio chooses 9 or 10, so 2 ways here.Tina gets a sum of 9: Only one way (4,5). Sergio must choose 10, so 1 way.In all, there are ways. Tina chooses twodistinct numbers in ways while Sergio chooses a number inways, so there are ways in all. Since , ouranswer is .25、In trapezoid with bases and , we have ,, , and . The area of isSolutionSolution 1It shouldn't be hard to use trigonometry to bash this and find the height, but there is a much easier way. Extend and to meet atpoint :Since we have , with the ratio ofproportionality being . Thus So the sides of are , which we recognize to be aright triangle. Therefore (we could simplify some of the calculation using that the ratio of areas is equal to the ratio of the sides squared),Solution 2Draw altitudes from points and :Translate the triangle so that coincides with . We getthe following triangle:The length of in this triangle is equal to the length of the original, minus the length of . Thus .Therefore is a well-known right triangle. Its area is, and therefore its altitude is.Now the area of the original trapezoid is.。
美国数学竞赛AMC8 -- 2005年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 – 2005年真题解析(英文解析+中文解析)Problem 1Answer: BSolution:If x is the number, then 2x=60 and x=30. Dividing the number by 2 yields 15.中文解析:按照Connie的计算,这个数乘以2是60,可知这个数是30. 应该做的计算是30除以2,因而正确答案应该是15. 答案是B。
Problem 2Answer: CSolution:Karl paid 5*2.5=$12.5. 20% of this cost that he saved is 12.5*0.2=$2.5.中文解析:Karl按原价买了5个文件夹,支付的费用是:2.5*5=12.5. 折扣价是:1.25*0.8=10。
如果Karl 等一天,可以省2.5元。
答案是C.Problem 3Answer: DSolution:Rotating square ABCD counterclockwise 45° so that the line of symmetry BD is a vertical line makes it easier to see that 4 squares need to be colored to match its corresponding square.中文解析:如上图所示,以BD为对称轴,标蓝色的方块需要涂黑。
共4块,答案是D。
Problem 4Answer: CSolution:The perimeter of the triangle is 6.1+8.2+9.7=24cm. A square's perimeter is four times its side length, since all its side lengths are equal. If the square's perimeter is 24, the side length is24/4=6, and the area is 6*6=36.中文解析:三角形的周长是:6.1+8.2+9.7=24. 正方形的周长和三角形相等,也是24,则其边长是24/4=6. 其面积是:6*6=36. 答案是C。
考函数美国竞赛题已知abcd是满足abcde

1、(考函数)(美国竞赛题)已知a ,b ,c ,d 是满足a+b+c+d+e=8,2222216a b c d e ++++=的实数,试确定e 的最大值。
解:设2222()()()()y x a x b x c x d =-+-+-+- 则2222242(a b c d)()y x x a b c d =-+++++++因为2x 的系数是4,且y ≥0,所以,22222[2(a b c d)]44()0a b c d =-+++-⨯+++≤于是,224(8)16(16)0e e ---≤,解得1605e ≤≤。
当65a b c d ====时,165e =,所以e 的最大值为165。
(考递归数列)(2001年保加利亚数学奥林匹克竞赛试题)已知数列﹛a n ﹜适合a 0=4,a 1=22,且a n —6a n-1+a n -2=0(n ≥2),证明:存在两个正整数数列﹛xn ﹜和﹛yn ﹜满足an=27n n nx y y +-(n≥0)。
n y 证明:∵a n —6a n-1+a n -2=0(n ≥2)∴其特征方程是212610,33x x x x -+==+=-.令(3(3n n n a A B =++-。
又014,22a a ==,∴ A+B=4, ∴ A =(3(322A B ++-=, B =∴()(()(5353nnn a ⎤=++-⎥⎦∴()(()(2222214275353277nnna ⎡⎤-=++-+⨯-⎢⎥⎣⎦()(()(2145353nn⎡⎤=++-⎢⎥⎣⎦令()(()(11225353nnn y =++--,∴272n n y a +=令2()n n n x y a n N -=∈∴()(()(11222)53)53nnn n n x y a =+=+--33nn=++-∴21016,13,57.n n n x x x x x ++=-==∴*()n x N n N ∈∈ 同理21016,5,31.n n n y y y y y ++=-==∴*()n y N n N ∈∈∴数列{n x },{n y }即为满足题设的数列。
美国数学竞赛AMC8 -- 2019年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 -- 2019年真题解析(英文解析+中文解析)Problem 1Answer: DSolution:We maximize the number of sandwiches Mike and Ike can buy by finding the lowest multiple of 4.5 that is less than 30 This number is 6.Therefore, they can buy 6 sandwiches for 4.5*6=27. They spend the remaining money on soft drinks, so they buy30-27=3 soft drinks. Combining the items, Mike and Ike buy 6+3=9 items.中文解析:4.5*6=27. 因此买6个Sandwiches,剩下的3元钱买3个Drinks.Problem 2Answer: ESolution:Using the diagram we find that the larger side of the small rectangle is 2 times the length of the smaller side. Therefore the longer side is 5*2=10. So the area of the identical rectangles is5*10=50. We have 3 identical rectangles that form the large rectangle. Therefore the area of the large rectangle is 50*3=150.中文解析:长方形的短边的长度是5,则AD=5+5=10. CD=AD=10. 即长方形的长边是10. ABCD的面积是: AB *BC =(10+5)*10=150.答案是E。
一道美国初中数学竞赛题赏析

一道美国初中数学竞赛题赏析刘义勋【期刊名称】《湖南教育(下旬刊)》【年(卷),期】2016(000)002【总页数】1页(P59-59)【作者】刘义勋【作者单位】邵东县杨桥镇中心学校【正文语种】中文【英文原题】George walks 1 mile to school. He leaves home at the same time each day,walks at a steady speed of 3 miles per hour,and arrives just as school begins.Today he was distracted by the pleasant weather and walked the first 1/2 mile at a speed of only 2 miles per hour.At how many miles per hour must George run the last 1/2 mile in order to arrive just as school begins today?【中文意译】乔治走1英里去上学。
他每天在相同的时间离开家,以每小时3英里的匀速行走,能按时到达学校。
今天他因被宜人的风景分心,前英里的速度只有每小时2英里。
乔治后英里以每小时多少英里速度奔跑,才能按时到达学校?分析:行程问题中,将前半程速度与后半程速度的算术平均值看作全程的平均速度,是一种非理性的认识。
基于这样一种错误认识,很多人认为乔治后英里只要以每小时4英里的速度奔跑,就能按时到达学校。
这种认识之所以是错误的,是因为前后半程花费的时间不一样。
在本题中,乔治在前英里的速度慢,只有每小时2英里,因而耗时长,对全程的平均速度影响大。
乔治在后英里的速度快,耗时短,对全程的平均速度影响小。
如果乔治后英里只以每小时4英里的速度奔跑,全程的平均速度将会低于2、4的算术平均值3,乔治势必迟到。
关于一道美国大学生数学竞赛题

此 试题 启 发 我 们 提 出如 下 问 题 : 若将题 中的 厂 ( z )・g ( )与 f ( x ) / g ( x )在 z = 0处 可 微 改 为 厂 ( z )・ g ( z )与 厂 ( )+ g ( z )( 或 厂 ( )一 g ( )) 在 X = 0处 可 微 , 或 f ( x ) / g ( x )与 , ( )+ g ( z ) ( 或 ,( ) 一g ( z )) 在 一 0 处 可微 , 则 问题结 论是 否仍 然成 立 ?下 面我们将 以命 题形 式 给出相关 结论. 命题 1 设实 函数 fi x ), g ( ) 在 包含 z一 0的开 区间 内有定 义.若 fi x )・ g ( z ) 与f i x ) +g ( )
l i m g 兰 : g
m
( 6 )
M
m
l i m
卜 0
±曼 二[ ’ ! ± !
Z
( 7) ∞
m
由 于
±
g( 0) g( z)
一
.
二
+ ・ ( ) +g ( ) 一[ _ 厂 ( 0 ) +g ( 0 ) ]
3 2
一
一ห้องสมุดไป่ตู้
( 0)
厂 ( ) +g( z ) 一[ _ 厂 ( 0 ) +g ( 0 ) ]
Ⅵ . 。
_ 1 ,
又g ( )在 z一 0处 连续 且 g( 0 )≠ l / ( 0 ), 故 由上式及 ( 4 ) , ( 5 ) 知
l i m
一 0
二
一
g ( O ) 一 l 厂 ( 0 )’
第 2 9卷 第 6期
2 0 1 3年 1 2月
AMC美国数学竞赛AMC.B 试题及答案解析
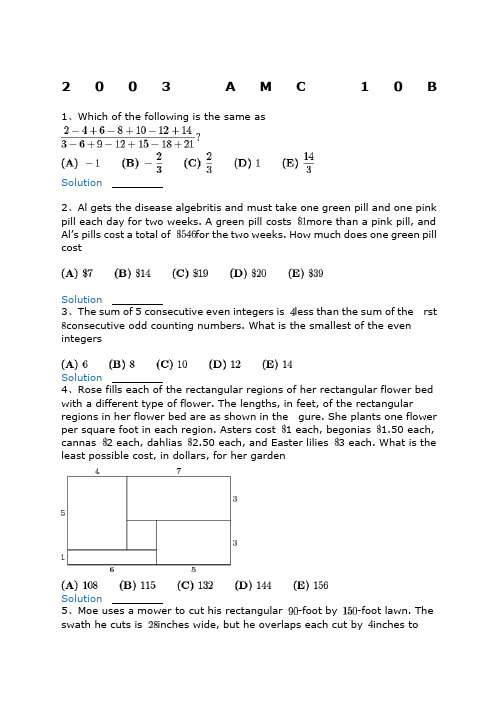
2003A M C10 B 1、Which of the following is the same asSolution2、Al gets the disease algebritis and must take one green pill and one pink pill each day for two weeks. A green pill costs more than a pink pill, and Al’s pills cost a total of for the two weeks. How much does one green pill costSolution3、The sum of 5 consecutive even integers is less than the sum of the rst consecutive odd counting numbers. What is the smallest of the even integersSolution4、Rose fills each of the rectangular regions of her rectangular flower bed with a different type of flower. The lengths, in feet, of the rectangular regions in her flower bed are as shown in the gure. She plants one flower per square foot in each region. Asters cost 1 each, begonias 1.50 each, cannas 2 each, dahlias 2.50 each, and Easter lilies 3 each. What is the least possible cost, in dollars, for her gardenSolution5、Moe uses a mower to cut his rectangular -foot by -foot lawn. The swath he cuts is inches wide, but he overlaps each cut by inches tomake sure that no grass is missed. He walks at the rate of feet per hour while pushing the mower. Which of the following is closest to the number of hours it will take Moe to mow his lawnSolution.6、Many television screens are rectangles that are measured by the length of their diagonals. The ratio of the horizontal length to the height in a standard television screen is . The horizontal length of a “-inch” television screen is closest, in inches, to which of the followingSolution7、The symbolism denotes the largest integer not exceeding . For example. , and . ComputeSolution.8、The second and fourth terms of a geometric sequence are and . Which of the following is a possible first termSolution9、Find the value of that satisfies the equationSolution10、Nebraska, the home of the AMC, changed its license plate scheme. Each old license plate consisted of a letter followed by four digits. Each new license plate consists of three letters followed by three digits. By how many times is the number of possible license plates increasedSolution11、A line with slope intersects a line with slope at the point . What is the distance between the -intercepts of these two linesSolution12、Al, Betty, and Clare split among them to be invested in different ways. Each begins with a different amount. At the end of one year they have a total of . Betty and Clare have both doubled their money, whereas Al has managed to lose . What was Al’s original portionSolution.13、Let denote the sum of the digits of the positive integer . For example, and . For how many two-digit values of isSolution14、Given that , where both and are positive integers, find the smallest possible value for .Solution15、There are players in a singles tennis tournament. The tournament is single elimination, meaning that a player who loses a match is eliminated. In the first round, the strongest players are given a bye, and the remaining players are paired off to play. After each round, the remaining players play in the next round. The match continues until only one player remains unbeaten. The total number of matches played isSolution16、A restaurant offers three desserts, and exactly twice as many appetizers as main courses. A dinner consists of an appetizer, a main course, and a dessert. What is the least number of main courses that the restaurant should offer so that a customer could have a different dinner each night in the yearSolution.17、An ice cream cone consists of a sphere of vanilla ice cream and a right circular cone that has the same diameter as the sphere. If the ice cream melts, it will exactly ll the cone. Assume that the melted ice cream occupies of the volume of the frozen ice cream. What is the ratio of the cone’s height to its radiusSolution18、What is the largest integer that is a divisor offor all positive even integersSolution19、Three semicircles of radius are constructed on diameter of a semicircle of radius . The centers of the small semicircles divide into four line segments of equal length, as shown. What is the area of the shaded region that lies within the large semicircle but outside the smaller semicirclesSolution20、In rectangle , and . Points and are on so that and . Lines and intersect at . Find the area of .Solution21、A bag contains two red beads and two green beads. You reach into the bag and pull out a bead, replacing it with a red bead regardless of the color you pulled out. What is the probability that all beads in the bag are red after three such replacementsSolution22、A clock chimes once at minutes past each hour and chimes on the hour according to the hour. For example, at 1 PM there is one chime and at noon and midnight there are twelve chimes. Starting at 11:15 AM on February , , on what date will the chime occurSolution23、A regular octagon has an area of one square unit. What is the area of the rectangleSolution24、The rst four terms in an arithmetic sequence are , , , and, in that order. What is the fth termSolution25、How many distinct four-digit numbers are divisible by and have as their last two digitsSolution。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
一道美国数学竞赛题的解法新探
吴家华(四川省遂宁中学校 629000)
摘 要 本文在文]1[对一道美国数学竞赛题的多种解法的基础上,又给出了它的几种不同的新解法.
关键词 美国数学;竞赛题;解法
美国第七届中学数学竞赛中有如下一道试题:
已知e d c b a ,,,,是实数且满足e d c b a ++++8=,1622222=++++e d c b a ,试确定e 的最大值.
笔者最近在查阅资料时,看到文]1[中给出了本题的五种解法,它们分别是判别式法,平均值不等式法,平均值代换法,构造函数法,空间解析几何法等. 笔者通过对它进行一番分析、研究后,又得到它的另外几种解法,现介绍如下:
解法1 (三角代换法)由已知得:01622222≥-=+++e d c b a ,则可令 γβαcos cos cos 162e a -=,γβαcos cos sin 162e b -=,
γβcos sin 162e c -=,γsin 162e d -=,)2,0[,πβα∈,),0[πγ∈. ∵8=++++e d c b a ,
∴d c b a e +++=-8,
)sin cos sin cos cos sin cos cos (cos 162γγβγβαγβα+++-=e ,
]sin cos sin cos cos )4sin(2[162γγβγβπ
α+++-=e ,
}sin cos ]sin cos )4sin(2{[162γγββπα+++
-=e , ]sin cos )sin(1)4
(sin 2[1622γγϕβπ
α++++-=e (其中2tan =ϕ), ]sin cos )sin(112[162γγϕβ+++⨯-≤e ,
]sin cos )sin(3[162γγϕβ++-≤e ,
)]sin(1)(sin 3[1622θγϕβ+++-=e (其中)sin(3tan ϕβθ+=)
, )sin(162)]sin(113[1622θγθγ+-=++⨯-≤e e , 2162e -≤, 即e e
-≥-81622.
解之,得:5160≤
≤e . 故e 的最大值为5
16. 评注 本解法中的三角代换得益于空间直角坐标系与球坐标系间的坐标变换关系的启发,是它的一种推广.
解法2 (Cauchy 不等式法)∵8=++++e d c b a ,∴e d c b a -=+++8. 又∵1622222=++++e d c b a ,∴2222216e d c b a -=+++.
由Cauchy 不等式得:
222222222)1111()1111)((⋅+⋅+⋅+⋅≥++++++d c b a d c b a ,
当且仅当d c b a ===时,等号成立.
即228)16(4)(e e -≥
-. 解之,得:5
160≤≤e . 由⎪⎪⎩
⎪⎪⎨⎧=====++++5168e d c b a e d c b a 解之,得:⎪⎪⎩⎪⎪⎨⎧=====51656e d c b a . ∴当且仅当56=
===d c b a 时,e 取得最大值516. 故e 的最大值为5
16. 解法3(向量法)设),,,(d c b a =,),1,1,1,1(=,与的夹角为θ)0(πθ≤≤,则e d c b a d c b a -=+++=⋅+⋅+⋅+⋅=⋅81111,
2222216||e d c b a m -=+++=,2||=. ∵θcos ||||n m n m =⋅,∴||||||n m n m ≤⋅, 即2162|8|e e -≤-. 解之,得:5160≤
≤e . 故e 的最大值为5
16. 解法4(利用方差的非负性)取一组数据d c b a ,,,且满足e d c b a -=+++8,则它们的平均数为)8(4
1)(41e d c b a x -=+++=.
∴这组数据的方差为
])()()()[(4
122222x d x c x b x a s -+-+-+-= 0)8(16
1)16(41)(412222222≥---=-+++=e e x d c b a . ∴0)8()16(422≥---e e . 解之,得:5160≤
≤e . 故e 的最大值为5
16. 参考文献
1.兰振万.一道美国数学竞赛题的多种解法.中学教研(数学),1988年Z1期.。
