河南省南阳市第一中学2014届高三上学期第三次周考(11月)试题
河南省南阳市2014届高三第三次联考(高考模拟)数学(理)试题(高清扫描,答案文档版)

2014春期南阳市高中毕业班模拟考试数学(理科)答案一、选择题:CACBCB DBDADB二、填空题: 13.4 14. -332 15. 5216. π6 三、解答题:17.解:(Ⅰ)∵121++=+n S S n n ,当2≥n 时n S S n n +=-12∴121+=+n n a a -----------------------------------------------2分 ∴()1211+=++n n a a 即2111=+++n n a a (2≥n )又1,22s 1112==+=s a s ∴32=a ∴21112=++a a ∴n n a 21=+ 即()*12N n a n n ∈-= ...........6分 (Ⅱ)∵12-=n n a ∴11(21)(21)222n n n n n nn n nb ++===----.....8分 ∴n n n T 223222132++++=,132221222121++-+++=n n n n n T ∴22212)221212121(21132<--=-++++=-+n n n n n nn T ...........12分 18.解(Ⅰ)由题意可知,样本容量8500.01610n ==⨯,20.0045010y ==⨯,0.10.0040.0100.0160.040.030x =----=. ················· 3分(Ⅱ)由题意可知,分数在[80,90)有5人,分数在[90,100]有2人,共7人.抽取的3名同学中得分在[80,90)的学生个数ξ的可能取值为1,2,3,则12523751(1)357C C P C ξ====,215237204(2)357C C P C ξ====,3537102(3)357C P C ξ====. 所以,ξ的分布列为ξ1 2 3 P174727X所以,142151237777E ξ=⨯+⨯+⨯=. 12分19.解:(1)证明 取DE 中点N ,连结,MN AN .在△EDC 中,,M N 分别为,EC ED 的中点,则MN ∥CD ,且12MN CD =.由已知AB ∥CD ,12AB CD =, 因此,MN ∥AB ,且MN AB =.所以,四边形ABMN 为平行四边形. 于是,BM ∥AN .又因为AN ⊂平面ADEF ,且BM ⊄平面ADEF , 所以BM ∥平面ADEF . ………..6分(2)按如图建立空间直角坐标系,点D 与坐标原点O 重合.设),,(z y x M ,则)2,,(-=z y x EM ,又)2,4,0(-=EC ,设(01)EM EC λλ=<<,则λλ22,4,0-===z y x ,即)22,4,0(λλ-M .设),,(111z y x n =是平面BDM 的法向量,则02211=+=⋅y x n OB ,0)22(411=-+=⋅z y n OM λλ.取11=x ,得λλ-=-=12,111z y ,即得平面BDM 的一个法向量为)12,1,1(λλ--=n . ……..10分 由题可知,)0,0,2(=OA 是平面ABF 的一个法向量. 因此,22||211|cos ,|,2||||6422(1)OA n OA n OA n λλλ⋅<>====⋅+-,即点M 为EC 中点.此时,2=DEM S ∆,AD 为三棱锥DEM B -的高, 所以,=-BDE M V 342231=⋅⋅=-DEM B V . ............. ………..12分 20解: (Ⅰ)直线)0(:>+=m m x y l 与圆54:221=+y x C 相切,所以5102.542==m m ……………4分 (Ⅱ) 将5102:+=x y l 代入得 1:22222=+by a x C 得:0585104)(2222222=-+++b a a x a x a b ①A BC DE FMYZ设),,(),,(221111y x B y x A 则)(252540)5102)(5102(;)(558,)(5104222222121222222122221b a b a b x x y y a b b a a x x a b a x x +-=++=+-=+-=+因为05)(4,222211=-+⇒⊥b a b a OB OA ②由已知224,3b a b c ==代人(2)4,10)1(2222==⇒=-a b b b所以椭圆2C 的方程为1422=+y x ……………8分 (Ⅲ)显然直线AS 的斜率存在,设为k 且0>k 则)2(:+=x k y AS依题意)1564,1534(k M ,由⎪⎩⎪⎨⎧=++=14)2(22y x x k y 得:041616)41(2222=-+++k x k x k设),(00y x S 则)2(,418241416)2(00220220+=+-=⇒+-=-⋅x k y kk x k k x 即 )414,4182(222kk k k S ++-,又B (2,0)所以,41200k x y k BS -=-= BS :)2(41--=x k y 由15161511564215115640),151-,1534(1534)2(41=⋅≥+=⇒>⇒⎪⎪⎩⎪⎪⎨⎧=--=k k k k MN k k N x x ky 所以81=k 时:1516min=MN ……………12分 21.解:(1)f(0)=0,斜率k=1)0(f '-=,所以,曲线y=f(x)在x =0处的切线方程为:x+y=0 ………………….2分恒成立矛盾这与0)(,0)0()1(h ≥=<-∴x h h a .综上,∴≥,1a a 的最小值为 1. ………7分12分22.【证明】(1)连结BC,∵AB 是直径, ∴∠ACB=90°,∴∠ACB=∠AGC=90°. ∵GC 切⊙O 于C,∴∠GCA=∠ABC.∴∠BAC=∠CAG . ………………….5分 (2)连结CF,∵EC 切⊙O 于C, ∴∠ACE=∠AFC. 又∠BAC=∠CAG , ∴△ACF ∽△AEC.∴AC AFAE AC=,∴AC 2=AE·AF. ……………….10分 23.解:(1)曲线C 的直角坐标方程为x y 42=,故曲线C 是顶点为O (0,0),焦点为F(1,0)的抛物线;………………….5分(2)直线l 的参数方程为⎩⎨⎧+==ααsin 1cos t y t x ( t 为参数,0≤α<π).故l 经过点(0,1);若直线l 经过点(1,0),则43πα=∴直线l 的参数方程为⎪⎪⎩⎪⎪⎨⎧+=+=-==t t y t t x 22143sin 12243cos ππ(t 为参数) 代入x y 42=,得02262=++t t设A 、B 对应的参数分别为21,t t ,则2,262121=-=+t t t t∴21221214)(t t t t t t AB -+=-==8 ……………….10分24.【答案】(1)f (x )=|x -a |≤3,即a -3≤x ≤a +3.依题意,⎩⎨⎧a -3≤-1,a +3≥3.由此得a 的取值范围是[0,2] ………………….5分(2)f (x -a )+f (x +a )=|x -2a |+|x |≥|(x -2a )-x |=2|a |.当且仅当(x -2a )x ≤0时取等号.解不等式2|a |≥1-2a ,得a ≥ 14.故a 的最小值为 14.……………….10分。
河南省南阳市第一中学校2023-2024学年高三上学期第三次月考地理试题(解析版)

南阳一中2023年秋期高三年级第三次月考地理试题一、选择题组(每小题1.5分,共40题)城市土地出让是指城市政府土地主管部门依法将城市土地的使用权出让给用地单位,是联系城市产业结构与空间结构的纽带。
土地出让空间区位选择与诸多社会经济因素有关,其中人口规模、经济发展水平对土地出让具有重要影响。
下图示意黄山市城市土地出让区位模式。
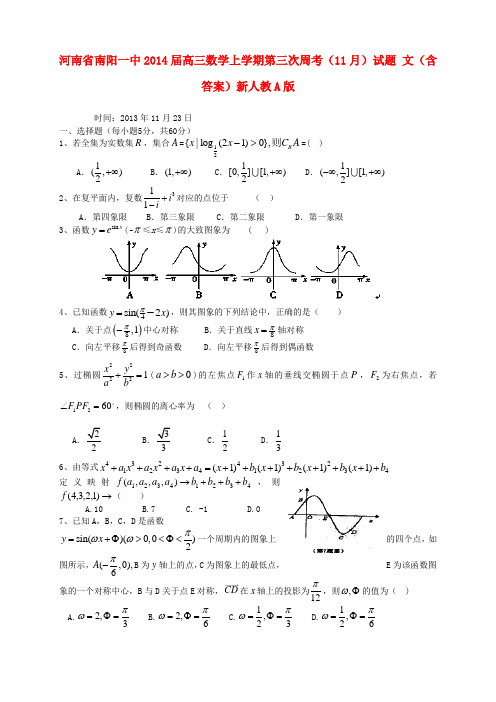
据此完成下面小题。
1.V区虽位于城市外围,但商业用地和住宅用地规模大,主要原因是()A.位于城市外围,土地租金廉价B.人口规模较市中心大,商业服务需求高C.中心城区环境差,商业服务外迁D.位于旅游景区,经济发展水平高2.黄山市城市用地的分布特点是()A.分布均衡,集聚程度较低B.住宅用地分布在近郊和旅游区C.集中度高,成多中心状态D.中心城区土地利用率较外围低【答案】1.D 2.C【解析】【1题详解】黄山市为旅游业发达的城市,V区可能为旅游区,虽然与市中心距离较远,但旅游业的发展使其土地租金并不廉价,A错误;V区位于城市外围,人口规模并不如市中心大,B错误;没有信息说明中心城区环境质量差,C错误;旅游业的发展带动了经济发展,该地经济发展水平高,商业和度假旅居型、疗养等服务业的发展,使得V区商业用地和住宅用地规模较大,D正确。
故选D。
【2题详解】据图及上题可知,黄山市三类用地区域位置差异较大,A错误;住宅用地主要集中于中心城区、重要旅游区附近,B错误;商业服务用地分布的热点区域主要集中于城市商业集聚区、大型公共设施和旅游景区周围、重要交通设施附近,因此,黄山市各类用地集中程度高,成多中心状态,C正确;中心城区土地利用率较外围高,D错误。
故选C。
【点睛】影响城市土地租金的因素有距离市中心的远近和交通通达度。
波兰首都华沙的工业园区逐步转型成为次级商务区(SBD),其与西南运输走廊(IBD)和主城区的中央商务区(CBD)共同构成华沙的经济增长极。
下图示意华沙经济增长极的空间分布读图,完成下面小题。
河南省南阳一中2014届高三数学上学期第三次周考(11月)试题 文(含答案)新人教A版
河南省南阳一中2014届高三数学上学期第三次周考(11月)试题 文(含答案)新人教A 版时间:2013年11月23日 一、选择题(每小题5分,共60分)1、若全集为实数集R ,集合A =12{|log (21)0},R x x C A ->则=( )A .1(,)2+∞B .(1,)+∞C .1[0,][1,)2+∞D .1(,][1,)2-∞+∞2、在复平面内,复数311i i+-对应的点位于 ( )A .第四象限B .第三象限C .第二象限D .第一象限 3、函数x e y sin =(π-≤x ≤π)的大致图象为 ( )4、已知函数4sin(2)y x π=-,则其图象的下列结论中,正确的是( ) A .关于点()8,1π-中心对称 B .关于直线8x π=轴对称C .向左平移8π后得到奇函数D .向左平移8π后得到偶函数5、过椭圆22221x y a b +=(0a b >>)的左焦点1F 作x 轴的垂线交椭圆于点P ,2F 为右焦点,若1260F PF ∠=,则椭圆的离心率为 ( )A .22 B .33 C .12D .13 6、由等式43223144322314)1()1()1()1(b x b x b x b x a x a x a x a x ++++++++=++++ 定义映射43214321),,,(b b b b a a a a f +++→,则→)1,2,3,4(f ( )A.10B.7C. -1D.07、已知A ,B ,C ,D 是函数sin()(0,0)2y x πωω=+Φ><Φ<一个周期内的图象上的四个点,如图所示,(,0),6A π-B 为y 轴上的点,C 为图象上的最低点,E 为该函数图象的一个对称中心,B 与D 关于点E 对称,CD 在x 轴上的投影为12π,则,ωΦ的值为( ) A.2,3πω=Φ= B.2,6πω=Φ= C.1,23πω=Φ= D.1,26πω=Φ=8、在△ABC 中,AB=4,∠ABC=30°,D 是边BC 上的一点,且AC AD AB AD ⋅=⋅, 则AB AD ⋅的值等于( )A .—4B .0C .4D .8 9、若20πα<<,02-<<βπ,31)4cos(=+απ,33)2-4cos(=βπ,则=+)2cos(βα( )A .33B .33-C .539D .69-10、若在区域34000x y x y +-≤⎧⎪≥⎨⎪≥⎩内任取一点P ,则点P 落在单位圆221x y +=内的概率( ) A .332πB.325πC.163πD.165π11、已知ABC ∆的三边长成公差为2的等差数列,且最大角的正弦值为23,则这个三角形的周长是( )A.18B.21C.24D.1512、设函数1(1)|-1|)=1(=1)x x f x x ⎧≠⎪⎨⎪⎩(,若关于x 的方程2[()]+()+c=0f x bf x 有三个不同的实数根123,,x x x ,则222123++x x x 等于( )A. 13B. 5C. 223c +2c D. 222b +2b第Ⅱ卷(非选择题 共90分)二、填空题(每题5分,共20分)13、已知倾斜角为α的直线l 与直线220x y -+=平行,则tan 2α的值为14、已知函数()f x 是R 上的奇函数,若对于0x ≥,都有()2()f x f x +=,[)()()20,2,log 1x f x x ∈=+当时时,())2014(2013f f +-的值为15、某校对高三年级的学生进行体检,现将高三男生的体重(单位:kg)数据进行整理后分成六组,并绘制频率分布直方图(如右图).已知图中从左到右第一、第六小组的频率分别为0.16,0.07,第一、第二、第三小组的频率成等比数列,第三、第四、第五、第六小组的频率成等差数列,且第三小组的频数为100,则该校高三年级的男生总数为16、已知各项均为正数的等比数列{}n a 满足7652a a a =+,若存在两项,m n a a 使得1144,m n a a a m n=+则的最小值为 三、解答题(本大题共7题,共70分)17、(本小题满分12分)在△ABC 中,a ,b ,c 分别是角A ,B ,C 的对边长,已知22223b c a ac --=。
河南省南阳一中2014届高三上学期第三次周考(11月)数学(理)试题 Word版含答案

时间:2013年11月23日一、选择题(每小题5分,共60分)1、若全集为实数集R ,集合A =12{|log (21)0},R x x C A ->则=( )A .1(,)+∞B .(1,)+∞ C .1[0,][1,)+∞D .1(,][1,)-∞+∞3、函数xey sin =(π-≤x ≤π)的大致图象为 ( )4、已知函数)24sin(x y -=π,则其图象的下列结论中,正确的是( ) A .关于点⎪⎭⎫⎝⎛1,8-π中心对称 B .关于直线8π=x 轴对称C .向左平移8π后得到奇函数D .向左平移8π后得到偶函数5、设11cos ,sin ,a xdx b xdx ==⎰⎰下列关系式成立的是 ( )A .a b >B .1a b +<C .a b <D .1a b +=6、由等式43223144322314)1()1()1()1(b x b x b x b x a x a x a x a x ++++++++=++++定义映射43214321),,,(b b b b a a a a f +++→,则→)1,2,3,4(f ( )A .10B .7C . -1D .07、已知A ,B ,C ,D 是函数sin()(0,0)2y x πωω=+Φ><Φ<一个周期内的图象上的四个点,如图所示,(,0),6A π-B 为y 轴上的点,C 为图象上的最低点,E 为该函数图象的一个对称中心,B 与D 关于点E 对称,CD 在x 轴上的投影为12π,则,ωΦ的值为( )A .2,3πω=Φ=B .2,6πω=Φ=C .1,23πω=Φ=D .1,26πω=Φ=8、在△ABC 中,AB=4,∠ABC=30°,D 是边BC 上的一点,且AC AD AB AD ⋅=⋅, 则AD 的值等于( )A .—4B .0C .4D .89、若20πα<<,02πβ-<<,31)4cos(=+απ,cos()42πβ-= 则=+)2cos(βα( )A. B. C. D.10、若在区域34000x y x y +-≤⎧⎪≥⎨⎪≥⎩内任取一点P ,则点P 落在单位圆221x y +=内的概率( ) A .332π B .325π C .163π D .165π 11、已知ABC ∆的三边长成公差为2的等差数列,且最大角的正弦值为23,则这个三角形的周长是( )A .18B .21C .24D .1512、设函数1(1)|-1|)=1(=1)x x f x x ⎧≠⎪⎨⎪⎩(,若关于x 的方程2[()]+()+c=0f x bf x 有三个不同的实数根123,,x x x ,则222123++x x x 等于( )A . 13B . 5C . 223c +2cD . 222b +2b第Ⅱ卷(非选择题 共90分)二、填空题(每题5分,共20分)13、用0,1,2,3,4排成无重复数字的五位数,要求偶数字相邻,奇数字也相邻,则这样的五位数的个数是14、已知函数()f x 是R 上的奇函数,若对于0x ≥,都有()2()f x f x +=,[)()()20,2,log 1x f x x ∈=+当时时,())2014(2013f f +-的值为15、某校对高三年级的学生进行体检,现将高三男生的体重(单位:kg)数据进行整理后分成六组,并绘制频率分布直方图(如右图).已知图中从左到右第一、第六小组的频率分别为0.16,0.07,第一、第二、第三小组的频率成等比数列,第三、第四、第五、第六小组的频率成等差数列,且第三小组的频数为100,则该校高三年级的男生总数为16、已知各项均为正数的等比数列{}n a 满足762a a a=+,若存在两项,m n a a 1144,a m n=+则的最小值为三、解答题(本大题共7题,共70分)17、(本小题满分12分)在△ABC 中,a ,b ,c 分别是角A ,B ,C 的对边长,已知22223b c a ac --=。
河南省南阳一中等高三语文天一大联考阶段测试(三)试题(含解析)

2014-2015学年高中毕业班阶段性测试(三)本试题卷分第1卷(阅读题)和第Ⅱ卷(表达题)两部分。
考生作答时,将答案答在答题卡上(答题注意事项见答题卡),在本试题卷上答题无效。
考试结束后,将本试题卷和答题卡一并交回。
【试卷综析】本套试题特点:依纲、平实稳定、可借鉴。
严格依据高考考纲的精神。
在学科考查的主体内容、相应学科能力、试卷的长度、需要阅读的文字总量、要求学生书写的字数、试卷的结构、题目的类型、题干的表述、试题的难中易及主客观试题的比例等方面体现了“考试说明”的精神。
试卷总体设计似曾相识,稳字当先。
基本保持了20014年高考试卷的风格,其中不乏变革、创新的成分。
试题中蕴涵的考纲理念,无形之中将中学语文教学纳入“有据可依”的轨道,有助于提高学生的学习积极性。
第1卷阅读题必考题一、现代文阅读(9分,每小题3分)【题文】M0阅读下面的文字,完成1~3题。
技术与人的关系,是一个不断演变的历史过程。
在手工劳动中,原始技术同劳动者不可分离。
手工劳动的技术,是最原始的技术,表现为劳动者的技能,即手控制手工工具的能力,亦称手工技能。
这种原始技术本质上是人的体能。
人的体能有两种功能:一是改变物体状态的能力,即体力。
二是控制物体的能力,在手工劳动中就表现为控制手工工具的能力。
这就是最早的技术——体技或手技。
所有的技术都是人对自己的超越。
人的双手的动作不准确、不精确,而手工技术追求的就是一准二精。
这种准确性和精确性的提高,不是通过工具,而是通过劳动者的苦练得来的。
“熟能生巧”,这“巧”是手之巧,靠的是熟练。
手工技能是由双手的动作的准和精表现出来的,它在一定程度上超越了人的生理局限,提高了人的生理功能,是“生理性技术”。
手工技能很难用语言文字来表达,它本身也不是知识,也不需要知识作为前提条件。
它只可意会,不可言传。
别人要学习这种技能,主要靠动作的模仿和用心去领悟。
这种技术的传授必须面对面进行。
这种手工技能是劳动者的身体所具有的,存在于劳动者体内,离开了劳动者的双手,这种技能就不再存在。
河南省南阳一中2014届高三上学期第三次周考(11月)英语试题 Word版含答案

英语试题命题、校对: 尹丽丽宋榕叶时间:2013年11月23日温馨提醒:机读卡请从第21题开始涂,注意题号。
第I卷(选择题,共85分)第二部分: 英语知识运用(共两节,满分45分)第一节单项填空(共15小题,每小题1分,共15分)21. Cooking calls for ______ patience and time but it is______ enjoyable experience.A. a; theB. /; anC. the; anD. a; /22. He lost his job three years ago; _____, he was seriously injured in an accident last week.A. in the first placeB. worse stillC. on the contraryD. in other words23. ---Did he tell you the bad news?---Yes, but I’d rather he ____.A. didn’tB. haven’tC. hadn’tD. wouldn’t24. ---Have you moved to the new house?---Not yet. It _____ at the moment.A.is decoratedB. had been decoratedC. is being decoratedD. has been decorated25. After talking with his father for about half an hour, he felt better and began to know how to ____ this kind of situation.A. dealB. solve withC. handleD. settle down26. When the news came _____ the war broke out, he decided to serve in the army.A. sinceB. whichC. thatD. because27. The stadium _____ by workers now will be finished by the end of 2014.A. to buildB. builtC. to be builtD. being built28. ______side of the street is lined with different shops, ______of which sell electronic products.A. Both; bothB. Either; allC. Neither; eitherD. Either; both29. Only when the final examination was coming ____ he had quite a lot to do.A. he realizedB. he had realizedC. did he realizeD. had he realized30. After three hours’ drive, they finally arrived in _____ was called “ Dreamland”.A. whichB. whatC. whereD. that31. I realized the purpose of the whole experience: I needed to learn to open my heart and give love without requiring anything _____.A. by designB. in caseC. in returnD. as a consequence32.--- Our holiday cost a lot of money.---Did it? Well, that doesn’t matter____ you enjoyed yourselves.A. unlessB. as far asC. as long asD. until33. She got the photos of her son ______ up next to her desk so that she could see them whenever she missed him.A. to pinB. pinningC. being pinnedD. pinned34. Samuel survived when the car _____ he was a passenger in turned off the road and hit a tree.A. whereB. thatC. asD. why35. ---Say, Tom, will you come with me to the concert Saturday?---____, Bob, but I promised Lucy I’d go with her.A. With pleasureB. Go aheadC. Take it easyD. Thanks第二节完形填空(共20小题;满分30分)Michael O’Neil lived alone with his father, and the two of them had a very special relationship. The father believed in encouragement.Though Michael was the 36 of the class when he entered high school, his 37 continued to encourage him, but also made it very clear that he did not have to play 38 if he didn’t want to.However, Michael loved football and was 39 to try his best at every practice. All through high school, he never 40 a practice or a game, but remained a bench warmer(替补队员) all four years. His 41 father was always in the stands, with words of 42 for him.It was the end of the football season, and as Michael ran slowly onto the practice field shortly 43 the big final game, the coach met him with a telegram. Michael 44 the telegram and became deathly silent—his father died that morning, and the sad man left for 45 immediately.In the third quarter, when the team was ten points 46 , a silent young man eagerly 47 onto the sidelines. The coach and his players were shocked to see their team-mate back so 48 .“Coach, please let me 49 . I have to play today,” said Michael. Feeling sorry for him, the coach 50 . Before long, nobody could believe their eyes. This small bench warmer played like a(n) 51 . He helped his team win finally.When the team was cheering for their 52 , Michael was sitting in the corner all alone. The coach came to him and said, “You were fantastic! Tell me 53 you did it?”Tears in eyes, Michael said, “Well, you knew my dad died, but did you know he was blind?” He 54 a smile, “Dad came to all my games, but today was the first time he could 55 me play, and I wanted to show him I could do it!”36. A. strongest B. smallest C. biggest D. youngest37. A. coach B. players C. classmates D. father38. A. football B. games C. practices D. basketball39. A. required B. determined C. satisfied D. reminded40. A. failed B. lost C. missed D. won41. A. sympathetic B. upset C. faithful D. optimistic42. A. encouragement B. judgment C. wisdom D. amusement43. A. after B. since C. towards D. before44. A. went through B. turned over C. got over D. tore up45. A. school B. home C. field D. class46. A. ahead B. beside C. behind D. away47. A. walked B. ran C. stepped D. slipped48. A. quietly B. sadly C. punctually D. soon49. A. go B. fight C. work D. play50. A. left B. refused C. agreed D. cried51. A. star B. son C. amateur D. trainer52. A. victory B. award C. memorial D. unity53. A. why B. when C. where D. how54. A. spread B. skipped C. forced D. stopped55. A. hear B. see C. feel D. make第三部分阅读理解(共20小题;每小题2分,满分40分)第一节(共15小题;每小题2分,满分30分)阅读下列短文,从每题所给的四个选项(A.B.C和D)中,选出最佳选项,并在答题卡上将该项涂黑。
河南省南阳市2014届高三第三次联考(高考模拟)英语试题 Word版含答案[ 高考]
![河南省南阳市2014届高三第三次联考(高考模拟)英语试题 Word版含答案[ 高考]](https://img.taocdn.com/s3/m/21f77057b84ae45c3a358c1c.png)
河南省南阳市2014届高中毕业班第三次模拟考试英语第二部分:阅读理解(共两节,满分40分)第一节(共15小题;每小题2分,满分30分)阅读下列短文,从每题所给的四个选项(A、B、C和D)中,选出最佳选项,并在答题卡上将该项涂黑。
ATerrie Hall, a cancer patient who made great contributions to the national anti-smoking ad campaign, died at the age of 53.When she was a young woman Terrie started smoking in high school. “I thought it was a cool thing to do.” she said. The smoking caused throat cancer and oral cancer and the surgeries and radiation treatment took away her natural voice. But they also brought out a level of bravery and strength of character that amazed all of us.In a society where women are often judged by their looks, Terrie did not hide the scars on her face caused by smoking. The scars on her jaw where part of her jaw had been cut out and on her throat where her larynx(喉)had been removed, only made her more determined to speak, especially to young people at school. She wanted young people to know the truth about tobacco.She became known around the world through her participation in CDC’s smoking ad campaign. The ad where she showed how she got ready each day—putting on her wig(假发), putting her hands-free device in the hole in her throat—is hard to watch.Her video has become the most-viewed video ever on CDC’ YouTube pages with, over 2.5 million viewings, and has inspired more comments than any other CDC video. Her second ad, which touched on the fact that her grandchild never heard her precancerous voice, inspired thousands more to quit.Lisa Hancock, a mother of a five-year-old child and a smoker smoking one pack to two packs of cigarettes a day, said she had tried to quit many time, before, but seeing Terrie’s ad helped her realize that smoking really did cause other types of cancer besides lung cancer, and helped motivate her to quit for good health.Just a few weeks after the second ad, Terrie’s doctors, found her cancer caused by smoking bad spread to her brain. In her remaining days, she chose to keep fighting and keep working to save lives by showing the real consequences of cigarette smoking. She insisted on being filmed in hospital just two days before her death, sharing the final effects of Smoking on her body.The disease that finally took her life never touched her great spirit. That spirit, the selfless work she did and the tens of thousands of lives she saved are Terrie Hall’s great legacies.21. Terrie Hall earned the respect of the public ________.A. because she fought against cancer bravelyB. because she inspired people to quit smokingC. because she started the anti-smoking campaignD. because she was asked to be filmed in hospital22. Knowing she had cancer Terrie must be ______.A. disappointedB. confusedC. regretfulD. anxious23. What does the sixth paragraph imply?A. Terrie’s ad shook Lisa up.B. Lisa paid little attention to her kid.C. Lisa has smoked for two years.D. Terrie popularized the knowledge of cancer.24. What does the author think of Terrie ?A. She shouldn’t have been filmed in hospital.B. She must have moved the public deeply.C. She should have accepted the doctor’s advice.D. She must have caused a shock in the world.BA recent survey showed that doctors in America are warning of an obesity time bomb, when children who are already overweight grow up. So, what should we do? Exercise more? Eat less? Or both? The government feels it has to take responsibility for this expanding problem.The cheerful Mr. Pickwick, the hero of the novel by Charles Dickens, is seen in illustrations as someone who is plump and happy. In the 18th century paintings, beauty is equated (使…等同)with rounded bodies and soft curves. But nowadays being overweight is seen as indicating neither a cheerful character nor beauty but an increased risk of heart disease, stroke and diabetes.So what do you do? Diet? Not according to England’s chief medical officer, Sir Liam Donaldson. He says that physical activity is the key for reducing the risks of obesity, cancer and heart disease. And the Health Secretary John Reid even said that being inactive is as serious a risk factor in heart disease as smoking.So, having bought some cross trainers, how much exercise should you do? According to Sir Liam Donaldson, at least 30 minutes of moderate activity five days a week. Is going to the gym the answer? Luckily for those who think that running machines are boring, the Health Development Agency believes that physical activity that fits into people’s lives may be more effective. They suggest taking the stairs rather than the lilt, walking up escalators, playing active games with your children, dancing or gardening. And according to a sports psychologist, Professor Biddle, gyms “are not making the nation fit”, and may even cause harm.There’s new scientific evidence that too much exercise may actually be bad for you. Scientists at the University of Ulster have found that unsuitable exercise releases dangerous free radicals that can oppositely affect normal function in unfit people. The only people who should push their bodies to that level of exercise on a regular basis are trained athletes.So, should we f orget about gyms and follow some expert’s advice to reduce sedentary(久坐不动的)activities and increase exercise in our daily life? After all, getting off the bus a stop early and walking the rest of the way can’t do any harm! One final thought, how come past generations lacked gym facilities but were leaner and fitter than people today?25. Mr. Pickwick and the paintings of the 18th century are used as examples to show that _______.A. beauty should be overweightB. a fat man is usually a cheerful characterC. fatness was considered something good at one timeD. fatness leads to an increased risk of disease26. According to Sir Liam Donaldson, we should _______.A. go on a dietB. do regular physical activityC. give up smokingD. go to the gym27. The underlined phrase cross trainers probably refers to ________.A. people who help you do exerciseB. places where you can do exerciseC. a kind of shoesD. a form of vehicles28. What is the passage mainly about?A. how to keep fit and avoid fatness.B. increased risks for overweight people.C. the dangers of exercise in the gym.D. the benefit or a balanced diet.CThe brown widow spider became established in Southern California in early 2000 and has become part of the local spider family in urban Los Angeles and San Diego. The brown widow spider is continuing its expansion in Southern California and could possibly move northward into Central California.The brown widow is suspected to have evolved in Africa although it was first described-from South America, which adds confusion as to where it might have originated. It is a tropical and subtropical spider having established populations in Hawaii, Florida, parts of Australia, South Africa and Japan. In North America, the brown widow was restricted for many decades to the Florida peninsula. However, around the year 2000, it started showing up in other Gulf Coast states. Brown widows are now known from Texas to Georgia and South Carolina.The brown widow builds its web in protected sites around homes and in woody vegetation with branches. Some typical sites selected by brown widows for web building are empty containers such as buckets and nursery pots, mail boxes, entry way comers, under eaves, storage closets and garages, undercarriages of motor vehicles that are stationary for long periods, and the undersides of outdoor furniture. They choose places that are more exposed than sites chosen by black widows and therefore, appear to be at higher risk for interactions with humans as far as bites are concerned.One recent study demonstrates that the brown widow spider is less poisonous than other widow species. The reason for the weaker effect of brown widow bites on humans is possibly because the brown widow does not have much poison as its larger relatives, but it is really a threat to humans as to its poison. The two major symptoms or a brown widow bite were that the bite hurt when it was given and it left a red mark. These two symptoms are not much different from the bite of normal household spiders.There is no specific information regarding the control of brown widows by farm chemicals. Most current advice is what is used for controlling spiders in general. Therefore, most commercially available farm chemicals should work on brown widows. Avoiding a mess of the house and the garage should reduce nest sites for them. Also, one should store garage items in plastic bags where there might be interactions with spiders. These items include rarely worn garments such as gardening clothes and gloves, recreational items like sports equipment(i.e., baseball gloves)and other items where spiders can crawl up into holes where fingers can be inserted.29. The author wrote this article to ________.A. announce the result of a research on spidersB. introduce the ways to get rid of the spidersC. warn readers against the brown widowD. report a new finding of the widow species30. From the passage we can conclude that ________.A. the brown widow originated from AfricaB. brown widows are spreading northwardC. brown widows now can be found in all countriesD. there used to be no brown widows in North America31. The third paragraph is mainly about ________.A. the habitat preferences of the brown widowB. the characteristics of the brown widowC. the brown wido w’s web buildingD. the brown widow’s threaten to human beings32. It can be inferred that ________.A. brown widows can be killed with any farm chemicalsB. victims bitten by the brown widow needn’t see the doctorC. the brown widow has more poison than the black widowD. at present people can only control brown widow spidersD33. A student from Japan who has been studying in England for a year and intends to go tocollege in a few months will ________.A. get money if taking a first degree courseB. get money from LEA when finishing his courseC. be unable to get money from any LEAD. have to open a bank account before getting money34. A 31-year-old nurse wishes to qualify as a doctor at a university. She has worked since she was25. How much extra money will she get a year?A. None.B. £515.C. £155.D. £615.35. A big bank offers a new student special services because _______.A. they hope he’ll be a potential customerB. they charge students extra interestC. they know he can get money regularlyD. they need student accounts badly第二节(共5小题;每小题2分,满分10分))根据短文内容,从短文后的选项中选出能填入空白处的最佳选项。
河南省南阳市2014届高三第三次联考(高考模拟)理科数学试卷(带解析)

河南省南阳市2014届高三第三次联考(高考模拟)理科数学试卷1.设全集U 是实数集R ,集合2={|2}M x x x >,2N={|log (1)0}x x -≤,则(C M )NU 为( )A .{|12}x x <<B .{|12}x x ≤≤C .{|12}x x <≤D .{|12}x x ≤< 【答案】C 【解析】试题分析:∵22x x >,∴2x >或0x <,∴{|02}M x x x =<>或,∵2log (1)0x -≤,∴011x <-≤,∴12x <≤,∴{|12}N x x =<≤,∴(C M)N {|12}U x x =<≤.考点:1.一元二次不等式的解法;2.对数不等式的解法;3.集合的补集、交集运算. 2.设复数z 满足(1)32z i i +=-+(i 为虚数单位),则z 的实部是( ) A .1 B .2 C .3 D .4 【答案】A 【解析】试题分析:∵(1)32z i i +=-+,∴32113iz i i-+=-=+,∴z 的实部是1. 考点:1.复数的除法运算;2.复数的实部与虚部.3.等差数列{}n a 中,如果14739a a a ++=,36927a a a ++=,则数列{}n a 前9项的和为( )A .297B .144C .99D .66 【答案】C 【解析】试题分析:∵14739a a a ++=,36927a a a ++=,∴1474339a a a a ++==,413a =,3696327a a a a ++==,69a =,∴2d =-,119a =,∴998199(2)992S ⨯=⨯+⨯-=. 考点:1.等差数列的性质;2.等差数列的通项公式;3.等差数列的前n 项和公式. 4.下列命题中正确命题的个数是( )(1)命题“若2320x x -+=,则1x =”的逆否命题为“若1x ≠,则2320x x -+≠”; (2)设回归直线方程12y x ∧=+中,x 增加1个单位时,y 一定增加2个单位; (3)若p q ∧为假命题,则,p q 均为假命题;(4)对命题0:p x R ∃∈,使得20010x x ++<,则:p x R ⌝∀∈,均有210x x ++≥;(5)设随机变量ξ服从正态分布(0,1)N ,若(1)P p ξ>=,则1(10)2P p ξ-<<=-. A .2 B .3 C .4 D .5 【答案】B 【解析】试题分析:(1)正确;(2)设回归直线方程12y x ∧=+中,x 增加1个单位时,y 平均增加2个单位;(3)若p q ∧为假命题,则,p q 至少有一个是假命题;(4)正确;(5)正确. 考点:1.命题的否定;2.复合命题的真假判断;3.回归直线方程;4.正态分布;5.逆否命题. 5.已知三棱锥的俯视图与侧视图如图所示,俯视图是变长为2的正三角形,侧视图是有一条直角边为2的直角三角形,则该三棱锥的正视图可能为( )【答案】C 【解析】试题分析:由条件得直观图如图所示:正视图是直角三角形,中间的线是看不见的线PA 形成的投影为虚线.考点:三视图.6.一个算法的程序框图如图,则其输出结果是( )A .0 B.2 C.12+1【答案】B 【解析】 试题分析:由题意可知:23456782014sinsinsin sin sin sin sin sin sin444444444S πππππππππ=+++++++++234562510(sinsinsin sin sin sin )444444ππππππ=⨯++++++2=. 考点:1.程序框图;2.三角函数的周期性.7.若函数()2sin f x x ω=(0)ω>的图像在(0,2)π上恰有一个极大值和一个极小值,则ω的取值范围是( )A .3(,1]4B .5(1,]4C .34(,]45D .35(,]44【答案】D 【解析】试题分析:∵函数()2sin f x x ω=(0)ω>的图像在(0,2)π上恰有一个极大值和一个极小值, ∴35222πππω<≤,∴3544ω<≤. 考点:1.三角函数图像;2.函数的极值. 8.已知,[,]22ππαβ∈-且sin sin 0ααββ->,则下面结论正确的是( )A .αβ>B .0αβ+>C .αβ<D .22αβ> 【答案】D 【解析】试题分析:设()sin f x x x =,[,]22x ππ∈-,∴'cos sin cos (tan )y x x x x x x =+=+, 当[,0]2x π∈-时,'0y <,∴()f x 为减函数,当[0,]2x π∈时,'0y >,∴()f x 为增函数,且函数()f x 为偶函数,∵sin sin 0ααββ->,∴sin sin ααββ>,∴||||αβ>,∴22αβ>.考点:1.函数的单调性;2.函数的奇偶性.9.已知ABC ∆的重心为G ,内角A ,B ,C 的对边分别为a ,b ,c ,若30a G A b G B c G C++=,则角A 为( ) A .6πB .4πC .3πD .2π【答案】A 【解析】试题分析:∵303aGA bGB cGC ++=,∴30a GA bc GB ⎛⎫⎛⎫+-= ⎪ ⎪ ⎪ ⎪⎝⎭⎝⎭,∴0,033a cbc -=-=,∴,33a b ==,∴22222211cos 22c c c b c a A bc +-+-===,∴6A π=. 考点:1.向量的运算;2.余弦定理.10.动圆C 经过点(1,0)F ,并且与直线1x =-相切,若动圆C 与直线1y x =+总有公共点,则圆C 的面积( )A .有最大值8πB .有最小值2πC .有最小值3πD .有最小值4π 【答案】D 【解析】试题分析:设圆心为(,)a b ,半径为r ,|||1|r CF a ==+,即222(1)(1)a b a -+=+,即214a b =, ∴圆心为21(,)4b b ,2114r b=+,圆心到直线1y x =+的距离为22|1|14b b b d -+=≤+, ∴3)b ≤-或2b ≥,当2b =时,min 14124r =⨯+=,∴2min 4S r ππ==. 考点:1.点到直线的距离;2.圆与直线的位置关系. 11.已知函数|ln |,(0)()2ln ,()x x e f x x x e <≤⎧=⎨->⎩,若a ,b ,c 互不相等,且()()()f a f b f c ==,则a b c ++的取值范围为( )A .2(1,1)e e e +++ B .21(2,2)e e e++C .2)e +D .12)e e+【答案】B 【解析】试题分析:由已知和图像可知: 201a b e c e <<<<<<,∵ln ln a b -=,∴1ab =,∵ln 2ln b c =-,∴2bc e =,∴12a b c e e++>+,22a b c e ++<+,∴a b c ++的取值范围是21(2,2)e e e++.考点:1.函数图像;2.图像的交点问题.12.设实数x ,y 满足约束条件2208400,0x y x y x y -+≥⎧⎪--≤⎨⎪≥≥⎩,若目标函数z abx y =+(0,0a b >>)的最大值为8,则a b +的最小值为 . 【答案】4 【解析】试题分析:约束条件所表示的区域如图所示:目标函数z abx y =+在(1,4)A 处取得最大值,所以48ab +=,即4ab =,所以4a b +≥=,当且仅当a b =时取等号.考点:线性规划.13.设2(sin 12cos )2xa x dx π=-+⎰,则62((2)x ∙+的展开式中常数项是 .【答案】-332 【解析】 试题分析:∵2(sin 12cos )2xa x dx π=-+⎰(cos sin )20x x π=-+=,∴6262((2)(2)x x∙+=∙+,∵663166(2(1)rrrr r r r r T C C x ---+==- ∴常数项为55333662(1)22(1)332C C -+-=-. 考点:1.定积分;2.二项式定理.14.已知()f x 、()g x 都是定义在R 上的函数,()0g x ≠,''()()()()f x g x f x g x <,()()x f x a g x =,(1)(1)5(1)(1)2f fg g -+=-,则关于x 的方程2502abx ++=((0,1)b ∈)有两个不同实根的概率为 . 【答案】52【解析】试题分析:∵()()xf x ag x =,∴()()x f x a g x =,∵''()()()()f x g x f x g x <,∴''()()()ln 0()x x f x a a a g x ==<,即ln 0a <,即01a <<,又∵(1)(1)5(1)(1)2f fg g -+=-,∴152a a +=,即12a =,∵关于x的方程2502abx ++=((0,1)b ∈)有两个不同实根,∴2100ab ∆=->即205b <<, ∴22515p ==.考点:1.几何概型;2.导数的运算.15.在三棱锥S ABC -中,AB BC ⊥,AB BC ==2SA SC ==,二面角S AC B--的余弦值是,,,S A B C 都在同一球面上,则该球的表面积是 . 【答案】π6【解析】试题分析:取AC 中点D ,连接SD BD ,,∵AB BC ==,∴B D A C ⊥,∵2S A S C ==,∴SD AC ⊥,AC ⊥平面SDB .∴SDB ∠为二面角S AC B --.在ABC ∆中,AB BC ⊥,AB BC ==∴=2AC .取等边SAC ∆的中心E ,作EO ⊥平面SAC ,过D 作DO ⊥平面ABC ,O 为外接球球心,∴ED S AC B --的余弦值是cos EDO ∠=,2OD =,∴BO OA OS OC =====,∴O 点为四面体的外接球球心,其半径6π. 考点:三棱锥的外接球.16.设数列{}n a 的前n 项和为n S ,已知11a =,121n n S S n +=++ *()n N ∈, (1)求数列{}n a 的通项公式; (2)若1n n nn b a a +=-,数列{}n b 的前n 项和为n T ,*n N ∈,证明:2n T <.【答案】(1)()*12N n a nn ∈-=;(2)证明过程详见解析.【解析】试题分析:本题主要考查等比数列的通项公式、配凑法求通项公式、错位相减法求和等基础知识,考查学生分析问题解决问题的能力,考查转化能力和计算能力.第一问,已知条件中只有一个等式,利用1n n n S S a --=,用1n -代替式子中的n ,得到一个新的表达式,两个式子相减得到121+=+n n a a ,再用配凑法,凑出等比数列,求出数列{}n a 的通项公式;第二问,利用第一问的结论,先化简n b 表达式,再利用错位相减法求数列的前n 项和,最后的结果与2比较大小.试题解析:(Ⅰ)∵121++=+n S S n n ,当2≥n 时n S S n n +=-12∴121+=+n n a a 2分 ∴()1211+=++n n a a 即2111=+++n n a a (2≥n )又1,22s 1112==+=s a s ∴32=a ∴21112=++a a ∴nn a 21=+ 即()*12N n a nn ∈-= 6分 (Ⅱ)∵12-=nn a ∴11(21)(21)222n n n n n nn n nb ++===---- 8分∴n n n T 223222132++++=,132221222121++-+++=n n n n n T ∴22212)221212121(21132<--=-++++=-+n n n n n nn T 12分 考点:1 由n S 求n a ;2 配凑法求通项公式;3 等比数列的通项公式;4 错位相减法 17.某中学举行了一次“环保知识竞赛”活动,为了了解本次竞赛学生成绩情况,从中抽取了部分学生的分数(得分取正整数,满分为100分)作为样本(样本容量为n )进行统计,按照[50,60),[60,70),[70,80),[80,90),[90,100]的分组作出频率分布直方图,并作出样本分数的茎叶图(图中仅列出了得分在[50,60),[90,100]的数据)(1)求样本容量n 和频率分布直方图中x ,y 的值;(2)在选取的样本中,从竞赛成绩是80分以上(含80分)的同学中随机抽取3名同学到市政广场参加环保知识宣传的志愿者活动,设ξ表示所抽取的3名同学中得分在[80,90)的学生个数,求ξ的分布列及其数学期望【答案】(1)50,0.030,0.004n x y ===;(2)分布列详见解析,157E ξ=【解析】试题分析:本题主要考查茎叶图、频率分布直方图的读法、离散型随机变量的分布列和数学期望等基础知识,考查学生的读图能力,考查学生的分析问题解决问题的能力和计算能力 第一问,由茎叶图可知,[50,60)之间有8个分数,由频率分布直方图可知[50,60)的高为0 016,利用=频率高组距,=频数频率样本容量,可求出样本容量n 的值,而y 是[90,100]的高,茎叶图中[90,100]是2个成绩,所以利用上述那2个公式可求出y 的值,在频率分布直方图中,所有频率之和为1,可求出x 的值;第二问,利用上述2个公式可得出每一个区间范围内的人数,得出80分以上共7人,在7人中抽取3人在[80,90)中的人数分别为1,2,3,分别求出概率,列出分布列,利用1122n n E x p x p x p ξ=+++求出数学期望试题解析:(Ⅰ)由题意可知,样本容量8500.01610n ==⨯,20.0045010y ==⨯,0.10.0040.0100.0160.040.030x =----= 3分(Ⅱ)由题意可知,分数在[80,90)有5人,分数在[90,100]有2人,共7人 抽取的3名同学中得分在[80,90)的学生个数ξ的可能取值为1,2,3,则12523751(1)357C C P C ξ====,215237204(2)357C C P C ξ====,3537102(3)357C P C ξ==== 所以,ξ的分布列为所以,142151237777E ξ=⨯+⨯+⨯= 12分考点:1 频率分布直方图;2 茎叶图;3 离散型随机变量的分布列和数学期望18.如图,正方形ADEF 与梯形ABCD 所在的平面互相垂直,AD CD ⊥,//AB CD ,122AB AD CD ===,点M 在线段EC 上(除端点外)(1)当点M 为EC 中点时,求证://BM 平面ADEF ;(2)若平面BDM 与平面ABF 锥M BDE -的体积【答案】(1)证明过程详见;(2)43V =【解析】试题分析:本题主要考查线线平行、线线垂直、线面平行、二面角、三棱锥的体积等基础知识,考查学生的空间想象能力和推理论证能力,考查用空间向量法解立体问题,考查学生的计算能力 第一问,取N 为ED 中点,利用中位线得1//2MN DC ,而2DC AB =,所以//MN AB ,所以ABMN 为平行四边形,所以//BM AN ,所以利用线面平行的判定可得BM ∥平面ADEF ;第二问,用向量法解题,关键是建立空间直角坐标系,求出平面BDM 和平面ABF 的法向量,利用夹角公式求出cos ,OA n <>,从而求出λ的值,即点M 为EC 中点,所以利用等体积转化法求三棱锥B DEM 的体积试题解析:(1)证明 取DE 中点N ,连结,MN AN 在△EDC 中,,M N 分别为,EC ED 的中点,则MN ∥CD ,且12MN CD =由已知AB ∥CD ,12AB CD =, 因此,MN ∥AB ,且MN AB = 所以,四边形ABMN 为平行四边形 于是,BM ∥AN 又因为AN ⊂平面ADEF ,且BM ⊄平面ADEF , 所以BM ∥平面ADEF 6分(2)按如图建立空间直角坐标系,点D 与坐标原点O 重合设),,(z y x M ,则)2,,(-=z y x ,又)2,4,0(-=,设(01)EM EC λλ=<<,则λλ22,4,0-===z y x ,即)22,4,0(λλ-M设),,(111z y x =是平面BDM 的法向量,则02211=+=⋅y x ,0)22(411=-+=⋅z y λλ取11=x ,得λλ-=-=12,111z y ,即得平面BDM 的一个法向量为)12,1,1(λλ--= …… 10分 由题可知,)0,0,2(=OA 是平面ABF 的一个法向量因此,||1|cos ,|2||||OA n OA n OA n λ⋅<>====⋅,即点M 为EC 中点 此时,2=DEM S ∆,AD 为三棱锥DEM B -的高, 所以,=-BDE M V 342231=⋅⋅=-DEM B V ……… 12分 考点:1 线面平行的判定;2 向量法;3 三棱锥的体积 19.已知圆2214:5C x y +=,直线:(0)l y x m m =+>与圆1C 相切,且交椭圆22222:1(0)x y C a b a b+=>>于11,A B 两点,c 是椭圆的半焦距,c =(1)求m 的值;(2)O 为坐标原点,若11OA OB ⊥,求椭圆2C 的方程;(3)在(2)的条件下,设椭圆2C 的左右顶点分别为A ,B ,动点0020(,)(0)S x y C y ∈>,直线,AS BS 与直线3415x =分别交于M ,N 两点,求线段MN 的长度的最小值【答案】(1)5m =;(2)1422=+y x ;(3)min 1615MN =【解析】试题分析:本题主要考查圆的标准方程、椭圆的标准方程、直线的标准方程、直线与圆的位置关系、直线与椭圆的位置关系等基础知识,考查数形结合思想,考查转化能力和计算能力 第一问,利用直线与圆相切,利用圆心到直线的距离为半径,列出等式,求出m ;第二问,直线与椭圆相交,两方程联立,消参,得到关于x 的方程,利用两根之和,两根之积和向量的数量积联立,得到2a 和2b ,从而求出椭圆的方程;第三问,设直线AS 的斜率,设出直线AS 的方程,直线与椭圆联立,消参,利用两根之积,得到0x 的值,则可以用k 表示S 坐标,利用B 点坐标,求出直线BS 的方程,直线BS 的方程与直线3415x =联立,求出N 点坐标,利用两点间距离公式,得到||MN 的表达式,利用均值定理求出最小值试题解析:(Ⅰ)直线)0(:>+=m m x y l 与圆54:221=+y x C 相切,=m =分 (Ⅱ) 将5102:+=x y l 代入得 1:22222=+by a x C 得:0585104)(2222222=-+++b a a x a x a b ①设),,(),,(221111y x B y x A 则)(252540)5102)(5102(;)(558,)(5104222222121222222122221b a b a b x x y y a b b a a x x a b a x x +-=++=+-=+-=+因为05)(4,222211=-+⇒⊥b a b a OB OA ②由已知224,3b a b c ==代人(2)4,10)1(2222==⇒=-a b b b所以椭圆2C 的方程为1422=+y x 8分 (Ⅲ)显然直线AS 的斜率存在,设为k 且0>k 则)2(:+=x k y AS依题意)1564,1534(k M ,由⎪⎩⎪⎨⎧=++=14)2(22y x x k y 得:041616)41(2222=-+++k x k x k设),(00y x S 则)2(,418241416)2(00220220+=+-=⇒+-=-⋅x k y kk x k k x 即 )414,4182(222k k k k S ++-,又B (2,0)所以,41200kx y k BS -=-= BS :)2(41--=x k y 由15161511564215115640),151-,1534(1534)2(41=⋅≥+=⇒>⇒⎪⎪⎩⎪⎪⎨⎧=--=k k k k MN k k N x x ky 所以81=k 时:1516min=MN 12分 考点:1 点到直线的距离;2 向量的数量积;3 韦达定理;4 均值定理 20.已知函数()ln(1)f x a x =+,21()2g x x x =-,a R ∈ (1)若1a =-,求曲线()y f x =在0x =处的切线方程;(2)若对任意的[0,)x ∈+∞,都有()()f x g x ≥恒成立,求a 的最小值;(3)设()(1)p x f x =-,0a >,若11(,)A x y ,22(,)B x y 为曲线()y p x =的两个不同点,满足120x x <<,且312(,)x x x ∃∈,使得曲线()y P x =在33(,())x f x 处的切线与直线AB 平行,求证:1232x x x +<【答案】(1)0x y +=;(2)1;(3)证明过程详见解析 【解析】 试题分析:第一问,当1a =时,先求出()f x 的解析式,对()f x 求导,将0x =代入到'()f x 中得到切线的斜率,将0x =代入到()f x 中得到切点的纵坐标,最后用点斜式写出切线方程;第二问,本问是恒成立问题,先转化成21ln(1)02a x x x +-+≥恒成立,即构造函数求函数()h x 的最小值大于等于0即可,对()h x 求导对参数a 进行讨论,分1a ≥和1a <,求导,利用导数求函数的最值,判断是否符合题意;第三问,先利用已知条件求出()p x 解析式,求出直线AB 的斜率,通过对()p x 求导,求出曲线在3x x =处的切线的斜率,由于两直线平行,所以两斜率相等,由于0a >,所以()p x 在定义域内单调递减,用分析法得欲证1232x x x +<,需证明''123()()2x x p x p +<,通过变形得2212112(1)ln 1x x x x x x ->+,即(1)l n 2(t t t +>-,构造新函数()q t ,通过求导判断函数的单调性和最值,只需证明最小值大于0即可试题解析:(1)(0)0f =,斜率'(0)1k f ==-,所以,曲线()y f x =在0x =处的切线方程为0x y += 2分 (2) 21ln(1)2a x x x +≥-恒成立21ln(1)02a x x x ⇔+-+≥恒成立 令21()ln(1)2h x a x x x =+-+,0x ≥,2'1()111a x a h x x x x +-=-+=++,0x ≥,(ⅰ)若1a ≥,则'()0h x ≥恒成立,∴函数()h x 在[0,)+∞为单调递增函数,()(0)h x h ≥恒成立,又∵(0)0h =,∴1a ≥符合条件(ⅱ)若1a <,由'()0h x =,可得21x a =-,解得x =和x =(舍去)当x ∈时,'()0h x <;当)x ∈+∞时,'()0h x >;∴()h x h =极小值(0)0,()0h h x ∴<=≥这与恒成立矛盾综上,∴≥,1a a 的最小值为1 7分 (Ⅲ)()(1)ln p x f x a x =-=,2121ln ln AB a x a x k x x -=-又∵'()a p x x =,∴'33()a p x x =,∴21213ln ln a x a x a x x x -=- 由'()ap x x=,0a >,易知其在定义域内为单调递减函数欲证1232x x x +<⇔证明''123()()2x xp x p +< 即212112ln ln 2a x a x a x x x x ->-+,变形可得:22211211212(1)2()ln 1x x x x x x x x x x -->=++令21x t x =,1t >,原不等式等价于2(1)ln 1t t t ->+,等价于(1)ln 2(1)t t t +>-构造函数()(1)ln 2(1)q t t t t =+--,1t >则'1()ln 1q t t t =+-,1t >,令1()ln 1r t t t=+-,1t >,当1t >时,'22111()0t r t t t t-=-=>, ∴'()q t 在(1,)+∞上为单调递增函数,''()(1)0q t q >= ∴()q t 在(1,)+∞上为单调递增函数, ∴()(1)0q t q >=,∴()0q t >在(1,)+∞上恒成立 ∴(1)ln 2(1)t t t +>-成立,∴1232x x x +<得证 考点:1 利用导数研究函数的单调性;2 利用导数求函数的极值和最值 21.如图,直线AB 过圆心O ,交O 于F (不与B 重合),直线l 与O 相切于C ,交AB 于E ,且与AF 垂直,垂足为G ,连结AC求证:(1)BAC CAG ∠=∠;(2)2AC AE AF =∙【答案】(1)证明过程详见解析;(2)证明过程详见解析 【解析】 试题分析:本题主要考查以圆为背景考查角相等的证明及相似三角形等基础知识,考查学生的转化能力和推理论证能力 第一问,通过AB 为直径,所以ACB ∠为直角,又因为GC 切⊙O 于C ,所以GCA ABC ∠=∠,所以得证;第二问,利用EC 与⊙O 相切,得出ACE AFC ∠=∠,所以三角形相似得ACF ∆与AEC ∆相似,利用相似三角形的性质,得出比例值,化简即可,得证试题解析:(1)连结BC ,∵AB 是直径, ∴090ACB ∠=,∴090ACB AGC ∠=∠= ∵GC 切O 于C ,∴GCA ABC ∠=∠∴BAC CAG ∠=∠ 5分(2)连结CF ,∵EC 切O 于C , ∴ACE AFC ∠=∠ 又BAC CAG ∠=∠, ∴ACF AEC ∆∆∴AC AF AE AC=,∴2AC AE AF =∙ 10分考点:1 圆的切线的性质;2 相似三角形22.已知曲线C 的极坐标方程为2sin 4cos ρθθ=,直线l 的参数方程为cos 1sin x t y t αα=⎧⎨=+⎩(t为参数,0απ≤<)(1)把曲线C 的极坐标方程化为直角坐标方程,并说明曲线C 的形状; (2)若直线l 经过点(1,0),求直线l 被曲线C 截得的线段AB 的长【答案】(1)x y 42=,曲线C 是顶点为O (0,0),焦点为F(1,0)的抛物线;(2)8 【解析】试题分析:本题主要考查极坐标方程与直角坐标方程的互化,直线的参数方程,韦达定理等基础知识,考查学生的转化能力和计算能力 第一问,利用极坐标与直角坐标的互化公式cos x ρθ=,sin y ρθ=进行互化,并写出图形形状;第二问,由直线l 的参数方程得出直线过(0,1),若还过(1,0),则34πα=,则直线l 的方程可进行转化,由于直线与曲线C 相交,所以两方程联立,得到关于t 的方程,设出A ,B 点对应的参数12,t t ,所以12||||AB t t =-,利用两根之和,两根之积进行转化求解试题解析:(1)曲线C 的直角坐标方程为x y 42=,故曲线C 是顶点为O (0,0),焦点为F(1,0)的抛物线; 5分 (2)直线l 的参数方程为⎩⎨⎧+==ααsin 1cos t y t x ( t 为参数,0≤α<π) 故l 经过点(0,1);若直线l 经过点(1,0),则43πα=∴直线l 的参数方程为⎪⎪⎩⎪⎪⎨⎧+=+=-==t t y t t x 22143sin 12243cos ππ(t 为参数) 代入x y 42=,得02262=++t t设A 、B 对应的参数分别为21,t t ,则2,262121=-=+t t t t∴21221214)(t t t t t t AB -+=-==8 10分考点:1极坐标与直角坐标的互化;2直线的参数方程;3直线与曲线的位置关系 23.设()||,f x x a a R =-∈(1)当13x -≤≤时,()3f x ≤,求a 的取值范围;(2)若对任意x R ∈,()()12f x a f x a a -++≥-恒成立,求实数a 的最小值 【答案】(1)[0,2];(2)14【解析】试题分析:本题主要考查绝对值不等式的解法、不等式的性质等基础知识,考查学生分析问题解决问题的能力,考查学生的转化能力和计算能力 第一问,利用绝对值不等式的解法,先解出||3x a -≤的解,再利用[1,3]-是[3,3]a a -+的子集,列不等式组,求解;第二问,先利用不等式的性质求出()()f x a f x a -++的最小值2||a ,将恒成立的表达式转化为2||2a a ≥-,再解绝对值不等式,求出a 的取值范围试题解析:(1)()||3f x x a =-≤,即33a x a -≤≤+ 依题意,3133a a -≤-⎧⎨+≥⎩,由此得a 的取值范围是[0,2] 5分(2)()()|2||||(2)|2||f x a f x a x a x x a x a -++=-+≥--= 当且仅当(2)0x a x -≤时取等号解不等式2||12a a ≥-,得14a ≥ 故a 的最小值为1410分 考点:1 绝对值不等式的解法;2 集合的子集关系;3 不等式的性质;4 恒成立问题。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
河南省南阳一中2014届高三上学期第三次周考(11月)文科数学试题时间:2013年11月23日一、选择题(每小题5分,共60分)1、若全集为实数集R ,集合A =12{|log (21)0},R x x C A ->则=( )A .1(,)+∞B .(1,)+∞ C .1[0,][1,)+∞D .1(,][1,)-∞+∞3、函数x e y sin =(π-≤x ≤π)的大致图象为 ( )4、已知函数4sin(2)y x π=-,则其图象的下列结论中,正确的是( )A .关于点()8,1π-中心对称 B .关于直线8x π=轴对称 C .向左平移8π后得到奇函数 D .向左平移8π后得到偶函数5、过椭圆22221x y a b+=(0a b >>)的左焦点1F 作x 轴的垂线交椭圆于点P ,2F 为右焦点,若1260F PF ∠=,则椭圆的离心率为 ( )A .2 B .3C .12D .136、由等式43223144322314)1()1()1()1(b x b x b x b x a x a x a x a x ++++++++=++++ 定义映射43214321),,,(b b b b a a a a f +++→,则→)1,2,3,4(f ( )A .10B .7C . -1D .07、已知A ,B ,C ,D 是函数sin()(0,0)2y x πωω=+Φ><Φ<一个周期内的图象上的四个点,如图所示,(,0),6A π-B 为y 轴上的点,C 为图象上的最低点,E 为该函数图象的一个对称中心,B 与D 关于点E 对称,CD 在x 轴上的投影为12π,则,ωΦ的值为( )A .2,3πω=Φ= B .2,6πω=Φ=C .1,23πω=Φ=D .1,26πω=Φ=8、在△ABC 中,AB=4,∠ABC=30°,D 是边BC 上的一点,且⋅=⋅, 则AD 的值等于( )A .—4B .0C .4D .8 9、若20πα<<,02-<<βπ,31)4cos(=+απ,33)2-4cos(=βπ,则=+)2cos(βα( ) A. B.C.D.-10、若在区域34000x y x y +-≤⎧⎪≥⎨⎪≥⎩内任取一点P ,则点P 落在单位圆221x y +=内的概率( ) A .332π B .325π C .163π D .165π11、已知ABC ∆的三边长成公差为2的等差数列,且最大角的正弦值为23,则这个三角形的周长是( )A .18B .21C .24D .1512、设函数1(1)|-1|)=1(=1)x x f x x ⎧≠⎪⎨⎪⎩(,若关于x 的方程2[()]+()+c=0f x bf x 有三个不同的实数根123,,x x x ,则222123++x x x 等于( )A . 13B . 5C . 223c +2cD . 222b +2b第Ⅱ卷(非选择题 共90分)二、填空题(每题5分,共20分)13、已知倾斜角为α的直线l 与直线220x y -+=平行,则tan 2α的值为14、已知函数()f x 是R 上的奇函数,若对于0x ≥,都有()2()f x f x +=,[)()()20,2,log 1x f x x ∈=+当时时,())2014(2013f f +-的值为15、某校对高三年级的学生进行体检,现将高三男生的体重(单位:kg)数据进行整理后分成六组,并绘制频率分布直方图(如右图).已知图中从左到右第一、第六小组的频率分别为0.16,0.07,第一、第二、第三小组的频率成等比数列,第三、第四、第五、第六小组的频率成等差数列,且第三小组的频数为100,则该校高三年级的男生总数为16、已知各项均为正数的等比数列{}n a 满足7652a a a =+,若存在两项,m n a a 使得1144,a m n=+则的最小值为 三、解答题(本大题共7题,共70分)17、(本小题满分12分)在△ABC 中,a ,b ,c 分别是角A ,B ,C 的对边长,已知22223b c a ac --=。
(Ⅰ)求cosB 及tan 2A C +的值;(Ⅱ)若b =,△ABC的面积为sinA +sinC 的值18. (本小题满分12分)如图,从A 1(1,0,0),A 2(2,0,0),B 1(0,1,0,)B 2(0,2,0),C 1(0,0,1),C 2(0,0,2)这6个点中随机选取3个点。
(1) 求这3点与原点O 恰好是正三棱锥的四个顶点的概率; (2) 求这3点与原点O 共面的概率。
19、(本小题满分12分)已知函数3()f x x mx =-+在(0,1)上是增函数, (Ⅰ)实数m 的取值集合为A ,当m 取集合A 中的最小值时,定义数列{}n a 满足13,a =且0,n a >1n a +=,求数列{a n }的通项公式;(Ⅱ)若n n b na =,数列{}n b 的前n 项和为n S ,求证:34n S >.20、(本小题满分12分)如图,一个半圆和长方形组成的铁皮,长方形的边AD 为半圆的直径,O 为半圆的圆心,AB =1,BC =2,现要将此铁皮剪出一个等腰三角形PMN ,其底边MN ⊥BC . (Ⅰ)设∠MOD =30°,求三角形铁皮PMN 的面积; (Ⅱ)求剪下的铁皮三角形PMN 的最大面积21、(本小题满分12分)已知函数f (x )=-13x 3+a2x 2-2x (a ∈R ).(1)当a =3时,求函数f (x )的单调区间; (2)若对于任意x ∈[1,+∞)都有f ′(x )<2(a -1)成立,求实数a 的取值范围;(3)若过点⎝⎛⎭⎫0,-13可作函数y =f (x )图象的三条不同切线,求实数a 的取值范围.请考生在第(22)、(23)两题中任选一题作答。
注意:只能做所选定的题目。
如果多做,则按所做的第一个题目计分。
22、(本小题满分10分)如图,AB 是圆O 的直径,C 是半径OB 的中点, D 是OB 延长线上一点,且BD=OB ,直线MD 与圆O 相交于点M 、T (不与A 、B 重合),DN 与圆O 相切于点N ,连结MC ,MB ,OT .(I )求证:DC DO DM DT ⋅=⋅;(II ) 若 60=∠DOT ,试求BMC ∠的大小.23、(本小题满分10分) 已知函数()1f x x =- (I )解不等式: 1()(1)2f x f x ≤+-≤; (II )若0>a ,求证:()()f ax af x -≤()f a .南阳市一中2013年秋期第三次周考文科数学试题答案一、选择题二、填空题 13、43 14、-1 15、400 16、32三、17、解:(Ⅰ)由b 2-c 2=a 2-23ac ,得cos B =a 2+c 2-b 22ac =13. 由0<B <π知sin B =1-cos 2B =223. tan A +C 2=tan π-B 2=sin (π2-B 2)cos (π2-B 2)=cos B 2sin B 2=2cos 2B 22sin B 2cosB 2=1+cos B sin B= 2. (5分)(Ⅱ)由12acsin B =2,ac =3,由b 2-c 2=a 2-23ac ,得(a +c)2=b 2+83ac =16,即a +c =4.由正弦定理得sin A +sin C =a +c b ×sin B =43.(10分)19、解:(1)由题意得f′(x )=﹣3x 2+m , ∵f (x )=﹣x 3+mx 在(0,1)上是增函数,∴f′(x )=﹣3x 2+m≥0在(0,1)上恒成立, 即m≥3x 2,得m≥3,-----------------------------2分 故所求的集合A 为[3,+∞);所以m=3,∴f′(x )=﹣3x 2+3,∵,n a >0,∴=3n a ,即=3,∴数列{n a }是以3为首项和公比的等比数列,故nn a 3=; -------------------------------6分 (2)由(1)得,bn=na n =nn 3⋅, ∴Sn=1•3+2•32+3•33+…+n•3n ① 3Sn=1•32+2•33+3•34+…+n•3n +1 ② ①﹣②得,﹣2Sn=3+32+33+…+3n ﹣n•3n +1=﹣13+⋅n n化简得,Sn=>.----------------------------12分20、(本小题满分12分)21、【解析】(1)当a =3时,f (x )=-13x 3+32x 2-2x ,得f ′(x )=-x 2+3x -2.因为f ′(x )=-x 2+3x -2=-(x -1)(x -2),所以当1<x <2时,f ′(x )>0,函数f (x )单调递增; 当x <1或x >2时,f ′(x )<0,函数f (x )单调递减.故函数f (x )的单调递增区间为(1,2),单调递减区间为(-∞,1)和(2,+∞).(2)方法一:由f (x )=-13x 3+a 2x 2-2x ,得f ′(x )=-x 2+ax -2.因为对于任意x ∈[1,+∞)都有f ′(x )<2(a -1)成立, 即对于任意x ∈[1,+∞)都有-x 2+ax -2<2(a -1)成立,即对于任意x ∈[1,+∞)都有x 2-ax +2a >0成立.令h (x )=x 2-ax +2a ,要使h (x )对任意x ∈[1,+∞)都有h (x )>0成立,必须满足Δ<0,或⎩⎨⎧Δ≥0,a2≤1,h即a 2-8a <0或⎩⎪⎨⎪⎧a 2-8a ≥0,a2≤1,1+a >0.所以实数a 的取值范围为(-1,8).方法二:由f (x )=-13x 3+a2x 2-2x ,得f ′(x )=-x 2+ax -2.因为对于任意x ∈[1,+∞)都有f ′(x )<2(a -1)成立,即对于任意x ∈[1,+∞)都有f ′(x )max <2(a -1).因为f ′(x )=-⎝⎛⎭⎫x -a 22+a 24-2,其图象开口向下,对称轴为x =a2.①当a 2<1,即a <2时,f ′(x )在[1,+∞)上单调递减,所以f ′(x )max =f ′(1)=a -3.由a -3<2(a -1),得a >-1,此时-1<a <2;②当a 2≥1,即a ≥2时,f ′(x )在⎣⎡⎦⎤1,a 2上单调递增,在⎝⎛⎭⎫a 2,+∞上单调递减,所以f ′(x )max =f ′⎝⎛⎭⎫a 2=a 24-2.由a24-2<2(a -1),得0<a <8,此时2≤a <8.综上①②可得,实数a 的取值范围为(-1,8).(3)设点P ⎝⎛⎭⎫t ,-13t 3+a 2t 2-2t 是函数y =f (x )图象上的切点,则过点P 的切线的斜率为k =f ′(t )=-t 2+at -2,所以过点P 的切线方程为y +13t 3-a 2t 2+2t =(-t 2+at -2)(x -t ).因为点⎝⎛⎭⎫0,-13在切线上,所以-13+13t 3-a 2t 2+2t =(-t 2+at -2)(0-t ),即23t 3-12at 2+13=0.若过点⎝⎛⎭⎫0,-13可作函数y =f (x )图象的三条不同切线,则方程23t 3-12at 2+13=0有三个不同的实数解.令g (t )=23t 3-12at 2+13,则函数y =g (t )与t 轴有三个不同的交点.令g ′(t )=2t 2-at =0,解得t =0或t =a 2.因为g (0)=13,g ⎝⎛⎭⎫a 2=-1243+13,所以g⎝⎛⎭⎫a 2=-124a 3+13<0,即a >2. 所以实数a 的取值范围为(2,+∞).22、(1)证明:因MD 与圆O 相交于点T ,由切割线定理DM DT DN ⋅=2,DA DB DN ⋅=2,得DA DB DM DT ⋅=⋅,设半径OB=)0(>r r ,因BD=OB ,且BC=OC=2r , 则233r r r DA DB =⋅=⋅,23232r rr DC DO =⋅=⋅, 所以.DC DO DM DT ⋅=⋅------------------5分(2)由(1)可知,DC DO DM DT ⋅=⋅,且CDM TDO ∠=∠, 故DTO ∆∽CM D ∆,所以DMC DOT ∠=∠;根据圆周角定理得,DMB 2DOT ∠=∠,则.30=∠BMC --------10分。
