Interacting electrons in disordered wires Anderson localization and low-temperature transpo

合集下载

Magnetic-field-dependent quasiparticle energy relaxation in mesoscopic wires

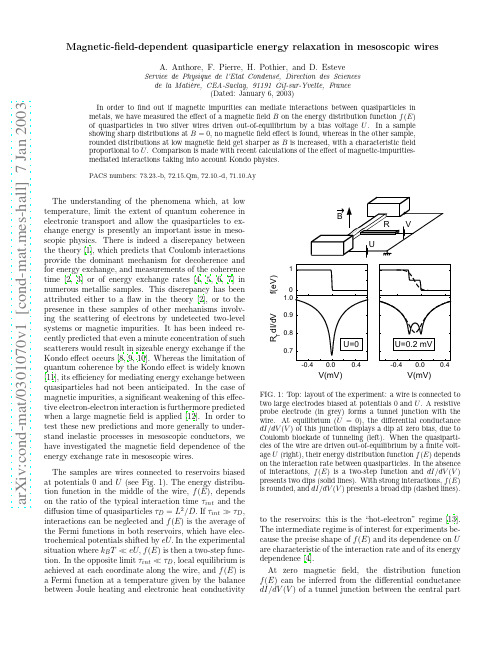
a r X i v :c o n d -m a t /0301070v 1 [c o n d -m a t .m e s -h a l l ] 7 J a n 2003Magnetic-ﬁeld-dependent quasiparticle energy relaxation in mesoscopic wiresA.Anthore,F.Pierre,H.Pothier,and D.EsteveService de Physique de l’Etat Condens´e ,Direction des Sciences de la Mati`e re,CEA-Saclay,91191Gif-sur-Yvette,France(Dated:January 6,2003)In order to ﬁnd out if magnetic impurities can mediate interactions between quasiparticles in metals,we have measured the eﬀect of a magnetic ﬁeld B on the energy distribution function f (E )of quasiparticles in two silver wires driven out-of-equilibrium by a bias voltage U .In a sample showing sharp distributions at B =0,no magnetic ﬁeld eﬀect is found,whereas in the other sample,rounded distributions at low magnetic ﬁeld get sharper as B is increased,with a characteristic ﬁeld proportional to U .Comparison is made with recent calculations of the eﬀect of magnetic-impurities-mediated interactions taking into account Kondo physics.PACS numbers:73.23.-b,72.15.Qm,72.10.-d,71.10.AyThe understanding of the phenomena which,at low temperature,limit the extent of quantum coherence in electronic transport and allow the quasiparticles to ex-change energy is presently an important issue in meso-scopic physics.There is indeed a discrepancy between the theory [1],which predicts that Coulomb interactions provide the dominant mechanism for decoherence and for energy exchange,and measurements of the coherence time [2,3]or of energy exchange rates [4,5,6,7]in numerous metallic samples.This discrepancy has been attributed either to a ﬂaw in the theory [2],or to the presence in these samples of other mechanisms involv-ing the scattering of electrons by undetected two-level systems or magnetic impurities.It has been indeed re-cently predicted that even a minute concentration of such scatterers would result in sizeable energy exchange if the Kondo eﬀect occurs [8,9,10].Whereas the limitation of quantum coherence by the Kondo eﬀect is widely known [11],its eﬃciency for mediating energy exchange between quasiparticles had not been anticipated.In the case of magnetic impurities,a signiﬁcant weakening of this eﬀec-tive electron-electron interaction is furthermore predicted when a large magnetic ﬁeld is applied [12].In order to test these new predictions and more generally to under-stand inelastic processes in mesoscopic conductors,we have investigated the magnetic ﬁeld dependence of the energy exchange rate in mesoscopic wires.The samples are wires connected to reservoirs biased at potentials 0and U (see Fig.1).The energy distribu-tion function in the middle of the wire,f (E ),depends on the ratio of the typical interaction time τint and the diﬀusion time of quasiparticles τD =L 2/D.If τint ≫τD ,interactions can be neglected and f (E )is the average of the Fermi functions in both reservoirs,which have elec-trochemical potentials shifted by eU.In the experimental situation where k B T ≪eU,f (E )is then a two-step func-tion.In the opposite limit τint ≪τD ,local equilibrium is achieved at each coordinate along the wire,and f (E )is a Fermi function at a temperature given by the balance between Joule heating and electronic heat conductivityT d I /d VRV(mV)V(mV)f (e V )FIG.1:Top:layout of the experiment:a wire is connected totwo large electrodes biased at potentials 0and U .A resistive probe electrode (in grey)forms a tunnel junction with the wire.At equilibrium (U =0),the diﬀerential conductance dI/dV (V )of this junction displays a dip at zero bias,due to Coulomb blockade of tunneling (left).When the quasiparti-cles of the wire are driven out-of-equilibrium by a ﬁnite volt-age U (right),their energy distribution function f (E )depends on the interaction rate between quasiparticles.In the absence of interactions,f (E )is a two-step function and dI/dV (V )presents two dips (solid lines).With strong interactions,f (E )is rounded,and dI/dV (V )presents a broad dip (dashed lines).to the reservoirs:this is the “hot-electron”regime [13].The intermediate regime is of interest for experiments be-cause the precise shape of f (E )and its dependence on U are characteristic of the interaction rate and of its energy dependence [4].At zero magnetic ﬁeld,the distribution function f (E )can be inferred from the diﬀerential conductance dI/dV (V )of a tunnel junction between the central partof the wire and a superconducting(aluminum)probe electrode biased at potential V[4].In magneticﬁelds larger than the criticalﬁeld B c∼0.1T of the supercon-ducting electrode,another method is required.Here,we have taken advantage of the nonlinearity of the current-voltage characteristic of a tunnel junction placed in series with a resistance R.When both electrodes of the junc-tion are in the normal state and at thermal equilibrium, the diﬀerential conductance dI/dV(V)displays a dip at V=0(see Fig.1),due to the Coulomb blockade of tun-neling[14].Assuming that the two electrodes have dif-ferent distribution functions f and f ref,the diﬀerential conductance reads:dIR TdE f(E) dεP(ε)(1)×∂2π e J(t)+iεt/ the probability for an electronto tunnel through the barrier while releasing to the envi-ronment an energyε,J(t)= dωR K e−iωt−1ω2Re[Z(ω)]V (mV)V (mV)R T d I /d VV (mV)FIG.3:Magnetic ﬁeld eﬀect in sample #1:diﬀerential con-ductance dI/dV (V )at U =0.1,0.2and 0.3mV,for B ranging (from bottom to top)from 0.3to 1.5T by steps of 0.3T.Suc-cessive curves have been vertically oﬀset by steps of 0.033,for clarity.at U =0.2mV ,and 1.5T at U =0.25mV (not shown),hence presenting a linear increase with U.The compari-son of the raw data on sample #1and sample #2in Fig.2already allows to conclude that sample #1presents an extra interaction which can be strongly reduced by ap-plying a magnetic ﬁeld.We now compare the experimental data with theoret-ical predictions.The distribution function is calculated by solving the stationary Boltzmann equation in the dif-fusive regime [17,18]:1∂x 2=−I in coll (x,E,{f })+I outcoll (x,E,{f })(2)where I in coll and I outcoll are the rates at which quasiparticles are scattered in and out of a state at energy E by inelastic processes.Assuming that the dominant inelastic process is a two-quasiparticle interaction which is local on the scale of variations of the distribution function,I in coll = d εd E ′K (ε)f x E +ε(1−f x E )f x E ′(1−f x E ′−ε)(3)where the shorthand f x E stands for f (x,E ).The out-collision term I outcoll has a similar form.The kernel func-tion K (ε)is proportional to the averaged squared in-teraction between two quasiparticles exchanging an en-ergy ε.Coulomb interactions lead,in diﬀusive wires,toK (ε)=κ/ε3/2[1],where κ=(π 2 3/2νF S )−1with νF the density of states at the Fermi level [19].The B =0data for sample #2can be well ﬁt using this term with κ=0.12ns −1meV −1/2,of the same order of magni-tude as the theoretical value 0.07ns −1meV −1/2[20],and a term of lesser importance describing phonon emission [21].The B =0data for sample #1can be ﬁt simi-larly,with κ=2.4ns −1meV −1/2,however the reduction of the energy exchange rate with B indicates that an extra process is present at B =0.We have in the fol-lowing ﬁxed κto the best ﬁt value obtained for the large ﬁeld,low U data,where the B −dependent interactionhas essentially vanished:κ=0.5ns −1meV −1/2[20].The remaining part of the energy exchange rate was ﬁt with the theory of G¨o ppert et al.[12,22],which accounts for the eﬀective interaction in the presence of a concentra-tion c of spin-14Cu samples,a material in which the oxyde at the sur-face of theﬁlms was found to cause dephasing at low temperature[24].Data on energy exchange[25]could also beﬁt with the theory of G¨o ppert et al[12],using T K=300mK,c=4.8ppm,g=2.3,νJ=0.1,on top ofa Coulombic term with intensityκ=0.5ns−1meV−1/2[22].This result gives evidence that the anomalous inter-actions observed in many Cu wires at B=0[4,7]are also due to magnetic impurities.Here also,measurements of the phase coherence time[7]are explained by signiﬁ-cantly smaller impurity concentrations(∼0.3ppm).This repeated discrepancy on the concentrations deduced from the two types of measurements remains an open problem. From an experimental point of view,a more quantitative test of theory could be obtained in samples with added, identiﬁed magnetic impurities at a known concentration [27].To conclude,we have found that anomalous energy exchange rates between quasiparticles were strongly re-duced by the application of a magneticﬁeld.Moreover, the energy and magneticﬁeld dependence of the exchange rate can be accurately accounted for by the presence of a small concentration of Kondo magnetic impurities[12]. It is worthwhile to compare this result with recent mea-surements on Aharonov-Bohm rings,which show that the small phase-coherence times found at B=0were in-creased in aﬁnite magneticﬁeld[28].All these measure-ments indicate that the presence of very dilute magnetic impurities is a very plausible candidate to explain both extra dephasing and extra energy exchange observed in many mesoscopic samples.We acknowledge the technical help of P.Orﬁla,fruit-ful discussions and correspondence with G.G¨o ppert,H. Grabert and N.Birge,and permanent input from M.De-voret,P.Joyez,C.Urbina and D.Vion.[1]For a review,see B.L.Altshuler and A.G.Aronov,in Electron-Electron Interactions in Disordered Systems, Ed.A.L.Efros and M.Pollak(Elsevier Science Publish-ers B.V.,1985).[2]P.Mohanty,E.M.Q.Jariwala and R.A.Webb,Phys.Rev.Lett.78,3366(1997).[3]For a review,see J.J.Lin and J.P.Bird,J.Phys.:Con-dens.Matter.14,R501(2002).[4]H.Pothier et al.,Phys.Rev.Lett.79,3490(1997).[5]F.Pierre et al.,J.Low Temp.Phys.118,437(2000).[6]F.Pierre et al.,in Kondo Eﬀect and Dephasing in Low-Dimensional Metallic Systems,Ed.V.Chandrasekhar,C.Van Haesendonck,and A.Zawadowski(Kluwer Aca-demic Publishers,Dordrecht,The Netherlands,2001), p.119(cond-mat/0012038).[7]F.Pierre,Ann.Phys.(Paris)26N4(2001).[8]A.Kaminski and L.I.Glazman,Phys.Rev.Lett.86,2400(2001).[9]G.G¨o ppert,H.Grabert,Phys.Rev.B64,033301(2001).[10]J.Kroha and A.Zawadowski,Phys.Rev.Lett.88,176803(2002).[11]C.Van Haesendonck,J.Vranken,Y.Bruynseraede,Phys.Rev.Lett.58,1968(1987).[12]G.G¨o ppert et al.,Phys.Rev.B66,195328(2002).[13]A.H.Steinbach,J.M.Martinis,and M.H.Devoret,Phys.Rev.Lett.76,3806(1996).[14]For a review,see G.-L.Ingold and Yu.Nazarov,in SingleCharge Tunneling,Ed.H.Grabert and M.H.Devoret (Plenum Press,New York,1992).[15]M.Tinkham,in Introduction to Superconductivity,Ed.Mc Graw-Hill(1985),section2-10.5.[16]Because the signal to noise ratio is not large enough,nu-merical deconvolution of the dI/dV(V)curves proved to be unreliable.In particular,the preliminary f(E)curves in[25]and in[12]were artiﬁcially rounded.We prefer to present here raw data.[17]V.I.Kozub and A.M.Rudin,Phys.Rev.B52,7853(1995).[18]K.E.Nagaev,Phys.Lett.A169,103(1992);Phys.Rev.B52,4740(1995).[19]A.Kamenev and A.Andreev,Phys.Rev.B60,2218(1999).[20]In other experiments with silver wires[5],theﬁt value ofκwas found to be between3and10times the theoretical one[7].This scatter in the intensity of the interaction is presently not understood.[21]F.Pierre et al.,Phys.Rev.Lett.86,1078(2001).Theamplitude of the electron-phonon interactions is set toa value deduced from weak localization data:κph=8ns−1meV−3.When taking this term into account,the data of[5]are wellﬁt by Coulomb interactions,see[7].A slight heating of the environment with U was also taken into account in theﬁts.[22]In Ref.[12],the agreement with experiment was poor fortwo reasons:ﬁrst,the deconvolved data are artiﬁcially rounded;second,the eﬀect of usual Coulombic interac-tions have not been taken into account in the calculation.[23]The dI/dV(V)are slightly asymetric because the mea-suring probe is out of the center of the wire by0.01L.Note that the increase of the interaction rate at inter-mediate magneticﬁelds[25],when the Zeeman splitting of the impurity states is smaller than eU,is not visible because f(E)is already close to a Fermi distribution at B=0.This experiment is much more sensitive to the decrease of the exchange rate,which leads to a sharper structure in f(E).[24]J.Vranken,C.Van Haesendonck,and Y.Bruynseraede,Phys.Rev.B37,8502(1988).[25]A.Anthore et al.,in Electronic Correlations:FromMeso-to Nano-Physics,Ed.T.Martin,G.Montam-baux and J.Trˆa n Thanh Vˆa n(EDP Sciences,2001) [cond-mat/0109297][26]M.B.Maple,Magnetism,edited by H.Suhl(Academic,New York,1973),Vol.5.[27]In practice,we have found that tunnel junctions with asilver electrode evolve,at room temperature,to an open circuit within a few hours,hence forbidding implantation of magnetic impurities.[28]F.Pierre and N.O.Birge,Phys.Rev.Lett.89,206804(2002).。

绝缘体与安德森绝缘体

Thouless’ picture
A very important step to modern theory
G e 2 g ( E F ) DLd 2
e 2 hD N ( EF ) 2 h L
dimensionless
Thouless energy
ET
hD h 2 L T
2 , is level spacing, 2 is for spin 2e 2 ET E G~ g~ T h N ( EF ) ~
Question: Is the presence of electronic band gap a necessary and/or sufficient condition for the formation of insulators?
Answer: It is neither necessary nor sufficient. Counter examples: Anderson insulators: insulating states without band gap (but with a mobility gap), so that it not necessary. Superconductors: there is a electronic band gap (superconducting gap) like insulators due to electron pairing, but current can flow without resistance. Since the systems support collective excitations beside single-electronic excitations. The super-current in the system is a collective excitations.

大三英语下翻译

Unit11. The devices,called defibrillators, fire an electrical shock to jolt the heart back into abnormalrhythm if it starts to beat in a disordered way that can cause sudden death.若心脏跳动失常，则有可能导致猝死。

这时，这种叫做心脏除颤器的装置，能电击心脏，使之恢复正常心律。

In people who truly need them ,for conditions that can fatally disrupt heart rhythm , defibrillators can be life-saving.对于那些确实需要除颤器的病人来说，这种疾病会使心脏节律遭受致命的破坏，除颤器可以挽救他们的生命。

2. Professional societies set guidelines that specify when the defibrillators should be used,based on studies showing which patients they help.基于除颤器对哪些患者有帮助的研究，职业协会制定操作指南，详细说明什么时候可以使用它。

To find out if doctors have been complying ,researchers examined the records of 111707 people who received the implants at 1227 hospitals in the United States from January 2006to June 2009 .The records were part of a national registry ,and the National Heart ,Lung and Blood Institute paid for the study .为了弄清楚医生是否在遵守这些指导方针，研究人员对111707名病人的医疗档案进行了检查，这些病人在2006年1月至2009年6月之间在美国的1227家医院植入了除颤器。

Direct-current nanogenerator driven by ultrasonic waves

Phys. Rev. Lett. 63, 2508 (1989). 4. T. J. Gramila, J. P. Eisenstein, A. H. MacDonald,
L. N. Pfeiffer, K. W. West, Phys. Rev. Lett. 66, 1216 (1991). 5. U. Sivan, P. M. Solomon, H. Shtrikman, Phys. Rev. Lett. 68, 1196 (1992). 6. M. P. Lilly, J. P. Eisenstein, L. N. Pfeiffer, K. W. West, Phys. Rev. Lett. 80, 1714 (1998). 7. J. G. S. Lok et al., Phys. Rev. B 63, 041305 (2001). 8. A. G. Rojo, J. Phys. Condens. Matter 11, R31 (1999). 9. D. Snoke, Science 298, 1368 (2002). 10. R. Pillarisetty et al., Phys. Rev. B 71, 115307 (2005). 11. M. Yamamoto, M. Stopa, Y. Tokura, Y. Hirayama, S. Tarucha, Science 313, 204 (2006). 12. B. N. Narozhny, I. L. Aleiner, Phys. Rev. Lett. 84, 5383 (2000). 13. N. A. Mortensen, K. Flensberg, A.-P. Jauho, Phys. Rev. B 65, 085317 (2002). 14. I. V. Lerner, Phys. Lett. A 133, 253 (1988).

博士自我介绍4篇

博士自我介绍4篇good morning, dear professors:it’s my honor to introduce myself. my name is xxx, i am fromxxcountyxxprovince, december xxxx i was born in a poor family, and my parents are farmers, i love and respect them very much. we were delight with my becoming a fresh man in september 1997. luckily, i was permitted to be a graduate student after 4 years colorful life on campus. i received my bachelor degreeXX inxx institute of science and technology, then a master degreeXX inxx university of science and technology. for those 7 years my major was die design. before i received my master degree, i had done the subject of xxxxxxxxxxx. for the past 3 years, i have been inxxxxxxcollege, where i have been and still am a teacher. i teach students machine design etc. i have published more than 10 first-author papers.自我介绍的第一句话，很关键，不用说的很复杂。

安德森先生的生平英文小作文

安德森先生的生平英文小作文Philip Anderson was born in Indianapolis and spent his childhood in Urbana, Illinois. He graduated from Urbana High School in 1940. He then went to Harvard University to complete his bachelor's degree and entered graduate school. Study physics under the tutelage of John Van Fleck. Anderson spent time at the U.S. Naval Research Laboratory during the war. From 1949 to 1984, Anderson worked at Bell Laboratories in New Jersey and extensively studied many problems in condensed matter physics. During this time he discovered the concept of Anderson localization; wrote the Anderson Hamiltonian to describe electrons in transition metal systems. In addition he advised particle physicists to look for the mechanism that produces the mass of particles (later called the Higgs mechanism); developed computational methods in the BCS theory of superconductors. In 1963, Anderson was elected to the American Academy of Arts and Sciences. Anderson was a professor of theoretical physics at the University of Cambridge from 1967 to 1975. In 1977 he was awarded the Nobel Prize in Physics together with Neville Mott and John Van Fleck for "fundamental theoreticalinvestigations of the electronic structure of magnetism and disordered systems". This research provided a theoretical basis for the technology of switching and memory in electronic components, and made important contributions to the later development of computers. In 1982 he received the National Medal of Science. Since 1984, he has retired from Bell Labs to chair the Joseph Henry Professor of Physics at Princeton University.。

电子显微镜检查在呼吸道感染病原学诊断中的意义

•6 •国际儿科学杂志2021年1月第48卷第1期Int J Pediatr.Jan 2021，Vol.48，No. 1•综述.电子显微镜检查在呼吸道感染病原学诊断中的意义汪亚南（综述）曹玲（审校）首都儿科研究所附属儿童医院呼吸内科，北京100020通信作者：曹玲，Email:caoling9919@ 163. com【摘要】电子显微镜技术在医学领域发挥着越来越重要的作用，常用于各系统的超微结构观察、机制研究和疾病的临床诊断等，其中在呼吸道病原学诊断中的作用不容忽视，但其相关的综述较少。

利用电子显微镜可以准确观察呼吸道病原体的形态学特征，并对新发呼吸道感染病原体进行初步的分类筛选。

但由于设备昂贵、技术难度大、操作复杂等因素，电子显微镜技术很少应用于常规的病原学诊断，而更多的用于实验室基础研究。

该文综述了电子显微镜检査在呼吸道病原学诊断方面的优缺点及意义。

【关键词】电子显微镜；诊断；呼吸道病原基金项目：北京市医院管理中心儿科学科协同发展中心专项经费资助项目（XTCX201821)DOI ：10.3760/cma.j.issn.1673-4408. 2021.01.002The significance of electron microscopy in the etiological diagnosis of respiratory tract infectionWang Yanan, Cao LingDepartment of Respiratory Medicine, Children's Hospital Affiliated to the Capital Institute o f Pediatrics, Beijing100020, ChinaCorresponding author：Cao Ling，Email：caoling9919@ 163. com【A bstract】At present，electron microscopy has played a more and more important role in the field ofmedicine. It is often used in the ultrastructure observation of various systems, mechanism research and clinicaldiagnosis of diseases. The role of electron microscopy in the etiological diagnosis of respiratory tract infectioncannot be ignored, but its related review is less. By using electron microscopy, the moqDhological characteristics of respiratory tract pathogens can be accurately observed and the new respiratory tract infection pathogenscan be classified and screened. In addition, through the combination of samples with patients' serum and observation of immuno-electron microscopy, the host response to pathogens can also be understood. However, dueto the high equipment requirements, technical difficulties，complex operation and other factors, electron microscopy is rarely used in conventional etiological diagnosis, and more used in laboratory basic research. In thispaper, the advantages, disadvantages and significance of electron microscopy in the etiological diagnosis of respiratory tract are reviewed.【Key words 】Electron microscopy; Diagnosis ；Respiratory tract pathogenFund program ：Special Fund Support Project of Pediatrics Collaborative Development Center of BeijingHospital Management Center (XTCX201821)DOI ：10. 3760/cma. j. issn. 1673-4408. 2021.01.002呼吸道感染是一种常见的感染性疾病，在人群中广泛存在。

The Physics of Correlated Electron Systems

The Physics of Correlated ElectronSystems在现实生活中，电子是常见的物质，我们每天都在使用电子设备，比如手机、电脑等等。

然而，当电子聚集在一起时，会出现一些奇特的物理现象，这些现象被称为“相关电子系统”的物理现象。

相关电子系统指的是多个电子相互耦合形成的量子系统，它们的性质被电子之间的相互作用所决定，因此其性质常常比单个电子更为复杂和有趣。

在“相关电子系统”中，电子之间存在着相互作用，这种相互作用可以理解为是各种微小的相互作用力在作用。

这些微小的力导致电子之间发生相互作用，从而改变电子的行为。

例如，在磁场作用下，存在一种称为“霍尔效应”的现象，这时电子会产生横向的电压，这种现象是由于不同电子在磁场中的受力不同导致的。

此外，还存在许多在相关电子系统中特有的现象，比如超导、量子霍尔效应、玻色-爱因斯坦凝聚等等。

其中，超导是相关电子系统中最具代表性的现象之一。

超导是指在特定条件下，某些物质的电阻突然消失，更为重要的是，这种物质的电导率会变得极高，达到无限大。

这种现象的发现在20世纪初期引起了许多科学家的极大兴趣，并催生了一场对新物理学的热潮。

目前，虽然超导现象的机理仍然不是十分清楚，但我们已经发现，电子之间的相互作用是超导现象的重要原因之一。

量子霍尔效应是另一个相关电子系统中的重要现象。

它是指在二维电子系统中，当磁场作用下，电子在垂直于磁场方向的电场作用下产生的电流只存在于边缘区域，而在中心区域电流为零。

这种行为进一步证明了电子之间相互作用的重要性，同时也验证了量子力学对物理学的重要贡献。

此外，在相关电子系统中，还有一种非常有趣的现象称为玻色-爱因斯坦凝聚。

在经典物理学中，玻色和爱因斯坦提出了一个关于粒子性质的假设，他们认为，具有相同自旋的一组粒子，可以处于同一个量子状态中，形成一种新的量子态，这种新态被称为玻色-爱因斯坦凝聚态。

在实验中，我们可以将冷却气体中的玻色子降温到绝对零度附近，将其束缚在一个非常小的区域内，这时玻色子会在这个区域中形成一个集体，出现一系列固有的线性和非线性的性质。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

lem, which is also of direct experimental relevance; see,
e.g., Refs. [6] and [7, 8], where the crossover from WL to
SL with lowering T was studied for 1D and 2D systems,
interaction) will be discussed in the end. At suﬃciently high T , the conductivity σ(T ) ≃ σD +
cluded that transport is of VRH character. These results are, however, in conﬂict with the argument [13] – sup-
ported by our analysis – that elementary hops in the low-T limit are forbidden for d < 3 even for the case of long-range (1/r) Coulomb interaction, since energy con-
PACS numbers: 72.20.-i, 72.15.Rn, 71.30.+h, 73.63.-b
In a pathbreaking paper [1] Anderson demonstrated
that a quantum particle may become localized by a ran-
dom potential. In particular, in non-interacting systems
of one-dimensional (1D) or two-dimensional (2D) geom-
etry even weak disorder localizes all electronic states [2], thus leading to the exactly zero conductivity, σ(T ) = 0, whatever temperature T . A non-zero σ(T ) in such sys-
arXiv:cond-mat/0506411v1 [cond-mat.dis-nn] 16 Jun 2005
Interacting electrons in disordered wires: Anderson localization and low-T transport
I.V. Gornyi1,∗, A.D. Mirlin1,2,†, and D.G. Polyakov1,∗ 1Institut fu¨r Nanotechnologie, Forschungszentrum Karlsruhe, 76021 Karlsruhe, Germany 2Institut fu¨r Theorie der kondensierten Materie, Universit¨at Karlsruhe, 76128 Karlsruhe, Germany
For the case of electron-phonon scattering the an-
swer is well known. The conductivity is then governed
by Mott’s variable-range hopping (VRH) [4], yielding σ(T ) ∝ exp{−(T0/T )µ} with µ = 1/(d+1), where d is the spatial dimensionality. In the presence of a long-range
tems may only occur due to inelastic scattering processes
leading to dephasing of electrons. Two qualitatively dif-
ferent sources of dephasing are possible: (i) scattering of
phonon coupling is negligibly weak and the only source
of the inelastic scattering is the e-e interaction? Our pur-
pose here is to solve this long-standing fundamental prob-
where no mobility edge exists, activation to which otherwise might give σ(T ) = 0. If neither VRH nor activation,
then what?
Let us now specify the model. We consider a manychannel weakly disordered wire, so that the relevant length scales satisfy kF−1 ≪ l ≪ ξ, where kF is the Fermi momentum, l the mean free path, and ξ ∼ πνD the localization length (ν is the density of states per unit length and D the diﬀusion constant) [15, 16]. The corresponding energy scales are the Fermi energy EF , the elastic scattering rate τ −1, and the level spacing in the localization volume, ∆ξ = 1/νξ, with EF ≫ τ −1 ≫ ∆ξ. We will assume a short-range interaction U (r − r′) be-
respectively. For deﬁniteness, we concentrate on the case
of a many-channel 1D system with a short-range interac-
tion. Our results are, however, more general (including
weak-localization (WL) correction to the Drude conduc-
tivity. This correction behaves as ln τφ in 2D and as τφ1/2 in quasi-1D (many-channel wire) systems [3], and thus
(Dated: May 20, 2005)
We study transport of interacting electrons in a low-dimensional disordered system at low temperature T . In view of localization by disorder, the conductivity σ(T ) may only be non-zero due to electron-electron scattering. For weak interactions, the weak-localization regime crosses over with lowering T into a dephasing-induced “power-law hopping”. As T is further decreased, the Anderson localization in Fock space crucially aﬀects σ(T ), inducing a transition at T = Tc, so that σ(T < Tc) = 0. The critical behavior of σ(T ) above Tc is ln σ(T ) ∝ −(T − Tc)−1/2. The mechanism of transport in the critical regime is many-particle transitions between distant states in Fock space.
single-channel wires, 2D systems, Coulomb interaction),
as we discuss in the end of the paper.
It was proposed in [9] that the e-e interaction by it-
self is suﬃcient to induce VRH at low T . This idea was
Coulomb interaction, the Coulomb gap in the tunneling
density
of
states
modiﬁes
the
VRH].
But what is the low-T behavior of σ(T ) if the electron-
servation cannot be respected when an electron attempts a real transition by exciting an electron-hole pair [14].
The situation is particularly interesting in 1D and 2D,