公司理财第九版中文答案
中央财经大学公司理财中文版第九版第二章答案
第2章会计报表与现金流1.是非题：所有的资产在付出某种代价的情况下都具有流动性。
请解释。
答：正确。
所有资产都可以以某种价格转换为现金。
然而，当我们提到流动性资产的时候，一个附加的假设变得很重要，这个假设是资产可以以市场价值或接近市场价值的价格转换成现金。
2.为什么标准的利润表上列示的收入和成本不代表当期实际的现金流入和现金流出？答：财务会计里面的确认原则和匹配原则要求将收入和产生收入相关的费用在收入过程实际完成的时候就记录下来，而不必等到收到现金或支付账单的时候。
我们要注意到这种方式并不必然是正确的，主要取决于会计师的选择。
3.在会计现金流量表上，最后一栏表示什么？这个数字对于分析一家公司有何用处？答：最后一栏的数据显示了资产负债表上现金余额的变化。
既然如此，它对于分析一个公司来说并不是一个有用的数据。
4.财务现金流量表与会计现金流量表有何不同？哪个对于公司分析者更有用？答：主要的不同在于对利息费用的处理，会计现金流量表将利息视为经营现金流，而财务现金流量将其视为融资现金流。
会计现金流量的逻辑是，既然利息列支在利润表上，而利润表显示的是一定时期企业的经营状况，因此利息也就是经营性的现金流。
而事实上，利息是一种融资费用，是公司选择债务融资还是股权融资的结果，我们将在以后的章节中有更为详细的讲解。
相比两种现金流量表，财务现金流量表以其正确对待利息费用而成为分析公司业绩的更恰当的方法。
5.按照会计规定，一家公司的负债有可能超过资产，所有者权益为负，这种情况在市场价值上有没有可能发生？为什么？答：市场的价值不可能为负。
假设有一股股票，价格为负的20美元，这意味着如果你买入100股，你不仅得到了股票，而且还得到了一1200美元的现金支票，那么你会想买入多少股呢？更一般的情形是，根据公司破产和个人破产法，个人或公司的净资产不能为负，这意味着从市场价值的角度看，负债不可能超过资产。
6.为什么说在一个特定的期间资产的现金流量为负不一定不好？答：例如，对于一个正在迅速扩的成功企业来说，它的资本性支出将会很大，这可能会导致出现负的现金流。
(公司理财)公司理财中文版第九版第三章答案
第三章财务报表分析与长期财务规划1.由于不同行业的公司在财务比率上存在很大的差异，财务比率本身提供的信息有限，分析公司的财务比率有两种基本方法：时间趋势分析法和同类公司分析。
为什么这些方法会有用呢？每种方法能够告诉你哪些关于公司财务健康状况的信息？答：时间趋势分析描绘了公司财务状况随时间而变化的图景。
通过公司本身的跨期比较分析，财务经理可以评价公司的经营、融资或投资活动的某些方面是否已经发生了变化。
同类公司比较分析涉及到将某一特定公司的财务比率和经营绩效与同一行业或业务相同的其他公司进行比较。
通过同类公司比较分析，财务经理可以评价公司的经营、融资或投资活动的某些方面是否越出常规，从而为调整这些比率采取恰当的行动提供指南，如果这种调整合适的话。
两种方法都从财务的视角研究一个公司究竟有什么不同，但两种方法都不能告诉我们这种不同是积极正面的还是消极负面的。
例如，假设一个公司的流动比率是随时间而提高，这可能意味着该公司在过去一直面临流动性问题，一直在纠正这些问题；或者它也可能意味着该公司已经在管理流动资产和负债方面变得更低效。
同类公司比较分析中也存在类似的争论。
一个公司的流动比率低于同类公司，也许是它在管理流动资产和负债方面更具效率，也可能是它面临流动性问题。
没有哪种方法告诉我们某个比率是好还是坏。
两种方法只是表明有些东西存在差异，并告诉我们去哪里寻找。
2.所谓的“同店销售”是像沃尔玛和希尔斯之类的十分分散的公司的一项重要指标，顾名思义，分析同店销售就是比较同样的店铺或餐馆在两个不同的时间点上的销售额。
为什么公司总是关注同店销售而不是总销售？答：如果一家公司通过开设新店来不断增长，那么可以推测其总收入将上升。
比较两个不同时点的总销售额可能会误导。
同店销售方法只看在特定时期开设的店铺的销售额控制了这一误差。
3.为什么多数长期财务计划都从销售预测开始？或者说，为什么未来销售额是关键？答：理由是，最终，消费是商业背后的驱动力。
公司理财 课后答案 第九版
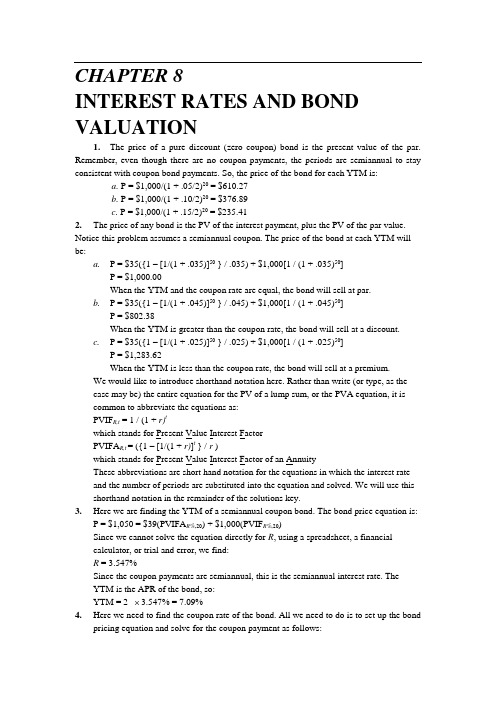
CHAPTER 8INTEREST RATES AND BOND VALUATION1.The price of a pure discount (zero coupon) bond is the present value of the par. Remember, even though there are no coupon payments, the periods are semiannual to stay consistent with coupon bond payments. So, the price of the bond for each YTM is:a. P = $1,000/(1 + .05/2)20 = $610.27b. P = $1,000/(1 + .10/2)20 = $376.89c. P = $1,000/(1 + .15/2)20 = $235.412.The price of any bond is the PV of the interest payment, plus the PV of the par value. Notice this problem assumes a semiannual coupon. The price of the bond at each YTM will be:a.P = $35({1 – [1/(1 + .035)]50 } / .035) + $1,000[1 / (1 + .035)50]P = $1,000.00When the YTM and the coupon rate are equal, the bond will sell at par.b.P = $35({1 – [1/(1 + .045)]50 } / .045) + $1,000[1 / (1 + .045)50]P = $802.38When the YTM is greater than the coupon rate, the bond will sell at a discount.c.P = $35({1 – [1/(1 + .025)]50 } / .025) + $1,000[1 / (1 + .025)50]P = $1,283.62When the YTM is less than the coupon rate, the bond will sell at a premium.We would like to introduce shorthand notation here. Rather than write (or type, as the case may be) the entire equation for the PV of a lump sum, or the PVA equation, it is common to abbreviate the equations as:PVIF R,t = 1 / (1 + r)twhich stands for Present Value Interest FactorPVIFA R,t= ({1 – [1/(1 + r)]t } / r )which stands for Present Value Interest Factor of an AnnuityThese abbreviations are short hand notation for the equations in which the interest rate and the number of periods are substituted into the equation and solved. We will use this shorthand notation in the remainder of the solutions key.3.Here we are finding the YTM of a semiannual coupon bond. The bond price equation is:P = $1,050 = $39(PVIFA R%,20) + $1,000(PVIF R%,20)Since we cannot solve the equation directly for R, using a spreadsheet, a financialcalculator, or trial and error, we find:R = 3.547%Since the coupon payments are semiannual, this is the semiannual interest rate. TheYTM is the APR of the bond, so:YTM = 2 3.547% = 7.09%4.Here we need to find the coupon rate of the bond. All we need to do is to set up the bondpricing equation and solve for the coupon payment as follows:P = $1,175 = C(PVIFA3.8%,27) + $1,000(PVIF3.8%,27)Solving for the coupon payment, we get:C = $48.48Since this is the semiannual payment, the annual coupon payment is:2 × $48.48 = $96.96And the coupon rate is the annual coupon payment divided by par value, so:Coupon rate = $96.96 / $1,000 = .09696 or 9.70%5.The price of any bond is the PV of the interest payment, plus the PV of the par value.The fact that the bond is denominated in euros is irrelevant. Notice this problem assumes an annual coupon. The price of the bond will be:P = €84({1 – [1/(1 + .076)]15 } / .076) + €1,000[1 / (1 + .076)15]P = €1,070.186.Here we are finding the YTM of an annual coupon bond. The fact that the bond is denominated in yen is irrelevant. The bond price equation is:P = ¥87,000 = ¥5,400(PVIFA R%,21) + ¥100,000(PVIF R%,21)Since we cannot solve the equation directly for R, using a spreadsheet, a financial calculator, or trial and error, we find:R = 6.56%Since the coupon payments are annual, this is the yield to maturity.7.The approximate relationship between nominal interest rates (R), real interest rates (r),and inflation (h) is:R = r + hApproximate r = .05 –.039 =.011 or 1.10%The Fisher equation, which shows the exact relationship between nominal interest rates, real interest rates, and inflation is:(1 + R) = (1 + r)(1 + h)(1 + .05) = (1 + r)(1 + .039)Exact r = [(1 + .05) / (1 + .039)] – 1 = .0106 or 1.06%8.The Fisher equation, which shows the exact relationship between nominal interest rates,real interest rates, and inflation, is:(1 + R) = (1 + r)(1 + h)R = (1 + .025)(1 + .047) – 1 = .0732 or 7.32%9. The Fisher equation, which shows the exact relationship between nominal interest rates,real interest rates, and inflation, is:(1 + R) = (1 + r)(1 + h)h = [(1 + .17) / (1 + .11)] – 1 = .0541 or 5.41%10.The Fisher equation, which shows the exact relationship between nominal interest rates,real interest rates, and inflation, is:(1 + R) = (1 + r)(1 + h)r = [(1 + .141) / (1.068)] – 1 = .0684 or 6.84%11.The coupon rate, located in the first column of the quote is 6.125%. The bid price is:Bid price = 119:19 = 119 19/32 = 119.59375% $1,000 = $1,195.9375The previous day’s ask price is found by:Previous day’s asked price = Today’s asked price – Change = 119 21/32 – (–17/32) = 120 6/32The previous day’s price in dollars was:Previous day’s dollar price = 120.1875% $1,000 = $1,201.87512.This is a premium bond because it sells for more than 100% of face value. The current yield is:Current yield = Annual coupon payment / Asked price = $75/$1,347.1875 = .0557 or 5.57%The YTM is located under the “Asked yield” column, so the YTM is 4.4817%.The bid-ask spread is the difference between the bid price and the ask price, so:Bid-Ask spread = 134:23 – 134:22 = 1/32Intermediate13. Here we are finding the YTM of semiannual coupon bonds for various maturity lengths.The bond price equation is:P = C(PVIFA R%,t) + $1,000(PVIF R%,t)Miller Corporation bond:P0 = $45(PVIFA3.5%,26) + $1,000(PVIF3.5%,26) = $1,168.90P1 = $45(PVIFA3.5%,24) + $1,000(PVIF3.5%,24) = $1,160.58P3 = $45(PVIFA3.5%,20) + $1,000(PVIF3.5%,20) = $1,142.12P8 = $45(PVIFA3.5%,10) + $1,000(PVIF3.5%,10) = $1,083.17P12= $45(PVIFA3.5%,2) +$1,000(PVIF3.5%,2) = $1,019.00P13= $1,000Modigliani Company bond:P0 = $35(PVIFA4.5%,26) + $1,000(PVIF4.5%,26) = $848.53P1 = $35(PVIFA4.5%,24) + $1,000(PVIF4.5%,24) = $855.05P3 = $35(PVIFA4.5%,20) + $1,000(PVIF4.5%,20) = $869.92P8 = $35(PVIFA4.5%,10) + $1,000(PVIF4.5%,10) = $920.87P12= $35(PVIFA4.5%,2) +$1,000(PVIF4.5%,2) = $981.27P13= $1,000All else held equal, the premium over par value for a premium bond declines as maturity approaches, and the discount from par value for a discount bond declines as maturity approaches. This is called “pull to par.” In both cases, the largest percentage price changes occur at the shortest maturity lengths.Also, notice that the price of each bond when no time is left to maturity is the par value, even though the purchaser would receive the par value plus the coupon payment immediately. This is because we calculate the clean price of the bond.14.Any bond that sells at par has a YTM equal to the coupon rate. Both bonds sell at par, sothe initial YTM on both bonds is the coupon rate, 8 percent. If the YTM suddenly rises to 10 percent:P Laurel= $40(PVIFA5%,4) + $1,000(PVIF5%,4) = $964.54P Hardy= $40(PVIFA5%,30) + $1,000(PVIF5%,30) = $846.28The percentage change in price is calculated as:Percentage change in price = (New price – Original price) / Original price∆P Laurel% = ($964.54 – 1,000) / $1,000 = –0.0355 or –3.55%∆P Hardy% = ($846.28 – 1,000) / $1,000 = –0.1537 or –15.37%If the YTM suddenly falls to 6 percent:P Laurel= $40(PVIFA3%,4) + $1,000(PVIF3%,4) = $1,037.17P Hardy= $40(PVIFA3%,30) + $1,000(PVIF3%,30) = $1,196.00∆P Laurel% = ($1,037.17 – 1,000) / $1,000 = +0.0372 or 3.72%∆P Hardy% = ($1,196.002 – 1,000) / $1,000 = +0.1960 or 19.60%All else the same, the longer the maturity of a bond, the greater is its price sensitivity to changes in interest rates. Notice also that for the same interest rate change, the gain froma decline in interest rates is larger than the loss from the same magnitude change. For aplain vanilla bond, this is always true.15.Initially, at a YTM of 10 percent, the prices of the two bonds are:P Faulk= $30(PVIFA5%,16) + $1,000(PVIF5%,16) = $783.24P Gonas= $70(PVIFA5%,16) + $1,000(PVIF5%,16) = $1,216.76If the YTM rises from 10 percent to 12 percent:P Faulk= $30(PVIFA6%,16) + $1,000(PVIF6%,16) = $696.82P Gonas= $70(PVIFA6%,16) + $1,000(PVIF6%,16) = $1,101.06The percentage change in price is calculated as:Percentage change in price = (New price – Original price) / Original price∆P Faulk% = ($696.82 – 783.24) / $783.24 = –0.1103 or –11.03%∆P Gonas% = ($1,101.06 – 1,216.76) / $1,216.76 = –0.0951 or –9.51% If the YTM declines from 10 percent to 8 percent:P Faulk= $30(PVIFA4%,16) + $1,000(PVIF4%,16) = $883.48P Gonas= $70(PVIFA4%,16) + $1,000(PVIF4%,16) = $1,349.57∆P Faulk% = ($883.48 – 783.24) / $783.24 = +0.1280 or 12.80%∆P Gonas% = ($1,349.57 – 1,216.76) / $1,216.76 = +0.1092 or 10.92% All else the same, the lower the coupon rate on a bond, the greater is its price sensitivity to changes in interest rates.16.The bond price equation for this bond is:P0 = $960 = $37(PVIFA R%,18) + $1,000(PVIF R%,18)Using a spreadsheet, financial calculator, or trial and error we find:R = 4.016%This is the semiannual interest rate, so the YTM is:YTM = 2 ⨯ 4.016% = 8.03%The current yield is:Current yield = Annual coupon payment / Price = $74 / $960 = .0771 or 7.71%The effective annual yield is the same as the EAR, so using the EAR equation from the previous chapter:Effective annual yield = (1 + 0.04016)2– 1 = .0819 or 8.19%17.The company should set the coupon rate on its new bonds equal to the required return.The required return can be observed in the market by finding the YTM on outstanding bonds of the company. So, the YTM on the bonds currently sold in the market is:P = $1,063 = $50(PVIFA R%,40) + $1,000(PVIF R%,40)Using a spreadsheet, financial calculator, or trial and error we find:R = 4.650%This is the semiannual interest rate, so the YTM is:YTM = 2 ⨯ 4.650% = 9.30%18. Accrued interest is the coupon payment for the period times the fraction of the periodthat has passed since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six months is one-half of the annual coupon payment. There are two months until the next coupon payment, so four months have passed since the last coupon payment. The accrued interest for the bond is:Accrued interest = $84/2 × 4/6 = $28And we calculate the clean price as:Clean price = Dirty price – Accrued interest = $1,090 – 28 = $1,06219. Accrued interest is the coupon payment for the period times the fraction of the periodthat has passed since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six months is one-half of the annual coupon payment. There are four months until the next coupon payment, so two months have passed since the last coupon payment. The accrued interest for the bond is:Accrued interest = $72/2 × 2/6 = $12.00And we calculate the dirty price as:Dirty price = Clean price + Accrued interest = $904 + 12 = $916.0020.To find the number of years to maturity for the bond, we need to find the price of thebond. Since we already have the coupon rate, we can use the bond price equation, and solve for the number of years to maturity. We are given the current yield of the bond, so we can calculate the price as:Current yield = .0842 = $90/P0P0 = $90/.0842 = $1,068.88Now that we have the price of the bond, the bond price equation is:P = $1,068.88 = $90{[(1 – (1/1.0781)t ] / .0781} + $1,000/1.0781tWe can solve this equation for t as follows:$1,068.88 (1.0781)t = $1,152.37 (1.0781)t– 1,152.37 + 1,000152.37 = 83.49(1.0781)t1.8251 = 1.0781tt = log 1.8251 / log 1.0781 = 8.0004 ≈ 8 yearsThe bond has 8 years to maturity.21.The bond has 10 years to maturity, so the bond price equation is:P = $871.55 = $41.25(PVIFA R%,20) + $1,000(PVIF R%,20)Using a spreadsheet, financial calculator, or trial and error we find:R = 5.171%This is the semiannual interest rate, so the YTM is:YTM = 2 5.171% = 10.34%The current yield is the annual coupon payment divided by the bond price, so:Current yield = $82.50 / $871.55 = .0947 or 9.47%22.We found the maturity of a bond in Problem 20. However, in this case, the maturity isindeterminate. A bond selling at par can have any length of maturity. In other words, when we solve the bond pricing equation as we did in Problem 20, the number of periods can be any positive number.Challenge23.To find the capital gains yield and the current yield, we need to find the price of the bond.The current price of Bond P and the price of Bond P in one year is:P: P0 = $90(PVIFA7%,5) + $1,000(PVIF7%,5) = $1,082.00P1 = $90(PVIFA7%,4) + $1,000(PVIF7%,4) = $1,067.74Current yield = $90 / $1,082.00 = .0832 or 8.32%The capital gains yield is:Capital gains yield = (New price – Original price) / Original priceCapital gains yield = ($1,067.74 – 1,082.00) / $1,082.00 = –0.0132 or –1.32% The current price of Bond D and the price of Bond D in one year is:D: P0 = $50(PVIFA7%,5) + $1,000(PVIF7%,5) = $918.00P1 = $50(PVIFA7%,4) + $1,000(PVIF7%,4) = $932.26Current yield = $50 / $918.00 = 0.0545 or 5.45%Capital gains yield = ($932.26 – 918.00) / $918.00 = 0.0155 or 1.55% All else held constant, premium bonds pay a high current income while having price depreciation as maturity nears; discount bonds pay a lower current income but have price appreciation as maturity nears. For either bond, the total return is still 7%, but this return is distributed differently between current income and capital gains.24.a. The rate of return you expect to earn if you purchase a bond and hold it until maturity is the YTM. The bond price equation for this bond is:P0 = $1,140 = $90(PVIFA R%,10) + $1,000(PVIF R%,10)Using a spreadsheet, financial calculator, or trial and error we find:R = YTM = 7.01%b. To find our HPY, we need to find the price of the bond in two years. The price ofthe bond in two years, at the new interest rate, will be:P2 = $90(PVIFA6.01%,8) + $1,000(PVIF6.01%,8) = $1,185.87To calculate the HPY, we need to find the interest rate that equates the price wepaid for the bond with the cash flows we received. The cash flows we receivedwere $90 each year for two years, and the price of the bond when we sold it. Theequation to find our HPY is:P0 = $1,140 = $90(PVIFA R%,2) + $1,185.87(PVIF R%,2)Solving for R, we get:R = HPY = 9.81%The realized HPY is greater than the expected YTM when the bond was boughtbecause interest rates dropped by 1 percent; bond prices rise when yields fall.25.The price of any bond (or financial instrument) is the PV of the future cash flows. Eventhough Bond M makes different coupons payments, to find the price of the bond, we just find the PV of the cash flows. The PV of the cash flows for Bond M is:P M = $800(PVIFA4%,16)(PVIF4%,12) + $1,000(PVIFA4%,12)(PVIF4%,28) +$20,000(PVIF4%,40)P M = $13,117.88Notice that for the coupon payments of $800, we found the PVA for the coupon payments, and then discounted the lump sum back to today.Bond N is a zero coupon bond with a $20,000 par value; therefore, the price of the bond is the PV of the par, or:P N = $20,000(PVIF4%,40) = $4,165.7826.To find the present value, we need to find the real weekly interest rate. To find the realreturn, we need to use the effective annual rates in the Fisher equation. So, we find the real EAR is:(1 + R) = (1 + r)(1 + h)1 + .107 = (1 + r)(1 + .035)r = .0696 or 6.96%Now, to find the weekly interest rate, we need to find the APR. Using the equation for discrete compounding:EAR = [1 + (APR / m)]m– 1We can solve for the APR. Doing so, we get:APR = m[(1 + EAR)1/m– 1]APR = 52[(1 + .0696)1/52– 1]APR = .0673 or 6.73%So, the weekly interest rate is:Weekly rate = APR / 52Weekly rate = .0673 / 52Weekly rate = .0013 or 0.13%Now we can find the present value of the cost of the roses. The real cash flows are an ordinary annuity, discounted at the real interest rate. So, the present value of the cost of the roses is:PVA = C({1 – [1/(1 + r)]t } / r)PVA = $8({1 – [1/(1 + .0013)]30(52)} / .0013)PVA = $5,359.6427.To answer this question, we need to find the monthly interest rate, which is the APRdivided by 12. We also must be careful to use the real interest rate. The Fisher equation uses the effective annual rate, so, the real effective annual interest rates, and the monthly interest rates for each account are:Stock account:(1 + R) = (1 + r)(1 + h)1 + .12 = (1 + r)(1 + .04)r = .0769 or 7.69%APR = m[(1 + EAR)1/m– 1]APR = 12[(1 + .0769)1/12– 1]APR = .0743 or 7.43%Monthly rate = APR / 12Monthly rate = .0743 / 12Monthly rate = .0062 or 0.62%Bond account:(1 + R) = (1 + r)(1 + h)1 + .07 = (1 + r)(1 + .04)r = .0288 or 2.88%APR = m[(1 + EAR)1/m– 1]APR = 12[(1 + .0288)1/12– 1]APR = .0285 or 2.85%Monthly rate = APR / 12Monthly rate = .0285 / 12Monthly rate = .0024 or 0.24%Now we can find the future value of the retirement account in real terms. The future value of each account will be:Stock account:FVA = C {(1 + r )t– 1] / r}FVA = $800{[(1 + .0062)360 – 1] / .0062]}FVA = $1,063,761.75Bond account:FVA = C {(1 + r )t– 1] / r}FVA = $400{[(1 + .0024)360 – 1] / .0024]}FVA = $227,089.04The total future value of the retirement account will be the sum of the two accounts, or: Account value = $1,063,761.75 + 227,089.04Account value = $1,290,850.79Now we need to find the monthly interest rate in retirement. We can use the same procedure that we used to find the monthly interest rates for the stock and bond accounts, so:(1 + R) = (1 + r)(1 + h)1 + .08 = (1 + r)(1 + .04)r = .0385 or 3.85%APR = m[(1 + EAR)1/m– 1]APR = 12[(1 + .0385)1/12– 1]APR = .0378 or 3.78%Monthly rate = APR / 12Monthly rate = .0378 / 12Monthly rate = .0031 or 0.31%Now we can find the real monthly withdrawal in retirement. Using the present value of an annuity equation and solving for the payment, we find:PVA = C({1 – [1/(1 + r)]t } / r )$1,290,850.79 = C({1 – [1/(1 + .0031)]300 } / .0031)C = $6,657.74This is the real dollar amount of the monthly withdrawals. The nominal monthly withdrawals will increase by the inflation rate each month. To find the nominal dollar amount of the last withdrawal, we can increase the real dollar withdrawal by the inflation rate. We can increase the real withdrawal by the effective annual inflation rate since we are only interested in the nominal amount of the last withdrawal. So, the last withdrawal in nominal terms will be: FV = PV(1 + r)tFV = $6,657.74(1 + .04)(30 + 25)FV = $57,565.3028.In this problem, we need to calculate the future value of the annual savings after the fiveyears of operations. The savings are the revenues minus the costs, or:Savings = Revenue – CostsSince the annual fee and the number of members are increasing, we need to calculate the effective growth rate for revenues, which is:Effective growth rate = (1 + .06)(1 + .03) – 1Effective growth rate = .0918 or 9.18%The revenue for the current year is the number of members times the annual fee, or:Current revenue = 500($500)Current revenue = $250,000The revenue will grow at 9.18 percent, and the costs will grow at 2 percent, so the savings each year for the next five years will be:Year Revenue Costs Savings1 $ 272,950.00 $ 76,500.00 $ 196,450.002 298,006.81 78,030.00 219,976.813 325,363.84 79,590.60 245,773.244 355,232.24 81,182.41 274,049.825 387,842.55 82,806.06 305,036.49Now we can find the value of each year’s savi ngs using the future value of a lump sum equation, so:FV = PV(1 + r)tYear Future Value1 $196,450.00(1 + .09)4 = $277,305.212 $219,976.81(1 + .09)3 = 284,876.353 $245,773.24(1 + .09)2 = 292,003.184 $274,049.82(1 + .09)1 = 298,714.315 305,036.49Total future value of savings = $1,457,935.54He will spend $500,000 on a luxury boat, so the value of his account will be:Value of account = $1,457,935.54 – 500,000Value of account = $957,935.54Now we can use the present value of an annuity equation to find the payment. Doing so, we find:PVA = C({1 – [1/(1 + r)]t } / r )$957,935.54 = C({1 – [1/(1 + .09)]25 } / .09)C = $97,523.83CHAPTER 91.We need to find the required return of the stock. Using the constant growth model, wecan solve the equation for R. Doing so, we find:R = (D1 / P0) + g = ($2.85 / $58) + .06 = .1091 or 10.91%2.The dividend yield is the dividend next year divided by the current price, so the dividendyield is:Dividend yield = D1 / P0 = $2.85 / $58 = .0491 or 4.91%The capital gains yield, or percentage increase in the stock price, is the same as the dividend growth rate, so:Capital gains yield = 6%3.We know the stock has a required return of 13 percent, and the dividend and capitalgains yield are equal, so:Dividend yield = 1/2(.13) = .065 = Capital gains yieldNow we know both the dividend yield and capital gains yield. The dividend is simply the stock price times the dividend yield, so:D1 = .065($64) = $4.16This is the dividend next year. The question asks for the dividend this year. Using the relationship between the dividend this year and the dividend next year:D1 = D0(1 + g)We can solve for the dividend that was just paid:$4.16 = D0 (1 + .065)D0 = $4.16 / 1.065 = $3.914.The price of a share of preferred stock is the dividend divided by the required return.This is the same equation as the constant growth model, with a dividend growth rate of zero percent. Remember that most preferred stock pays a fixed dividend, so the growth rate is zero. Using this equation, we find the price per share of the preferred stock is:R = D/P0 = $6.40/$103 = .0621 or 6.21%5.This stock has a constant growth rate of dividends, but the required return changes twice.To find the value of the stock today, we will begin by finding the price of the stock at Year 6, when both the dividend growth rate and the required return are stable forever.The price of the stock in Year 6 will be the dividend in Year 7, divided by the required return minus the growth rate in dividends. So:P6 = D6(1 + g) / (R–g) = D0(1 + g)7/ (R–g) = $2.75(1.06)7/ (.11 – .06) = $82.70Now we can find the price of the stock in Year 3. We need to find the price here since the required return changes at that time. The price of the stock in Year 3 is the PV of the dividends in Years 4, 5, and 6, plus the PV of the stock price in Year 6. The price of the stock in Year 3 is:P3= $2.75(1.06)4/ 1.14 + $2.75(1.06)5/ 1.142 + $2.75(1.06)6/ 1.143 + $82.70 / 1.143 P3= $64.33Finally, we can find the price of the stock today. The price today will be the PV of the dividends in Years 1, 2, and 3, plus the PV of the stock in Year 3. The price of the stock today is:P0= $2.75(1.06) / 1.16 + $2.75(1.06)2/ (1.16)2+ $2.75(1.06)3/ (1.16)3+ $64.33 /(1.16)3P0= $48.126.The price of a stock is the PV of the future dividends. This stock is paying five dividends,so the price of the stock is the PV of these dividends using the required return. The price of the stock is:P0 = $13 / 1.11 + $16 / 1.112 + $19 / 1.113 + $22 / 1.114 + $25 / 1.115 = $67.927.Here we need to find the dividend next year for a stock experiencing differential growth.We know the stock price, the dividend growth rates, and the required return, but not the dividend. First, we need to realize that the dividend in Year 3 is the current dividend times the FVIF. The dividend in Year 3 will be:D3 = D0(1.30)3And the dividend in Year 4 will be the dividend in Year 3 times one plus the growth rate, or:D4 = D0(1.30)3(1.18)The stock begins constant growth after the 4th dividend is paid, so we can find the price of the stock in Year 4 as the dividend in Year 5, divided by the required return minus the growth rate. The equation for the price of the stock in Year 4 is:P4= D4(1 + g) / (R– g)Now we can substitute the previous dividend in Year 4 into this equation as follows:P4 = D0(1 + g1)3(1 + g2) (1 + g3) / (R–g3)P4= D0(1.30)3(1.18) (1.08) / (.13 – .08) = 56.00D0When we solve this equation, we find that the stock price in Year 4 is 56.00 times as large as the dividend today. Now we need to find the equation for the stock price today.The stock price today is the PV of the dividends in Years 1, 2, 3, and 4, plus the PV of the Year 4 price. So:P0= D0(1.30)/1.13 + D0(1.30)2/1.132+ D0(1.30)3/1.133+ D0(1.30)3(1.18)/1.134+56.00D0/1.134We can factor out D0 in the equation, and combine the last two terms. Doing so, we get: P0 = $65.00 = D0{1.30/1.13 + 1.302/1.132 + 1.303/1.133 + [(1.30)3(1.18) + 56.00] / 1.134} Reducing the equation even further by solving all of the terms in the braces, we get:$65 = $39.86D0D0 = $65.00 / $39.86 = $1.63This is the dividend today, so the projected dividend for the next year will be:D1 = $1.63(1.30) = $2.128.The price of a share of preferred stock is the dividend payment divided by the requiredreturn. We know the dividend payment in Year 5, so we can find the price of the stock in Year 4, one year before the first dividend payment. Doing so, we get:P4 = $7.00 / .06 = $116.67The price of the stock today is the PV of the stock price in the future, so the price today will be:P0 = $116.67 / (1.06)4 = $92.419.To find the number of shares owned, we can divide the amount invested by the stockprice. The share price of any financial asset is the present value of the cash flows, so, tofind the price of the stock we need to find the cash flows. The cash flows are the two dividend payments plus the sale price. We also need to find the aftertax dividends since the assumption is all dividends are taxed at the same rate for all investors. The aftertax dividends are the dividends times one minus the tax rate, so:Year 1 aftertax dividend = $1.50(1 – .28)Year 1 aftertax dividend = $1.08Year 2 aftertax dividend = $2.25(1 – .28)Year 2 aftertax dividend = $1.62We can now discount all cash flows from the stock at the required return. Doing so, we find the price of the stock is:P = $1.08/1.15 + $1.62/(1.15)2 + $60/(1+.15)3P = $41.62The number of shares owned is the total investment divided by the stock price, which is: Shares owned = $100,000 / $41.62Shares owned = 2,402.9810.The required return of a stock consists of two components, the capital gains yield and thedividend yield. In the constant dividend growth model (growing perpetuity equation), the capital gains yield is the same as the dividend growth rate, or algebraically:R = D1/P0 + gWe can find the dividend growth rate by the growth rate equation, or:g = ROE ×bg = .16 × .80g = .1280 or 12.80%This is also the growth rate in dividends. To find the current dividend, we can use the information provided about the net income, shares outstanding, and payout ratio. The total dividends paid is the net income times the payout ratio. To find the dividend per share, we can divide the total dividends paid by the number of shares outstanding. So: Dividend per share = (Net income × Payout ratio) / Shares outstandingDividend per share = ($10,000,000 × .20) / 2,000,000Dividend per share = $1.00Now we can use the initial equation for the required return. We must remember that the equation uses the dividend in one year, so:R = D1/P0 + gR = $1(1 + .1280)/$85 + .1280R = .1413 or 14.13%11. a.If the company does not make any new investments, the stock price will be thepresent value of the constant perpetual dividends. In this case, all earnings are paiddividends, so, applying the perpetuity equation, we get:P = Dividend / RP = $8.25 / .12P = $68.75。
公司理财中文版第九版第三章答案
公司理财中文版第九版第三章答案第三章财务报表分析与长期财务规划1.由于不同行业的公司在财务比率上存在很大的差异，财务比率本身提供的信息有限，分析公司的财务比率有两种基本方法：时间趋势分析法和同类公司分析。
为什么这些方法会有用呢？每种方法能够告诉你哪些关于公司财务健康状况的信息？答：时间趋势分析描绘了公司财务状况随时间而变化的图景。
通过公司本身的跨期比较分析，财务经理可以评价公司的经营、融资或投资活动的某些方面是否已经发生了变化。
同类公司比较分析涉及到将某一特定公司的财务比率和经营绩效与同一行业或业务相同的其他公司进行比较。
通过同类公司比较分析，财务经理可以评价公司的经营、融资或投资活动的某些方面是否越出常规，从而为调整这些比率采取恰当的行动提供指南，如果这种调整合适的话。
两种方法都从财务的视角研究一个公司究竟有什么不同，但两种方法都不能告诉我们这种不同是积极正面的还是消极负面的。
例如，假设一个公司的流动比率是随时间而提高，这可能意味着该公司在过去一直面临流动性问题，一直在纠正这些问题；或者它也可能意味着该公司已经在管理流动资产和负债方面变得更低效。
同类公司比较分析中也存在类似的争论。
一个公司的流动比率低于同类公司，也许是它在管理流动资产和负债方面更具效率，也可能是它面临流动性问题。
没有哪种方法告诉我们某个比率是好还是坏。
两种方法只是表明有些东西存在差异，并告诉我们去哪里寻找。
2.所谓的“同店销售”是像沃尔玛和希尔斯之类的十分分散的公司的一项重要指标，顾名思义，分析同店销售就是比较同样的店铺或餐馆在两个不同的时间点上的销售额。
为什么公司总是关注同店销售而不是总销售？答：如果一家公司通过开设新店来不断增长，那么可以推测其总收入将上升。
比较两个不同时点的总销售额可能会误导。
同店销售方法只看在特定时期开设的店铺的销售额控制了这一误差。
3.为什么多数长期财务计划都从销售预测开始？或者说，为什么未来销售额是关键？答：理由是，最终，消费是商业背后的驱动力。
罗斯《公司理财》(第9版)课后习题(第1~3章)【圣才出品】
罗斯《公司理财》（第9版）课后习题第1章公司理财导论一、概念题1．资本预算（capital budgeting）答：资本预算是指综合反映投资资金来源与运用的预算，是为了获得未来产生现金流量的长期资产而现在投资支出的预算。
资本预算决策也称为长期投资决策，它是公司创造价值的主要方法。
资本预算决策一般指固定资产投资决策，耗资大，周期长，长期影响公司的产销能力和财务状况，决策正确与否影响公司的生存与发展。
完整的资本预算过程包括：寻找增长机会，制定长期投资战略，预测投资项目的现金流，分析评估投资项目，控制投资项目的执行情况。
资本预算可通过不同的资本预算方法来解决，如回收期法、净现值法和内部收益率法等。
2．货币市场（money markets）答：货币市场指期限不超过一年的资金借贷和短期有价证券交易的金融市场，亦称“短期金融市场”或“短期资金市场”，包括同业拆借市场、银行短期存贷市场、票据市场、短期证券市场、大额可转让存单市场、回购协议市场等。
其参加者为各种政府机构、各种银行和非银行金融机构及公司等。
货币市场具有四个基本特征：①融资期限短，一般在一年以内，最短的只有半天，主要用于满足短期资金周转的需要；②流动性强，金融工具可以在市场上随时兑现，交易对象主要是期限短、流动性强、风险小的信用工具，如票据、存单等，这些工具变现能力强，近似于货币，可称为“准货币”，故称货币市场；③安全性高，由于货币市场上的交易大多采用即期交易，即成交后马上结清，通常不存在因成交与结算日之间时间相对过长而引起价格巨大波动的现象，对投资者来说，收益具有较大保障；④政策性明显，货币市场由货币当局直接参加，是中央银行同商业银行及其他金融机构的资金连接的主渠道，是国家利用货币政策工具调节全国金融活动的杠杆支点。
货币市场的交易主体是短期资金的供需者。
需求者是为了获得现实的支付手段，调节资金的流动性并保持必要的支付能力，供应者提供的资金也大多是短期临时闲置性的资金。
公司理财第九版 中文答案 这个是word可以自己修改
公司理财第九版中文答案这个是word可以自己修改第1章企业融资简介概念问题的答案1。
在企业的所有制形式，股东是公司的所有者。
的股东选出该法团的董事，谁又将委任公司的管理层。
是什么原因造成这种分离，所有权控制在企业的组织形式存在的代理问题。
管理可能在其自己或别人的最佳利益行事，而不是股东。
如果这样的事件发生时，他们可能会最大化的股权，该公司的股价目标相矛盾。
2。
这些组织经常追求社会或政治任务，所以许多不同的目标，是可以想象的。
一个经常被援引的目标是收入最小化，也就是说，提供力所能及的提供商品和服务，在尽可能低的社会成本。
一个更好的办法可能是观察，即使是一个不以营利为目的的企业拥有股权。
因此，一个答案是，在适当的目标是价值最大化的股权。
3。
据推测，目前的股票价值反映风险，所有未来现金流量的时间和幅度，短期和长期的。
如果这是正确的，那么陈述是错误的。
4。
参数可以两种方式。
在一个极端，我们可以认为，在市场经济中，所有这些东西的价格。
因此，有一个最佳的水平，例如，道德和/或非法行为，以及股票估值的框架明确包括这些。
在另一个极端，我们可以说，这些都是非经济现象，并通过政治进程是最好的处理。
一个经典的（高度相关）的思想说明了这一点辩论的问题是这样的：“估计，一个企业之一，其产品的成本，提高了安全额为3,000万元。
不过，该公司认为，提高了产品的安全性，不仅节省了2000万美元的产品责任索赔。
企业应该做些什么？“5。
我们的目标将是相同的，但最好的行动实现这一目标的过程中可能会有所不同，因为不同的社会，政治和经济机构。
6。
管理的目标应该是最大限度地提高现有股东的股价。
如果管理层认为它可以提高公司的盈利能力，从而使股票价格将超过$ 35，那么他们应该争取从外面公司的报价。
如果管理层认为该投标人或其他身份不明的投标人将实际支付超过每股35美元收购该公司，那么他们还是应该打的报价。
但是，如果目前的管理不能增加公司价值超越的买入价，并没有其他出价更高的，当时的管理层不采取行动，战斗要约股东的利益。
中央财经大学公司理财中文版第九版第二章答案
第2 章会计报表与现金流1. 是非题：所有的资产在付出某种代价的情况下都具有流动性。
请解释。
答：正确。
所有资产都可以以某种价格转换为现金。
然而，当我们提到流动性资产的时候，一个附加的假设变得很重要，这个假设是资产可以以市场价值或接近市场价值的价格转换成现金。
2. 为什么标准的利润表上列示的收入和成本不代表当期实际的现金流入和现金流出？答：财务会计里面的确认原则和匹配原则要求将收入和产生收入相关的费用在收入过程实际完成的时候就记录下来，而不必等到收到现金或支付账单的时候。
我们要注意到这种方式并不必然是正确的，主要取决于会计师的选择。
3. 在会计现金流量表上，最后一栏表示什么？这个数字对于分析一家公司有何用处？答：最后一栏的数据显示了资产负债表上现金余额的变化。
既然如此，它对于分析一个公司来说并不是一个有用的数据。
4. 财务现金流量表与会计现金流量表有何不同？哪个对于公司分析者更有用？答：主要的不同在于对利息费用的处理，会计现金流量表将利息视为经营现金流，而财务现金流量将其视为融资现金流。
会计现金流量的逻辑是，既然利息列支在利润表上，而利润表显示的是一定时期内企业的经营状况，因此利息也就是经营性的现金流。
而事实上，利息是一种融资费用，是公司选择债务融资还是股权融资的结果，我们将在以后的章节中有更为详细的讲解。
相比两种现金流量表，财务现金流量表以其正确对待利息费用而成为分析公司业绩的更恰当的方法。
5. 按照会计规定，一家公司的负债有可能超过资产，所有者权益为负，这种情况在市场价值上有没有可能发生？为什么？答：市场的价值不可能为负。
假设有一股股票，价格为负的20 美元，这意味着如果你买入100股，你不仅得到了股票，而且还得到了一张1200美元的现金支票，那么你会想买入多少股呢？更一般的情形是，根据公司破产和个人破产法，个人或公司的净资产不能为负，这意味着从市场价值的角度看，负债不可能超过资产。
6. 为什么说在一个特定的期间内资产的现金流量为负不一定不好？答：例如，对于一个正在迅速扩张的成功企业来说，它的资本性支出将会很大，这可能会导致出现负的现金流。
第九版-公司理财-罗斯-中文答案-第五章汇编
1、如果项目带来的是常规的现金流，而且其回收期短于该项目的生命周期，还不能准备判断其净现值的正负。
仍需要其采用的折现率和其内部收益率IRR 做对比。
当折现率小于IRRA时，净现值为正值，当折现率大于IRRA时，净现值为负值，两者相等时，净现值为零。
如果一个项目的折现回收期短于该项目的生命周期，则净现值一定为正值。
2、项目有常规的现金流，且NPV为正值，则各期流入的现金流折现总和一定大于期初项目资金流出。
而各期流入的现金流总和肯定大于折现总和，所以该项目的回收期一定短于其生命周期。
同时折现回收期是用和净现值同样的NPV计算出来的，所以折现回收期也一定短于其生命周期。
同样净现值为正值，说明初始投资所带来的后续现金流的现值大于初始投资，所以盈利指数PI一定大于1。
如果使用内部收益率折现各期现金流量时，净现值为零。
而以折现率折现各期现金流量时，净现值为正，说明折现率小于内部收益率。
3、a 回收期是指投资引起的现金流入累计到与投资相等所需要的时间。
它代表收回投资所需要的年限。
回收年限越短，方案越有利。
其缺陷就是忽略了回收期内现金流量的时间序列，也忽略了回收期以后的现金支付，同时对于回收期的选择也存在主观臆断。
选择一个具体的回收期决策标准，当项目的回收期小于标准的就可行，大于标准的则拒绝。
b 平均会计收益率是指为扣除所得税和折旧之后的项目平均收益除以整个项目期限内的平均账面投资额。
其缺陷是使用账面收益而非现金流量，忽略了折旧对现金流量的影响，忽视了净收益的时间分布对项目经济价值的影响。
当项目的平均会计收益率小于目标平均会计收益率时，则拒绝项目，反之接受。
c 内部收益率就是令项目净现值为0的折现率。
其缺点是对于特殊项目无法用一般原则进行判断，并且有些项目可能会出现多个收益率的现象。
同时对于互斥项目容易忽视其规模问题和时间序列问题。
一般原则是当折现率小于IRR时，接受该项目，反之则拒绝。
d 盈利指数是初始投资所带来的后续现金流的现值和初始投资的比值。
公司理财Corporate_Finance_第九版_CASE答案(完整资料).doc
【最新整理，下载后即可编辑】Case SolutionsFundamentals of Corporate FinanceRoss, Westerfield, and Jordan9th editionCHAPTER 1THE McGEE CAKE COMPANY1.The advantages to a LLC are: 1) Reduction of personal liability. A soleproprietor has unlimited liability, which can include the potential loss of all personal assets. 2) Taxes. Forming an LLC may mean that more expenses can be considered business expenses and be deducted from the company’s income. 3) Improved credibility. The business may have increased credibility in the business world compared to a sole proprietorship. 4) Ability to attract investment. Corporations, even LLCs, can raise capital through the sale of equity. 5) Continuous life. Sole proprietorships have a limited life, while corporations have a potentially perpetual life. 6) Transfer of ownership. It is easier to transfer ownership in a corporation through the sale of stock.The biggest disadvantage is the potential cost, although the cost of forminga LLC can be relatively small. There are also other potential costs, includingmore expansive record-keeping.2.Forming a corporation has the same advantages as forming a LLC, but thecosts are likely to be higher.3.As a small company, changing to a LLC is probably the most advantageousdecision at the current time. If the company grows, and Doc and Lyn are willing to sell more equity ownership, the company can reorganize as a corporation at a later date. Additionally, forming a LLC is likely to be less expensive than forming a corporation.CHAPTER 2CASH FLOWS AND FINANCIAL STATEMENTS AT SUNSET BOARDS Below are the financial statements that you are asked to prepare.1.The income statement for each year will look like this:Income statement2008 2009Sales $247,259 $301,392Cost of goods sold 126,038 159,143Selling & administrative 24,787 32,352Depreciation 35,581 40,217EBIT $60,853 $69,680Interest 7,735 8,866EBT $53,118 $60,814Taxes 10,624 12,163Net income $42,494 $48,651Dividends $21,247 $24,326Addition to retainedearnings 21,247 24,3262.The balance sheet for each year will be:Balance sheet as of Dec. 31, 2008C-26 CASE SOLUTIONSCash $18,187 Accounts payable $32,143 Accountsreceivable 12,887 Notes payable 14,651 Inventory 27,119 Current liabilities $46,794 Current assets $58,193Long-term debt $79,235 Net fixed assets $156,975 Owners' equity 89,139Total assets $215,168 Total liab. &equity $215,168In the first year, equity is not given. Therefore, we must calculate equity as a plug variable. Since total liabilities & equity is equal to total assets, equity can be calculated as:Equity = $215,168 – 46,794 – 79,235Equity = $89,139CHAPTER 2 C-5Balance sheet as of Dec. 31, 2009Cash $27,478 Accounts payable $36,404 Accountsreceivable 16,717 Notes payable 15,997 Inventory 37,216 Current liabilities $52,401 Current assets $81,411Long-term debt $91,195 Net fixed assets $191,250 Owners' equity 129,065Total assets $272,661 Total liab. &equity $272,661The owner’s equity for 2009 is the beginning of year owner’s equity, plus the addition to retained earnings, plus the new equity, so:Equity = $89,139 + 24,326 + 15,600Equity = $129,065ing the OCF equation:OCF = EBIT + Depreciation – TaxesThe OCF for each year is:OCF2008 = $60,853 + 35,581 – 10,624OCF2008 = $85,180OCF2009 = $69,680 + 40,217 – 12,163OCF2009 = $97,734C-26 CASE SOLUTIONS4.To calculate the cash flow from assets, we need to find the capital spendingand change in net working capital. The capital spending for the year was: Capital spendingEnding net fixed assets $191,250– Beginning net fixedassets 156,975+ Depreciation 40,217Net capital spending $74,492And the change in net working capital was:Change in net working capitalEnding NWC $29,010– Beginning NWC 11,399Change in NWC $17,611CHAPTER 2 C-5 So, the cash flow from assets was:Cash flow from assetsOperating cash flow $97,734– Net capital spending 74,492– Change in NWC 17,611Cash flow from assets $ 5,6315.The cash flow to creditors was:Cash flow to creditorsInterest paid $8,866– Net new borrowing 11,960Cash flow to creditors –$3,0946.The cash flow to stockholders was:Cash flow tostockholdersDividends paid $24,326– Net new equityraised 15,600Cash flow tostockholders $8,726Answers to questions1.The firm had positive earnings in an accounting sense (NI > 0) and hadpositive cash flow from operations. The firm invested $17,611 in new netC-26 CASE SOLUTIONSworking capital and $74,492 in new fixed assets. The firm gave $5,631 to its stakeholders. It raised $3,094 from bondholders, and paid $8,726 to stockholders.2.The expansion plans may be a little risky. The company does have a positivecash flow, but a large portion of the operating cash flow is already going to capital spending. The company has had to raise capital from creditors and stockholders for its current operations. So, the expansion plans may be too aggressive at this time. On the other hand, companies do need capital to grow. Before investing or loaning the company money, you would want to know where the current capital spending is going, and why the company is spending so much in this area already.CHAPTER 3RATIOS ANALYSIS AT S&S AIR1.The calculations for the ratios listed are:Current ratio = $2,186,520 / $2,919,000Current ratio = 0.75 timesQuick ratio = ($2,186,250 – 1,037,120) / $2,919,000Quick ratio = 0.39 timesCash ratio = $441,000 / $2,919,000Cash ratio = 0.15 timesTotal asset turnover = $30,499,420 / $18,308,920Total asset turnover = 1.67 timesInventory turnover = $22,224,580 / $1,037,120Inventory turnover = 21.43 timesReceivables turnover = $30,499,420 / $708,400Receivables turnover = 43.05 timesTotal debt ratio = ($18,308,920 – 10,069,920) / $18,308,920 Total debt ratio = 0.45 timesDebt-equity ratio = ($2,919,000 + 5,320,000) / $10,069,920C-26 CASE SOLUTIONSDebt-equity ratio = 0.82 timesEquity multiplier = $18,308,920 / $10,069,920Equity multiplier = 1.82 timesTimes interest earned = $3,040,660 / $478,240Times interest earned = 6.36 timesCash coverage = ($3,040,660 + 1,366,680) / $478,420 Cash coverage = 9.22 timesProfit margin = $1,537,452 / $30,499,420Profit margin = 5.04%Return on assets = $1,537,452 / $18,308,920Return on assets = 8.40%Return on equity = $1,537,452 / $10,069,920Return on equity = 15.27%CHAPTER 3 C-11 2. Boeing is probably not a good aspirant company. Even though bothcompanies manufacture airplanes, S&S Air manufactures small airplanes, while Boeing manufactures large, commercial aircraft. These are two different markets. Additionally, Boeing is heavily involved in the defense industry, as well as Boeing Capital, which finances airplanes.Bombardier is a Canadian company that builds business jets, short-range airliners and fire-fighting amphibious aircraft and also provides defense-related services. It is the third largest commercial aircraft manufacturer in the world. Embraer is a Brazilian manufacturer than manufactures commercial, military, and corporate airplanes. Additionally, the Brazilian government is a part owner of the company. Bombardier and Embraer are probably not good aspirant companies because of the diverse range of products and manufacture of larger aircraft.Cirrus is the world's second largest manufacturer of single-engine, piston-powered aircraft. Its SR22 is the world's best selling plane in its class. The company is noted for its innovative small aircraft and is a good aspirant company.Cessna is a well known manufacturer of small airplanes. The company produces business jets, freight- and passenger-hauling utility Caravans, personal and small-business single engine pistons. It may be a good aspirant company, however, its products could be considered too broad and diversified since S&S Air produces only small personal airplanes.3. S&S is below the median industry ratios for the current and cash ratios.This implies the company has less liquidity than the industry in general.However, both ratios are above the lower quartile, so there are companiesC-26 CASE SOLUTIONSin the industry with lower liquidity ratios than S&S Air. The company may have more predictable cash flows, or more access to short-term borrowing.If you created an Inventory to Current liabilities ratio, S&S Air would havea ratio that is lower than the industry median. The current ratio is below theindustry median, while the quick ratio is above the industry median. This implies that S&S Air has less inventory to current liabilities than the industry median. S&S Air has less inventory than the industry median, but more accounts receivable than the industry since the cash ratio is lower than the industry median.The turnover ratios are all higher than the industry median; in fact, all three turnover ratios are above the upper quartile. This may mean that S&S Air is more efficient than the industry.The financial leverage ratios are all below the industry median, but above the lower quartile. S&S Air generally has less debt than comparable companies, but still within the normal range.The profit margin, ROA, and ROE are all slightly below the industry median, however, not dramatically lower. The company may want to examine its costs structure to determine if costs can be reduced, or price can be increased.Overall, S&S Air’s performance seems good, although the liquidity ratios indicate that a closer look may be needed in this area.CHAPTER 3 C-11 Below is a list of possible reasons it may be good or bad that each ratio is higher or lower than the industry. Note that the list is not exhaustive, but merely one possible explanation for each ratio.Ratio Good BadCurrent ratio Better at managingcurrent accounts. May be having liquidity problems.Quick ratio Better at managingcurrent accounts. May be having liquidity problems.Cash ratio Better at managingcurrent accounts. May be having liquidity problems.Total asset turnover Better at utilizing assets. Assets may be older anddepreciated, requiringextensive investmentsoon.Inventory turnover Better at inventorymanagement, possibly dueto better procedures.Could be experiencinginventory shortages.Receivables turnover Better at collectingreceivables.May have credit termsthat are too strict.Decreasing receivablesturnover may increasesales.Total debt ratio Less debt than industrymedian means thecompany is less likely toexperience creditproblems. Increasing the amount of debt can increase shareholder returns. Especially notice that it will increase ROE.Debt-equity Less debt than industry Increasing the amount ofC-26 CASE SOLUTIONSratio median means thecompany is less likely toexperience creditproblems. debt can increase shareholder returns. Especially notice that it will increase ROE.Equity multiplier Less debt than industrymedian means thecompany is less likely toexperience creditproblems.Increasing the amount ofdebt can increaseshareholder returns.Especially notice that itwill increase ROE.TIE Higher quality materialscould be increasing costs. The company may have more difficulty meeting interest payments in a downturn.Cash coverage Less debt than industrymedian means thecompany is less likely toexperience creditproblems. Increasing the amount of debt can increase shareholder returns. Especially notice that it will increase ROE.Profit margin The PM is slightly belowthe industry median. Itcould be a result of higherquality materials or bettermanufacturing. Company may be having trouble controlling costs.ROA Company may have newerassets than the industry. Company may have newer assets than the industry.ROE Lower profit margin maybe a result of higherquality. Profit margin and EM are lower than industry, which results in the lower ROE.CHAPTER 4PLANNING FOR GROWTH AT S&S AIR1.To calculate the internal growth rate, we first need to find the ROA and theretention ratio, so:ROA = NI / TAROA = $1,537,452 / $18,309,920ROA = .0840 or 8.40%b = Addition to RE / NIb = $977,452 / $1,537,452b = 0.64Now we can use the internal growth rate equation to get:Internal growth rate = (ROA × b) / [1 – (ROA × b)]Internal growth rate = [0.0840(.64)] / [1 – 0.0840(.64)]Internal growth rate = .0564 or 5.64%To find the sustainable growth rate, we need the ROE, which is:ROE = NI / TEROE = $1,537,452 / $10,069,920ROE = .1527 or 15.27%C-26 CASE SOLUTIONSUsing the retention ratio we previously calculated, the sustainable growth rate is:Sustainable growth rate = (ROE × b) / [1 – (ROE × b)]Sustainable growth rate = [0.1527(.64)] / [1 – 0.1527(.64)]Sustainable growth rate = .1075 or 10.75%The internal growth rate is the growth rate the company can achieve with no outside financing of any sort. The sustainable growth rate is the growth rate the company can achieve by raising outside debt based on its retained earnings and current capital structure.CHAPTER 4 C-21 2.Pro forma financial statements for next year at a 12 percent growth rate are:Income statementSales $ 34,159,35COGS 24,891,530 Other expenses 4,331,600 Depreciation 1,366,680EBIT $ 3,569,541Interest 478,240Taxable income $ 3,091,301Taxes (40%) 1,236,520Net income $ 1,854,78Dividends $ 675,583C-26 CASE SOLUTIONSAdd to RE 1,179,197Balance sheetAssets Liabilities & EquityCurrent Assets Current LiabilitiesCash $ 493,92AccountsPayable $ 995,680Accounts rec. 793,408 Notes Payable 2,030,000 Inventory 1,161,574 Total CL $ 3,025,680 Total CA $ 2,448,902Long-term debt $ 5,320,000ShareholderEquityCommon stock $ 350,000Fixed assets Retainedearnings 10,899,117Net PP&E $ 18,057,088 Total Equity $ 11,249,117Total Assets $ 20,505,990 Total L&E $ 19,594,787CHAPTER 4 C-21 So, the EFN is:EFN = Total assets – Total liabilities and equityEFN = $20,505,990 – 19,594,797EFN = $911,193The company can grow at this rate by changing the way it operates. For example, if profit margin increases, say by reducing costs, the ROE increases, it will increase the sustainable growth rate. In general, as long as the company increases the profit margin, total asset turnover, or equity multiplier, the higher growth rate is possible. Note however, that changing any one of these will have the effect of changing the pro forma financial statements.C-26 CASE SOLUTIONS3.Now we are assuming the company can only build in amounts of $5 million.We will assume that the company will go ahead with the fixed asset acquisition. To estimate the new depreciation charge, we will find the current depreciation as a percentage of fixed assets, then, apply this percentage to the new fixed assets. The depreciation as a percentage of assets this year was:Depreciation percentage = $1,366,680 / $16,122,400Depreciation percentage = .0848 or 8.48%The new level of fixed assets with the $5 million purchase will be:New fixed assets = $16,122,400 + 5,000,000 = $21,122,400So, the pro forma depreciation will be:Pro forma depreciation = .0848($21,122,400)Pro forma depreciation = $1,790,525We will use this amount in the pro forma income statement. So, the pro forma income statement will be:Income statementSales $ 34,159,35COGS 24,891,530 Other expensesCHAPTER 4 C-214,331,600Depreciation 1,790,525EBIT $ 3,145,696Interest 478,240Taxable income $ 2,667,456Taxes (40%) 1,066,982Net income $ 1,600,473Dividends $ 582,955Add to RE 1,017,519C-26 CASE SOLUTIONSThe pro forma balance sheet will remain the same except for the fixed asset and equity accounts. The fixed asset account will increase by $5 million, rather than the growth rate of sales.Balance sheetAssets Liabilities & EquityCurrent Assets Current LiabilitiesCash $ 493,92AccountsPayable $ 995,680Accounts rec. 793,408 Notes Payable 2,030,000 Inventory 1,161,574 Total CL $ 3,025,680 Total CA $ 2,448,902Long-term debt $ 5,320,000ShareholderEquityCommon stock $ 350,000Fixed assets Retainedearnings 10,737,439Net PP&E $ 21,122,400 Total Equity $ 11,087,439Total Assets $ 23,571,302 Total L&E $ 19,433,119CHAPTER 4 C-21 So, the EFN is:EFN = Total assets – Total liabilities and equityEFN = $23,581,302 – 19,433,119EFN = $4,138,184Since the fixed assets have increased at a faster percentage than sales, the capacity utilization for next year will decrease.CHAPTER 6THE MBA DECISION1. Age is obviously an important factor. The younger an individual is, the moretime there is for the (hopefully) increased salary to offset the cost of the decision to return to school for an MBA. The cost includes both the explicit costs such as tuition, as well as the opportunity cost of the lost salary.2. Perhaps the most important nonquantifiable factors would be whether ornot he is married and if he has any children. With a spouse and/or children, he may be less inclined to return for an MBA since his family may be less amenable to the time and money constraints imposed by classes. Other factors would include his willingness and desire to pursue an MBA, job satisfaction, and how important the prestige of a job is to him, regardless of the salary.3.He has three choices: remain at his current job, pursue a Wilton MBA, orpursue a Mt. Perry MBA. In this analysis, room and board costs are irrelevant since presumably they will be the same whether he attends college or keeps his current job. We need to find the aftertax value of each, so:Remain at current job:Aftertax salary = $55,000(1 – .26) = $40,700CHAPTER 6 C-27 His salary will grow at 3 percent per year, so the present value of his aftertax salary is:PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]PV = $40,700{[1 – [(1 +.065)/(1 + .03)]38} / (.065 – .03)PV = $836,227.34Wilton MBA:Costs:Total direct costs = $63,000 + 2,500 + 3,000 = $68,500PV of direct costs = $68,500 + 68,500 / (1.065) = $132,819.25PV of indirect costs (lost salary) = $40,700 / (1.065) + $40,700(1 + .03) / (1 + .065)2 = $75,176.00Salary:PV of aftertax bonus paid in 2 years = $15,000(1 –.31) / 1.0652= $9,125.17Aftertax salary = $98,000(1 – .31) = $67,620C-26 CASE SOLUTIONSHis salary will grow at 4 percent per year. We must also remember that he will now only work for 36 years, so the present value of his aftertax salary is: PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]PV = $67,620{[1 – [(1 +.065)/(1 + .04)]36} / (.065 – .04)PV = $1,554,663.22Since the first salary payment will be received three years from today, so we need to discount this for two years to find the value today, which will be: PV = $1,544,663.22 / 1.0652PV = $1,370,683.26So, the total value of a Wilton MBA is:Value = –$75,160 – 132,819.25 + 9,125.17 + 1,370,683.26 =$1,171,813.18Mount Perry MBA:Costs:Total direct costs = $78,000 + 3,500 + 3,000 = $86,500. Note, this is also the PV of the direct costs since they are all paid today.PV of indirect costs (lost salary) = $40,700 / (1.065) = $38,215.96Salary:CHAPTER 6 C-27 PV of aftertax bonus paid in 1 year = $10,000(1 – .29) / 1.065 = $6,666.67 Aftertax salary = $81,000(1 – .29) = $57,510His salary will grow at 3.5 percent per year. We must also remember that he will now only work for 37 years, so the present value of his aftertax salary is: PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]PV = $57,510{[1 – [(1 +.065)/(1 + .035)]37} / (.065 – .035)PV = $1,250,991.81Since the first salary payment will be received two years from today, so we need to discount this for one year to find the value today, which will be:PV = $1,250,991.81 / 1.065PV = $1,174,640.20So, the total value of a Mount Perry MBA is:Value = –$86,500 – 38,215.96 + 6,666.67 + 1,174,640.20 = $1,056,590.90C-26 CASE SOLUTIONS4.He is somewhat correct. Calculating the future value of each decision willresult in the option with the highest present value having the highest future value. Thus, a future value analysis will result in the same decision. However, his statement that a future value analysis is the correct method is wrong since a present value analysis will give the correct answer as well.5. To find the salary offer he would need to make the Wilton MBA asfinancially attractive as the as the current job, we need to take the PV of his current job, add the costs of attending Wilton, and the PV of the bonus on an aftertax basis. So, the necessary PV to make the Wilton MBA the same as his current job will be:PV = $836,227.34 + 132,819.25 + 75,176.00 – 9,125.17 = $1,035,097.42This PV will make his current job exactly equal to the Wilton MBA on a financial basis. Since his salary will still be a growing annuity, the aftertax salary needed is:PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]$1,035,097.42 = C {[1 – [(1 +.065)/(1 + .04)]36} / (.065 – .04)C = $45,021.51This is the aftertax salary. So, the pretax salary must be:Pretax salary = $45,021.51 / (1 – .31) = $65,248.576.The cost (interest rate) of the decision depends on the riskiness of the use offunds, not the source of the funds. Therefore, whether he can pay cash orCHAPTER 6 C-27 must borrow is irrelevant. This is an important concept which will be discussed further in capital budgeting and the cost of capital in later chapters.CHAPTER 7FINANCING S&S AIR’S EXPANSION PLANS WITH A BOND ISSUEA rule of thumb with bond provisions is to determine who benefits by theprovision. If the company benefits, the bond will have a higher coupon rate.If the bondholders benefit, the bond will have a lower coupon rate.1. A bond with collateral will have a lower coupon rate. Bondholders have theclaim on the collateral, even in bankruptcy. Collateral provides an asset that bondholders can claim, which lowers their risk in default. The downside of collateral is that the company generally cannot sell the asset used as collateral, and they will generally have to keep the asset in good working order.2.The more senior the bond is, the lower the coupon rate. Senior bonds getfull payment in bankruptcy proceedings before subordinated bonds receive any payment. A potential problem may arise in that the bond covenant may restrict the company from issuing any future bonds senior to the current bonds.3. A sinking fund will reduce the coupon rate because it is a partial guaranteeto bondholders. The problem with a sinking fund is that the company must make the interim payments into a sinking fund or face default. This means the company must be able to generate these cash flows.4. A provision with a specific call date and prices would increase the couponrate. The call provision would only be used when it is to the company’s advantage, thus the bondholder’s disadvantage. The downside is theCHAPTER 7 C-29 higher coupon rate. The company benefits by being able to refinance at a lower rate if interest rates fall significantly, that is, enough to offset the call provision cost.5. A deferred call would reduce the coupon rate relative to a call provision witha deferred call. The bond will still have a higher rate relative to a plain vanillabond. The deferred call means that the company cannot call the bond for a specified period. This offers the bondholders protection for this period. The disadvantage of a deferred call is that the company cannot call the bond during the call protection period. Interest rates could potentially fall to the point where it would be beneficial for the company to call the bond, yet the company is unable to do so.6. A make-whole call provision should lower the coupon rate in comparison toa call provision with specific dates since the make-whole call repays thebondholder the present value of the future cash flows. However, a make-whole call provision should not affect the coupon rate in comparison to a plain vanilla bond. Since the bondholders are made whole, they should be indifferent between a plain vanilla bond and a make-whole bond. If a bond with a make-whole provision is called, bondholders receive the market value of the bond, which they can reinvest in another bond with similar characteristics. If we compare this to a bond with a specific call price, investors rarely receive the full market value of the future cash flows.CASE 3 C-30 7. A positive covenant would reduce the coupon rate. The presence of positivecovenants protects bondholders by forcing the company to undertake actions that benefit bondholders. Examples of positive covenants would be: the company must maintain audited financial statements; the company must maintain a minimum specified level of working capital or a minimum specified current ratio; the company must maintain any collateral in good working order. The negative side of positive covenants is that the company is restricted in its actions. The positive covenant may force the company into actions in the future that it would rather not undertake.8. A negative covenant would reduce the coupon rate. The presence ofnegative covenants protects bondholders from actions by the company that would harm the bondholders. Remember, the goal of a corporation is to maximize shareholder wealth. This says nothing about bondholders.Examples of negative covenants would be: the company cannot increase dividends, or at least increase beyond a specified level; the company cannot issue new bonds senior to the current bond issue; the company cannot sell any collateral. The downside of negative covenants is the restriction of the company’s actions.9.Even though the company is not public, a conversion feature would likelylower the coupon rate. The conversion feature would permit bondholders to benefit if the company does well and also goes public. The downside is that the company may be selling equity at a discounted price.10. The downside of a floating-rate coupon is that if interest rates rise, thecompany has to pay a higher interest rate. However, if interest rates fall, the company pays a lower interest rate.CHAPTER 8STOCK VALUATION AT RAGAN, INC.1.The total dividends paid by the company were $126,000. Since there are100,000 shares outstanding, the total earnings for the company were: Total earnings = 100,000($4.54) = $454,000This means the payout ratio was:Payout ratio = $126,000/$454,000 = 0.28So, the retention ratio was:Retention ratio = 1 – .28 = 0.72Using the retention ratio, the company’s growth rate is:g = ROE × b = 0.25*(.72) = .1806 or 18.06%The dividend per share paid this year was:= $63,000 / 50,000D= $1.26DNow we can find the stock price, which is:C-84 CASE SOLUTIONSP 0 = D 1 / (R – g )P 0 = $1.26(1.1806) / (.20 – .1806)P 0 = $76.752.Since Expert HVAC had a write off which affected its earnings per share, we need to recalculate the industry EPS. So, the industry EPS is:Industry EPS = ($0.79 + 1.38 + 1.06) / 3 = $1.08Using this industry EPS, the industry payout ratio is:Industry payout ratio = $0.40/$1.08 = .3715 or 37.15%So, the industry retention ratio isIndustry retention ratio = 1 – .3715 = .6285 or 62.85%。
公司理财第九版课后习题答案第二章
公司理财第九版课后习题答案第二章CHAPTER 2FINANCIAL STATEMENTS AND CASH FLOWAnswe rs to Concepts Review and Critical Thinking Questions1. True. Every asset can be converted to cash at some price. However, when we are referring to a liquidasset, the added assumption that the asset can be quickly converted to cash at or near market value is important.2. The recognition and matching principles in financial accounting call for revenues, and the costsassociated with producing those revenues, to be ―booked‖when the revenue process isessentiallycomplete, not necessarily when the cash is collected or bills are paid. Note that this way is notnecessarily correct; it‘s the way accountants have chosen to do it.3. The bottom line number shows the change in the ca sh balanc e on the balance sheet. As such, it is nota use ful number for analyzing a company.4. The major difference is the treatment of interest expense. The accounting statement of cash flowstreats interest as an operating ca sh flow, while the financial ca sh flows treat interest as a financing cash flow. The logic of the accounting statement of cash flows is that since interest appears on the income statement, which shows the operations for the period, it is an operating cash flow. In reality, interest is a financing expense, which results from the company‘s choice of debt and equity. We will have more to say about this in a laterchapter. When compa ring the two c ash flow statements, the financial statement of cash flows is a more appropriate measure of the company‘s performa ncebecause of its treatment of interest.5. Market values can never be negative. Imagine a share of stock selling for –$20. This would meanthat if you placed an order for 100 shares, you would get the stock along with a check for $2,000.How ma ny shares do you want to buy? More generally, because of corpora te andindividualbankruptcy laws, net worth for a person or a corporation cannot be negative, implying that liabilities cannot exceed assets in market value.6. For a successful c ompany that is rapidly expanding, for example, capital outlays will be large,possibly leading to negative c ash flow from assets. In general, what matters is whether the money is spent wisely, not whe ther cash flow from assets is positive or negative.7. It‘s probably not a good sign for an e stablished company to have negative cash flow from operations,but it would be fairly ordinary for a start-up, so it depends.would have this effect. Negative net c apital spending would mea n more long-lived assets wereliquidated than purchased.49.10. If a company raises more money from selling stock than it pays in dividends in a particular period,its cash flow to stockholders will be negative. If a companyborrows more than it pays in interest and principal, its cash flow to creditors will be negative.The adjustments discussed were purely accounting changes; they had no cash flow or market value consequences unless the new accounting information caused stockholders to revalue the derivatives.Solutions to Questions and Proble msNOTE: All end-of-chapter problems were solved using a spreadsheet. Many problems require multiplesteps. Due to space and readability constraints, when these intermediate steps are included in thissolutions manual, rounding may appear to have occurred. However, the final answer for each problem is found without rounding during any step in the problem.Basic1. To find owners‘ equity, we must construct a balance sheet as follows:Balance SheetCA $ 5,300 CL $ 3,900NFA 26,000 LTD 14,200OE ??TA $31,300 TL & OE $31,300We know that total liabilities and owners‘ equity (TL & OE) must equal total assets of $31,300. We also know that TL & OE is equal to current liabilities plus long-term debt plus owner‘s equity, soowner‘s equity is:OE = $31,300 –14,200 – 3,900 = $13,200NWC = CA – CL = $5,300 – 3,900 = $1,4002. The income statement for the company is:Income StatementSales $493,000Costs 210,000Depreciation 35,000EBIT $248,000Interest 19,000EBT $229,000Taxes 80,150Net income $148,8503.4.5.6. One equation for net income is:Net income = Dividends + Addition to retained earningsRearranging, we get:Addition to retained earnings = Net income – Divide ndsAddition to retained earnings = $148,850 – 50,000Addition to retained earnings = $98,850To find the book value of current assets, we use: NWC = CA – CL. Rearranging to solve for current assets, we get:CA = NWC + CL = $800,000 + 2,100,000 = $2,900,000The market value of current assets and net fixed assets is given, so:Book value CA= $2,900,000 Market value CA= $2,800,000Book value NFA = $5,000,000 Market value NFA= $6,300,000 Book value assets = $7,900,000 Market value assets= $9,100,000Taxes = 0.15($50K) + 0.25($25K) + 0.34($25K) + 0.39($246K – 100K)Taxes = $79,190The average tax ra te is the total tax paid divided by net income, so:Average tax rate = $79,190 / $246,000Average tax rate = 32.19%The marginal tax rate is the tax rate on the next $1 of earnings, so the marginal tax ra te = 39%.To calculate OCF, we first need the income state ment:Income StatementSales $14,900Costs 5,800Depreciation 1,300EBIT $7,800Interest 780Taxable income $7,020Taxes 2,808Net income $4,212OCF = EBIT + Depreciation – TaxesOCF = $7,800 + 1,300 – 2,808OCF = $6,292Net capital spending = $1,730,000 – 1,650,000 + 284,000Net capital spending = $364,0007. The long-term debt account will increase by $10 million, the amount of the new long-term debt issue.Since the company sold 10 million new shares of stock with a $1 par value, the common stockaccount will increase by $10 million. The capital surplus account will increase by $33 million, thevalue of the new stoc k sold above its par value. Since the company had a net income of $9million,and pa id $2 million in dividends, the addition to retained earnings was $7 million, which willinc rease the accumulated retained earnings account. So, the new long-term debt a nd stockholders‘ equity portion of the balance sheet will be:Long-term debt $82,000,000Total long-term debt $82,000,000Shareholders equityPreferred stock $9,000,000Common stock ($1 par value) 30,000,000Ac cumulated retained earnings 104,000,000Capital surplus 76,000,000Total equity $ 219,000,000Total Liabilities & Equity $ 301,000,0008.9. Cash flow to creditors = Interest paid – Net new borrowingCash flow to creditors = $118,000 – ($1,390,000 – 1,340,000) Cash flow to creditors = $118,000 – 50,000Cash flow to creditors = $68,000Cash flow to stockholders = Dividends paid – Net new equity Cash flow to stockholders = $385,000 – [(Common+ APIS) –(Common+ APIS)]end end beg beg10. Cash flow to stockholders = $385,000 –[($450,000 + 3,050,000) – ($430,000 + 2,600,000)] Cash flow to stockholders = $385,000 – ($3,500,000 – 3,030,000)Cash flow to stockholders = –$85,000Note, APIS is the additional paid-in surplus.Cash flow from assets= Cash flow to creditors + Cash flow to stockholders= $68,000 – 85,000= –$17,000Cash flow from assets= –$17,000 = OCF – Change in NWC –Net capital spending–$17,000 = OCF – (–$69,000) – 875,000Operating cash flowOperating cash flow= –$17,000 – 69,000 + 875,000= $789,000Cash flow to creditors = $118,000 – (LTD– LTD)11. a. IntermediateThe accounting statement of cash flows explains the change in cash during the year. Theaccounting statement of cash flows will be:Statement of cash flowsOperationsNet income $105Depreciation 90Changes in other current assets (55)Accounts payable (10)Total cash flow from operations $170Investing activitiesAcquisition of fixed assets $(140)Total cash flow from investing activities $(140)Financing activitiesProc eeds of long-term debt $30Dividends (45)Total cash flow from financing activities ($15)Change in cash (on balance sheet) $15b.Change in NWC= NWC e nd– NWC beg= (CA end–CL en d ) – (CA beg–CL be g)c.= [($50 + 155) – 85] – [($35 + 140) – 95)= $120 – 80= $40To find the cash flow generated by the firm‘s assets, we need the operating cash flow, and thecapital spending. So, calculating each of these, we find:Operating cash flowNet income $105Depreciation 90Operating cash flow $195Note that we can calculate OCF in this manner since there a re no taxes.Capital spendingEnding fixed assets Beginning fixed assets DepreciationCapital spending $340 (290)90 $140Now we c an calculate the cash flow gene rated by the firm‘s assets, which is: Cash flow from assetsOperating cash flow Capital spending Change in NWC Cash flow from assets $195 (140) (40) $1512. With the information provided, the cash flows from the firm are the capital spending and the changein net working capital, so:Cash flows from the firmCapital spending $(15,000)Additions to NWC (1,500)Cash flows from the firm $(16,500)And the cash flows to the investors of the firm are:Cash flows to investors of the firmSale of long-term debt (19,000)Sale of common stock (3,000)Dividends paid 19,500Cash flows to investors of the firm $(2,500)13. a.b. The interest expense for the company is the amount of debt times the interest rate on the debt. So, the income statement for the company is:Income StatementSales $1,200,000Cost of goods sold 450,000Selling costs 225,000Depreciation 110,000EBIT $415,000Interest 81,000Taxable income $334,000Taxes 116,900Net income $217,100And the opera ting cash flow is:OCF = EBIT + Depreciation – TaxesOCF = $415,000 + 110,000 – 116,900OCF = $408,10014. To find the OCF, we first calculate net income.Income StatementSales $167,000Costs 91,000Depreciation 8,000Other expe nses 5,400EBIT $62,600Interest 11,000Taxable income $51,600Taxes18,060Net income $33,540Dividends $9,500Additions to RE $24,040a.OCF = EBIT + Depreciation – TaxesOCF = $62,600 + 8,000 – 18,060OCF = $52,540b.CFC = Interest – Net new LTDCFC = $11,000 – (–$7,100)CFC = $18,100Note that the net new long-term debt is negative because the compa ny repaid part of its long-term debt.c.CFS = Dividends – Net new equityCFS = $9,500 – 7,250CFS = $2,250d.We know that CFA = CFC + CFS, so:CFA = $18,100 + 2,250 = $20,350CFA is also equal to OCF – Net capital spending – Change in NWC. We already know OCF.Net capital spending is equal to:Net capital spending = Increase in NFA + De preciationNet capital spending = $22,400 + 8,000Net capital spending = $30,400Now we c an use:CFA = OCF – Net capital spending – Change in NWC$20,350 = $52,540 – 30,400 – Change in NWC.Solving for the change in NWC gives $1,790, me aning the company increased its NWC by$1,790.15. The solution to this question works the income statement backwards. Starting at the bottom:Net income = Dividends + Addition to ret. earningsNet income = $1,530 + 5,300Net income = $6,830Now, looking at the income statement:EBT –(EBT × Tax rate) = Net incomeRecognize that EBT × tax rate is simply the calculation for ta xes. Solving this for EBT yields: EBT = NI / (1– Tax rate) EBT = $6,830 / (1 – 0.65)EBT = $10,507.69Now we can calculate:EBIT = EBT + InterestEBIT = $10,507.69 + 1,900EBIT = $12,407.69The last step is to use:EBIT = Sales – Costs – Depreciation$12,407.69 = $43,000 – 27,500 – DepreciationDepreciation = $3,092.31Solving for depreciation, we find that depreciation = $3,092.3116. The balance sheet for the company looks like this:Balance SheetCash $183,000 Accounts payableAc counts receivable 138,000 Notes payableInventory 297,000 Current liabilitiesCurrent assets $618,000 Long-term debtTotal liabilities Tangible net fixed assets 3,200,000Intangible net fixed assets 695,000 Common stockAccumulated ret. earnings Total assets $4,513,000 Total liab. & owners‘ equity Total liabilities and owners‘ equity is: TL & OE = T otal debt + Common stock + Accumulated retained earnings Solving for this equation for equity gives us: Common stock = $4,513,000 – 1,960,000 – 2,160,000Common stock = $393,000$465,000145,000 $610,000 1,550,000 $2,160,0001,960,000 $4,513,00017.18.19. The market value of shareholders‘ equity canno t be negative. A negative market value in this casewould imply that the company would pay you to own the stock. The market value of sha reholders‘ equity can be stated as: Shareholders‘ equity = Max [(TA –TL), 0]. So, if TA is $9,700, equity isequal to $800, and if TA is $6,800, e quity is equal to $0. We should note here that while the market value of equity cannot be negative, the book value of shareholders‘ equity can be negative.a.Taxes Growth= 0.15($50K) + 0.25($25K) + 0.34($3K) = $14,770Taxes Income= 0.15($50K) + 0.25($25K) + 0.34($25K) + 0.39($235K) + 0.34($7.465M)= $2,652,000b. Each firm has a marginal tax rate of 34% on the next$10,000 of taxa ble income, despite theirdifferent average ta x rates, so both firms will pay an additional $3,400 in taxes.Income State mentSales $740,000COGS 610,000A&S expenses 100,000Depreciation 140,000EBIT ($115,000)Interest 70,000Taxable income ($185,000)Taxes (35%) 0/doc/5b4332add1f34693daef3eb1.html income ($185,000)b.OCF = EBIT + Depreciation – TaxesOCF = ($115,000) + 140,000 – 0OCF = $25,00020.21. c. Net income was negative because of the tax deductibility of depreciation and interest expense.However, the actual cash flow from operations wa s positive because de preciation is a non-cashexpense and interest is a financing expense, not an operating expense.A firm can still pay out dividends if net income is negative; it just has to be sure there is sufficientcash flow to make the dividend payments.Change in NWC = Net ca pital spending = Net new equity = 0. (Given)Cash flow from assets = OCF – Change in NWC – Net capitalspendingCash flow from assets = $25,000 – 0 – 0 = $25,000Cash flow to stockholders = Divide nds – Net new equityCash flow to stockholders = $30,000 – 0 = $30,000Cash flow to creditors = Cash flow from assets – Cash flow to stockholdersCash flow to creditors = $25,000 – 30,000Cash flow to creditors = –$5,000Cash flow to creditors is also:Cash flow to creditors = Interest – Net new LTDSo:Net new LTD = Interest – Cash flow to creditorsNet new LTD = $70,000 – (–5,000)Net new LTD = $75,000a. The income statement is:Income StatementSales $15,300Cost of good sold 10,900Depreciation 2,100EBIT $ 2,300Interest 520Taxable income $ 1,780Taxes712Net income $1,068b.OCF= EBIT + Depreciation – TaxesOCF = $2,300 + 2,100 – 712OCF = $3,688c. Change in NWC=NWC end– NWC beg= (CA end–CL en d ) – (CA beg–CL be g)22.= ($3,950 – 1,950) – ($3,400 – 1,900)= $2,000 – 1,500 = $500Ne t capital spending= NFA end– NFA beg+ Depreciation= $12,900 – 11,800 + 2,100= $3,200CFA= OCF – Change in NWC – Net capital spending= $3,688 – 500 – 3,200= –$12The cash flow from assets can be positive or ne gative, since it represents whether the firm raisedfunds or distributed funds on a net basis. In this problem, even though net income and OCF arepositive, the firm invested heavily in both fixed assets and net working capital; it had to raise a net $12 in funds from its stockholders and creditors to make these investments.d. Ca sh flow to creditors= Interest – Net new LTD= $520 – 0= $520Ca sh flow to stoc kholders = Cash flow from assets – Cash flow to creditors= –$12 – 520= –$532We can also calculate the cash flow to stockholders as:Ca sh flow to stoc kholders = Dividends – Ne t new equity Solving for net new equity, we get:Net new equity= $500 – (–532)= $1,032The firm had positive earnings in an accounting sense (NI > 0) and had positive cash flow fromoperations. The firm invested $500 in new net working capital and $3,200 in new fixed assets. The firm had to raise $12 from its stakeholders to support this new inve stment. It accomplished this by raising $1,032 in the form of new equity. After paying out $500 of this in the form of dividends to shareholders and $520 in the form of interest to creditors, $12 was left to meet the firm‘s ca sh flow needs for investment.a. Total assets 2009= $780 + 3,480 = $4,260Total liabilities 2009= $318 + 1,800 = $2,118Owners‘ equity 2009 = $4,260 – 2,118 = $2,142Total assets 2010= $846 + 4,080 = $4,926Total liabilities 2010= $348 + 2,064 = $2,412Owners‘ equity 2010= $4,926 – 2,412 = $2,51414b. NWC 2009NWC 2010Change in NWC = CA09 – CL09 = $780 – 318 = $462= CA10 – CL10 = $846 – 348 = $498= NWC10 – NWC09 = $498 – 462 = $36c.d. We can calculate net capital spe nding as:Net capital spending = Net fixed assets 2010 –Net fixed assets 2009 + Deprec iationNet capital spending = $4,080 – 3,480 + 960Net capital spending = $1,560So, the company had a net capital spending cash flow of$1,560. We also know that net capital spending is:Net capital spending = Fixed assets bought –Fixed assets sold$1,560= $1,800 – Fixed assets soldFixed assets sold= $1,800 – 1,560 = $240To c alculate the cash flow from assets, we must first calculate the operating cash flow. Theoperating cash flow is calculated as follows (you can also prepare a traditional incomestatement):EBIT = Sales – Costs – DepreciationEBIT = $10,320 – 4,980 – 960EBIT = $4,380EBT = EBIT – InterestEBT = $4,380 – 259EBT = $4,121Taxes = EBT ? .35Taxes = $4,121 ? .35Taxes = $1,442OCF = EBIT + Depreciation – TaxesOCF = $4,380 + 960 – 1,442OCF = $3,898Ca sh flow from a ssets = OCF – Change in NWC – Net capital spending.Ca sh flow from a ssets = $3,898 – 36 – 1,560Ca sh flow from a ssets = $2,302Net new borrowing = LTD10 – LTD09Net new borrowing = $2,064 – 1,800Net new borrowing = $264Ca sh flow to creditors = Interest – Net ne w LTDCa sh flow to creditors = $259 – 264Ca sh flow to creditors = –$5Net new borrowing = $264 = Debt issue d – Debt retired Debt retired = $360 – 264 = $9623.CashAccounts receivable InventoryCurrent assetsNet fixed assets Total assetsCashAccounts receivable InventoryCurrent assetsNet fixed assets Total assets Balance sheet as of Dec. 31, 2009 $2,739 Accounts payable3,626 Notes payable6,447 Current liabilities$12,812Long-term debt$22,970 Owners' equity$35,782 Total liab. & equityBalance sheet as of Dec. 31, 2010$2,802Accounts payable4,085 Notes payable6,625Current liabilities$13,512Long-term debt$23,518Owners' equity$37,030Total liab. & equity$2,877529$3,406$9,173$23,203$35,782$2,790497$3,287$10,702$23,041$37,03024.2009 Income StatementSales $5,223.00COGS 1,797.00Othe r expenses 426.00Depreciation 750.00EBIT $2,250.00Interest 350.00EBT $1,900.00Taxes646.00Net income $1,254.00Dividends $637.00Additions to RE 617.00OCF = EBIT + Depreciation – TaxesOCF = $2,459 + 751 – 699.38OCF = $2,510.62Change in NWC = NWC end– NWC beg= (CA – CL)end 2010 Income StatementSales $5,606.00COGS 2,040.00Other expense s 356.00Depreciation 751.00EBIT $2,459.00Interest 402.00EBT $2,057.00Taxes699.38Net income $1,357.62Dividends $701.00Additions to RE 656.62– (CA – CL)begChange in NWC = ($13,512 – 3,287) – ($12,812 – 3,406)Change in NWC = $819Net capital spending = $23,518 – 22,970 + 751Net capital spending = $1,299Net capital spending = NFA– NFA+ Depreciation25. Cash flow from assets = OCF –Change in NWC –Net capital spendingCash flow from assets = $2,510.62 – 819 – 1,299Cash flow from assets = $396.62Cash flow to creditors = Interest – Net new LTDNet new LTD = LTD end– LTD begCash flow to creditors = $402 – ($10,702 – 9,173)Cash flow to creditors = –$1,127Common stock + Retained earnings = Total owners‘ equity Net new equity = (OE – RE)end– (OE – RE)begRE end= RE beg+ Additions to RENet new equity = $23,041 – 23,203 – 656.62 = –$818.62Cash flow to stockholders = Dividends – Net new equityCash flow to stockholders = $701 – (–$818.62)Cash flow to stockholders = $1,519.62As a check, ca sh flow from assets is $396.62.Cash flow from assets = Cash flow from creditors + Cash flow to stockholdersCash flow from assets = –$1,127 + 1,519.62Cash flow from assets = $392.62ChallengeWe will begin by calculating the operating cash flow. First, we need the EBIT, which c an becalculated as:EBIT = Net income + Current taxes + Deferred taxes + Inte restEBIT = $144 + 82 + 16 + 43EBIT = $380Now we can calculate the operating cash flow as:Operating cash flowEarnings before interest and taxes $285Depreciation 78Current taxes (82)Operating cash flow $281Net new equity = Common stock– Common stockNet new equity = OE– OE+ RE– RE∴ Net new equity= OE– OE+ RE– (RE+ Additions to RE)= OE– OE– Additions to REThe cash flow from assets is found in the investing activities portion of the accounting statement of cash flows, so: Cash flow from assetsAcquisition of fixed a ssets $148Sale of fixed assets (19)Capital spending $129The net working capital cash flows are all found in theoperations cash flow section of theaccounting statement of cash flows. However, instead of c alculating the net working capital cashflows as the change in net working capital, we must calculate each item individually. Doing so, wefind:Net working capital cash flowCash $42Accounts receivable 15Inventories (18)Accounts payable (14)Accrued expenses 7Notes payable (5)Other (2)NWC cash flow $25Except for the interest expense and note s payable, the ca sh flow to creditors is found in the financing activities of the accounting statement of cash flows. The inte rest expense from the income statementis given, so:Cash flow to creditorsInterest $43Retirement of debt 135Debt service $178Proceeds from sale of long-term debt (97)Total $81And we can find the cash flow to stockholders in the financing se ction of the accounting stateme nt of cash flows. The cash flow to stockholders was:Cash flow to stockholdersDividends $ 72Repurchase of stock 11Cash to stockholders $ 83Proceeds from new stock issue(37)Total $ 4626. Net capital spending= (NFA– NFA + Depreciation) + (Depreciation + AD) – AD= (NFA+ AD) – (NFA+ ADbeg) =FAbeg– FAend end beg beg end beg27. a.b.c. The tax bubble causes average tax rates to catch up to marginal tax rates, thus eliminating the tax advantage of low marginal rates for high inc ome corporations.Assuming a taxable income of $335,000, the taxes will be:Taxes = 0.15($50K) + 0.25($25K) + 0.34($25K) + 0.39($235K) = $113.9KAverage tax rate = $113.9K / $335K = 34%The marginal tax rate on the next dollar of income is 34 percent.For corporate taxable income levels of $335K to $10M, average tax rates are equal to marginal tax rates.Taxes = 0.34($10M) + 0.35($5M) + 0.38($3.333M) = $6,416,667Average tax rate = $6,416,667 / $18,333,334 = 35%The marginal tax rate on the ne xt dollar of income is 35 percent. For corporate taxable income levels over $18,333,334, ave rage tax rates are again e qual to marginal tax rates.Taxes= 0.34($200K) = $68K = 0.15($50K) + 0.25($25K) + 0.34($25K) + X($100K);X($100K)= $68K – 22.25K = $45.75KX= $45.75K / $100KX= 45.75%=NFA– NFAend= (NFAbeg– NFA)+ AD– AD。
公司理财Corporate_Finance_第九版_CASE答案(完整资料).doc
【最新整理，下载后即可编辑】Case SolutionsFundamentals of Corporate FinanceRoss, Westerfield, and Jordan9th editionCHAPTER 1THE McGEE CAKE COMPANY1.The advantages to a LLC are: 1) Reduction of personal liability. A soleproprietor has unlimited liability, which can include the potential loss of all personal assets. 2) Taxes. Forming an LLC may mean that more expenses can be considered business expenses and be deducted from the company’s income. 3) Improved credibility. The business may have increased credibility in the business world compared to a sole proprietorship. 4) Ability to attract investment. Corporations, even LLCs, can raise capital through the sale of equity. 5) Continuous life. Sole proprietorships have a limited life, while corporations have a potentially perpetual life. 6) Transfer of ownership. It is easier to transfer ownership in a corporation through the sale of stock.The biggest disadvantage is the potential cost, although the cost of forminga LLC can be relatively small. There are also other potential costs, includingmore expansive record-keeping.2.Forming a corporation has the same advantages as forming a LLC, but thecosts are likely to be higher.3.As a small company, changing to a LLC is probably the most advantageousdecision at the current time. If the company grows, and Doc and Lyn are willing to sell more equity ownership, the company can reorganize as a corporation at a later date. Additionally, forming a LLC is likely to be less expensive than forming a corporation.CHAPTER 2CASH FLOWS AND FINANCIAL STATEMENTS AT SUNSET BOARDS Below are the financial statements that you are asked to prepare.1.The income statement for each year will look like this:Income statement2008 2009Sales $247,259 $301,392Cost of goods sold 126,038 159,143Selling & administrative 24,787 32,352Depreciation 35,581 40,217EBIT $60,853 $69,680Interest 7,735 8,866EBT $53,118 $60,814Taxes 10,624 12,163Net income $42,494 $48,651Dividends $21,247 $24,326Addition to retainedearnings 21,247 24,3262.The balance sheet for each year will be:Balance sheet as of Dec. 31, 2008C-26 CASE SOLUTIONSCash $18,187 Accounts payable $32,143 Accountsreceivable 12,887 Notes payable 14,651 Inventory 27,119 Current liabilities $46,794 Current assets $58,193Long-term debt $79,235 Net fixed assets $156,975 Owners' equity 89,139Total assets $215,168 Total liab. &equity $215,168In the first year, equity is not given. Therefore, we must calculate equity as a plug variable. Since total liabilities & equity is equal to total assets, equity can be calculated as:Equity = $215,168 – 46,794 – 79,235Equity = $89,139CHAPTER 2 C-5Balance sheet as of Dec. 31, 2009Cash $27,478 Accounts payable $36,404 Accountsreceivable 16,717 Notes payable 15,997 Inventory 37,216 Current liabilities $52,401 Current assets $81,411Long-term debt $91,195 Net fixed assets $191,250 Owners' equity 129,065Total assets $272,661 Total liab. &equity $272,661The owner’s equity for 2009 is the beginning of year owner’s equity, plus the addition to retained earnings, plus the new equity, so:Equity = $89,139 + 24,326 + 15,600Equity = $129,065ing the OCF equation:OCF = EBIT + Depreciation – TaxesThe OCF for each year is:OCF2008 = $60,853 + 35,581 – 10,624OCF2008 = $85,180OCF2009 = $69,680 + 40,217 – 12,163OCF2009 = $97,734C-26 CASE SOLUTIONS4.To calculate the cash flow from assets, we need to find the capital spendingand change in net working capital. The capital spending for the year was: Capital spendingEnding net fixed assets $191,250– Beginning net fixedassets 156,975+ Depreciation 40,217Net capital spending $74,492And the change in net working capital was:Change in net working capitalEnding NWC $29,010– Beginning NWC 11,399Change in NWC $17,611CHAPTER 2 C-5 So, the cash flow from assets was:Cash flow from assetsOperating cash flow $97,734– Net capital spending 74,492– Change in NWC 17,611Cash flow from assets $ 5,6315.The cash flow to creditors was:Cash flow to creditorsInterest paid $8,866– Net new borrowing 11,960Cash flow to creditors –$3,0946.The cash flow to stockholders was:Cash flow tostockholdersDividends paid $24,326– Net new equityraised 15,600Cash flow tostockholders $8,726Answers to questions1.The firm had positive earnings in an accounting sense (NI > 0) and hadpositive cash flow from operations. The firm invested $17,611 in new netC-26 CASE SOLUTIONSworking capital and $74,492 in new fixed assets. The firm gave $5,631 to its stakeholders. It raised $3,094 from bondholders, and paid $8,726 to stockholders.2.The expansion plans may be a little risky. The company does have a positivecash flow, but a large portion of the operating cash flow is already going to capital spending. The company has had to raise capital from creditors and stockholders for its current operations. So, the expansion plans may be too aggressive at this time. On the other hand, companies do need capital to grow. Before investing or loaning the company money, you would want to know where the current capital spending is going, and why the company is spending so much in this area already.CHAPTER 3RATIOS ANALYSIS AT S&S AIR1.The calculations for the ratios listed are:Current ratio = $2,186,520 / $2,919,000Current ratio = 0.75 timesQuick ratio = ($2,186,250 – 1,037,120) / $2,919,000Quick ratio = 0.39 timesCash ratio = $441,000 / $2,919,000Cash ratio = 0.15 timesTotal asset turnover = $30,499,420 / $18,308,920Total asset turnover = 1.67 timesInventory turnover = $22,224,580 / $1,037,120Inventory turnover = 21.43 timesReceivables turnover = $30,499,420 / $708,400Receivables turnover = 43.05 timesTotal debt ratio = ($18,308,920 – 10,069,920) / $18,308,920 Total debt ratio = 0.45 timesDebt-equity ratio = ($2,919,000 + 5,320,000) / $10,069,920C-26 CASE SOLUTIONSDebt-equity ratio = 0.82 timesEquity multiplier = $18,308,920 / $10,069,920Equity multiplier = 1.82 timesTimes interest earned = $3,040,660 / $478,240Times interest earned = 6.36 timesCash coverage = ($3,040,660 + 1,366,680) / $478,420 Cash coverage = 9.22 timesProfit margin = $1,537,452 / $30,499,420Profit margin = 5.04%Return on assets = $1,537,452 / $18,308,920Return on assets = 8.40%Return on equity = $1,537,452 / $10,069,920Return on equity = 15.27%CHAPTER 3 C-11 2. Boeing is probably not a good aspirant company. Even though bothcompanies manufacture airplanes, S&S Air manufactures small airplanes, while Boeing manufactures large, commercial aircraft. These are two different markets. Additionally, Boeing is heavily involved in the defense industry, as well as Boeing Capital, which finances airplanes.Bombardier is a Canadian company that builds business jets, short-range airliners and fire-fighting amphibious aircraft and also provides defense-related services. It is the third largest commercial aircraft manufacturer in the world. Embraer is a Brazilian manufacturer than manufactures commercial, military, and corporate airplanes. Additionally, the Brazilian government is a part owner of the company. Bombardier and Embraer are probably not good aspirant companies because of the diverse range of products and manufacture of larger aircraft.Cirrus is the world's second largest manufacturer of single-engine, piston-powered aircraft. Its SR22 is the world's best selling plane in its class. The company is noted for its innovative small aircraft and is a good aspirant company.Cessna is a well known manufacturer of small airplanes. The company produces business jets, freight- and passenger-hauling utility Caravans, personal and small-business single engine pistons. It may be a good aspirant company, however, its products could be considered too broad and diversified since S&S Air produces only small personal airplanes.3. S&S is below the median industry ratios for the current and cash ratios.This implies the company has less liquidity than the industry in general.However, both ratios are above the lower quartile, so there are companiesC-26 CASE SOLUTIONSin the industry with lower liquidity ratios than S&S Air. The company may have more predictable cash flows, or more access to short-term borrowing.If you created an Inventory to Current liabilities ratio, S&S Air would havea ratio that is lower than the industry median. The current ratio is below theindustry median, while the quick ratio is above the industry median. This implies that S&S Air has less inventory to current liabilities than the industry median. S&S Air has less inventory than the industry median, but more accounts receivable than the industry since the cash ratio is lower than the industry median.The turnover ratios are all higher than the industry median; in fact, all three turnover ratios are above the upper quartile. This may mean that S&S Air is more efficient than the industry.The financial leverage ratios are all below the industry median, but above the lower quartile. S&S Air generally has less debt than comparable companies, but still within the normal range.The profit margin, ROA, and ROE are all slightly below the industry median, however, not dramatically lower. The company may want to examine its costs structure to determine if costs can be reduced, or price can be increased.Overall, S&S Air’s performance seems good, although the liquidity ratios indicate that a closer look may be needed in this area.CHAPTER 3 C-11 Below is a list of possible reasons it may be good or bad that each ratio is higher or lower than the industry. Note that the list is not exhaustive, but merely one possible explanation for each ratio.Ratio Good BadCurrent ratio Better at managingcurrent accounts. May be having liquidity problems.Quick ratio Better at managingcurrent accounts. May be having liquidity problems.Cash ratio Better at managingcurrent accounts. May be having liquidity problems.Total asset turnover Better at utilizing assets. Assets may be older anddepreciated, requiringextensive investmentsoon.Inventory turnover Better at inventorymanagement, possibly dueto better procedures.Could be experiencinginventory shortages.Receivables turnover Better at collectingreceivables.May have credit termsthat are too strict.Decreasing receivablesturnover may increasesales.Total debt ratio Less debt than industrymedian means thecompany is less likely toexperience creditproblems. Increasing the amount of debt can increase shareholder returns. Especially notice that it will increase ROE.Debt-equity Less debt than industry Increasing the amount ofC-26 CASE SOLUTIONSratio median means thecompany is less likely toexperience creditproblems. debt can increase shareholder returns. Especially notice that it will increase ROE.Equity multiplier Less debt than industrymedian means thecompany is less likely toexperience creditproblems.Increasing the amount ofdebt can increaseshareholder returns.Especially notice that itwill increase ROE.TIE Higher quality materialscould be increasing costs. The company may have more difficulty meeting interest payments in a downturn.Cash coverage Less debt than industrymedian means thecompany is less likely toexperience creditproblems. Increasing the amount of debt can increase shareholder returns. Especially notice that it will increase ROE.Profit margin The PM is slightly belowthe industry median. Itcould be a result of higherquality materials or bettermanufacturing. Company may be having trouble controlling costs.ROA Company may have newerassets than the industry. Company may have newer assets than the industry.ROE Lower profit margin maybe a result of higherquality. Profit margin and EM are lower than industry, which results in the lower ROE.CHAPTER 4PLANNING FOR GROWTH AT S&S AIR1.To calculate the internal growth rate, we first need to find the ROA and theretention ratio, so:ROA = NI / TAROA = $1,537,452 / $18,309,920ROA = .0840 or 8.40%b = Addition to RE / NIb = $977,452 / $1,537,452b = 0.64Now we can use the internal growth rate equation to get:Internal growth rate = (ROA × b) / [1 – (ROA × b)]Internal growth rate = [0.0840(.64)] / [1 – 0.0840(.64)]Internal growth rate = .0564 or 5.64%To find the sustainable growth rate, we need the ROE, which is:ROE = NI / TEROE = $1,537,452 / $10,069,920ROE = .1527 or 15.27%C-26 CASE SOLUTIONSUsing the retention ratio we previously calculated, the sustainable growth rate is:Sustainable growth rate = (ROE × b) / [1 – (ROE × b)]Sustainable growth rate = [0.1527(.64)] / [1 – 0.1527(.64)]Sustainable growth rate = .1075 or 10.75%The internal growth rate is the growth rate the company can achieve with no outside financing of any sort. The sustainable growth rate is the growth rate the company can achieve by raising outside debt based on its retained earnings and current capital structure.CHAPTER 4 C-21 2.Pro forma financial statements for next year at a 12 percent growth rate are:Income statementSales $ 34,159,35COGS 24,891,530 Other expenses 4,331,600 Depreciation 1,366,680EBIT $ 3,569,541Interest 478,240Taxable income $ 3,091,301Taxes (40%) 1,236,520Net income $ 1,854,78Dividends $ 675,583C-26 CASE SOLUTIONSAdd to RE 1,179,197Balance sheetAssets Liabilities & EquityCurrent Assets Current LiabilitiesCash $ 493,92AccountsPayable $ 995,680Accounts rec. 793,408 Notes Payable 2,030,000 Inventory 1,161,574 Total CL $ 3,025,680 Total CA $ 2,448,902Long-term debt $ 5,320,000ShareholderEquityCommon stock $ 350,000Fixed assets Retainedearnings 10,899,117Net PP&E $ 18,057,088 Total Equity $ 11,249,117Total Assets $ 20,505,990 Total L&E $ 19,594,787CHAPTER 4 C-21 So, the EFN is:EFN = Total assets – Total liabilities and equityEFN = $20,505,990 – 19,594,797EFN = $911,193The company can grow at this rate by changing the way it operates. For example, if profit margin increases, say by reducing costs, the ROE increases, it will increase the sustainable growth rate. In general, as long as the company increases the profit margin, total asset turnover, or equity multiplier, the higher growth rate is possible. Note however, that changing any one of these will have the effect of changing the pro forma financial statements.C-26 CASE SOLUTIONS3.Now we are assuming the company can only build in amounts of $5 million.We will assume that the company will go ahead with the fixed asset acquisition. To estimate the new depreciation charge, we will find the current depreciation as a percentage of fixed assets, then, apply this percentage to the new fixed assets. The depreciation as a percentage of assets this year was:Depreciation percentage = $1,366,680 / $16,122,400Depreciation percentage = .0848 or 8.48%The new level of fixed assets with the $5 million purchase will be:New fixed assets = $16,122,400 + 5,000,000 = $21,122,400So, the pro forma depreciation will be:Pro forma depreciation = .0848($21,122,400)Pro forma depreciation = $1,790,525We will use this amount in the pro forma income statement. So, the pro forma income statement will be:Income statementSales $ 34,159,35COGS 24,891,530 Other expensesCHAPTER 4 C-214,331,600Depreciation 1,790,525EBIT $ 3,145,696Interest 478,240Taxable income $ 2,667,456Taxes (40%) 1,066,982Net income $ 1,600,473Dividends $ 582,955Add to RE 1,017,519C-26 CASE SOLUTIONSThe pro forma balance sheet will remain the same except for the fixed asset and equity accounts. The fixed asset account will increase by $5 million, rather than the growth rate of sales.Balance sheetAssets Liabilities & EquityCurrent Assets Current LiabilitiesCash $ 493,92AccountsPayable $ 995,680Accounts rec. 793,408 Notes Payable 2,030,000 Inventory 1,161,574 Total CL $ 3,025,680 Total CA $ 2,448,902Long-term debt $ 5,320,000ShareholderEquityCommon stock $ 350,000Fixed assets Retainedearnings 10,737,439Net PP&E $ 21,122,400 Total Equity $ 11,087,439Total Assets $ 23,571,302 Total L&E $ 19,433,119CHAPTER 4 C-21 So, the EFN is:EFN = Total assets – Total liabilities and equityEFN = $23,581,302 – 19,433,119EFN = $4,138,184Since the fixed assets have increased at a faster percentage than sales, the capacity utilization for next year will decrease.CHAPTER 6THE MBA DECISION1. Age is obviously an important factor. The younger an individual is, the moretime there is for the (hopefully) increased salary to offset the cost of the decision to return to school for an MBA. The cost includes both the explicit costs such as tuition, as well as the opportunity cost of the lost salary.2. Perhaps the most important nonquantifiable factors would be whether ornot he is married and if he has any children. With a spouse and/or children, he may be less inclined to return for an MBA since his family may be less amenable to the time and money constraints imposed by classes. Other factors would include his willingness and desire to pursue an MBA, job satisfaction, and how important the prestige of a job is to him, regardless of the salary.3.He has three choices: remain at his current job, pursue a Wilton MBA, orpursue a Mt. Perry MBA. In this analysis, room and board costs are irrelevant since presumably they will be the same whether he attends college or keeps his current job. We need to find the aftertax value of each, so:Remain at current job:Aftertax salary = $55,000(1 – .26) = $40,700CHAPTER 6 C-27 His salary will grow at 3 percent per year, so the present value of his aftertax salary is:PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]PV = $40,700{[1 – [(1 +.065)/(1 + .03)]38} / (.065 – .03)PV = $836,227.34Wilton MBA:Costs:Total direct costs = $63,000 + 2,500 + 3,000 = $68,500PV of direct costs = $68,500 + 68,500 / (1.065) = $132,819.25PV of indirect costs (lost salary) = $40,700 / (1.065) + $40,700(1 + .03) / (1 + .065)2 = $75,176.00Salary:PV of aftertax bonus paid in 2 years = $15,000(1 –.31) / 1.0652= $9,125.17Aftertax salary = $98,000(1 – .31) = $67,620C-26 CASE SOLUTIONSHis salary will grow at 4 percent per year. We must also remember that he will now only work for 36 years, so the present value of his aftertax salary is: PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]PV = $67,620{[1 – [(1 +.065)/(1 + .04)]36} / (.065 – .04)PV = $1,554,663.22Since the first salary payment will be received three years from today, so we need to discount this for two years to find the value today, which will be: PV = $1,544,663.22 / 1.0652PV = $1,370,683.26So, the total value of a Wilton MBA is:Value = –$75,160 – 132,819.25 + 9,125.17 + 1,370,683.26 =$1,171,813.18Mount Perry MBA:Costs:Total direct costs = $78,000 + 3,500 + 3,000 = $86,500. Note, this is also the PV of the direct costs since they are all paid today.PV of indirect costs (lost salary) = $40,700 / (1.065) = $38,215.96Salary:CHAPTER 6 C-27 PV of aftertax bonus paid in 1 year = $10,000(1 – .29) / 1.065 = $6,666.67 Aftertax salary = $81,000(1 – .29) = $57,510His salary will grow at 3.5 percent per year. We must also remember that he will now only work for 37 years, so the present value of his aftertax salary is: PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]PV = $57,510{[1 – [(1 +.065)/(1 + .035)]37} / (.065 – .035)PV = $1,250,991.81Since the first salary payment will be received two years from today, so we need to discount this for one year to find the value today, which will be:PV = $1,250,991.81 / 1.065PV = $1,174,640.20So, the total value of a Mount Perry MBA is:Value = –$86,500 – 38,215.96 + 6,666.67 + 1,174,640.20 = $1,056,590.90C-26 CASE SOLUTIONS4.He is somewhat correct. Calculating the future value of each decision willresult in the option with the highest present value having the highest future value. Thus, a future value analysis will result in the same decision. However, his statement that a future value analysis is the correct method is wrong since a present value analysis will give the correct answer as well.5. To find the salary offer he would need to make the Wilton MBA asfinancially attractive as the as the current job, we need to take the PV of his current job, add the costs of attending Wilton, and the PV of the bonus on an aftertax basis. So, the necessary PV to make the Wilton MBA the same as his current job will be:PV = $836,227.34 + 132,819.25 + 75,176.00 – 9,125.17 = $1,035,097.42This PV will make his current job exactly equal to the Wilton MBA on a financial basis. Since his salary will still be a growing annuity, the aftertax salary needed is:PV = C {1 – [(1 + g)/(1 + r)]t} / (r–g)]$1,035,097.42 = C {[1 – [(1 +.065)/(1 + .04)]36} / (.065 – .04)C = $45,021.51This is the aftertax salary. So, the pretax salary must be:Pretax salary = $45,021.51 / (1 – .31) = $65,248.576.The cost (interest rate) of the decision depends on the riskiness of the use offunds, not the source of the funds. Therefore, whether he can pay cash orCHAPTER 6 C-27 must borrow is irrelevant. This is an important concept which will be discussed further in capital budgeting and the cost of capital in later chapters.CHAPTER 7FINANCING S&S AIR’S EXPANSION PLANS WITH A BOND ISSUEA rule of thumb with bond provisions is to determine who benefits by theprovision. If the company benefits, the bond will have a higher coupon rate.If the bondholders benefit, the bond will have a lower coupon rate.1. A bond with collateral will have a lower coupon rate. Bondholders have theclaim on the collateral, even in bankruptcy. Collateral provides an asset that bondholders can claim, which lowers their risk in default. The downside of collateral is that the company generally cannot sell the asset used as collateral, and they will generally have to keep the asset in good working order.2.The more senior the bond is, the lower the coupon rate. Senior bonds getfull payment in bankruptcy proceedings before subordinated bonds receive any payment. A potential problem may arise in that the bond covenant may restrict the company from issuing any future bonds senior to the current bonds.3. A sinking fund will reduce the coupon rate because it is a partial guaranteeto bondholders. The problem with a sinking fund is that the company must make the interim payments into a sinking fund or face default. This means the company must be able to generate these cash flows.4. A provision with a specific call date and prices would increase the couponrate. The call provision would only be used when it is to the company’s advantage, thus the bondholder’s disadvantage. The downside is theCHAPTER 7 C-29 higher coupon rate. The company benefits by being able to refinance at a lower rate if interest rates fall significantly, that is, enough to offset the call provision cost.5. A deferred call would reduce the coupon rate relative to a call provision witha deferred call. The bond will still have a higher rate relative to a plain vanillabond. The deferred call means that the company cannot call the bond for a specified period. This offers the bondholders protection for this period. The disadvantage of a deferred call is that the company cannot call the bond during the call protection period. Interest rates could potentially fall to the point where it would be beneficial for the company to call the bond, yet the company is unable to do so.6. A make-whole call provision should lower the coupon rate in comparison toa call provision with specific dates since the make-whole call repays thebondholder the present value of the future cash flows. However, a make-whole call provision should not affect the coupon rate in comparison to a plain vanilla bond. Since the bondholders are made whole, they should be indifferent between a plain vanilla bond and a make-whole bond. If a bond with a make-whole provision is called, bondholders receive the market value of the bond, which they can reinvest in another bond with similar characteristics. If we compare this to a bond with a specific call price, investors rarely receive the full market value of the future cash flows.CASE 3 C-30 7. A positive covenant would reduce the coupon rate. The presence of positivecovenants protects bondholders by forcing the company to undertake actions that benefit bondholders. Examples of positive covenants would be: the company must maintain audited financial statements; the company must maintain a minimum specified level of working capital or a minimum specified current ratio; the company must maintain any collateral in good working order. The negative side of positive covenants is that the company is restricted in its actions. The positive covenant may force the company into actions in the future that it would rather not undertake.8. A negative covenant would reduce the coupon rate. The presence ofnegative covenants protects bondholders from actions by the company that would harm the bondholders. Remember, the goal of a corporation is to maximize shareholder wealth. This says nothing about bondholders.Examples of negative covenants would be: the company cannot increase dividends, or at least increase beyond a specified level; the company cannot issue new bonds senior to the current bond issue; the company cannot sell any collateral. The downside of negative covenants is the restriction of the company’s actions.9.Even though the company is not public, a conversion feature would likelylower the coupon rate. The conversion feature would permit bondholders to benefit if the company does well and also goes public. The downside is that the company may be selling equity at a discounted price.10. The downside of a floating-rate coupon is that if interest rates rise, thecompany has to pay a higher interest rate. However, if interest rates fall, the company pays a lower interest rate.CHAPTER 8STOCK VALUATION AT RAGAN, INC.1.The total dividends paid by the company were $126,000. Since there are100,000 shares outstanding, the total earnings for the company were: Total earnings = 100,000($4.54) = $454,000This means the payout ratio was:Payout ratio = $126,000/$454,000 = 0.28So, the retention ratio was:Retention ratio = 1 – .28 = 0.72Using the retention ratio, the company’s growth rate is:g = ROE × b = 0.25*(.72) = .1806 or 18.06%The dividend per share paid this year was:= $63,000 / 50,000D= $1.26DNow we can find the stock price, which is:C-84 CASE SOLUTIONSP 0 = D 1 / (R – g )P 0 = $1.26(1.1806) / (.20 – .1806)P 0 = $76.752.Since Expert HVAC had a write off which affected its earnings per share, we need to recalculate the industry EPS. So, the industry EPS is:Industry EPS = ($0.79 + 1.38 + 1.06) / 3 = $1.08Using this industry EPS, the industry payout ratio is:Industry payout ratio = $0.40/$1.08 = .3715 or 37.15%So, the industry retention ratio isIndustry retention ratio = 1 – .3715 = .6285 or 62.85%。
罗斯《公司理财》(第9版)笔记和课后习题(含考研真题)详解
罗斯《公司理财》（第9版）笔记和课后习题详解第1章公司理财导论1.1复习笔记公司的首要目标——股东财富最大化决定了公司理财的目标。
公司理财研究的是稀缺资金如何在企业和市场内进行有效配置，它是在股份有限公司已成为现代企业制度最主要组织形式的时代背景下，就公司经营过程中的资金运动进行预测、组织、协调、分析和控制的一种决策与管理活动。
从决策角度来讲，公司理财的决策内容包括投资决策、筹资决策、股利决策和净流动资金决策；从管理角度来讲，公司理财的管理职能主要是指对资金筹集和资金投放的管理。
公司理财的基本内容包括：投资决策（资本预算）、融资决策（资本结构）、短期财务管理（营运资本）。
1．资产负债表资产负债表是总括反映企业某一特定日期财务状况的会计报表，它是根据资产、负债和所有者权益之间的相互关系，按照一定的分类标准和一定的顺序，把企业一定日期的资产、负债和所有者权益各项目予以适当排列，并对日常工作中形成的大量数据进行高度浓缩整理后编制而成的。
资产负债表可以反映资本预算、资本支出、资本结构以及经营中的现金流量管理等方面的内容。
2．资本结构资本结构是指企业各种资本的构成及其比例关系，它有广义和狭义之分。
广义资本结构，亦称财务结构，指企业全部资本的构成，既包括长期资本，也包括短期资本（主要指短期债务资本）。
狭义资本结构，主要指企业长期资本的构成，而不包括短期资本。
通常人们将资本结构表示为债务资本与权益资本的比例关系（D/E）或债务资本在总资本的构成（D/A）。
准确地讲，企业的资本结构应定义为有偿负债与所有者权益的比例。
资本结构是由企业采用各种筹资方式筹集资本形成的。
筹资方式的选择及组合决定着企业资本结构及其变化。
资本结构是企业筹资决策的核心问题。
企业应综合考虑影响资本结构的因素，运用适当方法优化资本结构，从而实现最佳资本结构。
资本结构优化有利于降低资本成本，获取财务杠杆利益。
3．财务经理财务经理是公司管理团队中的重要成员，其主要职责是通过资本预算、融资和资产流动性管理为公司创造价值。
公司理财中文版第九版第一章答案[优秀范文5篇]
![公司理财中文版第九版第一章答案[优秀范文5篇]](https://img.taocdn.com/s3/m/944feb428f9951e79b89680203d8ce2f00666520.png)
公司理财中文版第九版第一章答案[优秀范文5篇]第一篇：公司理财中文版第九版第一章答案第一章公司理财导论思考与练习答案1、谁拥有公司？描述所有者控制公司管理层的过程。
代理关系在公司的组织形式中存在的主要原因是什么？在这种环境下，可能出现什么样的问题？答：在公司制企业中，公司归股东所有。
股东选出公司董事会成员，再由董事会任命公司的管理者。
公司制企业中所有权与控制权的分离是产生代理问题的原因所在。
管理者也许会根据自己或其他一些人的最大利益行事，而不是为了股东利益。
若果真如此，也许就会与公司股票价格最大化的目标相矛盾。
2、假设你是一家非盈利企业（或许是非盈利医院）的财务经理，你认为什么样的财务管理目标将是恰当的？答：这些组织经常追求社会或政治使命，因此，有许多不同的目标是可以想象的。
经常被引用的一个目标就是利润最小化，即以尽可能低的价格向社会提供商品和服务。
也许一个更佳的视角是，我们观察到即使非营利性组织也有权益，因此，一个答案就是，恰当的目标是最大化权益的价值。
3、评价下面这句话：管理者不应该只关注现在的股票价格，应为这么做将会导致过分强调短期利润而牺牲长期利润。
答：据推测，当前的股票价值反映了未来（包括短期和长期）所有现金流的风险、现金流产生的时间以及现金流量的数量。
如果这是正确的，那么该陈述就错了。
4、股票价值最大化的目标可能和其他目标，比如避免不道德或者非法行为相冲突吗？特别是，你认为顾客和员工的安全、环境和社会的总体利益是否在这个框架之内，或者他们完全被忽略了？考虑一些具体的情形来阐述你的回答。
答：可以以另一种方式来讨论这个问题。
在一个极端，我们可以认为在市场经济中，所有的东西都被定价，因此存在一个合乎道德的行为和（或）非法行为的最优水平，股票估值框架明确包含这些内容。
在另一个极端，我们可以认为这些不是经济现象，最好由政治程序来处理。
一个解释此争论的经典（也是高度相关的）问题是这样表述的：“一个企业估计改进其产品安全性的成本是3000万美元，然而，该企业相信改进其产品安全性只能节省2000万美元的产品赔偿责任，企业应该怎么做呢？”5、股票价值最大化的财务管理目标在国外会有不同吗？为什么？答：股票价值最大化的财务管理目标在外国是一样的，但因各国的社会、政治、经济制度的不同，实现该目标的最优措施也可能会有所不同。
第九版-公司理财-罗斯-中文答案-
1、如果项目带来的是常规的现金流，而且其回收期短于该项目的生命周期，还不能准备判断其净现值的正负。
仍需要其采用的折现率和其内部收益率IRR 做对比。
当折现率小于IRRA 时，净现值为正值，当折现率大于IRRA时，净现值为负值，两者相等时，净现值为零。
如果一个项目的折现回收期短于该项目的生命周期，则净现值一定为正值。
2、项目有常规的现金流，且NPV为正值，则各期流入的现金流折现总和一定大于期初项目资金流出。
而各期流入的现金流总和肯定大于折现总和，所以该项目的回收期一定短于其生命周期。
同时折现回收期是用和净现值同样的NPV计算出来的，所以折现回收期也一定短于其生命周期。
同样净现值为正值，说明初始投资所带来的后续现金流的现值大于初始投资，所以盈利指数PI 一定大于1。
如果使用内部收益率折现各期现金流量时，净现值为零。
而以折现率折现各期现金流量时，净现值为正，说明折现率小于内部收益率。
3、a 回收期是指投资引起的现金流入累计到与投资相等所需要的时间。
它代表收回投资所需要的年限。
回收年限越短，方案越有利。
其缺陷就是忽略了回收期内现金流量的时间序列，也忽略了回收期以后的现金支付，同时对于回收期的选择也存在主观臆断。
选择一个具体的回收期决策标准，当项目的回收期小于标准的就可行，大于标准的则拒绝。
b 平均会计收益率是指为扣除所得税和折旧之后的项目平均收益除以整个项目期限内的平均账面投资额。
其缺陷是使用账面收益而非现金流量，忽略了折旧对现金流量的影响，忽视了净收益的时间分布对项目经济价值的影响。
当项目的平均会计收益率小于目标平均会计收益率时，则拒绝项目，反之接受。
c 内部收益率就是令项目净现值为0的折现率。
其缺点是对于特殊项目无法用一般原则进行判断，并且有些项目可能会出现多个收益率的现象。
同时对于互斥项目容易忽视其规模问题和时间序列问题。
一般原则是当折现率小于IRR时，接受该项目，反之则拒绝。
d 盈利指数是初始投资所带来的后续现金流的现值和初始投资的比值。
公司理财原书第九版中文版课后答案
公司理财原书第九版中文版课后答案第1章1、在所有权形式的公司中，股东是公司的所有者。
股东选举公司的董事会,董事会任命该公司的管理层。
企业的所有权和控制权分离的组织形式是导致的代理关系存在的主要原因。
管理者可能追求自身或别人的利益最大化，而不是股东的利益最大化。
在这种环境下，他们可能因为目标不一致而存在代理问题。
2、非营利公司经常追求社会或政治任务等各种目标。
非营利公司财务管理的目标是获取并有效使用资金以最大限度地实现组织的社会使命。
3、这句话是不正确的。
管理者实施财务管理的目标就是最大化现有股票的每股价值，当前的股票价值反映了短期和长期的风险、时间以及未来现金流量。
4、有两种结论。
一种极端，在市场经济中所有的东西都被定价。
因此所有目标都有一个最优水平，包括避免不道德或非法的行为，股票价值最大化。
另一种极端，我们可以认为这是非经济现象,最好的处理方式是通过政治手段。
一个经典的思考问题给出了这种争论的答案：公司估计提高某种产品安全性的成本是30美元万。
然而,该公司认为提高产品的安全性只会节省20美元万。
请问公司应该怎么做呢?”5、财务管理的目标都是相同的，但实现目标的最好方式可能是不同的，因为不同的国家有不同的社会、政治环境和经济制度。
6、管理层的目标是最大化股东现有股票的每股价值。
如果管理层认为能提高公司利润，使股价超过35美元,那么他们应该展开对恶意收购的斗争。
如果管理层认为该投标人或其它未知的投标人将支付超过每股35美元的价格收购公司,那么他们也应该展开斗争。
然而,如果管理层不能增加企业的价值并且没有其他更高的投标价格,那么管理层不是在为股东的最大化权益行事。
现在的管理层经常在公司面临这些恶意收购的情况时迷失自己的方向。
7、其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。
较少的私人投资者能减少不同的企业目标。
高比重的机构所有权导致高学历的股东和管理层讨论决策风险项目。
此外,机构投资者比私人投资者可以根据自己的资源和经验更好地对管理层实施有效的监督机制。
公司管理系统理财第九版中文问题详解
第一章1.在所有权形式的公司中,股东是公司的所有者。
股东选举公司的董事会,董事会任命该公司的管理层。
企业的所有权和控制权分离的组织形式是导致的代理关系存在的主要原因。
管理者可能追求自身或别人的利益最大化,而不是股东的利益最大化。
在这种环境下,他们可能因为目标不一致而存在代理问题。
2.非营利公司经常追求社会或政治任务等各种目标。
非营利公司财务管理的目标是获取并有效使用资金以最大限度地实现组织的社会使命。
3.这句话是不正确的。
管理者实施财务管理的目标就是最大化现有股票的每股价值，当前的股票价值反映了短期和长期的风险、时间以及未来现金流量。
4.有两种结论。
一种极端,在市场经济中所有的东西都被定价。
因此所有目标都有一个最优水平，包括避免不道德或非法的行为，股票价值最大化。
另一种极端,我们可以认为这是非经济现象,最好的处理方式是通过政治手段。
一个经典的思考问题给出了这种争论的答案:公司估计提高某种产品安全性的成本是30美元万。
然而,该公司认为提高产品的安全性只会节省20美元万。
请问公司应该怎么做呢?”5.财务管理的目标都是相同的,但实现目标的最好方式可能是不同的,因为不同的国家有不同的社会、政治环境和经济制度。
6.管理层的目标是最大化股东现有股票的每股价值。
如果管理层认为能提高公司利润，使股价超过35美元,那么他们应该展开对恶意收购的斗争。
如果管理层认为该投标人或其它未知的投标人将支付超过每股35美元的价格收购公司,那么他们也应该展开斗争。
然而,如果管理层不能增加企业的价值,并且没有其他更高的投标价格,那么管理层不是在为股东的最大化权益行事。
现在的管理层经常在公司面临这些恶意收购的情况时迷失自己的方向。
7.其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。
较少的私人投资者能减少不同的企业目标。
高比重的机构所有权导致高学历的股东和管理层讨论决策风险项目。
此外,机构投资者比私人投资者可以根据自己的资源和经验更好地对管理层实施有效的监督机制。
- 1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。
第一章
1.在所有权形式的公司中,股东是公司的所有者。
股东选举公司的董事会,董事会任命该公司的管理层。
企业的所有权和控制权分离的组织形式是导致的代理关系存在的主要原因。
管理者可能追求自身或别人的利益最大化,而不是股东的利益最大化。
在这种环境下,他们可能因为目标不一致而存在代理问题。
2.非营利公司经常追求社会或政治任务等各种目标。
非营利公司财务管理的目标是获取并有效使用资金以最大限度地实现组织的社会使命。
3.这句话是不正确的。
管理者实施财务管理的目标就是最大化现有股票的每股价值，当前的股票价值反映了短期和长期的风险、时间以及未来现金流量。
4.有两种结论。
一种极端,在市场经济中所有的东西都被定价。
因此所有目标都有一个最优水平，包括避免不道德或非法的行为，股票价值最大化。
另一种极端,我们可以认为这是非经济现象,最好的处理方式是通过政治手段。
一个经典的思考问题给出了这种争论的答案:公司估计提高某种产品安全性的成本是30美元万。
然而,该公司认为提高产品的安全性只会节省20美元万。
请问公司应该怎么做呢?”
5.财务管理的目标都是相同的,但实现目标的最好方式可能是不同的,因为不同的国家有不同的社会、政治环境和经济制度。
6.管理层的目标是最大化股东现有股票的每股价值。
如果管理层认为能提高公司利润，使股价超过35美元,那么他们应该展开对恶意收购的斗争。
如果管理层认为该投标人或其它未知的投标人将支付超过每股35美元的价格收购公司,那么他们也应该展开斗争。
然而,如果管理层不能增加企业的价值,并且没有其他更高的投标价格,那么管理层不是在为股东的最大化权益行事。
现在的管理层经常在公司面临这些恶意收购的情况时迷失自己的方向。
7.其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。
较少的私人投资者能减少不同的企业目标。
高比重的机构所有权导致高学历的股东和管理层讨论决策风险项目。
此外,机构投资者比私人投资者可以根据自己的资源和经验更好地对管理层实施有效的监督机制。
8.大型金融机构成为股票的主要持有者可能减少美国公司的代理问题，形成更有效率的公司控制权市场。
但也不一定能。
如果共同基金或者退休基金的管理层并不关心的投资者的利益，代理问题可能仍然存在,甚至有可能增加基金和投资者之间的代理问题。
9.就像市场需求其他劳动力一样，市场也需求首席执行官，首席执行官的薪酬是由市场决定的。
这同样适用于运动员和演员。
首席执行官薪酬大幅度增长的一个主要原因是现在越来越多的公司实行股票报酬，这样的改革是为了更好的协调股东和管理者的利益。
这些报酬有时被认为仅仅对股票价格上涨的回报,而不是对管理能力的奖励。
或许在将来,高管薪酬仅用来奖励特别的能力,即,股票价格的上涨增加了超过一般的市场。
10.最大化现在公司股票的价格和最大化未来股票价格是一样的。
股票的价值取决于公司未来所有的现金流量。
从另一方面来看,支付大量的现金股利给股东,股票的预期价格将会上升。
第二章
1.正确。
所有的资产都可以以某种价格转换为现金。
但是提及流动资产,假定该资产转换为现金时可达到或接近其市场价值是很重要的。
2.按公认会计原则中配比准则的要求，收入应与费用相配比，这样，在收入发生或应计的时候，即使没有现金流量，也要在利润表上报告。
注意,这种方式是不正确的;但是会计必须这么做。
3.现金流量表最后一栏数字表明了现金流量的变化。
这个数字对于分析一家公司并没有太大的作用。
4.两种现金流量主要的区别在于利息费用的处理。
会计现金流量将利息作为营运现金流量,而财务现金流量将利息作为财务现金流量。
会计现金流量的逻辑是，利息在利润表的营运阶段出现，因此利息是营运现金流量。
事实上,利息是财务费用,这是公司对负债和权益的选择的结果。
比较这两种现金流量，财务现金流量更适合衡量公司业绩。
5.市场价值不可能为负。
想象某种股票价格为- 20美元。
这就意味着如果你订购100股的股票,你会损失两万美元的支票。
你会想要买多少这种股票?根据企业和个人破产法,个人或公司的净值不能为负,这意味着负债不能超过资产的市场价值。
6.比如，作为一家成功并且飞速发展的公司，资本支出是巨大的,可能导致负的资产现金流量。
一般来说,最重要的问题是资本使用是否恰当,而不是资产的现金流量是正还是负。
7.对于已建立的公司出现负的经营性现金流量可能不是好的表象,但对于刚起步的公司这种现象是很正常的。