Igcse 数学 历年真题
IGCSE数学
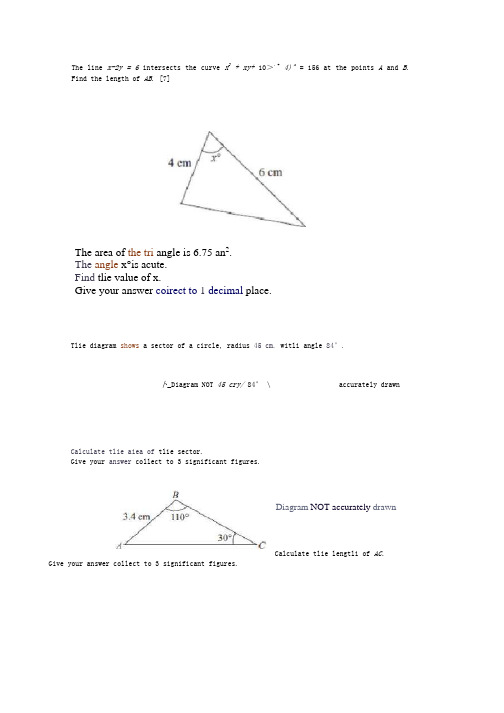
The line x-2y = 6 intersects the curve x2 + xy+ 10>,+4)^ = 156 at the points A and B.Find the length of AB. [7]The area of the tri angle is 6.75 an2.The angle x°is acute.Find tlie value of x.Give your answer coirect to 1 decimal place.Tlie diagram shows a sector of a circle, radius 45 cm. witli angle 84°.卜_Diagram NOT 45 cry/84° \ accurately drawnCalculate tlie aiea of tlie sector.Give your answer collect to 3 significant figures.Diagram NOT accurately drawnCalculate tlie lengtli of AC.Give your answer collect to 3 significant figures.A cone has slant height 4 cm and base radius r cm.In the diagram the lines AB and CD are parallel. The lines AD and BC intersect at X.Angle XDC = 35。
and angle CXD = 120°. (a) (i) Write down the size of angle BAX.Answer(a)( i) Angle BAX = .................................. [........................................................................... 1 ](ii) Write down the size of angle ABX.Answer(a)( ii) Angle ABX = ............................... [1 .............................................................................. ](b) Complete the statementTriangle AXB is .................................................... t o triangle DXC. [1 ](c) AB — 8.3cm, BX — 5.5cm and CD — 16.6cm.Calculate the length of CX.Diagram NOT accurately drawnTlie total surface area of tlie cone is33 2—兀 cnr.4Calculate the value of r.NOT TOSCALEIn quadrilateral ABCD, AB = 77 m, BC = 120 m, CD = 60 m and diagonal AC = 55 m. Angle CAD = 45°, angle BAC = x° and angle ADC = y°. (a) Calculate the value of x. (b) Calculate the value of y.(c) The bearing of D from A is 090°. Find the bearing of(i) A from C, (ii) B from A.NOTTG SCALEDiagram NOT accurately drawnA, B, C and D are four points on the circumference of a circle. The chords AC and BDintersect at E.AE =3.6 ctn. CE = 2.8 cm, DE = 2.4 cm and AD = 4.9 cm. (a' Calculate the length of BE.(b‘ Calculate the size of angle AED.Give your answer correct to 3 significant figures, cABCD is a cyclic quadrilateral.AB = 9.5cm, ffC=lLlcm, angle ABC = 70°and angle CAD = 37°. (a) Calculate the length of AC. (b) Explain why angle ADC = Il0°. (c) Calculate the length of AD.(d) A point E lies on the circle such that triangle ACE is isosceles, with EA = EC.(i) Write down the size of angle A£C. <(ii) Calculate the area of triangle ACE.NOT TO SCALE44°Diagram NOTaccurately drawnL08°Q, R, sand y are points on the circumference of a circle. PU is a tangent io the circle at J.PQR is a straight line.Angle PQT=L08°.Angle STR = 44。
ALEVEL IGCSE 数学试卷-1

Paper 11
Mark Scheme Notes Marks are of the following three types: M Method mark, awarded for a valid method applied to the problem. Method marks are not lost for numerical errors, algebraic slips or errors in units. However, it is not usually sufficient for a candidate just to indicate an intention of using some method or just to quote a formula; the formula or idea must be applied to the specific problem in hand, e.g. by substituting the relevant quantities into the formula. Correct application of a formula without the formula being quoted obviously earns the M mark and in some cases an M mark can be implied from a correct answer. Accuracy mark, awarded for a correct answer or intermediate step correctly obtained. Accuracy marks cannot be given unless the associated method mark is earned (or implied). Mark for a correct result or statement independent of method marks.
Igcse-数学-历年真题

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................(2)C is another town.The bearing of C from A is 050.(b) Find the bearing of A from C.....................................(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journe y by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rosetta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A B......................................(ii) How many members are in A B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P Q = {3, 4}P R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to 15.75 m.Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102+ 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
第55届(2014)国际数学奥林匹克试题 英文版

Tuesday,July8,2014 Problem1.Let a0<a1<a2<···be an in nite sequence of positive integers.Prove that there exists a unique integer n≥1such thata n<a0+a1+···+a nn≤a n+1.Problem2.Let n≥2be an integer.Consider an n×n chessboard consisting of n2unit squares.A con guration of n rooks on this board is peaceful if every row and every column contains exactly one rook.Find the greatest positive integer k such that,for each peaceful con guration of n rooks, there is a k×k square which does not contain a rook on any of its k2unit squares.Problem3.Convex quadrilateral ABCD has∠ABC=∠CDA=90◦.Point H is the foot of the perpendicular from A to BD.Points S and T lie on sides AB and AD,respectively,such that H lies inside triangle SCT and∠CHS−∠CSB=90◦,∠T HC−∠DT C=90◦.Prove that line BD is tangent to the circumcircle of triangle T SH.Wednesday,July 9,2014Problem 4.Points P and Q lie on side BC of acute-angled triangle ABC so that ∠P AB =∠BCA and ∠CAQ =∠ABC .Points M and N lie on lines AP and AQ ,respectively,such that P is the midpoint of AM ,and Q is the midpoint of AN .Prove that lines BM and CN intersect on the circumcircle of triangle ABC .Problem 5.For each positive integer n ,the Bank of Cape Town issues coins of denomination 1n .Given a nite collection of such coins (of not necessarily di erent denominations)with total value atmost 99+12,prove that it is possible to split this collection into 100or fewer groups,such that each group has total value at most 1.Problem 6.A set of lines in the plane is in general position if no two are parallel and no three pass through the same point.A set of lines in general position cuts the plane into regions,some of which have nite area;we call these its nite regions .Prove that for all su ciently large n ,in any set of n lines in general position it is possible to colour at least √n of the lines blue in such a way that none of its nite regions has a completely blue boundary.Note:Results with √n replaced by c √n will be awarded points depending on the value of theconstant c .。
2010IGCSE 试卷12

This document consists of 15 printed pages and 1 blank page.IB10 06_0620_12/RP© UCLES 2010[Turn over*8778752636*UNIVERSITY OF CAMBRIDGE INTERNATIONAL EXAMINATIONS International General Certificate of Secondary EducationCHEMISTRY 0620/12Paper 1 Multiple Choice May/June 201045 MinutesAdditional Materials: Multiple Choice Answer Sheet Soft clean eraserSoft pencil (type B or HB is recommended)READ THESE INSTRUCTIONS FIRSTWrite in soft pencil.Do not use staples, paper clips, highlighters, glue or correction fluid.Write your name, Centre number and candidate number on the Answer Sheet in the spaces provided unless this has been done for you.There are forty questions on this paper. Answer all questions. For each question there are four possibl e answers A , B , C and D .Choose the one you consider correct and record your choice in soft pencil on the separate Answer Sheet.Read the instructions on the Answer Sheet very carefully.Each correct answer will score one mark. A mark will not be deducted for a wrong answer. Any rough working should be done in this booklet. A copy of the Periodic Table is printed on page 16. You may use a calculator.© UCLES 2010 0620/12/M/J/101 The diagram shows a cup of tea.Which row describes the water particles in the air above the cup compared with the water particles in the cup?moving fastercloser togetherA B C D2 Which row shows the change that takes place when element X gains the new particle shown?particle gained changeA electron an isotope of element X is formedB electron the element one place to the right of X in the Periodic Table is formedC proton an isotope of element X is formedDprotonthe element one place to the right of X in the Periodic Table is formed3 The symbols of two atoms may be written as shown.X 5223Y5224Which statement about these atoms is correct?A They are different elements because they have different numbers of neutrons.B They are different elements because they have different numbers of protons.C They are isotopes of the same element because they have the same nucleon number.D They are isotopes of the same element because they have the same proton number.4The diagram shows an atom.electronnucleus containingnine particles What is the proton number and neutron number of the atom?proton number neutron numberA 4 5B 4 9C 5 4D 5 95 A frui t dri nk coloured orange contai ns a di ssolved mi xture of red and yellow colouri ng agents.One of these colouring agents is suspected of being illegal.Which method could be used to show the presence of this illegal colouring agent?A chromatographyB distillationC evaporationD filtration6 A student carries out an experiment to find how fast 3cm pieces of magnesium ribbon dissolve in10cm3 samples of sulfuric acid at different temperatures.Which piece of apparatus does the student not need?A balanceB measuring cylinderC stop-clockD thermometer© UCLES 2010 0620/12/M/J/10 [Turn over© UCLES 2010 0620/12/M/J/10The electrolytes are listed below.cell 1 aqueous sodium chloride cell 2concentrated hydrochloric acid cell 3molten lead(II ) bromideIn which cells is a gas formed at both electrodes? A 1 and 2B 1 and 3C2 onlyD 3 only8 The diagram shows apparatus for plating a spoon with silver.Which statement is not correct?A Silver would stick to the spoon because it is a very reactive metal.B The electrolyte would be a silver salt dissolved in water.C The metal electrode would be made from silver.D The spoon would be connected to the negative of the power supply.9 Aqueous copper(II ) sulfate solution is electrolysed using inert electrodes.Copper(II ) i ons (Cu 2+), hydrogen i ons (H +), hydroxi de i ons (OH –) and sulfate i ons (−24SO ) are present in the solution.To which electrodes are the ions attracted during this electrolysis?attracted to anodeattracted to cathodeA Cu 2+ and H + OH – and −24SOB Cu 2+ and −24SOH + and OH –C H + and OH – Cu 2+ and −24SOD OH – and −24SO Cu 2+ and H +© UCLES 2010 0620/12/M/J/10[Turn over10 In which compounds are pairs of electrons shared between atoms?1 sodium chloride2 me t hane3 lead bromideA 1 onlyB 2 onlyC 1 and 3D 1, 2 and 311 Element X has six electrons in its outer shell.= electronkeyeHow could the element react?A by gaining two electrons to form a positive ionB by losing six electrons to form a negative ionC by sharing two electrons with two electrons from another element to form two covalent bondsD by sharing two electrons with two electrons from another element to form four covalent bonds12 Hydrogen and chlorine react as shown.1 molecule of hydrogen + 1 molecule of chlorine →2 moleculesof hydrogen chlorideWhat is the equation for this reaction?A 2H + 2C l → 2HC lB 2H + 2C l → H 2C l 2 C H 2 + C l 2 → 2HC lD H 2 + C l 2 → H 2C l 213 Which name is given to mixtures of metals?A alloysB compoundsC oresD salts14Iron is extracted from iron oxide using carbon monoxide as shown in the equation.iron oxide + carbon monoxide → iron + carbon dioxide What does the equation show?A Carbon monoxide is oxidised to carbon dioxide.B Carbon monoxide is reduced to carbon dioxide.C Iron is oxidised to iron oxide.D Iron oxide is oxidised to iron.15 A student investigates the rate of reaction between marble chips and hydrochloric acid.The loss in mass of the reaction flask is measured.The graph shows the results of two experiments, P and Q.mass ofreaction flaskWhich change explains the difference between P and Q?A A catalyst is added in P.B A higher temperature is used in P.C Bigger marble chips are used in Q.D Hydrochloric acid is more concentrated in Q.© UCLES 2010 0620/12/M/J/10© UCLES 2010 0620/12/M/J/10[Turn over16clouds seawater vapourWha t is the energy cha nge a nd wha t na me is given to the type of cha nge when wa ter evaporates?energy change type of change A energy given out endothermic B energy given out exothermic C energy taken in endothermic Denergy taken inexothermic17 Which process is not exothermic?A burning a fossil fuelB obtaining lime from limestoneC radioactive decay of 235UD reacting hydrogen with oxygen18 When pink cobalt(II ) sulfate crystals are heated, they form steam and a blue solid.When water is added to the blue solid, it turns pink and becomes hot.Which terms describe the pink cobalt(II ) sulfate crystals and the reactions?pink cob lt sulf te re ctionsA aqueous irreversible B aqueous reversible C hydr ted irreversible D hydr ted reversible19 An element melts at 1455°C, has a density of 8.90g/cm3 and forms a green chloride.Where in the Periodic Table is this element found?A BCD20An excess of copper(II) oxide is a dded to dilute sulfuric a cid to ma ke crysta ls of hydra ted copper(II) sulfate.The processes listed may be used to obtain crystals of hydrated copper(II) sulfate.1 concentrate the resulting solution2 filter3 heat the crystals4 wash the crystalsWhich processes are needed and in which order?A1, 2, 3 and 4B1, 2, 4 and 3C2, 1, 2 and 3D2, 1, 2 and 421 Which is not a property of Group I metals?A They are soft and can be cut with a knife.B They corrode rapidly when exposed to oxygen in the air.C They produce an acidic solution when they react with water.D They react rapidly with water producing hydrogen gas.© UCLES 2010 0620/12/M/J/1022Aqueous sodium hydroxide is added to a solid, X, and the mixture is heated.A green precipitate is formed and an alkaline gas is given off.Which ions are present in X?NH and Fe2+A +4NH and Fe3+B +4C OH– and Fe2+D OH– and Fe3+23An aqueous solution of the organic compound methylamine has a pH greater than 7.Which statement about methylamine is correct?A It neutralises an aqueous solution of sodium hydroxide.B It reacts with copper(II) carbonate to give carbon dioxide.C It reacts with hydrochloric acid to form a salt.D It turns blue litmus red.24The positions in the Periodic Table of four elements are shown.Which element is most likely to form an acidic oxide?ABCD© UCLES 2010 0620/12/M/J/10 [Turn overgas XWhat is gas X?A carbon dioxideB chlorineC hydrogenD oxygen26 A student added dilute hydrochloric acid to four metals and recorded the results.Not all of the results are correct.resultsmetal gas given off1 copper yes2 iron yes3 magnesium no4 zinc yesWhich two results are correct?A 1 and 3B 1 and 4C 2 and 3D 2 and 4© UCLES 2010 0620/12/M/J/101127 An element does not conduct electricity and exists as diatomic molecules. In which area of the Periodic Table is the element to be found?BAC D28 Copper, iron and zinc are all used as pure metals.Which of these three metals are also used in alloys?c o pper ir o n zincAB C D29 Solutions of a halogen and a sodium halide are mixed.Which mixture darkens in colour because a reaction occurs?A bromine and sodium chlorideB bromine and sodium fluorideC chlorine and sodium fluorideD chlorine and sodium iodide30 Some properties of four elements are shown in the table.Which element is a metal?melting point / °Celectrical conductivitywhen liquid electrical conductivitywhen solidA –7 l o w l o wB 801 high l ow C 1535 high high D 3550 l o w l ow31The diagram shows three types of item.cutlery cooking pan instruments used in hospitalsWhich method of rust prevention can be used for all three types of item?A coating with plasticB covering with greaseC galvanisingD using stainless steel32Aluminium is an important metal with many uses.Some of its properties are listed.1It is a good conductor of heat.2It is a reactive metal.3It has a low density.4It has an oxide layer that prevents corrosion.Which set of properties help to explain the use of aluminium for cooking and storing food?A 1, 2 and 3B 1, 2 and 4C 1, 3 and 4D 2, 3 and 433To grow roses, a fertiliser containing nitrogen, phosphorus and potassium is needed.For the best flowers, the fertiliser should contain a high proportion of potassium.Which fertiliser is best for roses?proportion by massfertiliserN P KA 9 0 25B 13 13 20C 29 5 0D 29 15 534 Which statements about water are correct?1 Water is treated with chlorine to kill bacteria.2 Household water may contain salts in solution.3 Water is used in industry for cooling.4Water for household use is filtered to remove soluble impurities.A 1, 2 and 3B 1 and 4C 2, 3 and 4D 1, 2, 3 and 435 Which statement about methane is not correct?A It is a liquid produced by distilling petroleum.B It is produced as vegetation decomposes.C It is produced by animals such as cows.D It is used as a fuel.36 Which compound in polluted air can damage stonework and kill trees?A carbon dioxideB carbon monoxideC lead compo undsD s u lf ur dioxide37 Diesel, petrol and bitumen are allA fuels.B hydrocarbons.C lubricants.D waxes.38 A macromolecule is a very large molecule.Macromolecules can be made by joining smaller molecules together. This is called polymerisation.Which row in the table describes the formation of a polymer?monomer polymerA ethane poly(ethane)B ethene poly(ethene)C ethane poly(ethene) Dethene poly(ethane)39 Which structure shows a compound that belongs to a different homologous series to propane?A B C DCC HH H H HC H HHHC H HHC C C H HH HH HHC H H HCC C H H H H H HHHH40 Which structure is incorrect ?HH H HHHC C C A HH H HHHC C BHH O HOC CHD HH H HOC C HHBLANK PAGE16Per mission to r epr oduce items wher e thir d-par ty owned mater ial pr otected by copyr ight is included has been sought and clear ed wher e possible. Ever y reasonable effort has been made by the publisher (UCLES) to trace copyright holders, but if any items requiring clearance have unwittingly been included, the publisher will be pleased to make amends at the earliest possible opportunity.University of Cambridge International Examinations is part of the Cambridge Assessment Group. Cambridge Assessment is the brand name of University of Cambridge Local Examinations Syndicate (UCLES), which is itself a department of the University of Cambridge. G r o u p140C eC e r i u m 58141P rP r a s e o d y m i u m 59144N d N e o d y m i u m 60P mP r o m e t h i u m61150S mS a m a r i u m62152E uE u r o p i u m63157G dG a d o l i n i u m64159T bT e r b i u m65162D yD y s p r o s i u m66165H oH o l m i u m67167E rE r b i u m68169T mT h u l i u m69173Y bY t t e r b i u m70175L uL u t e t i u m71232T hT h o r i u m 90P aP r o t a c t i n i u m 91238UU r a n i u m92N pN e p t u n i u m93P uP l u t o n i u m94A mA m e r i c i u m95C mC u r i u m96B kB e r k e l i u m97C fC a l i f o r n i u m98E sE i n s t e i n i u m99F mF e r m i u m100M dM e n d e l e v i u m101N oN o b e l i u m102L rL a w r e n c i u m1031HH y d r o g e n17L iL i t h i u m 323N aS o d i u m 1124M gM a g n e s i u m1240C aC a l c i u m 2045S c S c a n d i u m 2148T i T i t a n i u m2251V V a n a d i u m 2352C r C h r o m i u m 2455M n M a n g a n e s e 2556F e I r o n 2659C o C o b a l t 2759N i N i c k e l 2864C u C o p p e r 2965Z n Z i n c3070G aG a l l i u m3127A l A l u m i n i u m1311B B o r o n 512C C a r b o n614NN i t r o g e n716OO x y g e n819FF l u o r i n e928S iS i l i c o n1431PP h o s p h o r u s1532SS u l f u r1635.5C lC h l o r i n e1740A rA r g o n1820N eN e o n104H eH e l i u m273G eG e r m a n i u m3275A sA r s e n i c3379S eS e l e n i u m3480B rB r o m i n e3584K rK r y p t o n3639KP o t a s s i u m 1988S rS t r o n t i u m 3889Y Y t t r i u m 3991Z r Z i r c o n i u m4093N b N i o b i u m 4196M o M o l y b d e n u m 42T c T e c h n e t i u m 43101R u R u t h e n i u m 44103R h R h o d i u m 45106P d P a l l a d i u m 46108A g S i l v e r47112C dC a d m i u m48115I nI n d i u m49119S nT i n50122S bA n t i m o n y51128T eT e l l u r i u m52127II o d i n e53131X eX e n o n54137B aB a r i u m 56139L a L a n t h a n u m 57*178H fH a f n i u m72181T a T a n t a l u m 73184W T u n g s t e n 74186R e R h e n i u m 75190O s O s m i u m 76192I rI r i d i u m 77195P t P l a t i n u m78197A uG o l d79201H gM e r c u r y80204T lT h a l l i u m81207P bL e a d82209B iB i s m u t h83P oP o l o n i u m84A tA s t a t i n e85R nR a d o n86F rF r a n c i u m 87227A cA c t i n i u m899B eB e r y l l i u m4II I I I II V V V I V I I 085R bR u b i d i u m 37133C sC a e s i u m 55226R a R a d i u m 88T h e v o l u m e o f o n e m o l e o f a n y g a s i s 24d m 3a t r o o m t e m p e r a t u r e a n d p r e s s u r e (r .t .p .).a Xb a = r e l a t i v e a t o m ic m a s sX = a t o m i c s y m b o lb = p r o t o n (a t o m ic ) n u m b e rK e y *58-71 L a n t h a n o i d s e r i e s 90-103 A c t i n o i d s e r i e s D A T A S H E E T T h e P e r i o d i c T a b l e o f t h e E l e m e n t s。
Igcse数学历年真题

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper. You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets. Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2). There are 22 questions in this question paper. The total mark for this paper is 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance withEdexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................︒(2)C is another town.The bearing of C from A is 050︒.(b) Find the bearing of A from C.....................................︒(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b) Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rose tta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A ⋂B......................................(ii) How many members are in A ⋃B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P ⋂Q = {3, 4}P ⋂R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519.The depth of water in a reservoir increases from 14 m to 15.75 m. Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x°is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, of some trees.(a) Calculate an estimate for the number of trees with heights in the interval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPC to be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102 + 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
0606_m19_ms_12(IGCSE 0606 2019年3月试题)

Mark schemes should be read in conjunction with the question paper and the Principal Examiner Report for Teachers.
Cambridge International will not enter into discussions about these mark schemes.
Cambridge Assessment International Education Cambridge International General Certificate of Secondary Education
ADDITIONAL MATHEMATICS Paper 12 MARK SCHEME Maximum Mark: 80
GENERIC MARKING PRINCIPLE 1:
Marks must be awarded in line with:
• the specific content of the mark scheme or the generic level descriptors for the question • the specific skills defined in the mark scheme or in the generic level descriptors for the question • the standard of response required by a candidate as exemplified by the standardisation scripts.
Igcse-数学-历年真题-2

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance with Edexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................︒(2)C is another town.The bearing of C from A is 050︒.(b) Find the bearing of A from C.....................................︒(2) (Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rose tta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A ⋂B......................................(ii) How many members are in A ⋃B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P ⋂Q = {3, 4}P ⋂R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to 15.75 m.Work out the percentage increase.................................. %(Total 3 marks) 10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram s hows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram = a and = c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that = a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102+ 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKS END。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: . (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance with Edexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................(2)C is another town.The bearing of C from A is 050.(b) Find the bearing of A from C.....................................(2)(Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red isThe probability that it will land on blue isImad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rosetta’s journ ey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A B......................................(ii) How many members are in A B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P Q = {3, 4}P R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to m.Work out the percentage increase.................................. %(Total 3 marks)10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram shows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) =.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram OA= a and OC= c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that OP= a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out × 102+ × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKSEND。
