逻辑连接词
考点03 逻辑联结词及数学归纳法(解析版)
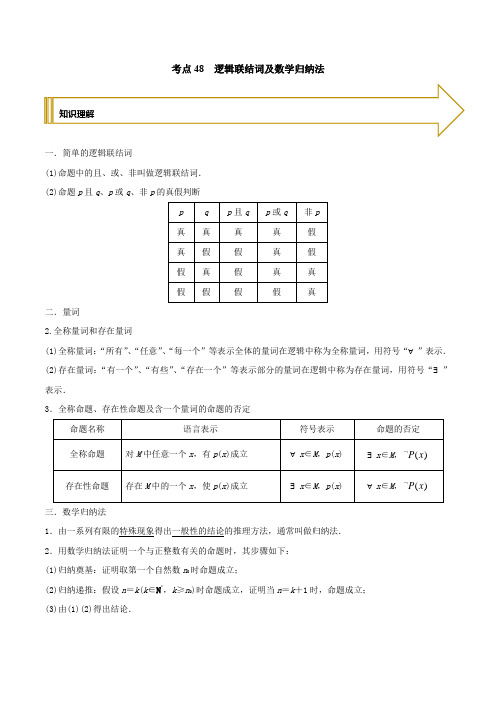
考点48 逻辑联结词及数学归纳法一.简单的逻辑联结词(1)命题中的且、或、非叫做逻辑联结词. (2)命题p 且q 、p 或q 、非p 的真假判断二.量词2.全称量词和存在量词(1)全称量词:“所有”、“任意”、“每一个”等表示全体的量词在逻辑中称为全称量词,用符号“∀”表示. (2)存在量词:“有一个”、“有些”、“存在一个”等表示部分的量词在逻辑中称为存在量词,用符号“∃”表示.3.全称命题、存在性命题及含一个量词的命题的否定三.数学归纳法1.由一系列有限的特殊现象得出一般性的结论的推理方法,通常叫做归纳法. 2.用数学归纳法证明一个与正整数有关的命题时,其步骤如下: (1)归纳奠基:证明取第一个自然数n 0时命题成立;(2)归纳递推:假设n =k (k ∈N *,k ≥n 0)时命题成立,证明当n =k +1时,命题成立; (3)由(1)(2)得出结论.知识理解考向一 命题的否定【例1】(2021·四川成都市·高三二模(理))命题“0x ∀>,210x x ++>”的否定为( )A .00x ∃≤,20010x x ++≤ B .0x ∀≤,210x x ++≤ C .00x ∃>,20010x x ++≤D .0x ∀>,210x x ++≤【答案】C【解析】因为全称命题的否定是特称命题,所以,命题“0x ∀>,210x x ++>”的否定是:00x ∃>,20010x x ++≤.故选:C .【举一反三】1.(2021·全国高三月考(理))命题“0x R ∃∈,002ln 0x x +≤”的否定是( ) A .x R ∀∈,2ln 0x x+≥ B .x R ∀∈,2ln 0x x+> C .0x R ∃∈,002ln 0x x +≥ D .0002,0x R lnx x ∃∈+> 【答案】B【解析】命题“0x R ∃∈,002ln 0x x +≤”为特称命题,该命题的否定为“x R ∀∈,2ln 0x x+>”. 故选:B.2.(2021·湖南岳阳市)命题“()1,x ∀∈+∞,21x e x ≥+”的否定是( ) A .()1,x ∃∈+∞,21x e x ≥+ B .()1,x ∀∈+∞,21x e x <+ C .()1,x ∃∈+∞,21x e x <+ D .()1,x ∀∈+∞,21x e x ≥+【答案】C【解析】命题“()1,x ∀∈+∞,21x e x ≥+”为全称命题,该命题的否定为“()1,x ∃∈+∞,21x e x <+”. 故选:C.考向分析3.(2021·泰州市第二中学)巳知命题p :0x ∃>,10x e x --≤,则命题p 的否定为( ) A .0x ∀≤,10x e x --> B .0x ∀>,10x e x --> C .0x ∃>,10x e x --≥ D .0x ∃≤,10x e x -->【答案】B【解析】命题p :0x ∃>,10x e x --≤,则命题p 的否定为0x ∀>,10x e x -->. 故选:B考向二 逻辑连接词求参数【例2】(2021·全国高三专题练习)若命题“200[1,2],2x x a ∃∈--+”是假命题,则实数a 的范围是( ) A .2a > B .2a C .2a >- D .2a -【答案】A【解析】若命题“200[1,2],2x x a ∃∈--+”是假命题,则命题“2[1,2],2x x a ∀∈--+<”是真命题, 当0x =时,()2max22x -+=,所以2a >.故选:A. 【举一反三】1.(2021·天水市第一中学高三月考(理))已知命题():1,3p x ∃∈-,220x a --≤.若p 为假命题,则a 的取值范围为( ) A .(),2-∞- B .(),1-∞-C .(),7-∞D .(),0-∞【答案】A 【解析】p 为假命题,∴():1,3p x ⌝∀∈-,220x a -->为真命题,故22a x <-恒成立,22y x =-在()1,3x ∈-的最小值为2-,∴2a <-. 故选:A.2.(2020·北京人大附中高三月考)若命题“x R ∃∈,使得2210ax x ++<成立”为假命题,则实数a 的取值范围是( ) A .[1,+∞) B .[0,+∞)C .(-∞,1)D .(-∞,0]【答案】A 【解析】命题“x R ∃∈,使得2210ax x ++<成立”为假命题, 则它的否定命题: “x R ∀∈,2210ax x ++≥”为真命题所以0440a a >⎧⎨∆=-≤⎩ 解得1a ≥,所以实数a 的取值范围是[1,)+∞ 故选:A.3.(2020·江西高三期中(文))存在[1,1]x ∈-,使得230x mx m +-≥,则m 的最大值为( ) A .1 B .14C .12D .-1【答案】C【解析】由不等式230x mx m +-≥,可化为23x m x≤-,设()[]2,1,13x f x x x=∈--,则()()()2226(6)33x x x x f x x x ---'==--,当[1,0)x ∈-时,()0f x '<,()f x 单调递减; 当(0,1]x ∈时,()0f x '>,()f x 单调递增,又由()11(1),142f f -==,所以函数()f x 的最大值为()112f =, 要使得存在[1,1]x ∈-,使得230x mx m +-≥,则12m ≤,则m 的最大值为12. 故选:C.考向三 数学归纳法【例3-1】(2020·全国高三专题练习(理))用数学归纳法证明不等式“1+12+13+…+121n -<n (n ∴N *,n ≥2)”时,由n =k (k ≥2)时不等式成立,推证n =k +1时,左边应增加的项数是( ) A .2k -1 B .2k -1 C .2k D .2k +1【答案】C【解析】n k =时,左边=1111 (2321)k ++++-,而n =k +1时,左边=11111111 (232122121)k k k k +++++++++-+-,增加了1111 (22121)k k k +++++-,共(2k +1-1)-(2k -1)=2k 项, 故选:C.【例3-2】.(2020·全国高三专题练习)设等比数列{}n a 满足113,34n n a a a n +==-. (1)计算23,a a ,猜想{}n a 的通项公式并加以证明; (2)求数列{}2nn a 的前n 项和n S .【答案】(1)25a =,37a =,21n a n =+,证明见解析;(2)1(21)22n n S n +=-+. 【解析】(1)由题意,等比数列{}n a 满足113,34n n a a a n +==-, 可得21345a a =-= ,323427a a =-⨯=,,猜想{}n a 的通项公式为21n a n =+,证明如下:(数学归纳法)当1,2,3n =时,显然成立; ∴ 假设n k =时,即21k a k =+成立;其中*(N )k ∈, 由134k k a a k +=-3(21)4k k =+-2(1)1k =++ ∴故假设成立,综上(1)(2),数列{}n a 的通项公式21n a n =+*()n N ∈.(2)令2(21)2n nn n b a n ==+,则前项和1212...3252...(21)2n n n S b b b n =+++=⨯+⨯+++ ∴由∴两边同乘以2得:23123252...(21)2(21)2n n n S n n +=⨯+⨯++-++ ∴由∴-∴的322112(12)3222...2(21)26(21)212n n n n n S n n -++--=⨯+⨯++-+=+-+-, 化简得1(21)22n n S n +=-+. 【举一反三】1.(2020·全国高三专题练习(理))用数学归纳法证明等式123(21)(1)(21)n n n +++++=++时,从n k=到1n k =+等式左边需增添的项是( ) A .22k + B .[]2(1)1k ++ C .[(22)(23)]k k +++ D .[][](1)12(1)1k k ++++ 【答案】C【解析】当n k =时,左边123(21)k =+++++,共21k +个连续自然数相加,当1n k =+时,左边123(21)(22)(23)k k k =+++++++++,所以从n k =到1n k =+,等式左边需增添的项是[(22)(23)]k k +++. 故选:C.2.(2021·全国高三专题练习)设集合T n ={1,2,3,…,n }(其中n ≥3,n ∴N *),将T n 的所有3元子集(含有3个元素的子集)中的最小元素的和记为S n . (1)求S 3,S 4,S 5的值; (2)试求S n 的表达式.【答案】(1)S 3=1,S 4=5,S 5=15;(2)41n C + .【解析】(1)当n =3时,T 3={1,2,3},3元子集有:{1,2,3},∴S 3=1;当n =4时,T 4={1,2,3,4},3元子集有:{1,2,3},{1,2,4},{1,3,4},{2,3,4},∴S 4=1×3+2=5;当n =5时,T 5={1,2,3,4,5},3元子集有:{1,2,3},{1,2,4},{1,2,5},{1,3,4},{1,3,5},{1,4,5},{2,3,4},{2,3,5},{2,4,5},{3,4,5},222543212315S C C C ∴=⨯+⨯+⨯=.(2)由S 3=1,S 4=5,S 5=15,S 6=35…归纳猜想出41n n S C +=(n ≥3).下面用数学归纳法证明猜想:∴当n =3时,S 3=1=44C ,结论成立;∴假设n =k (k ≥3,k ∴N *)时,结论成立,即S k =41k C +,则当n =k +1时,T k +1={1,2,3,4,…,k ,k +1},()()1111111232123...21k k k k k S S C C C k C k C +---⎡⎤=+++++-+-⎣⎦()()()(){}411111122112...21k k k C k C k C k k C k k C +--=+-+-++--+--⎡⎤⎡⎤⎣⎦⎣⎦ ()(){}4111111111211231...23...1k k k C k C C C C C C k C +--⎡⎤=++++-++++-⎣⎦ ()422311k k k k C kC kC C ++⎡⎤=+--⎣⎦ ()4341111k k k C C C ++++=+=∴当n =k +1时,结论成立. 综上:由∴∴可得()413n n S C n +=≥.1.(2021·涡阳县育萃高级中学)已知命题:p x R ∀∈,2104x x -+,则p ⌝( ) A .21,04x x x ∃∈-+R B .21,04x x x ∃∈-+>R C .21,04x x x ∀∈-+>R D .21,04x x x ∀∈-+<R 【答案】B【解析】命题p 为全称命题,根据全称命题的否定为特称命题,可得:p ⌝: 21,04x x x ∃∈-+>R 故选:B2.(2021·漠河市高级中学高三月考(文))下列说法正确的是( ) A .若p q ∨为真命题,则p q ∧为真命题B .命题“若cos cos x y ≠,则x y ≠”的否命题是“若cos cos x y =,则x y ≠”C .“0x <”是“20x x ->”的充要条件强化练习D .若p :x ∀∈R ,2320x x --<,则p ⌝:0x ∃∈R ,200320x x --.【答案】D【解析】对于A 选项,若p q ∨为真命题,可能p 真q 假,则p q ∧为假,故A 选项错误.对于B 选项,命题“若cos cos x y ≠,则x y ≠”的否命题是“若cos cos x y =,则x y =”,故B 选项错误. 对于C 选项,当2x =时,20x x ->,所以“0x <”不是“20x x ->”的充要条件,C 选项错误. 根据全称量词命题的否定的知识可知,D 选项正确. 故选:D3.(2021·全国高三专题练习)下列关于命题的说法中正确的是( )∴对于命题P :x R ∃∈,使得210x x ++<,则:P x R ⌝∀∈,均有210x x ++≥ ∴“1x =”是“2320x x -+=”的充分不必要条件∴命题“若2320x x -+=,则1x =”的逆否命题是“若1x ≠,则2320x x -+≠” ∴若p q ∧为假命题,则p 、q 均为假命题 A .∴∴∴ B .∴∴∴ C .∴∴∴∴ D .∴∴【答案】A【解析】∴对于命题:p x R ∃∈,使得210x x ++<,则:p x R ⌝∀∈均有210x x ++,故∴正确;∴由“1x =”可推得“2320x x -+=”,反之由“2320x x -+=”可能推出2x =,则“1x =”是“2320x x -+=”的充分不必要条件,故∴正确;∴命题“若2320x x -+=,则1x =”的逆否命题是“若1x ≠,则2320x x -+≠”,故∴正确; ∴若p q ∧为假命题,则p ,q 至少有一个为假命题,故∴错误. 则正确的命题的有∴∴∴. 故选:A4.(2021·河南高三其他模拟(文))命题:p “0,2sin 0x x x ∀≥-≥”的否定为( )A .0,2sin 0x x x ∀≥-<B .0,2sin 0x x x ∀<-<C .0000,2sin 0xx x ∃≥-< D .0000,2sin 0xx x ∃<-<【答案】C【解析】命题:p “0,2sin 0xx x ∀≥-≥”是全称命题,又全称命题的否定是特称命题,故“0x ∀≥,2sin 0x x -≥”的否定是“0000,2sin 0xx x ∃≥-<”.故选:C.5.(2021·山东菏泽市·高三一模)命题“2,0∈≥∀x R x ”的否定是( )A .2,0x R x ∃∈≥B .2,0x R x ∀∈<C .2,0x R x ∃∈<D .2,0x R x ∃∈≤【答案】C【解析】因为全称命题的否定是特称命题,所以命题:x R ∀∈,20x ≥的否定是:x R ∃∈,20x <.故选:C6.(2021·四川成都市·石室中学高三月考(理))设命题:0p x ∀≤x =-,则p ⌝为( ) A .0x ∀≤x ≠- B .00x ∃≤0x =- C .0x ∀>x =- D .00x ∃≤0x ≠-【答案】D【解析】命题p 为全称命题,该命题的否定为0:0p x ⌝∃≤0x ≠-. 故选:D.7.(2020·湖北武汉市·华中师大一附中高三期中)“0m >”是“x R ∃∈,2(1)2(1)30m x m x -+-+≤是假命题”的( ) A .充分不必要条件 B .必要不充分条件 C .充要条件 D .既不充分也不必要条件【答案】B【解析】由题意,命题“x R ∃∈,2(1)2(1)30m x m x -+-+≤是假命题” 可得命题“x R ∀∈,2(1)2(1)30m x m x -+-+>是真命题” 当10m -=时,即1m =时,不等式30>恒成立;当10m -≠时,即1m ≠时,则满足()()210214130m m m ->⎧⎪⎨⎡⎤---⨯<⎪⎣⎦⎩,解得14m <<,综上可得,实数14m ≤<,即命题“x R ∃∈,2(1)2(1)30m x m x -+-+≤是假命题”时,实数m 的取值范围是[1,4),又由“0m >”是“14m ≤<”的必要不充分条件,所以“0m >”是“x R ∃∈,2(1)2(1)30m x m x -+-+≤是假命题”的必要不充分条件, 故选:B.8.(2021·全国高三专题练习)若命题“∀[]1,4x ∈时,240x x m --≠”是假命题,则m 的取值范围( ) A .[4,3]-- B .()-∞,-4 C .[4,)-+∞ D .[4,0]-【答案】D【解析】若命题“[1x ∀∈,4]时,240x x m --≠”是假命题, 则命题“[1x ∃∈,4]时,240x x m --=”是真命题, 则24m x x =-,设22()4(2)4f x x x x =-=--, 当14x 时,4()0f x -,则40m -. 故选:D .9.(2020·江苏海门市·高三月考)命题“[]21220x x a ∀∈-≤,,”为真命题的一个充分不必要条件是( )A .2a ≤B .2a ≥C .4a ≤D .4a ≥【答案】D【解析】“[]21220x x a ∀∈-≤,,”为真命题,可得2a ≥,因为[)[)4,2,+∞⊂+∞ , 故选:D .10.(2021·全国高三专题练习)已知命题“02x ∃>,20040ax ax --<”是假命题,则a 的取值范围是( )A .[)2,+∞B .()2,+∞C .(],2-∞D .(),2-∞【答案】A【解析】因为命题“02x ∃>,20040ax ax --<”是假命题,所以240ax ax --≥对2x >恒成立, 所以()242a x x x≥>-恒成立.因为2x >, 所以22x x ->,则242x x<-, 故2a ≥. 故选:A11.(2020·全国高三专题练习)用数学归纳法证明“(1)(2)()213(21)nn n n n n ++⋅⋅⋅⋅⋅+=⋅⋅⋅⋅⋅⋅⋅-”,从“k到1k +”左端需增乘的代数式为( ) A .21k + B .2(21)k +C .211k k ++ D .231k k ++ 【答案】B【解析】当n k =时,等式的左边(1)(2)()k k k k =++⋅⋅⋅⋅⋅+,当1n k =+时,等式的左边(11)(12)()(1)(2)k k k k k k k k =++++⋅⋅⋅⋅⋅+++++, 所以当从“k 到1k +”左端增乘的代数式为(1)(2)2(21)1k k k k k k ++++=++.故选:B.12.(多选)(2021·恩施市第一中学)下列命题正确的有( ) A .命题“x R ∀∈,20x ≥”的否定是“x R ∃∈,20x <”. B .函数()cos f x x =向右平移2π个单位得到函数解析式为()sin g x x =. C .函数()21f x x =-的零点为()1,0-,()1,0.D .1弧度角表示:在任意圆中,等于半径长的弦所对的圆心角. 【答案】AB【解析】对A ,根据全称命题的否定性质,A 为正确的; 对B ,()cos f x x =向右平移2π个单位得到函数()cos()sin 2g x x x π=-=;对C ,函数零点是数而不是点,故C 错误;对D ,1弧度角表示为在任意圆中,等于半径长的弧所对的圆心角,故D 错误; 故选:AB.13.(多选)(2021·全国高三专题练习)下列命题中正确的是( ) A .(0,)x ∃∈+∞,23x x >B .(0,1)x ∃∈,23log log x x <C .(0,)x ∀∈+∞,121()log 2xx >D .1(0,)3x ∀∈,131()log 2xx < 【答案】BD【解析】对于选项A :当0x >时,22133xx x ⎛⎫=< ⎪⎝⎭,所以23x x <恒成立,故选项A 不正确;对于选项B :当(0,1)x ∈时,23log lg lg 3lg 31log lg 2lg lg 2x x x x =⨯=>,且3log 0x <,所以23log log x x <,故选项B 正确;对于选项C :当12x =时,1211()()222x ==,11221log log 12x ==,则121log ()2x x >,故选项C 不正确; 对于选项D :当13x =时,131log 13=,由对数函数和指数函数的性质可知,当1(0,)3x ∈时,131()1log 2x x <<,故选项D 正确; 故选:BD14.(多选)(2021·全国高三专题练习)若01,22x ⎡⎤∃∈⎢⎥⎣⎦,使得200210x x λ-+<成立是假命题,则实数λ可能取值是( ) A .32B.C .3 D .92【答案】AB【解析】由条件可知1,22x ⎡⎤∀∈⎢⎥⎣⎦,2210x x λ-+≥是真命题, 即22112x x x xλ+≤=+,即min 112,,22x x x λ⎛⎫⎡⎤≤+∈ ⎪⎢⎥⎝⎭⎣⎦,设()112,22f x x x x ⎡⎤=+≥=∈⎢⎥⎣⎦等号成立的条件是112,222x x x ⎡⎤=⇒=∈⎢⎥⎣⎦,所以()f x的最小值是即λ≤AB. 故选:AB15.(2021·江西高三其他模拟(文))已知命题“存在x ∈R ,使220ax x -+≤”是假命题,则实数a 的取值范围是___________. 【答案】18a >【解析】因为命题“存在x ∈R ,使220ax x -+≤”是假命题, 所以命题“R x ∀∈,使得220ax x -+>”是真命题,当0a =时,得2x <,故命题“R x ∀∈,使得220ax x -+>”是假命题,不合题意;当0a ≠时,得0180a a >⎧⎨∆=-<⎩,解得18a >.故答案为:18a >16.(2021·全国高三专题练习)若“存在x ∴[﹣1,1],3210x x a ⋅++>成立”为真命题,则a 的取值范围是___.【答案】9(,)2-+∞【解析】存在x ∴[﹣1,1],3210xxa ⋅++>成立,即213x xa +-<在[1,1]x ∈-上有解, 设2121()333x xx xf x +⎛⎫⎛⎫==+ ⎪ ⎪⎝⎭⎝⎭,[1,1]x ∈-, 易得y =f (x )在[﹣1,1]为减函数, 所以()[(1),(1)]f x f f ∈-,即213()3332f x +≤≤+,即91()2f x ≤≤, 即92a -<,所以92a >-, 故答案为:9(,)2-+∞.17.(2020·江西高三其他模拟(文))若命题:p x R ∃∈,210x mx -+<为假命题,则m 的取值范围是______. 【答案】[]22-,【解析】命题:p x R ∃∈,210x mx -+<为假命题,p ∴⌝:x R ∀∈,210x mx -+≥为真命题,则240m ∆=-≤,解得22m -≤≤,即m 的取值范围是[]22-,. 故答案为:[]22-,. 18.(2020·北京密云区·高三期中)若“01x ∃>,使得11x a x +<-.”为假命题,则实数a 的最大值为___________. 【答案】3【解析】由“∴x 0>1,使得11x a x +<-.”为假命题,可知,“11,1x x a x ∀>+≥-”为真命题, 11a x x ∴≤+-恒成立,由11111311x x x x +=-++≥=--,当且仅当2x =时取等号, 即a 的最大值为3. 故答案为:3.19.(2021·湖南永州市·高三二模)若对[]1,2x ∀∈,都有20ax x -≤,则实数a 的取值范围是___________. 【答案】1,2⎛⎤-∞ ⎥⎝⎦【解析】解:因为[]1,2x ∀∈,都有20ax x -≤,所以[]1,2x ∀∈,都有1a x≤,令()1g x x =,[]1,2x ∈,因为()1g x x=,在[]1,2x ∈上单调递减,所以()()min 122g x g ==,所以12a ≤,即实数a 的取值范围是1,2⎛⎤-∞ ⎥⎝⎦;故答案为:1,2⎛⎤-∞ ⎥⎝⎦20.(2020·全国高三月考(文))已知命题():0,p x ∀∈+∞,2230x mx -+>,命题:q m a <;若p 是q 的充分不必要条件,则实数a 的取值范围为______.【答案】()+∞【解析】设命题():0,p x ∀∈+∞,2230x mx -+>成立对应的m 的范围为集合A ,{}|B m m a =<若()0,x ∀∈+∞,223x mx +>,则32x m x +>,所以min 32m x x ⎛⎫<+ ⎪⎝⎭而32x x +≥32x x =,即x =时等号成立,所以min32x x ⎛⎫+= ⎪⎝⎭m <{|A m m =<,因为p 是q 的充分不必要条件,所以A B,所以a > 即实数a的取值范围为()+∞.故选答案为:()+∞21.(2020·凌海市第二高级中学高三月考)命题“2,1x R x t ∀∈>+”为真命题,则实数t 的取值范围是__________. 【答案】(),1-∞- 【解析】命题“2,1x R x t ∀∈>+”为真命题,且20x ≥,10t ∴+<,则1t <-,故实数t 的取值范围是(),1-∞-.故答案为:(),1-∞-.22.(2020·上海徐汇区·高三一模)用数学归纳法证明()2511222n n N -*++++∈能被31整除时,从k 到1k +添加的项数共有__________________项(填多少项即可). 【答案】5【解析】当n k =时,原式为:251122...2k -++++,当1n k =+时,原式为251551525354122...222222k k k k k k -+++++++++++++, 比较后可知多了55152535422222k k k k k ++++++++,共5项. 故答案为:523.(2020·浙江高三其他模拟)用数学归纳法证明:111111111234212122n n n n n-+-++-=+++-++,第一步应验证的等式是__________;从“n k =”到“1n k =+”左边需增加的等式是_________.【答案】11122-=()()1121121k k -+-+ 【解析】当1n =时,应当验证的第一个式子是11122-=,从“n k =”到“1n k =+”左边需增加的式子是()()1121121k k -+-+24.(2021·全国高三专题练习)设数列{}n a 满足11a =,12(23)n n a a n +=--. (1)计算2a ,3a .猜想{}n a 的通项公式并利用数学归纳法加以证明; (2)记2n nn b a =⋅,求数列{}n b 的前n 项和n S .【答案】(1)23a =,35a =,21n a n =-;证明见解析;(2)1(23)26n n S n +=-⨯+.【解析】(1)由题意可得2121213a a =+=+=,3221615a a =-=-=, 由数列{}n a 的前三项可猜想数列{}n a 是以1为首项,2为公差的等差数列, 即21n a n =-, 证明如下:当1n =时,12111a =⨯-=成立; 假设n k =时,21k a k =-成立.那么1n k =+时,12(23)2(21)(23)212(1)1k k a a k k k k k +=--=---=+=+-也成立. 则对任意的*n ∈N ,都有21n a n =-成立;(2)因为(21)2n n b n =-.∴23123252(21)2n n S n =⨯+⨯+⨯++-⨯,∴ 23412123252(21)2n n S n +=⨯+⨯+⨯++-⨯,∴∴-∴得:2341222222222(21)2n n n S n +-=+⨯+⨯+⨯++⨯--⨯()211122122(21)26(23)212n n n n n -++⨯-=+--⨯=---⨯-.∴1(23)26n n S n +=-⨯+.25.(2020·全国高三专题练习)已知数列{}n a 满足:11a =,点()()*1,n n a a n +∈N 在直线21y x =+上.(1)求2a ,3a ,4a 的值,并猜想数列{}n a 的通项公式; (2)用数学归纳法证明(1)中你的猜想.【答案】(1)2343,7,15a a a ===,21n n a =-;(2)证明见解析.【解析】(1)因为点()()*1,n n a a n N +∈在直线21y x =+上所以121n n a a +=+, 因为11a =,故22113a =⨯+=,32317a =⨯+=, 427115a =⨯+=,由上述结果,猜想:21nn a =-.(2)1︒,当1n =时,1211a =-=成立,2︒,假设当()1,n k k k N =≥∈时,21kk a =-成立,那么,当1n k =+时,()1121221121kk k k a a ++=+=-+=-成立,由1︒,2︒可得21nn a =-.26.(2020·黑龙江哈尔滨市·高三月考(理))已知数列{}n a 满足1a m =,2n a ≠,11210n n n a a a ++-⋅-=. (1)求2a ,3a ,4a ;(2)猜想{}n a 的通项公式,并用数学归纳法加以证明. 【答案】(1)212a m =-,3232m a m -=-,43243ma m-=-;(2)()()()121n n n m a n n m ---=--;证明见解析.【解析】1)因为11210n n n a a a ++-⋅-=,2n a ≠,所以112n na a +=-,又因为1a m = 211122a a m ==--,3212232m a a m -==--,43132243ma a m-==-- (2)()()()121n n n ma n n m---=--证明:1n =时,()1011ma m --==,结论成立 假设n k =时,结论成立,即()()()121k k k ma k k m---=--当1n k =+时:()()()()()()()()()11111122211221211k kk k m a k k m k k m k k m a k km k k m k k m+--====-------+--+------ 结论成立.综上,数列通项为()()()121n n n m a n n m---=-- 27(2020·云南师大附中高三月考(理))设数列{}n a 满足11a =,23a =,当()11112n n n n n a a a n a a -+-+=+++.(1)计算3a ,4a ,猜想{}n a 的通项公式,并加以证明. (2)求证:()()()2221244474111n a a a +++<+++. 【答案】(1)35a =,47a =,21n a n =-,证明见解析;(2)证明见解析. 【解析】(1)解:由11a =,23a =, 所以()123121225a a a a a +=++=+,()234231327a a a a a +=++=+. 猜想:21n a n =-,证明:当2n =时,由11a =,23a =,故成立;假设n k =(2k ≥)时成立,即21k a k =-, 所以()()1111221211k k k k k a a a k k k a a -+-+=++=+=+-+,即当1n k =+时成立,综上所述,21n a n =-. (2)证明:由(1)知,()22411n n a =+, 所以()()()22212444111n a a a ++++++22222211111111221311n n =+++<++++--- ()()1111132411n n =++++⨯⨯-+111111111111232435211n n n n ⎛⎫=+-+-+-++-+- ⎪--+⎝⎭11117112214n n ⎛⎫=++--< ⎪+⎝⎭,证毕.。
逻辑连接词

逻辑连接词逻辑连接词,也被称为连词、连接词或连接词汇,是用来连接两个句子、短语或单词的词语。
它们在句子中起到连接和衔接关系的作用,使得文章更加连贯和通顺。
在写作中,正确使用逻辑连接词非常重要,可以使句子之间的关系更加明确,使文章结构更加清晰。
下面我将介绍一些常用的逻辑连接词,并给出使用示例。
1. 并列连接词:并列连接词用来连接并列的句子、短语或单词,表示相同、相似或并列的关系。
例如:- 而且(用来连接两个或多个陈述意见或事实的句子):我喜欢旅行,而且我认为旅行可以增长见识。
- 或者(用来表示选择):你可以选择去看电影或者去逛商场。
- 并且(用来连接两个相似的陈述或动作):她努力工作,并且她总是取得好成绩。
2. 递进连接词:递进连接词用来表示递进或增加的关系,表明后面的内容与前面的内容相比更进一步或更加详细。
例如:- 而且(用来表示进一步补充):他不仅会弹钢琴,而且还会演奏吉他。
- 此外(用来表示另外增加的信息):我喜欢旅行。
此外,我也喜欢尝试不同的美食。
- 而且还(用来进一步增加信息):这座城市不仅风景优美,而且还有许多历史名胜古迹。
3. 转折连接词:转折连接词用来表示转折或对比的关系,表明后面的内容与前面的内容相比有所不同。
例如:- 但是(用来表示转折):我很喜欢运动,但是我不太擅长游泳。
- 然而(用来表示转折或对比):他刚开始很有信心,然而最后还是失败了。
- 尽管(用来表示让步):尽管下雨了,但是我们还是决定去露营。
4. 因果连接词:因果连接词用来表示因果关系,表明前面的内容是后面内容的原因或结果。
例如:- 因为(用来表示原因):我昨天没有上课,因为我生病了。
- 所以(用来表示结果):她努力学习,所以她考试取得了好成绩。
- 由于(用来表示原因):由于天气不好,比赛被取消了。
5. 条件连接词:条件连接词用来表示条件关系,表明后面的内容是前面内容的条件。
例如:- 如果(用来表示假设或条件):如果你明天有时间,我们可以一起去看电影。
英语逻辑连接词汇总

英语连接词连接词的意义分类表递进moreover(而且,此外), in addition, what is more,furthermore(此外,而且), also, then, besides, etc.表转折however, nevertheless(然而,不过;虽然如此), on the other hand, on the contrary, etc. 表层次on the one hand, ... on the other hand; first, ... second, ... finally;表强调firstly, ... secondly, ... finally ...; first, ... then ... etc.表强调in fact, indeed, actually, as a matter of fact, obviously, apparently,表结果evidently, first of all, undoubtedly, without any shadow of doubt, etc.表结尾therefore, as a result, then, consequently, accordingly, thus, etc.表例举in a word, in conclusion, therefore, in short, to sum up, etc.表强调still, Indeed, apparently, oddly enough(说来也奇怪), of course, after all, significantly, interestingly, also, above all, surely, certainly, undoubtedly, in any case, anyway, above all (首先,尤其是), in fact, especially. Obviously, clearly.表比较like, similarly, likewise(同样的,也), in the same way, in the same manner, equally. 表对比by contrast(相比之下), on the contrary, while, whereas(然而,鉴于,反之), on the other hand, unlike, instead, but, conversely(相反地), different from, however, nevertheless(然而,不过,虽然如此), otherwise, whereas, unlike, yet, in contrast.表列举for example, for instance, such as, take ...for example. Except (for), to illustrate. 表时间later, next, then, finally, at last, eventually, meanwhile, from now on, at the same time, for the time being, in the end, immediately, in the meantime, in the meanwhile, recently, soon, now and then, during, nowadays, since, lately, as soon as, afterwards, temporarily, earlier, now, after a while. first after a few days eventually at that time in the meantime meanwhile afterward from then on表顺序first, second, third, then, finally, to begin with, first of all, in the first place, last, next, above all, last but not the least, first and most important.表可能presumably, probably, perhaps.表解释in other words, in fact, as a matter of fact, that is, namely, in simpler terms.表递进What is more, in addition, and, besides, also, furthermore, too, moreover, furthermore, as well as, additionally, again.表让步although, after all, in spite of..., despite, even if, even though, though, admittedly, whatever may happen.表转折however, rather than, instead of, but, yet, on the other hand, unfortunately. whereas 表原因for this reason, due to, thanks to, because, because of, as, since, owing to.表结果as a result, thus, hence, so, therefore, accordingly, consequently, as consequence.表总结on the whole, in conclusion, in a word, to sum up, in brief, in summary, to conclude, to summarize, in short.其他类型连接词Mostly, occasionally, currently, naturally, mainly, exactly, evidently, frankly, commonly, for this purpose, to a large extent, for most of us, in many cases, in this case,表空间near to far from in the front of beside behind to the right to the left on the other side of表举例for example to name a few, say , such as表递进in addition furthermore what’s more what’s worse表对比whereas while as opposed to by contrast by comparison表示时间与频率的词汇:in general, every, some, after, on the whole, usually, most, at other times, in most cases, frequently, main, finally, as a rule, rarely, before, meanwhile.表示附加的词:additionally, as well as, just as, again, along with, also, further, furthermore, likewise, in the same manner, in the same way, in addition to,引出例子:for example, namely, for instance, as an example, that is表示转折:although, instead, rather than, but, nevertheless, though, however, on the other hand, otherwise得出结论:all in all, in consequence, in brief, as a result, the point is, in conclusion, therefore, hence, in sum.表示增加的过渡词:also,and,and then,too,in addition,furthermore,moreover,again,on top of that,another,first second third等。
英语逻辑连接词汇总完美

英语连接词连接词的意义分类表递进 moreover, in addition, what is more,furthermore, also, then, besides, etc.表转折 however, nevertheless, on the other hand, on the contrary, etc.表层次 on the one hand, ... on the other hand; first, ... second, ... finally;表强调 firstly, ... secondly, ... finally ...; first, ... then ... etc.表强调 in fact, indeed, actually, as a matter of fact, obviously, apparently,表结果 evidently, first of all, undoubtedly, without any shadow of doubt, etc.表结尾 therefore, as a result, then, consequently, accordingly, thus, etc.表例举 in a word, in conclusion, therefore, in short, to sum up, etc.表强调 still, Indeed, apparently, oddly enough, of course, after all, significantly, interestingly, also, above all, surely, certainly, undoubtedly, in any case, anyway, above all, in fact, especially. Obviously, clearly.表比较 like, similarly, likewise, in the same way, in the same manner, equally.表对比 by contrast, on the contrary, while, whereas, on the other hand, unlike, instead, but, conversely, different from, however, nevertheless, otherwise, whereas, unlike, yet, in contrast.表列举 for example, for instance, such as, take ...for example. Except (for), to illustrate.表时间 later, next, then, finally, at last, eventually, meanwhile, from now on, at the same time, for the time being, in the end, immediately, in the meantime, in the meanwhile, recently, soon, now and then, during, nowadays, since, lately, as soon as, afterwards, temporarily, earlier, now, after a while. first after a few days eventually at that time in the meantime meanwhile afterward from then on表顺序 first, second, third, then, finally, to begin with, first of all, in the first place, last, next, above all, last but not the least, first and most important.表可能 presumably, probably, perhaps.表解释 in other words, in fact, as a matter of fact, that is, namely, in simpler terms.表递进 What is more, in addition, and, besides, also, furthermore, too, moreover, furthermore, as well as, additionally, again.表让步 although, after all, in spite of..., despite, even if, even though, though, admittedly, whatever may happen.表转折 however, rather than, instead of, but, yet, on the other hand, unfortunately. whereas表原因 for this reason, due to, thanks to, because, because of, as, since, owing to.表结果 as a result, thus, hence, so, therefore, accordingly, consequently, as consequence. 表总结 on the whole, in conclusion, in a word, to sum up, in brief, in summary, to conclude, to summarize, in short.其他类型连接词Mostly, occasionally, currently, naturally, mainly, exactly, evidently, frankly, commonly, forthis purpose, to a large extent, for most of us, in many cases, in this case,表空间 near to far from in the front of beside behind to the right to the left on the other side of表举例 for example to name a few, say , such as表递进 in addition furthermore what ’s more what ’s worse 表对比 whereas while as opposed to by contrast by comparison表示时间与频率的词汇 :in general, every, some, after, on the whole, usually, most, at other times, in most cases, frequently, main, finally, as a rule, rarely, before, meanwhile.表示附加的词 :additionally, as well as, just as, again, along with, also, further, furthermore, likewise,in the same manner, in the same way, in addition to,引出例子 :for example, namely, for instance, as an example, that is表示转折 :although, instead, rather than, but, nevertheless, though, however, on the other hand,otherwise得出结论 :all in all, in consequence, in brief, as a result, the point is, in conclusion, therefore, hence,in sum.表示增加的过渡词: also ,and ,and then ,too,in addition ,furthermore ,moreover ,again ,on topof that , another ,first second third 等。
中文学术逻辑词

中文中的连词定义:连词是用来连接词与词、词组与词组或句子与句子、表示某种逻辑关系的虚词。
连词可以表并列、承接、转折、因果、选择等并列连词:有和、跟、与、同、及、而、况、况且、何况、乃至等。
承接连词:有则、乃、就、而、于是、至于、说到、此外、像、如、一般、比方等。
转折连词:有却、但是、然而、而、偏偏、只是、不过、至于、致、不料、岂知等。
因果连词:有原来、因为、由于、以便、因此、所以、是故、以致等。
选择连词:有或、抑、非…即、不是…就是等。
假设连词:有若、如果、若是、假如、假使、倘若、要是、譬如等。
比较连词:有像、好比、如同、似乎、等于;不如、不及;与其…不如、若…则、虽然…可是等。
让步连词:有虽然、固然、尽管、纵然、即使等。
成语中也有使用连词的情况,如:宁缺勿滥、三思而行、好整以暇逻辑关系词怎么用?1、集合的关系,以及常考的典型例题,以及在解题中的易错点2、简单逻辑关系词的编写以及由简单逻辑关键词链接的复合命题的真假判定连词与逻辑关系词区别?连词和逻辑就是关联性,延续性,合理性hence是逻辑关系词吗?hence是表示因果关系的逻辑关系词。
逻辑关系词什么意思?逻辑关系词的意思是指在论证过程中所使用的能关连上下事实论据的词或称逻辑关系词。
逻辑关系词包括连词吗?逻辑关系词包括连词,逻辑关系(logic relationship)即“依赖关系”,是指在人类活动中和思维活动中,概念之间的逻辑关系、命题之间的逻辑关系、事物之间的逻辑关系,时间之间和空间之间的逻辑关系。
指表示两个活动(前导活动和后续活动)中一个活动的变更将会影响到另一个活动的关系。
连词和逻辑关系词的区别?连此是相加在一起!逻辑词是因果关系!表示逻辑顺序的逻辑词中文?表示逻辑顺序的词:首先、其次、再次、最后英语的逻辑和中文的逻辑有什么不同?思维方式、主要在生活习惯以及处事方式上的不同!比如说你家有一副画,我夸奖你家的这幅画好看,我们中国人的方式一般是会假把意思的谦虚一哈,说“哎呀,哪点好看嘛,丑的很、丑的很”,当然,不排除个别哈而在英语里面的说法就是“嗯、我也觉得是这样”,你如果像中国这边这样说“丑的很”的话,夸奖的人就会有“你看不起我嗦,我说好看的东西,你给我冒句丑的很”这样的想法这个貌似是我们高中的时候英语老师给我们这个样子讲的,也有可能是初中的英语老师讲的哈(这个可能性大点),记不清了,希望对你有帮助,嘿嘿相容关系词?莫逆之交肝胆相照亲密无间亲朋好友深情厚意情投意合情同手足关系词语?混乱关系良好关系恶劣关系that关系词造句?例如,that在定语从句中做关系词,I like the magazine that he bought yesterday首先等关系词?“首先”作为关联词时,一般属于承接关系。
英语逻辑连接词汇总

英语衔接词衔接词的意义分类表递进moreover, in addition, what is more,furthermore, also, then, besides, etc.表转折however, nevertheless, on the other hand, on the contrary, etc.表层次on the one hand, ... on the other hand; first, ...second, ... finally;表强调firstly, ... secondly, ... finally ...; first, ... then ... etc.表强调in fact, indeed, actually, as a matter of fact, obviously, apparently,表成果evidently, first of all, undoubtedly, without any shadow of doubt, etc.表结尾therefore, as a result, then, consequently, accordingly, thus, etc.表例举in a word, in conclusion, therefore, in short, to sum up, etc.表强调still, Indeed, apparently, oddly enough, of course, after all, significantly, interestingly, also, above all, surely, certainly, undoubtedly, in any case, anyway, above all, in fact, especially. Obviously, clearly.表比较like, similarly, likewise, in the same way, in the same manner, equally.表比较by contrast, on the contrary, while, whereas, on the other hand, unlike, instead, but, conversely, different from, however, nevertheless, otherwise, whereas, unlike, yet, in contrast.表列举for example, for instance, such as, take ...for example. Except (for), to illustrate.表时光later, next, then, finally, at last, eventually, meanwhile, from now on, at the same time, for the time being, in the end, immediately, in the meantime, in the meanwhile, recently, soon, now and then, during, nowadays, since, lately, as soon as, afterwards, temporarily, earlier, now, after a while. first after a few days eventually at that time in the meantime meanwhile afterward from then on表次序first, second, third, then, finally, to begin with, first of all, in the first place, last, next, above all, last but not the least, first and most important.表可能presumably, probably, perhaps.表解释in other words, in fact, as a matter of fact, that is, namely, in simpler terms.表递进What is more, in addition, and, besides, also, furthermore, too, moreover, furthermore, as well as, additionally, again.表妥协although, after all, in spite of..., despite, even if, even though, though, admittedly, whatever may happen.表转折however, rather than, instead of, but, yet, on theother hand, unfortunately. whereas表原因for this reason, due to, thanks to, because, because of, as, since, owing to.表成果as a result, thus, hence, so, therefore, accordingly, consequently, as consequence.表总结on the whole, in conclusion, in a word, to sum up, in brief, in summary, to conclude, tosummarize, in short.其他类型衔接词Mostly, occasionally, currently, naturally, mainly, exactly, evidently, frankly, commonly, for this purpose, to a large extent, for most of us, in many cases, in this case,表空间near to far from in the front of beside behind to the right to the left on the other side of表举例 for example to name a few, say , such as表递进in addition furthermore what’s more what’s w orse表比较whereas while as opposed to by contrast by comparison暗示时光与频率的词汇:in general, every, some, after, on the whole, usually, most, at other times, in most cases, frequently, main, finally, as a rule, rarely, before, meanwhile.暗示附加的词:additionally, as well as, just as, again, along with, also, further, furthermore, likewise, in the same manner, in the same way, in addition to,引出例子:for example, namely, for instance, as an example, that is暗示转折:although, instead, rather than, but, nevertheless, though, however, on the other hand, otherwise得出结论:all in all, in consequence, in brief, as a result, the point is, in conclusion, therefore, hence, in sum.暗示增长的过渡词:also,and,and then,too,inaddition,furthermore,moreover,again,on top ofthat,another,first second third等.暗示时光次序的过渡词:now,then,before,after,afterwards,earlier,later,immediately,soon,ne xt,in afew days,gradually,suddenly,finally等.暗示空间次序的过渡词:near(to),far(from),infrontof,behind,beside,beyond,above,below,tothe right(left),around,outside等.暗示比较的过渡词:in thesameway,justlike,justas等.暗示对比的过渡词:but,still,yet,however,on theotherhand,onthe contrary,in spite of,even though等.暗示结果和原因的过渡词:because,since,so,as aresult,therefore,hence,thus,otherwise等.暗示目标的过渡词:forthisreason,forthispurpose,so that等.暗示强调的过渡词:infact,indeed,surely,necessarily,certainly,withoutanydoubt,truly,torepeat,aboveall,mostimportant等.暗示解释解释的过渡词:forexample,in fact,in thiscase,foractually 等.暗示总结的过渡词:finally,atlast,inconclusion,asIhaveshown,in otherword,in brief,in short,in general,on the whole,ashasbeen stated等补充(Addition)in addition, furthermore, again, also, besides, moreover, what’s more, similarly, next, finally.比较(Comparison) in the same way, likewise, similarly, equally, in comparison, just as对比(Contrast) whereas, in contrast, on the other hand, instead, however, nevertheless, unlike, even though, on the contrary, while 因果(Cause and effect) because, because of, for, since, due to, owing to, thanks to, as a result (of), accordingly, hence, so, thus强调(Emphasis) certainly, above all, indeed, of course, surely, actually, as a matter of fact, chiefly, especially, primarily, in particular, undoubtedly, absolutely, most important6.妥协(Concession) although, though, after all, in spite of, nevertheless, still, provided, while it is true....7.例证(Exemplification) for example, for instance, that is, namely, such as, in other words, in this case, by way of illustration.总结(Conclusion) to sum up, to conclude, in a word, in short, in brief, all in all, in all, to put it in a nutshell, in summary揣摸(Inference) therefore, as a result(of), consequently, accordingly, so, otherwise时光和空间(Time and space) afterward, after, first, later, then, soon, outside, near, beyond, above, below, on the right(left), in the middle, opposite, in front of暗示枚举增长(递进)first, second, third, firstly, secondly, thirdly, first, then / next, after that / next, finally / last / last but not least, and equally important, on (the) one hand…, on the other hand…, besides / what’smore / in addition (to sth.) / furthermore / moreover / another / also / too, plus, as well, especially / particularly / in particular暗示时光次序now, at present, recently, after, afterwards, after that, after a while, in a few days, at first, at the beginning (of sth.), to begin with, to start withlater, next, finally, immediately, soon, suddenly = all of a sudden, at that moment, as soon as, the moment…, form now on,from then on, graduallyat the same time = meanwhile, till, not…until, before, after, when, while, as, during暗示解释解释now, in addition, for example, for instance, in this case, in fact / as a matter of fact / actually, frankly speaking, in other words, in simpler terms, that is to say, to put it differently暗示转折关系but, however, while, though, yet, still, nevertheless, or, otherwise, on the contrary / on the other hand, in spite of… / despite the fact that…, even though, except (for), instead, in stead of, of course, after all, even so暗示并列关系or, and, also, too, not only … but also…, as well as, both… and…, either …or…, neither…nor…暗示因果关系原因:because, because of = thanks to… = due to… = owing to…, since = now that, as, for,成果:so, therefore, thus, hence, as a result (of), on this / that account, so…that, such…that, in this way暗示前提关系as (so) long as, on condition that, if, unless暗示妥协关系thou gh, although, as, even if, even though, whether …or…, however, whoever, whatever, whichever, wherever, whenever, no matter how (who, what, which, where)暗示举例for example, for instance, such as…, like…, take… for example暗示比较be similar to, similarly, the same as, in contrast, compared with (to)…, just like暗示目标for this reason, for this purpose, so that, in order that, inorder to, so as to,暗示强调indeed, in fact, surely, certainly, no doubt, without any doubt, truly, obviously, above all暗示归纳综合归纳in a word, in short, in brief, in summary, on the whole, generally speaking, in general, in my opinion, as far as I am concerned, as what has been mentioned, to sum up, to conclude, in conclusion暗示“尤有进者”的意思:Again, also, then, besides, further, furthermore, moreover, next, in addition等,如:① Jason teaches diligently. Besides, he writes a lot.② English is a useful language. For one thing, it is anofficial language in the UN. Then, it is widely used in business, science and technology.暗示“反看法”: But, however, still, yet, after all, for all that, in spite of, on the contrary, on the other hand等,如:③ Jim is intelligent but lazy.④ Singapore is not a big country.On the contrary, it is very small.暗示“困果关系”:Therefore, so, hence, thus, accordingly, consequently, as a result 等,如:⑤ Some people are over-ambitious. As a result, they are usually unhappy.⑥ Tom did not work hard; hence, he failed.暗示“比较关系”:Likewise, similarly, in a like manner 等,如:⑦ You cannot writes without a pen. Likewise, you cannot cook without rice.⑧ No one is allowed to speak Mandarin in an English class. Similarly, no one is allowed to speak English in a Mandarin class.暗示“举例示范”:For example, in other words, for instance, that is 等,如:⑨ There are some common errors in his com position. For instance,it is wrong to use "he" to WordStr "the queen".暗示“停止”:To sum up, in brief, in short, on the whole, to conclude 等,如:⑩ Some say Singapore is a nice place to live in. Others say it is not so nice. It is too competitive. In short, some like Singapore; some do not.并列关系and, furthermore, more than that, also, likewise, moreover, in addition, what is more, for instance, for example转折关系although, however, on the contrary, but, in spite of, nevertheless, yet, otherwise, despite次序关系first, second, third, and so on, then, after, before, next因果关系as a result, for, thus, because, for this reason, so, therefore, as, since, consequently, on account of归纳关系as a result, finally, therefore, accordingly, in short, thus, consequently, in conclusion, so, in brief, in a word几个用得比较多的句子:As far as I am concerned, the advantages of … outweigh its disadvantages.Nevertheless, the disadvantages of … is undeniable.To sum up/ In general/ On the whole/ In brief/ In short/ In a word, it is true that … bring about both positive and negative results. But we can try our best to reduce the negative influence to the least extent.Obviously, in every aspect, …This diagram unfolds a clear comparison between…and…As to the other three, though the growth rates were not so high, they were indeed remarkable and impressive.表层进\表举例\表解释\表总结\表强调\表妥协\表比较\表转折\表时光\表层进first, firstly to begin with second, secondly to start withthird, thirdly what’s morealso and thenand equally importantbesides in additionfurther in the first placestill furthermorelast last but not the leastnext besidestoo moreoverfinally2. 表举例for example for instanceto illustrate as an illustration after all3. 表解释as a matter of fact frankly speaking in this case namelyin other words4. 表总结in summary in a wordin brief in conclusionto conclude in factindeed in shortin other words of courseit is true speciallynamely in allthat is to summarizethus as has been saidaltogether in other wordsfinally in simpler termsin particular that ison the whole to put it differently therefore5. 表强调of course indeedabove all most important emphasis certainly in fact6. 表妥协still neverthelessin spite of all the sameeven so after allconcession granted naturallyof course7. 表比较in comparison likewisesimilarly equallyhowever likewisein the same way8. 表转折by contrast althoughat the same time butin contrast nevertheless notwithstanding on the contrary on the other hand otherwise regardless stillthough yetdespite the fact that even soeven though for all that however in spite ofinstead9. 表时光after a while afterwardagain alsoand then as long asat last at lengthat that time beforebesides earliereventually finallyformerly furtherfurthermore in additionin the first place in the past last latelymeanwhile moreovernext nowpresently secondshortly simultaneouslysince so farsoon stillsubsequently thenthereafter toountil until nowwhen总结关系过渡词语in general, to some extent, in my view, as for me, as far as I am concerned, obviously, in brief, on the whole比较比较关系过渡词语similarly, on the contrary, on the one hand, on the other hand, otherwise, in sharp contrast, but, however, yet, nevertheless列举关系过渡词语as a case in point, such as, first of all, to begin with, furthermore, besides, in addition, for one thing, for another因果关系过渡词语because (of), since, for, owing to, thanks to, as a result of, consequently, for the reason that, therefore, hence妥协关系过渡词语although, even though, in spite of, despite强调关系过渡词语surely, obviously, particularly, in deed, needless to say, most important of all递进关系in addition, also, moreover, besides,what's more时光次序immediately, meanwhile, presently, shortly, since, soon,temporarily, while方法手腕as, as if, the way,by目标关系so that, lest,in order that1)表层次:First,firstly, to begin with, further, in the first place second,secondly, to start with, still, furthermorethird,thirdly, what is more, last, last but not least also, and then, next, besidesand equally important too moreoverbesides in addition finally2)表转折;by contrast although though yetat the same time but despitethe fact that even soin contrast nevertheless even though for all that notwithstanding on the contrary however in spite ofon the other hand otherwise instead stillregardless3)表因果;therefore consequently because of for the reasonthus hence due to owing toso accordingly thanks to on this accountsince as on that account in this wayfor as a result as a consequence4)表妥协:still nevertheless concession granted naturallyin spite of all the same of course despiteeven so after all5)表递近:furthermore moreover likewise what is more besides also not only...but also...too in addition6)表举例:for example for instance for one thing that isto illustrate as an illustration a case in point7)表解释:as a matter of fact frankly speaking in this case namelyin other words8)表总结:in summary in a word thus as has been saidin brief in conclusion altogether in other wordsto conclude in fact finally in simpler termsindeed in short in particular that isin other words of course on the whole to put it differently namely in all therefore to summarize表并列填补关系: what is more, besides, also, as well, moreover, furthermore, in addition表转折比较关系: but, however, yet, instead, on the other hand, on the contrary, although, different from, in contrast to, despite, in spite of, whereas, un?鄄like, nevertheless, not only...but also, here...there, years ago...today, this...that, the former...the latter, then...now, the first...whereas the second, once...now, on the one hand...on the other , some...others表因果关系: since, as, because (of), for, so, thus, therefore, as a result, so that表前提关系: if, on the condition (that), as long as, unless, or else表时光关系: when, after, before, until, as soon as, later, afterwards, soon, lately, recently, since, from then on, eventually, in the meantime, then, suddenly, at the same time, next, early this morning/year/century, after an interval, now, after, presently, later, after?鄄ward, somewhat later, finally, at last, all of a sudden表特定的次序关系: above all, first of all, firstly, first, secondly, the , next, finally, in the end, at last表换一种方法表达: in other words, that is to say, to put it another way表举例解释: for instance, for example, like, such as表陈述事实: in fact, actually, as a matter of fact, to tell you the truth表总结: on the whole, in short, all in all, general, in a word, in conclusion, in closing, in summary。
数学逻辑连接词

数学逻辑连接词数学逻辑连接词: 因果关系、充分条件、必要条件、等价、充分充要、充分非必要、必要非充分、充分非必要非、充分充要非、等价非、充分非必要非充分、必要非充分、充分充要非必要、等价非充分、充分非必要非充分非、必要非充分非、充分充要非必要非、等价非充分非、充分非必要非充分非必要非、必要非充分非必要非、等价非充分非必要非充分非必要非因果关系是数学逻辑中常见的一种连接词。
它表示两个事件或者两个命题之间的因果关系。
例如,如果A发生,那么B也会发生。
在数学推理中,我们经常使用因果关系来推导结论。
充分条件是另一种常见的逻辑连接词。
它表示如果A成立,那么B 也一定成立。
充分条件是一个充分推理的条件,它能够帮助我们得出结论。
必要条件是与充分条件相对应的逻辑连接词。
它表示如果B成立,那么A一定成立。
必要条件是一个必要推理的条件,它能够帮助我们确定前提。
等价是逻辑中常见的一种关系。
它表示两个命题具有相同的真值。
如果两个命题互为真或者互为假,那么它们是等价的。
等价关系可以帮助我们简化复杂的逻辑推理。
充分充要是充分条件与必要条件的合并。
它表示如果A成立,那么B一定成立,并且如果B成立,那么A也一定成立。
充分充要是一个同时包含充分条件和必要条件的逻辑连接词。
充分非必要是充分条件的否定。
它表示如果A成立,那么B不一定成立。
充分非必要是一个只包含充分条件的逻辑连接词。
必要非充分是必要条件的否定。
它表示如果B成立,那么A不一定成立。
必要非充分是一个只包含必要条件的逻辑连接词。
充分非必要非是充分条件和必要条件的否定。
它表示如果A成立,那么B不一定成立,并且如果B成立,那么A也不一定成立。
充分非必要非是一个同时包含充分条件和必要条件的逻辑连接词。
充分充要非是充分条件、必要条件和否定的合并。
它表示如果A成立,那么B一定成立,并且如果B成立,那么A也一定成立。
充分充要非是一个同时包含充分条件、必要条件和否定的逻辑连接词。
等价非是等价关系的否定。
英语逻辑连接词

1) 先后次序关系:at this time(这时候、此时); first; second; at last; next; previously(以前);simultaneously[saiməl'teiniəsli](同时);eventually(最后);last but not least(最后但并不是最不重要的);to begin with(首先、本来); to start with =for a start(首先); first of all(首先,第一);to end with(最后); finally(最后); since then(从那时以来); afterwards(然后、后来);following this:following This Trend顺应这种趋势;By Following This Strategy通过采用这种策略;只要遵循这项策略following This Pattern下面这个模式;Following This Opinion由此来看following This Way沿着这条思路;Following This Thought循此思路following This Commitment根据这一承诺;Following This Example下面这位比如;preceding this(在这之前).2) 因果关系:because; because of this; being that(既然、因为、由于); another important factor/reason of... ; since (既然、因为、由于、自…以来); as(因为、依照、当…时、随着、虽然); for; in that... (既然、因为、由于); owing to(由于,因为…); due to; for the reason that...; in view of(鉴于、考虑到); the reason seems to be obvious; for this reason; as a result of this; therefore; ...and so(因此、所以)...; consequently(所以、因此、结果); as a result; thus; hence; so; so that...; in consequence(因此、结果); as a consequence(因此、结果); accordingly(因此、于是); inevitably(不可避免地、必然地); under these conditions(在这种情形下、在此情况时、在这些情况下); thereupon [,ðεərə'pɔn ]于是; seeing... (因为;鉴于;由于);3) 转折关系:but; even so(虽然如此,即使如此); however; though(可是、虽然、然而、不过); even though (虽然、即使); independent of(不管); despite that(尽管、不管); in spite of that(尽管、不管); regardless of(不管); yet...(然而、但是); and yet(可是、然而); but unless(除非).4) 并列关系:and; also; too; as well as(也;和…一样;不但…而且); either...,or...(不是…就是…;或者…或者…); both...and...5) (补充)递进关系:furthermore(此外、而且); moreover(此外、而且); further(更远地;进一步地;而且); In this way(这样); still(但是;仍然;尽管如此); not only...but also...; not that…but that…(不是(因为)…而是(因为)…); in addition (to)(另外,此外(除…之外)); additionally(此外), much more interesting, more specifically(更具体地说), next(其次;然后), besides(而且;此外;除…之外); as far as... (至于,就…而言);in other words(换句话说); on the one hand...,on the other hand...(一方面,另一方面); even(甚至;即使); as a popular saying goes...( 常言说); in order to do it...( 为了做这件事); meanwhile(同时); at the same time; accordingly(因此,于是); In the first place...(首先),in the second place...(其次、第二); equally important(同样重要); of even greater appeal(更吸引).6) 比较关系:similarly(同样地); in like manner(同样地); in comparison with(与…比较);when compared with(当和...相比时); compared with(与...相比较); when in fact...(而实际上;但事实上); like...(如同); likewise(也;同样地); apart from (doing)...( 除…之外;且不说;);... rather than...( 而不是;宁可…也不愿);by doing so(通过这样来做); both…and...;in the same way(同样地;以同样的方式); not only...but (also);7) 对照(不同点):yet; still(但是;仍然;尽管如此); notwithstanding(尽管,虽然); rather(宁可);neither ... nor(既不是…也不是…); although; though; but; however; conversely(相反地);unlike(和…不同,不像); opposed to(反对); as opposed to(与…截然相反) ; in contrast (to)(与此相反);on the contrary(正相反); different from this; nevertheless(然而,不过); contrary to(与…相反);whereas [hwεə'æz] (反之;然而); while(而); but on the other hand(但另一方面).8) 举例关系:for example; for instance; in this case(假若这样); namely(也就是;换句话说);as you know(正如你知道的); as you may say(如你所说); like(像;如同); such as(比如;诸如);a case in point is...( 一个典型的例子); in particular(尤其,特别); put it simply(简单地说);for one thing...首先,for another...其次、第二; as an illustration(作为一个例证), I will say...;a good example (of...)would be...; It is interesting to note that...; in this situation(在这种情况下);take…as example(以……为例); as for(关于,至于); as regards(关于,至于); as to(关于,至于);according to(根据); on this occasion(场合).9) 强调关系:in fact; especially; particularly; moreover; naturally(自然地);what is more important is that…(更重要的是); in reality(实际上;事实上); certainly(当然);of course; indeed(的确); in particular(尤其,特别); not to mention...( 更不必说);believe it or not(信不信由你); undeniably(不可否认地); other thing being equal(另一件是同等的);it is certain/sure that...; to be true(说实话); by definition(明显地); definitely(明确地,肯定地); undoubtedly(无庸置疑地); without a doubt(无疑地); in truth(事实上); in any event(不论怎样);without reservation(毫无保留地); obviously(明显地), not only.. but(also...), both... and...10) 条件关系:if; unless; lest(唯恐); provided that(倘若;假如); if it is the case(如果是这样的情况);in this sense(在这一点上,从这点来说); once...(一旦); if possible(如果可能的话);if necessary(如果必要的话); if so(如果是这样的话); if anything(如果有什么区别的话).11) 归纳总结类:in other words(也就是说); on the whole(就全体而论); in sum(总而言之); therefore; hence; in short(总之;简言之); in brief(总之); to sum up(总之;概括地说);in conclusion(总之,综上所述); in summary(总之;概括起来); to conclude(最后);the conclusion can be drawn that... (可以得出的结论); for this reason(为此)12) 地点关系:beyond(在...较远的一边); opposite to(在…对面); adjacent to(接近); at the same place; there; over(从头到尾、在…之上); in the middle; around(在附近、在…周围); in front of; in the distance(在远处); farther(此外;更远地;更进一步地); here and there(各处,到处); above(在……之上;在上面);below(在下面;在…下面);at the right side(在右边);between(在…之间;在中间);on this side(在这边).13) 目的关系:for this purpose; in order that; in this way;since; so that; on that account(由于那个缘故);in case万一、假使;with a view to(鉴于、由于考虑到);for the same reason。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
变式一
新知运用
用逻辑联结词“且”改写下列命题,并判断它们 的真假: (1)1既是奇数,又是素数; (2)2和3都是素数.
【设计意图】针对学生易混淆的概念,要重点强调, 简单归纳常见词语的否定.培养学生的归纳能力.
探究三 qpp q
真假表的判定
p
q p∧q
p∨q
真真 真假 假真 假假
探究新知ቤተ መጻሕፍቲ ባይዱ
p ¬p 真 假
口诀:“全真才真,一假则假”,“全假才假,一真则真”,“真假相反”.
[设计意图]为准确地运用新知,作必要的铺垫.
探究二
探究新知
以前我们有没有学习过像这样用联结词连接的命 题?试举一些例子?
【设计意图】 :通过具体数学实例引入逻辑联结词,易
引发学生的学习兴趣.引导学生思考、讨论,目的是引出 逻辑联结词“且、或、非”,让学生较轻松地感受到用逻 辑联结词联结两个命题可以得到一个新命题的认识.
探究新知
(1)一般地,用联结词“且”把命题 和命题 联结 起来,就得到一个新命题,记作p∧q 读作“p且q ” . (2)一般地,用联结词“或”把命题 和命题 联结 起来,就得到一个新命题,记作p∨q 读作“p或q ” .
教材分析
本节内容是数学选修1-1 第一章 常用逻辑用语 的第 三节,是在学习了前两节 命题及其关系、充分条件与必 要条件 的知识后,对常用逻辑用语的再学习,可以看作 是对前面学习过的两节内容的延续,要求特别注重学生 思维的严密性品质的培养.本课题的重点是通过数学实例, 了解逻辑联结词“且、或、非”的含义,使学生能正确 地表述相关数学内容,难点是正确理解命题 “ p∧q”“p q”“ p ”真假的规定和判定,简洁、准 确地表述命题“ p∧q ”“p q ”“ p ”.通过数学实例, 可以很好地培养学生分析问题、解决问题的能力,能给 学生提供更多的机会从实际问题中学习“且、或、非” 的用法,体会运用逻辑用语表述数学内容的准确性和简 洁性,避免学生对这三个常用逻辑联结词的含义和用法 的机械记忆和抽象解释.
教法分析
依据现有学生的年龄特点和心理特征,结合他 们的认识水平,在遵循启发式教学原则的基础上, 在本节采用诱思探究为主,以讲解法,练习法为辅 的教学方法,意在通过老师的引导,调动学生学习 知识的积极性,从而培养学生观察问题,发现问题 和解决问题的能力。为此,依据新课程的改革要求, 本节课采用师生互动的方式,既是以教师为引导, 学生为主体的讨论式学习,真正实现新课标下的 “以学生为主”的教学摸式 。
???符号“∧”和“∨”与以 前学的那些知识类似?
【设计意图】先讲解联结词“且” “或”,注意与 生活中的联结词相区分,有意识与所学过的知识对 比,便于掌握
探究新知
(3)一般地,对一个命题 全盘否定,就得到 一个新命题,记作¬p 读作“ 非p”或“ p的否定”
???命题的否定与原命题的否命
题有什么区别?
例1
新知运用
将下列命题用“且”联结成新命题,并判断
它们的真假:
(1) :平行四边形的对角线互相平分, :平行四 边形的对角线相等; (2) :菱形的对角线互相垂直, :菱形的对角线 互相平分; (3) :35是15的倍数, :35是7的倍数.
[设计意图] 通过本例题可以让学生认识到三个方面:一是 简洁表示命题;二是体会常用逻辑用语表述数学内容的准 确性;三是根据“且”的含义,确定 的真假.
学法分析
现代教学理论认为,教师的“教”不仅要让学生 “学会知识”,更重要的是让学生“会学知识”,而 正确的学法指导是培养学生这种能力的关键,因此在 本节的教学中,教师指导学生运用观察,分析讨论, 模拟归纳等手段来进行本节课的学习,实现对知识的 理解和应用。 在教学上采取了以下的措施: (1)从学生已有的知识出发,精心设置一组例子,逐 步引导学生观察,探讨,联想,归纳出逻辑联结词的 含义,从中体会逻辑的思想。 (2)通过简单命题与复合命题的对比,明确它们存在 的区别和联系,加深对复合命题构成的理解,抓住其 本质特点。
学情分析
文科生和音体美学生由于知识底子弱,判断真假使学 生的难点。本节概念的理解对学生而言简单,判断真 假是学生的弱点。
教学目标
知识与技能
(1)掌握逻辑联结词“或、且、非”的含义 (2)正确应用逻辑联结词“或、且、非”解决问题 (3)掌握真值表并会应用真值表解决问题
过程与方法
在观察和思考中,在解题和证明题中,本节课要特别注重学生思维 的严密性品质的培养
教学工具
诱思. 探究 多媒体教学
引入新课
当今社会中,人们从事任何工作、学习,都离不开逻 辑.具有一定的逻辑知识是构成一个公民的文化素质的重 要方面.数学的特点是逻辑性强,特别是进入高中以后, 所学的数学比初中更强调逻辑性.如果不学习一定的逻辑 知识,将会在我们学习的过程中不知不觉地犯逻辑性的错 误.其实,同学们在初中已经开始接触一些简易逻辑的知 识. 在数学中,有时会使用一些联结词,如“且、或、非”, 在生活用语中,我们也使用这些联结词,但表达的含义和 用法与数学中的含义和用法不尽相同.下面介绍数学中使 用联结词“且、或、非”联结命题时的含义和用法. 为叙述简便,今后常用小写字母 p,q,r, s 表示命题
情感态度与价值观
激发学生的学习热情,激发学生的求知欲,培养严谨的学习态度, 培养积极进取的精神,通过探索、发现知识过程,获得成功的体验,锻 炼学生克服困难的意志,建立学习数学的自信心。,
重点难点
重点
通过数学实例,了解逻辑联结词“或、且”的含 义,使学生能正确地表述相关数学内容
难点
(1)、正确理解命题“p∧q”“p∨q”“¬p”真假 的规定和判定. (2)、简洁、准确地表述命题 “p∧q”“p∨q”“¬p”.
探究一
探究新知
下列各组命题中,几个命题间有什么关系?
(1)①12能被3整除;②12能被4整除;③12能 被3整除且能被4整除. (2)①27是7的倍数 ;②27是9的倍数 ;③27是
7的倍数或是9的倍数 .
(3)①方程 x2 x 1 0 有实数根;
②方程 x2 x 1 0无实数根.
【设计意图】 :通过具体数学实例引入逻辑联结词,易引发学生的学习兴趣.
