中国女子数学奥林匹克竞赛真题及参考答案
2023中国女子数学奥林匹克竞赛试题解析

2023中国女子数学奥林匹克竞赛试题解析2023年的中国女子数学奥林匹克竞赛试题备受瞩目,让我们一起来仔细解析其中的题目,看看这些问题究竟有哪些特点和解法。
题目一:三角形的内切圆已知三角形ABC的三个顶点坐标分别为A(a, b),B(c, d),C(e, f),求证三角形的内切圆存在且唯一。
解析:为了证明三角形ABC的内切圆存在且唯一,我们需要从几何和代数两个角度来进行证明。
首先,在几何上,我们可以根据三角形ABC的三个顶点坐标绘制出三个边长,分别为AB、BC、AC。
然后,通过作垂线等方法找到三角形的内切圆的圆心O。
根据内切圆的定义,三角形的三条边分别与内切圆相切,即OA、OB、OC都是相切线段。
同时,由于切线与半径垂直,所以OA⊥AB,OB⊥BC,OC⊥AC。
因此,根据几何性质,三条垂线OA、OB、OC三点共线,即圆心O位于三角形ABC的内切圆心构成的直角三角形HOI的垂心上。
其次,在代数上,我们可以利用三角形的面积和三角形内切圆的半径之间的关系进行证明。
设三角形ABC的面积为S,半周长为p,三角形ABC的内切圆半径为r。
根据三角形面积公式可知,S = √[p(p-AB)(p-BC)(p-AC)]。
同时,根据三角形面积和半周长之间的关系可知,S = p * r。
将这两个等式相等,得到r = √[(p-AB)(p-BC)(p-AC)/p],即三角形的内切圆半径可以用三角形的边长表示。
综上所述,我们以几何和代数两个角度完成了对三角形内切圆存在且唯一的证明。
题目二:函数的极限已知函数f(x) = (3x + 2) / (2x - 1),求lim(x→∞)(f(x))。
解析:要求函数f(x)在极限x→∞时的值,我们可以通过分子和分母的次数来判断极限的值。
首先,观察函数f(x)的分子和分母,分别为3x + 2和2x - 1。
其中,分子的次数为x,分母的次数也为x,因此可以得出结论:当x→∞时,分子和分母的增长速度相当。
2013中国女子数学奥林匹克试题及其解答

2013中国女子数学奥林匹克试题及其解答1.证明(张云华)1.证明(mavropnevma )Let , . The region is the triangle ,and , thus the triangles of largest possible area are and . But(equality for is for ,disallowed).(equality foris for ).EDIT. Referring to the next post - it is interesting to figure out the envelope of the linesis the parabola , but in some way it is irrelevant, since the problemfunctions for a fixed , with fixed on the parabola for some , and thetriangular area being delimited by the tangent to that parabola at .1.证明(kunny), thus theparabola is envelope of the linesWhich touches at .The domain of the family of the lines swept by is shown by the shaded region, excluded twopoints .Edit:I was misreading the context problem, thank you for pointing put it, mavropnevma.I have just attached another figure.Needless to say, mavropnevma's solution is perfect.P.S.I remember that the similar problem original problem has been posed in 1970's in Tokyo University entrance exam/Science.According to my memory, let be the maximum area of any triangle which is involved in theregion in original problem, then draw the graph of to find the extrema of .Here is the similar problem posed in Tokyo University entrance exam/Science, second round, 1978In the -plane, let be the part which is correspond to of the parabola ,that is to say, .Let the tangent Line of at intersects with the line at and intersects withthe line at .Let . We are to consider the questions as below in the range of.(1) Let denote the area of triangles by , respectively. Find the rangeof such that .(2) Let be the domain enclosed by line segments and .Note : contains line segments and .Let be the maximum area of the triangle with a vertex which is contained in .Find the function and draw the graph, then find the exterme value.Note : A function has local minimum (or local maximum) at a point, which means for all points which is closed to ,holds. We call local maximum, local minimum as extreme value.2.证明(Luis González)Let and Let be the incircleof touching at Clearly and are homothethic with incirclesbut since are symmetric about the midpoint of (isthe M-excircle of MAB), it follows that and are homotheticwith corresponding cevians2.证明(Andrew64)As shown in the figure below.is the intersection of and .Therefore , , meet at the same point .Attachments:link17.jpg [ 37.23 KiB | Viewed 52 times ]3.证明(mavropnevma)Since it is irrelevant which persons of the same gender know each other, we may assume there ore none such, and consider the bipartite graph having the left shore made ofthe boys and the right shore made of the girls, with an edge connecting a boy and a girl ifthey know each other. The condition means does not contain any induced cycle of length ,and the requirement is to show the number of edges satisfies .Thus it is an extremal graph theory question, for bipartite graphs with forbidden 's; bysymmetry we should also have .Denote by the set of girls that each knows exactly one boy, and by the set of girls that eachknows more than one boy; take and . We obviously have and.Let us count the number of objects , where is a girl, are distinctboys, and knows both . For each of the doubletons there is at mostone girl knowing them both (by the condition), so . Moreover, by pigeonholewe have .On the other hand, we have, by Jensen's inequality.We thus need , so.Finally, we get .For equality to be reached we obviously need , namely for each pair of boys having exactly one girl knowing both of them; and then we need .3.证明(crazyfehmy)Another solution: Consider the bipartite graph where there are girls andboys and denote the girls by 's and boys by 's as vertices.Let denote the number of edges from the vertex to set . If is connected to some andthen for any the girl must not connected to both and . Now let us count suchpairs. For every girl there are many pair of edges. Since all such edge pairs must bedistinct for all girls, and since there are at most such pairs, we haveor equivalentlyNow assume that are greater than or equal to and areor . Then we haveand we need to show that .Since for we have and hence .Now by Cauchy-Schwarz inequality we haveand if , we haveand we need to show that . Assume . Then we havewhich means that which is a contradiction. So, we are done.4.解(crazyfehmy)Let be called a nice pair if satisfies the conditionsstated in the problem.Firstly, we shall prove a lemma:If is a nice pair then for all integers and .Proof: Let or and divides for some integers and. Then we can find another pair such that and. (The proof is easy) Then consider the systemand . By the Chinese Remainder Theorem this system has a solution and such that both and isan element of which means that is not a nice pair becauseis divisible by .Now, we shall show that if or then has asolution for all integers . For the proof assume there exists an integer such thathas no solution in integers. Then it is easy to see thathas also no solution for all integers which are not divisibleby . Now take and consider the numbers . For all of these numbers,we have no solution and there are such numbers. Since all square residues have solutions(take ) and there are square residues modulo including zero, this means that forall nonsquare residues , the equation must have no solution. However, for the numberis not a square residue but so for we have asolution which is a contradiction. For the number is not a square residue buthas a solution since is a square residue. Hence we again obtain a contradiction so the second lemma is also proved.Now, since can take every value modulo and we must have andand also we must have and if is a nice pair. So, can take only three values. We will consider each case separately:If then we must have which meansIf then we must have whichmeansIf then we must have whichmeansIf we count these possibilities, if i am not wrong, we get.4.解(dinoboy)First, remark that it suffices for to be injective modulo and .For modulo simply note that we require .For modulo or we require for some thatNow, what values can take modulo ? It is a simple exercise to show allvalues modulo can be obtained (just express it as and then aswe can transform the problem to what values modulo can beexpressed as , which is known to be all of them). Therefore the only way this problemsworks out is if and .Therefore we simply require that and . For each value of there are valuesof modulo , 10 modulo and modulo so the answer should simply be.Note: To show takes all values modulo without relying onis not hard, but I'm lazy and felt like reducing it to an already solved problem.4.解(yunxiu),so ,hence satisfies the problem.So the answer should be .6.解(crazyfehmy)If let then satisfiessince is equivalent to whichhas always a solution in the set for all and since is odd.Now we will show that if then the condition does not satisfy. Let be theelements of the set . Consider the sums and let. Since 's are different modulo , the numbers 'sare also different modulo . On the other hand, none of 's can be equivalent to modulobecause otherwise we would have two equivalent terms. Hence is apermutation of and by adding up these equations we getwhich means. Now do the same procedure for all's to get. Let and. Then we have . So, we havemany numbers equivalent to each other modulo . However, we know that there are manynumbers modulo which are all equivalent to each other modulo . Hence in order for 's tobe different modulo, we must have which means that and hence. Soand we are done.Hence all possible values are .7.证明(Luis González ) Let cut again at Since is the exsimilicenter ofthen is midpoint of the arc ofbisects externally is midpoint of the arc ofis external bisector of andNote that is a Thebault circle of the cevian of externally tangent to itscircumcircle By Sawayama's lemma passes through its C-excenter isC-excenter ofis M-isosceles, i.e. Hence iscircumcenter of7.证明(Andrew64)As shown in the figure. Let be the intersection of and .It's fairly obviousSo we have, andare concyclic.So , andThus, Consequently .Namely is the bisector of.Attachments:link18.jpg [ 31.39 KiB | Viewed 93 times ]8.证明(duanby)hint:(a-b)(c-b)(a-d)(c-d)in detail: product (a-b)(c-b)(a-d)(c-d) for everya,c be the number on , b,d be the number onfor point x,y if they are not ajjectent then in the product, it will occur twice, if it's ajjectent it's appears only once, and also chick the point that are on and then we get it.iampengcheng1130 2013中国女子数学奥林匹克第7题的解答。
2008年第7届中国女子数学奥林匹克(CGMO)真题及答案_wrapper

n 3n(3n 1) 2 | 3n 1 . 2
所以, n 为奇数. 又当 n 为奇数时,可将1, 2, 3,, 2n 每两个一组,分成 n 个组,每组两数之和
可以排成一个公差为 1 的等差数列:
1 (n n 1), 3 (n n 1),, n (n 1) ;
xk xk 1
1 x8
k 1
=
x2 x1
1 x18
=7 8
于是,
xk 1
7 8
xk
xk7 ,则当
x1
0 时, xk
0,
k
2.
由
xk 1
xk
xk (xk8
1) ,则当 8
xk8
1 8
0 ,即 xk
1
88 时,有
xk1
xk
0 ,即
xk1 xk , k 1.
而
xk 1
7 8
xk
xk7
88
1 87
A
E F
。
B
P O
。
D
C
【附注】 事实上,当四边形 ABCD 不是菱形时,A、P、Q 三点共线与 B、E、F、D 四 点共圆互为充要条件. 可利用同一法给予说明:取定 E 点,考虑让 F 点沿着直线 AD 运动. 根据相似变换可知,这时 Q 点的轨迹必是一条直线,它经过 P 点(由充分
性保证). 以下只要说明这条轨迹与直线 AP 不重合即可,即只要论证 A 点不在轨迹
A'
A
E F
P
B
D
Q
C
而当四边形 ABCD 是菱形时,不管 E、F 位置如何,所得到的 P、Q 两点总 位于对角线 AC 上.
2019年第18届中国女子数学奥林匹克
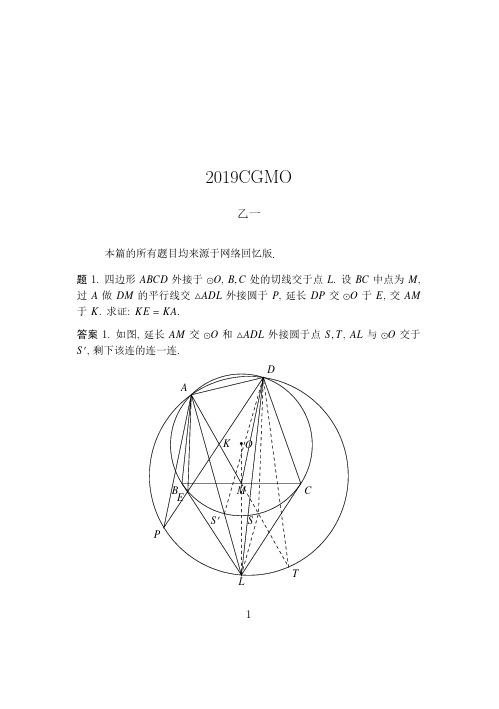
K •O
B
M
C
E
S′
S
P
T L
1
于是 KA = KE ⇔ ∠KAE = ∠KEA ⇔ AE ∥ DS . 由于 AP ∥ DM, 所以原命
题等价于 ∠PAE = ∠MDS . 由于 ∠APD = ∠AT D, 而 ∠AEP = 180◦ − ∠AED =
180◦ −∠AS D = ∠DS T , 所以 ∠PAE = ∠S DT, 故原命题等价于 DS 平分 ∠MDT .
答案 3. 可以这样操作的 n 为所有满足 n ≡ 0, 1 (mod 4) 的大于 3 的正整数 n.
3
先说明 n = 4k 的时候是可行的. 首先 n = 4 时, 我们有:
(1234) → (2314) → (2143) → (2431) → (4321)
当 n = 4k (k > 1) 时, 由于 4 之后有 4k − 4 个数, 所以可以用 2k − 2 步
本篇答案中第一段通过比角的方法丢掉了 P, E, 把问题变成了一个 △ABC 及其鸡爪点 S , 和一个动点 D 的问题. 但是这个时候 L, T 很不好 刻画, 因为 D 如果是动点的话, △ADL 的外接圆也是个动圆, 不好想象. 所以 这里的主要策略是将 A, D 固定住, 考虑 B, C 运动时候的的性质. 由于 B, C 可被 M 或 L 固定住, 所以就变成 ⊙O 上两点 A, D, 和点 M 或其反演点 L 的 性质的问题, 那这个时候就可以用反演的基本性质直接做.
a13 = a11 + a12 = 377 a14 = a12 + a13 = 610
a15 = a13 + a14 = 987 a16 = a7 + a15 = 1008
2007年第六届中国女子数学奥林匹克试题及解答

综上 ,最少取出 11 枚棋子 ,才可能满足要求 . 三、 定义集合 A = { m
k + 1| m ∈N+ , k ∈P} . k +1
.
i +1 数 ,则对任意的 k1 、 k2 ∈P 和正整数 m 1 、 m2 , m1 k1 + 1 = m2
由于对任意的 k 、 i ∈P ,且 k ≠i ,
是无理
由 m1 是正整数知 , 对 i = 1 ,2 ,3 ,4 ,5 , 满足这个 条件的 m1 的个数为 m
5
k +1 i +1 k +1 i +1
.
k 2 + 1 Ζ m 1 = m 2 , k1 = k 2 .
从而 , n =
注意到 A 是一个无穷集 . 现将 A 中的元素按从 小到大的顺序排成一个无穷数列 . 对于任意的正整 数 n ,设此数列中第 n 项为 m k + 1 . 接下来确定 n 与 m 、 k 间的关系 .
yx
2
当 x = 0 时 ,上式也成立 .
a1 a2 a2 a3 a n a1 故 2 + 2 + …+ 2 a2 + 1 a3 + 1 a1 + 1
2 2 2
4 . (1) 记 S 中的 n 个点为 A 1 , A 2 , …, A n .
建立直角坐标系 ,设 A i ( x i , y i ) ( i = 1 ,2 , …, n) . 易证
32
中 等 数 学
2007 女子数学奥林匹克
第一天
1. 设 m 为正整数 ,如果存在某个正整数
n ,使得 m 可以表示为 n 和 n 的正约数个数 (包括 1 和自身 ) 的商 , 则称 m 是 “好数” .求
2024年第22届中国女子奥林匹克竞赛数学试卷
2024年第22届中国女子奥林匹克竞赛数学试卷1、求所有的三元正整数组(aa,bb,cc),满足aa2aa=bb2bb+cc2cc.2、如图,用144根完全相同的长度为1的细棒摆成边长为8的正方形网格状图形.问:至少需要取走多少根细棒,才能使得剩余图形中不含矩形?请证明你的结论.3、设aa,bb,cc,dd都是不超过1的非负实数.证明:11+aa+bb+11+bb+cc+11+cc+dd+11+dd+aa⩽41+2√aabbccdd4.4、如图,四边形AAAAAAAA内接于圆Γ,对角线AAAA,AAAA互相垂直,交点为EE.设FF是边AAAA上一点,射线FFEE交Γ于点PP,线段PPEE上一点QQ满足PPQQ⋅PPFF=PPEE2,过点QQ且垂直于AAAA的直线交AAAA于点RR.证明:RRPP=RRQQ.5、如图,在锐角△AAAAAA 中,AAAA <AAAA ,AAAA 是高,GG 是重心,PP 、QQ 分别是内切圆与边AAAA 、AAAA 的切点,MM 、NN 分别是线段AAPP 、AAQQ 的中点.设AA 、EE 是△AAAAAA 内切圆上两点,满足:∠AAAAAA +∠AAAAAA =180°,∠AAEEAA +∠AAAAAA =180°.证明:直线MMAA ,NNEE ,GGAA 三线共点.6、设实数xx 1,xx 2,⋯,xx 22满足对任意1⩽ii ⩽22,有2ii−1⩽xx ii ⩽2ii .求(xx 1+xx 2+⋯+xx 22)�1xx 1+1xx 2+⋯+1xx 22� 7、给定奇素数pp 和正整数aa 、bb 、mm 、rr ,其中pp ∤aabb ,且aabb >mm 2.证明:至多只有一对正整数(xx ,yy )满足xx 与yy 互素,且aaxx 2+bbyy 2=mmpp rr .8、对于平面直角坐标系中任意两点AA (xx 1,yy 1)、AA (xx 2,yy 2),定义dd (AA ,AA )=|xx 1−xx 2|+|yy 1−yy 2|,设PP 1,PP 2,⋯,PP 2023是该坐标系中2023个两两不同的点.记λλ=mmaaxx 1⩽ii <jj⩽2023dd�PP ii ,PP jj �mmii mm 1⩽ii <jj⩽2023dd�PP ii ,PP jj �.(1) 证明:λλ⩾44.(2) 给出一组PP 1,PP 2,⋯,PP 2023,使得λλ=44.1 、【答案】(1,4,4),(2,4,4),(4,5,6),(4,6,5);【解析】设xx mm=mm2nn,则当nn⩾2时,xx mm−xx mm+1=mm−12nn+1>0,故12=xx1=xx2>xx3>xx4>⋯,不妨设bb⩽cc,由条件等式得aa<bb⩽cc,(1)若bb=cc,则aa2aa=bb2bb−1,故bb aa=2bb−aa−1∈ZZ,设bb=aaaa(aa>1),则aa=2aaaa−aa−1⩾aaaa−aa,即(aa−1)(aa−1)⩽1,由aa⩾2知aa=1或2,均有bb=2bb−2,得bb=4,(2)若bb<cc,则aa2aa⩽aa+12aa+1+aa+22aa+2=3aa+42aa+2①⇒aa⩽4,注意到,xx1=xx2=12,xx3=38,xx4=14,xx5=532,xx6=332<18,若aa=1或2,则xx bb+xx cc=12⇒xx bb>14⇒bb=3,此时cc无解.若aa=3,则xx bb+xx cc=38⇒xx bb>316⇒bb=4,此时cc无解;若aa=4,则式①等号成立,即bb=5,cc=6,经检验,满足要求,综上,所求(aa,bb,cc)为(1,4,4),(2,4,4),(4,5,6),(4,6,5).【标注】 ( 数论模块 )2 、【答案】43;【解析】首先证明至少需要移除43根细棒,假设图形中不含矩形,则每个有界连通区域至少由3个单位正方形组成,即面积至少为3,记[xx]表示不超过实数xx的最大整数,这样至多有�643�=21个有界连通区域,每取走一根细棒至多使得有界连通区域的个数减少1(将两个有界连通区域合并为一个有界连通区域,或者将一个有界连通区域与无界连通区域合并),最初时有64个有界连通区域,故至少取走64−21=43根细棒,下图给出了取走43根细棒的例子,其中每个有界连通区域的面积均是3,且图中不含矩形.【标注】 ( 数论模块 )3 、【答案】证明见解析;【解析】注意到,当√aacc⩽xx时,1xx+aa+1xx+cc−2xx+√aacc=(√aa−√cc)2(√aacc−xx)(xx+aa)(xx+cc)(xx+√aacc)⩽0,①由条件可知√aacc⩽1⩽1+bb,√aacc⩽1+dd,在式①中取xx=1+bb和xx=1+dd,分别得11+aa+bb+11+bb+cc⩽21+bb+√aacc,11+cc+dd+11+dd+aa⩽21+dd+√aacc,可见以√aacc代替aa和cc时,不等式左边不减,而右边不变,故不妨设aa=cc,类似地,不妨设bb=dd,这样,原不等式变为证明11+aa+bb⩽11+2√aabb,由均值不等式aa+bb⩾2√aabb可知上式成立.【标注】 ( 不等式 )4 、【答案】证明见解析;【解析】如图,作EEEE//AAFF,交AAPP于点EE,交AAPP于点YY,延长EEQQ、YYQQ,分别交AAAA于点SS、TT,联结AAPP,记⊙AAAAAA表示过AA,AA,AA三点的圆,由PPPP PPPP=PPPP PPPP=PPPP PPPP⇒EEQQ//AAEE,类似地,YYQQ//AAEE,由∠EEEESS=∠AAEEEE=∠AAAAAA=∠EEAASS⇒EE,EE,SS,AA四点共圆,由∠PPEEEE=∠PPAAAA=∠PPAAEE⇒EE,EE、PP,AA四点共圆,故EE,EE,SS,PP,AA五点共圆,类似地,YY,EE、TT,PP,AA五点共圆,由∠PPQQTT=∠PPEEAA=∠PPSSTT⇒PP,SS、QQ,TT四点共圆,由SSQQ//AAEE,TTQQ//AAEE,AAEE⊥AAEE⇒SSQQ⊥TTQQ,由∠RRQQSS=90°−∠QQEEYY=90°−∠QQTTSS=∠RRSSQQ,可知RR是⊙PPSSQQTT的圆心.从而,RRPP=RRQQ.【标注】 ( 平面几何 )5 、【答案】证明见解析;【解析】在△AAAAAA的外接圆上取点FF,使得AAAAAAFF是等腰梯形.直线FFAA与⊙AAAAAA的另一个交点为LL,与中线AAAA交于点GG′.如图,由AAFF=2AAAA⇒PPGG′GG′KK=PPPP HHKK=2⇒GG′是△AAAAAA的重心⇒点GG′与GG重合,故∠AALLAA=∠AALLFF=12AAFF⌢∘=12AAAA⌢∘=∠AAAAAA,结合条件∠AAAAAA+∠AAAAAA=180°得∠AAAAAA+∠AALLAA=180°⇒AA,LL,AA,AA四点共圆,类似可证∠AALLAA=∠AAAAAA,且AA,LL,AA、EE四点共圆,由于∠AALLAA=∠AAAAAA,PPAA与⊙AALLAAAA切于点AA,记△AAAAAA的内切圆为Γ,PPAA是Γ与⊙AALLAAAA的外公切线,由MMPP =MMAA 可知MM 是Γ与⊙AALLAAAA 的等幂点,从而,直线MMAA 是Γ与⊙AALLAAAA 的根轴,类似可证直线NNEE 是Γ与⊙AALLAAEE 的根轴,又直线GGAA 是⊙AALLAAAA 与⊙AALLAAEE 的根轴,故直线MMAA 、NNEE 、GGAA 要么三线共点,要么两两平行.若MMAA 、NNEE ,AAAA 两两平行,则⊙AALLAAAA 的圆心OO 1,⊙AALLAAEE 的圆心OO 2、Γ的圆心II 三点共线, 由于∠AAAAAA 与∠AAEEAA 都是钝角,于是,点OO 1,OO 2在AAAA 下方,显然点II 在AAAA 上方,设OO 1、OO 2、II 在AAAA 上的投影分别为EE 、YY 、ZZ ,则EE ,YY 分别是AAAA 、AAAA 的中点,由AAAA <AAAA 知点YY 、ZZ 在AAAA 同侧,且AAZZ =PPAA+BBAA−PPBB 2>BBAA 2>AAHH 2=AAYY , 故点ZZ 在线段EEYY 上.因此,OO 1、OO 2、II 不可能共线,矛盾, 从而,MMAA 、NNEE 、GGAA 三线共点.【标注】 ( 平面几何 )6 、【答案】 �212−1−1211�2 ;【解析】 设yy ii =xx ii 211(ii =1,2,⋯,22) , 注意到, ff (tt )=tt +1tt在区间(0,1]上递减,在区间[1,+∞)上递增,对1⩽ii ⩽11,有1212−ii ⩽yy ii ⩽1211−ii ⇒yy ii +1yy ii ⩽212−ii +1212−ii ; 对12⩽ii ⩽22,有 2ii−12⩽yy ii ⩽2ii−11⇒yy ii +1yy ii ⩽2ii−11+12ii −11, 则 �∑22ii=1xx ii ��∑22ii=11xx ii �=�∑22ii=1yy ii ��∑22ii=11yy ii� ⩽14���yy ii +1yy ii �mm ii=1�2⩽14���212−ii +1212−ii �11ii=1+��2ii−11+12ii −11�22ii=12�2=�21+22+⋯+211+121+122+⋯+1211�2 =�212−1−1211�2, 当xx ii =�2ii−1,1⩽ii ⩽112ii ,12⩽ii ⩽22 时,上式等号成立, 故所求最大值是 是�212−1−1211�2. 【标注】 ( 不等式 )7 、【答案】 证明见解析;【解析】 反证法.假设有两对不同的正整数解 (xx 1,yy 1)、(xx 2,yy 2),由于xx 1与yy 1互素,于是,pp ∤xx 1yy 1, 类似地,pp ∤xx 2yy 2,由 aaxx 12≡−bbyy 12(mod pp rr )aaxx 22≡−bbyy 22(mod pp rr ),可知 aabbxx 12yy 22≡aabbxx 22yy 12(mod pp rr ) 又pp ∤aabb ,故pp rr |(xx 12yy 22−xx 22yy 12), 注意到,xx 1yy 2−xx 2yy 1与xx 1yy 2+xx 2yy 1不能都被pp 整除,否则,pp |2xx 1yy 2,这与pp 是奇素数且pp ∤xx 1yy 1xx 2yy 2矛盾, 故pp rr |(xx 1yy 2−xx 2yy 1)或pp rr |(xx 1yy 2+xx 2yy 1), 若xx 1yy 2−xx 2yy 1=0,则 xx 1xx 2=yy1yy 2, 结合aaxx 12+bbyy 12=aaxx 22+bbyy 22,可知xx 1=xx 2,yy 1=yy 2,这与(xx 1,yy 1)≠(xx 2,yy 2)矛盾, 因而,xx 1yy 2−xx 2yy 1≠0, 若pp rr |(xx 1yy 2+xx 2yy 1),则xx 1yy 2+xx 2yy 1⩾pp rr ,若pp rr |(xx 1yy 2−xx 2yy 1),则xx 1yy 2+xx 2yy 1⩾|xx 1yy 2−xx 2yy 1|⩾pp rr ,因此总有xx 1yy 2+xx 2yy 1⩾pp rr ,利用条件aabb>mm2和上式有mm2pp2rr=(aaxx12+bbyy12)(aaxx22+bbyy22)=(aaxx1xx2−bbyy1yy2)2+aabb(xx1yy2+xx2yy1)2⩾aabb(xx1yy2+xx2yy1)>mm2pp2rr,矛盾.故假设不成立,原命题成立.【标注】 ( 数论模块 )8 、【答案】 (1) 证明见解析;(2) 见解析;【解析】 (1) 对aa=1,2,⋯,2023,设PP aa(xx aa,yy aa),记uu aa=xx aa+yy aa,vv aa=xx aa−yy aa,记AA=mmaaxx1⩽ii⩽jj⩽2023dd�PP ii,PP jj�,则对于任意1⩽ii、jj⩽2023,有|uu ii−uu jj|=|�xx ii−xx jj�+�yy1−yy jj�|⩽|xx ii−xx jj|+|yy ii−yy jj|=dd�PP ii,PP jj�⩽AA,因此,uu1,uu2,⋯,uu2023中的最大数与最小数之差不超过AA,即全在某个区间[aa,aa+AA]中,类似地,vv1,vv2,⋯,vv mm全在某个区间[bb,bb+AA]中,对aa、ll=1,2,⋯,44,考虑区域AA aa,ll=��uu+vv2,uu−vv2�|aa+aa−144AA⩽uu⩽aa+aa44AA,bb+ll−144AA⩽vv⩽bb+ll44AA�,点PP ii,PP2,⋯,PP2023落在这442=1936个区域中,由抽屉原理知存在两点在同一区域,假设PP1、PP jj∈AA aa,ll,记UU=uu ii−uu jj,VV=vv ii−vv jj,则−DD44⩽UU、VV⩽DD44,dd�PP ii,PP jj�=|xx ii−xx jj|+|+|yy ii−yy jj|=�uu ii+vv ii−uu jj+vv jj�+�uu ii−vv ii−uu jj−vv jj�=�UU+VV 2�+�UU−VV 2� ∈�±UU+VV 2±UU−VV 2�={UU ,−UU ,VV ,−VV },由于每种情况都有 dd�PP ii ,PP jj �⩽mmaaxx {|UU |,|VV |}⩽DD 44, 故 mmii nn 1⩽ii<jj⩽2023dd�PP ii ,PP jj �⩽dd�PP ii ,PP jj �⩽DD 44⇒λλ⩾44. (2) 关于构造,取点集MM ={(xx ,yy )∈ZZ 2|xx ,yy 同奇偶,|xx +yy |⩽44,|xx −yy |⩽44} =��uu+vv 2,uu−vv 2�|uu =0,±2,±4,⋯,±44;vv =0,±2,±4,⋯,±44�, 集合MM 中共有452=2025个点,从中任选2023个点作为PP 1,PP 2,⋯,PP 2023,则 dd�PP ii ,PP jj �=|xx ii −xx jj |+|yy ii −yy jj |是偶数且大于0,即dd�PP ii ,PP jj �⩾2, 另一方面,dd�PP ii ,PP jj �=|xx ii −xx jj |+|yy ii −yy jj |⩽mmaaxx�|(xx ii +yy ii )−�xx jj +yy jj �|,|(ii yy ii )−�xx jj −yy jj �|�⩽88, 故此时λλ=mmaaxx 1⩽ii <jj⩽2023dd�PP ii ,PP jj �mmii mm 1⩽ii <jj⩽2023dd�PP ii ,PP jj �⩽882,由(1)知此时λλ=44, 图1是nn =25个点满足λλ=4的例子,图2是16个区域划分,可以用来证明nn =17个点时λλ⩾4.第11页, 共11页【标注】。
2004年第3届中国女子数学奥林匹克(CGMO)真题及参考答案_wrapper

解:答案:15 个 首先证明最多可放 15 个“十字形”。用反证法,假设可放 16 个“十字形”。对每个“十 字形”,我们称其中心的方格为“心”(记为*)。将 10×11 的棋盘去掉周围一圈方格, 得到一个 8×9 的方格表,显然,每个“十字形”的“心”都只能出现在这个 8×9 的方 格表中。
E0M 0 F0 N 0
FN EM
. (2)
由(1),(2)得 E0 M 0 F0 N 0 E0 F0 M 0 N 0 (定值).
7
解:答案:如果记
A(
p,
q,
n)
(n 1)(n
第 3 届女子数学奥林匹克试题
第 3 届女子数学奥林匹克于 2004 年 8 月 11 日至 15 日在江西省南昌市举行,共有 45 个代表队的 179 名选手参加了此次竞赛,他们分别来自北京,上海等数十个城市以及美国, 俄罗斯,菲律宾,香港,澳门等国家和地区。竞赛共安排两天考试,每天四个小时,各考四 道题。通过竞赛,有 18 名选手获得金牌,39 名选手获得银牌,58 名选手获得铜牌。
k : 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 ak : 8 7 6 5 4 3 2 1 16 15 14 13 12 11 10 9 19 18 17
。
2 解:答案:最小值为 12 12 2
x a 2b c
令
y
a
b
2c
,则有
x
y
b,
现在,我们将 8×9 的方格表分为三个 8×3 的方格表,自左至右依次称它们为表(a), 表(b)和表(c)。由于 8×9 的方格表中共有 16 个“心”,所有必有一个 8×3 的方格 表中有 6 个“心” 如果在表(b)中有 6 个“心”,由于 8×3 的方格表中只有两种相互对称的放置 6 个“心” 的办法,故可不妨设 6 个“心”分布如下方左图:
2008年第七届中国女子数学奥林匹克试题及解答

2008年第七届中国女子数学奥林匹克1.(a ) 问能否将集合{}1,2,,96 表示为它的32个三元子集的并集,且三元子集的元素之和都相等;(b ) 问能否将集合{}1,2,,99 表示为它的33个三元子集的并集,且三元子集的元素之和都相等.(刘诗雄供题)解:(a )不能.因为96(961)32|129648972⨯++++==⨯ .(b )能.每个三元集的元素和为129999(991)15033332+++⨯+==⨯ .将1,2,3,,66每两个一组,分成33个组,,每组两数之和可以排成一个公差为1的等差数列:150,349,,3334,+++ 266,465,,3251+++ .故如下33组数,每组三个数之和均相等:{}{}{}1,50,99,3,49,98,,33,34,83, {}{}{}2,66,82,4,65,81,,32,51,67. .注:此题的一般情况是设集合{}1,2,3,,3M n = 的三元子集族{},,i i i i A x y z =,1,2,i n = 满足12n A A A M ⋃⋃⋃= .记i i i s x y z =++,求所有的整数n ,使对任意,(1)i j i j n ≤≠≤,i j s s =.解:首先,|1233n n ++++ ,即3(31)2|312n n n n +⇒+.所以,n 为奇数.又当n 为奇数时,可将1,2,3,,2n 每两个一组,分成n 个组,每组两数之和可以排成一个公差为1的等差数列:111(),3(),,(1)22n n n n n n +-++++++ ;322,4(21),,(1)()2n n n n n +++--++.其通项公式为1121(1)1,2213[12(1)][2(1)].22k n n k n k k a n n n k n k k n ++⎧-+++-≤≤⎪⎪=⎨++⎪-+-++--≤≤⎪⎩ 易知93312k n a n k +++-=为一常数,故如下n 组数每组三个数之和均相等:1111,,3,3,,31,,,1,31222n n n n n n n n n n +-+⎧⎫⎧⎫⎧⎫++-++-⎨⎬⎨⎬⎨⎬⎩⎭⎩⎭⎩⎭;332,2,31,,1,,2122n n n n n n n ++⎧⎫⎧⎫+--++⎨⎬⎨⎬⎩⎭⎩⎭.当n 为奇数时,依次取上述数组为12,,,n A A A ,则其为满足题设的三元子集族.故n 为所有的奇数.2.已知实系数多项式32()x ax bx cx d ϕ=+++有三个正根,且(0)0ϕ<.求证:322970b a d abc +-≤. ①证明:设实系数多项式32()x ax bx cx d ϕ=+++的三个正根分别为1x ,2x ,3x ,由韦达定理有123b x x x a++=-,122331c x x x x x x a++=,123d x x x a=-.由(0)0ϕ<,可得0d <,故0a >. 不等式①两边同除以3a ,不等式①等价于3729b c b d a a a a ⎛⎫⎛⎫⎛⎫-≤-+- ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭, 31231223311231237()()2()9x x x x x x x x x x x x x x x ⇔++++≤+++, 2222223331213212331321232()x x x x x x x x x x x x x x x ⇔+++++≤++ ②因为1x ,2x ,3x 大于0,所以221212()()0.x x x x --≥也就是2233122112.x x x x x x +≤+同理22332233233223311331,.x x x x x x x x x x x x +≤++≤+三个不等式相加可得不等式②,当且仅当123x x x ==时不等式等号成立.3.求最小常数1a >,使得对正方形A B C D 内部任一点P ,都存在,,,P A B P B C P C D P D A ∆∆∆∆中的某两个三角形,使得它们的面积之比属于区间1[,]aa -.解:m i n 152a +=.首先证明m i n 152a +≤,记152ϕ+=.不妨设正方形边长为2.对正方形A B C D 内部一点P ,令1S ,2S ,3S ,4S 分别表示P A B ∆,P B C ∆,P C D ∆,P D A ∆的面积,不妨设1243S S S S ≥≥≥.令1224,S S S S λμ==,如果,λμϕ>,由13241S S S S +=+=,得221S S μ=-,得21S μμ=+.故2121111111S S λμλϕϕλμϕμϕ===>==++++,矛盾.故{}m in ,λμϕ≤,这表明m in a ϕ≤.反过来对于任意(1,)a ϕ∈,取定15(,)2t a +∈,使得2819tb t=>+.我们在正方形A B C D 内取点P ,使得12342,,,1b b S b S S S b tt====-,则我们有122315(,)2S S t a S S +==∈,3242,(1)4(1)S b b a S t b b =>>>--由此我们得到对任意{},1,2,3,4i j ∈,有1[,]i jS aa S-∉.这表明m in a ϕ=.4. 在凸四边形ABCD 的外部分别作正三角形ABQ ,正三角形BCR ,正三角形CDS ,正三角形DAP ,记四边形ABCD 的对角线之和为x ,四边形PQRS 的对边中点连线之和为y ,求y x的最大值.(熊斌供题)解:若四边形ABCD 是正方形时,可得y x132+=.下面证明:y x132+≤.设1111,,,P Q R S 分别是边DA ,AB ,BC ,CD 的中点,SP ,PQ ,QR ,RS 的中点分别为E ,F ,G ,H .则1111P Q R S 是平行四边形.连接11,P E S E ,设点M ,N 分别是DP ,DS 的中点,则11D S S N D N E M ===, 11D P P M M D E N ===,又113606060P D S P D S ∠=︒-︒-︒-∠240(180)60E N D E N D=︒-︒-∠=︒+∠11E N S E M P =∠=∠,所以 1111D P S M P E N E S ∆≅∆≅∆, 从而,△11E P S 是正三角形.同理可得,△11G Q R 也是正三角形.设U ,V 分别是11P S ,11Q R 的中点,于是有1111113322E G E U U V V G P S P Q Q R ≤++=++111113322P Q P S B D A C =+=+,同理可得 1322F H A C B D ≤+,把上面两式相加,得132y x +≤,即y x132+≤.5. 已知凸四边形ABCD 满足AB =BC ,AD =DC .E 是线段AB 上一点,F 是线段AD 上一点,满足B ,E ,F ,D 四点共圆.作△DPE 顺向相似于△ADC ;作△BQF 顺向相似于△ABC .求证:A ,P ,Q 三点共线.(叶中豪供题)(注:两个三角形顺向相似是指它们的对应顶点同按顺时针方向或同按逆时针方向排列.) 证明将B 、E 、F 、D 四点所共圆的圆心记作O .联结OB 、OF 、BD .QPCABDEF在△BDF 中,O 是外心,故∠BOF =2∠BDA ; 又△ABD ∽△CBD ,故∠CDA =2∠BDA . 于是∠BOF =∠CDA =∠EPD ,由此可知等腰△BOF ∽△EPD . ①另一方面,由B 、E 、F 、D 四点共圆知△ABF ∽△ADE . ② 综合①,②可知,四边形ABOF ∽四边形ADPE , 由此得∠BAO =∠DAP . ③同理,可得∠BAO =∠DAQ . ④ ③,④表明A 、P 、Q 三点共线. 【附注】事实上,当四边形ABCD 不是菱形时,A 、P 、Q 三点共线 与B 、E 、F 、D 四点共圆互为充要条件.可利用同一法给予说明:取定E 点,考虑让F 点沿着直线 AD 运动.根据相似变换可知,这时Q 点的轨迹必是一条直线,它经 过P 点(由充分性保证).以下只要说明这条轨迹与直线AP 不重合即可,即只要论 证A 点不在轨迹上.为此,作△BAA ′∽△BQF ∽△ABC .于是由∠BAA ′=∠ABC , 可得A ′A ∥BC .又因四边形ABCD 不是菱形,故AD 不平于BC .这就表明A ′、A 、D 三点不共线,也就保证了A 点不在轨迹上. 因此,只有当B 、E 、F 、D 四点共圆时,Q 点才落在直线AP 上. 而当四边形ABCD 是菱形时,不管E 、F 位置如何,所得到的 P 、Q 两点总位于对角线AC 上.6.设正数列12,,,,n x x x 满足721187)8x x x -=(及 88211171,2()k k k k k k k x x x x x k x x -+----=≥.求正实数a ,使得当1x a >时,有单调性12n x x x >>>> ; 当10x a <<时,不具有单调性. 解:由88211171()k k k k k k k x x x x x x x -+----=,有1881111k k kk k k x x x x x x +---=-即1288811111117==8k kkkk k x x x x x x x xx+---=-=-A'QPCABDEFP QCBDAFE。
