闭环系统的过冲和相位裕度关系的分
机械工程控制基础-----填空简答题知识点

1、反馈:输出信号被测量环节引回到输入端参与控制的作用。
2、开环控制系统与闭环控制系统的根本区别:有无反馈。
3、线性及非线性系统的定义及根本区别:当系统的数学模型能用线性微分方程描述时,该系统的称为线性系统。
非线性系统:一个系统,如果其输出不与其输入成正比,则它是非线性的。
根本区别:线性系统遵从叠加原理,而非线性系统不然。
4、传递函数的定义及特点:零初始条件下,系统输出量的拉斯变换与输入量的拉斯变换的比值。
用G〔s〕表示。
特点:1〕、传递函数是否有量纲取决于输入与输出的性质,同性质无量纲。
2〕、传递函数分母中S的阶数必n不小于分子中的S的阶数m,既n=>m ,因为系统具有惯性。
3〕、假设输入已给定,则系统的输出完全取决于其传递函数。
4〕、物理量性质不同的系统,环节和元件可以具有相同类型的传递函数。
5〕、传递函数的分母与分子分别反映系统本身与外界无关的固有特性和系统同外界的关系。
5、开环函数的定义:前向通道传递函数G〔s〕与反馈回路传递函数H(s)之积。
6、时间响应的定义和组成:系统在激励信号作用下,输出随时间的变化关系。
按振动来源分为:零状态响应和零输入响应。
按振动性质:自由响应和强迫响应。
7、瞬态性能指标以及反映系统什么特性:性能指标:上升时间tr、峰值时间tp、最大超调量Mp、调整时间ts、振荡次数N。
这些性能指标主要反映系统对输入的响应的快速性。
8、稳态误差的定义及计算公式:系统进入稳态后的误差。
稳态误差反映稳态响应偏离系统希望值的程度。
衡量控制精度的程度。
稳态误差不仅取决于系统自身结构参数,而且与输入信号有关。
系统误差:输入信号与反馈信号之差。
9、减少输入引起稳态误差的措施:增大干扰作用点之前的回路的放大倍数K1,以及增加这一段回路中积分环节的数目。
10、频率响应的概念:线性定常系统对谐波输入的稳态响应称为频率响应。
11、频率特性的组成:幅频特性和相频特性。
12、稳定性的概念:系统在扰动作用下,输出偏离原平衡状态,待扰动消除后,系统能回到原平衡状态〔无静差系统〕或到达新的平衡状态〔有静差系统〕。
第六章-5-相角裕度和幅值裕度以及闭环频率特性指标

1/h < 1,
h>1
LmG(jω) ωΦ 幅值裕度, Lm (+) ) h(
Lmh Lm G ( j x ) 0
1/h -1 ωx
-90°
Φ γ(+) ω ωΦ G(jω)
-135 135° -180° -225 225° -270°
ωx 相位裕度, γ(+)
ω→
G(jω)的极坐标图 G(jω)的对数幅频曲线和相频曲线 9
1 h 1.25 0.8
1
1 h
Im j
GH
1 Re
x
0
A
j
0
例6-20 的极坐标图
浙江大学控制科学与工程学系
15
Phase Margin and Gain Margin
相角裕度和幅值裕度的求解方法——Bode图法 Bode图法
(三)Bode图法 画出系统的Bode图,由开环对数幅频特性与零分贝线(即 轴)的交 点频率 ,求出对应的相频特性与- 求出对应的相频特性与 1800线的相移量,即为相角裕度 线的相移量 为相角裕度
G(jω)
ω
-225° -270°
G(jω)的极坐标图
G(jω)的对数幅频曲线和相频曲线 6 浙江大学控制科学与工程学系
Phase Margin and Gain Margin
相角裕度和幅值裕度以及与稳定性的关系
对于闭环稳定系统,如果系统的开环相频特性再滞后 相角裕度γ 度,则系统将处于临界稳定状态. 滞后该角度将使得极坐标图穿越–1 1点 对于最小相位系统来说,相角裕度为正,系统稳定,负的相角裕 度表示系统是不稳定的. 相角裕度与系统阻尼比 有关,一般来讲,相角裕度在 有关 一般来讲 相角裕度在45°到 到 60°之间的系统响应是能令人满意的。
东北大学19春学期《自动控制原理Ⅰ》在线作业123答案

19春学期《自动控制原理Ⅰ》在线作业1最大超调量越大,说明系统过渡过程越不平稳。
A.是B.否正确答案:A调节时间的长短反映了系统动态响应过程的波动程度,它反映了系统的平稳性。
A.是B.否正确答案:BA.是B.否正确答案:A对于最小相位系统,相位裕度等于零的时候表示闭环系统处于临界稳定状态。
()A.是B.否正确答案:A开环对数频率特性的中频段反映了系统的稳定性和暂态性能。
()A.是B.否正确答案:A典型的非线性环节有继电器特性环节、饱和特性环节、不灵敏区特性环节等。
A.是B.否正确答案:A振荡次数越少,说明系统的平稳性越好。
A.是B.否正确答案:A系统结构图等效变换的原则是,换位前后的()保持不变。
A.输入信号B.输出信号C.反馈信号D.偏差信号正确答案:B上升时间指系统的输出量第一次达到输出稳态值所对应的时刻。
A.是B.否正确答案:A开环对数频率特性的低频段反映了系统的控制精度。
()A.是B.否正确答案:A在典型二阶系统中,当()时,系统是不稳定的。
A.AB.BC.CD.D正确答案:DA.是B.否正确答案:B典型二阶系统,()时为二阶工程最佳参数。
A.AB.BC.CD.D正确答案:B时滞环节在一定条件下可以近似为()A.比例环节B.积分环节C.微分环节D.惯性环节正确答案:D最大超调量反映了系统的平稳性。
A.是B.否正确答案:A自动控制系统按其主要元件的特性方程式的输入输出特性,可以分为线性系统和非线性系统。
A.是B.否正确答案:A频率特性可分解为相频特性和幅频特性。
()A.是B.否正确答案:A对于最小相位系统,相位裕度大于零的时候表示闭环系统是不稳定的。
()A.是B.否正确答案:B在典型二阶系统中,()时,系统是不稳定的。
A.过阻尼状态B.欠阻尼状态C.临界阻尼状态D.无阻尼状态正确答案:D最大超调量反映了系统的A.稳定性B.快速性C.平稳性D.准确性正确答案:C对于一般的控制系统,输出量的暂态过程中,下列哪种情况是稳定的A.持续振荡过程B.衰减振荡过程C.发散振荡过程D.等幅振荡过程正确答案:B振荡次数越多,说明系统的平稳性越好。
闭环系统的过冲和相位裕度关系的分

Overshoot as a Function of Phase MarginJ. C. DalyElectrical and Computer EngineeringUniversity of Rhode Island4/19/03Figure 1Figure 2 Amplifier frequency response.PM wt /weqQ%OS55o0.700 0.925 13.3% 60o0.580 0.817 8.7% 65o0.470 0.717 4.7% 70o0.360 0.622 1.4% 75o0.270 0.527 0.008%When an amplifier with a gain A(s) is put in afeedback loop as shown in Figure 1, the closed loop gain, V o /V in = A CL(1) The system is unstable when the loop gain, ß A(s), equals -1. That is, ß A(s) has a magnitude of one and a phase of -180degrees. An unstable system oscillates. A system close to being unstable has a large ringing overshoot in response to a step input.The phase margin is a measure of how close the phase of the loop gain is to -180 degrees, when the magnitude of the loop gain is one. The phase margin is the additional phase required to bring the phase of the loop gain to -180 degrees. Phase Margin = Phase of loop gain - (-180). The loop gain has a dominant pole at. Higher order poles can berepresented by an equivalent pole at . The amplifier is approximated by a function with two poles as shown in Equation 2.(2)Since for frequencies of interest where the loop gain magnitude is close to unity,(3)And,(4)(5)Defining ,Table IPM is the phase margin. w t is the unity gain frequency (rad/sec).w eq is the frequency of the equivalent higher order pole (rad/sec).Q is the system Quality factor.OS is the Over Shoot.(6)For frequencies of interest (frequencies close to the unity gain frequency), the amplifier gain can be written,(7)Plugging Equation 7 into Equation 1 results in the following expression for the closed loop gain.(8)Equation 8 is the transfer function for a second order system. The general form for the response of a second order system, where system properties are described by its Q and resonant frequence w o , is shown in Equation 10.(9)By comparing Equation 8 to Equation 9 we can get an expression for the resonant frequency and Q of the amplifier closed loop gain. (Equate coefficients of like powers of s in the dominators.)(10)(11)The loop gain is the feedback factor ß multiplied by the amplifier gain A(s).(12)The phase margin is a function of the phase of the loop gain at the frequency where the magnitude of the loop gain is unity.(13)where is the loop gain unity gain frequency. It follows from Equations 12 and 13 that,(14)Also, solving for w ta and dividing by w eq ,(15)It follows from Equations 11 and 15 that,(16)The phase of the loop gain (Equation 13) is.Phase of loop gain(17)The phase margin is the additional phase required to bring the phase of the loop gain to -180 degrees.Phase Margin = Phase of loop gain - (-180).Phase Margin(18)A well known property of second order systems is that the percent overshoot is a function of the Q and is given by,(19)Both phase margin (Equation 18) and Q (Equation 16) are a function of wt/weq . This allows us to use Equation 19 to create tables and plots of percent overshoot as a function of phase margin. As shown in Figures 3 and 4, and in Table I.Figure 3 Overshoot as a functionof phase margin. Plot generatedusingMATLAB code.Figure 4 Q as a function of phase margin. Plot generated using MATLAB code.。
环路测试结果分析及注意事项
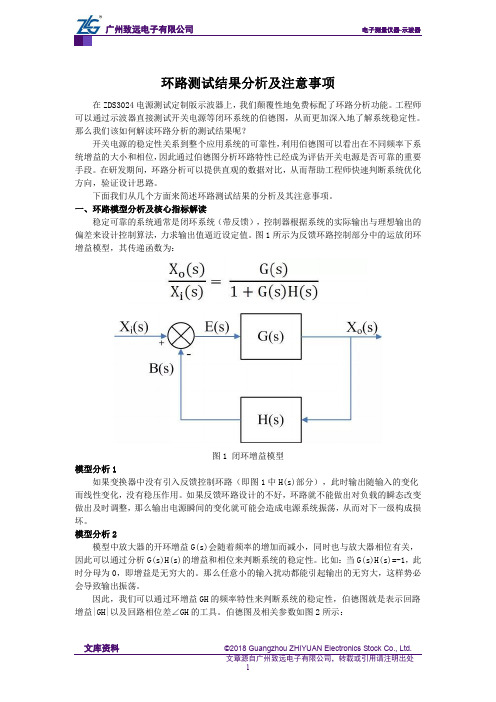
环路测试结果分析及注意事项在ZDS3024电源测试定制版示波器上,我们颠覆性地免费标配了环路分析功能。
工程师可以通过示波器直接测试开关电源等闭环系统的伯德图,从而更加深入地了解系统稳定性。
那么我们该如何解读环路分析的测试结果呢?开关电源的稳定性关系到整个应用系统的可靠性,利用伯德图可以看出在不同频率下系统增益的大小和相位,因此通过伯德图分析环路特性已经成为评估开关电源是否可靠的重要手段。
在研发期间,环路分析可以提供直观的数据对比,从而帮助工程师快速判断系统优化方向,验证设计思路。
下面我们从几个方面来简述环路测试结果的分析及其注意事项。
一、环路模型分析及核心指标解读稳定可靠的系统通常是闭环系统(带反馈),控制器根据系统的实际输出与理想输出的偏差来设计控制算法,力求输出值逼近设定值。
图1所示为反馈环路控制部分中的运放闭环增益模型,其传递函数为:图1 闭环增益模型模型分析1如果变换器中没有引入反馈控制环路(即图1中H(s)部分),此时输出随输入的变化而线性变化,没有稳压作用。
如果反馈环路设计的不好,环路就不能做出对负载的瞬态改变做出及时调整,那么输出电源瞬间的变化就可能会造成电源系统振荡,从而对下一级构成损坏。
模型分析2模型中放大器的开环增益G(s)会随着频率的增加而减小,同时也与放大器相位有关,因此可以通过分析G(s)H(s)的增益和相位来判断系统的稳定性。
比如:当G(s)H(s)=-1,此时分母为0,即增益是无穷大的。
那么任意小的输入扰动都能引起输出的无穷大,这样势必会导致输出振荡。
因此,我们可以通过环增益GH的频率特性来判断系统的稳定性,伯德图就是表示回路增益|GH|以及回路相位差∠GH的工具。
伯德图及相关参数如图2所示:图2 伯德图及相关参数伯德图有以下3个核心指标:●穿越频点:增益为0dB时对应的频率;●相位裕度:增益为0dB时对应的相位差;●增益裕度:相位为0°时对应的增益差。
由此可见,闭环系统的稳定性可以通过伯德图中的相位裕度,增益裕度,穿越频点来衡量。
环路测试结果分析及注意事项.pdf
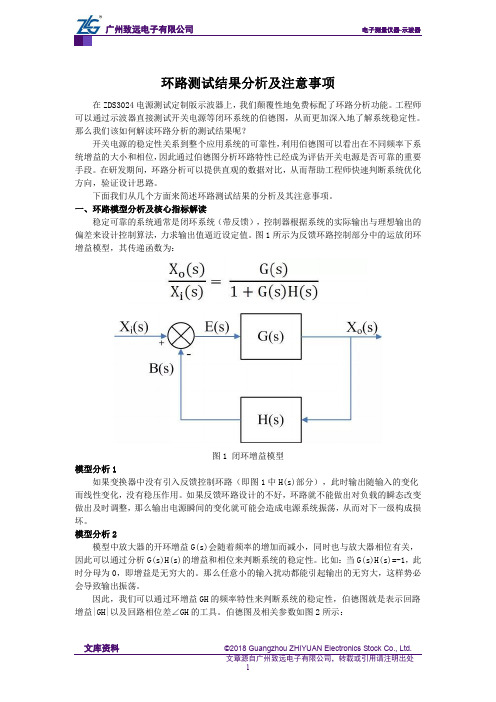
环路测试结果分析及注意事项在ZDS3024电源测试定制版示波器上,我们颠覆性地免费标配了环路分析功能。
工程师可以通过示波器直接测试开关电源等闭环系统的伯德图,从而更加深入地了解系统稳定性。
那么我们该如何解读环路分析的测试结果呢?开关电源的稳定性关系到整个应用系统的可靠性,利用伯德图可以看出在不同频率下系统增益的大小和相位,因此通过伯德图分析环路特性已经成为评估开关电源是否可靠的重要手段。
在研发期间,环路分析可以提供直观的数据对比,从而帮助工程师快速判断系统优化方向,验证设计思路。
下面我们从几个方面来简述环路测试结果的分析及其注意事项。
一、环路模型分析及核心指标解读稳定可靠的系统通常是闭环系统(带反馈),控制器根据系统的实际输出与理想输出的偏差来设计控制算法,力求输出值逼近设定值。
图1所示为反馈环路控制部分中的运放闭环增益模型,其传递函数为:图1 闭环增益模型模型分析1如果变换器中没有引入反馈控制环路(即图1中H(s)部分),此时输出随输入的变化而线性变化,没有稳压作用。
如果反馈环路设计的不好,环路就不能做出对负载的瞬态改变做出及时调整,那么输出电源瞬间的变化就可能会造成电源系统振荡,从而对下一级构成损坏。
模型分析2模型中放大器的开环增益G(s)会随着频率的增加而减小,同时也与放大器相位有关,因此可以通过分析G(s)H(s)的增益和相位来判断系统的稳定性。
比如:当G(s)H(s)=-1,此时分母为0,即增益是无穷大的。
那么任意小的输入扰动都能引起输出的无穷大,这样势必会导致输出振荡。
因此,我们可以通过环增益GH的频率特性来判断系统的稳定性,伯德图就是表示回路增益|GH|以及回路相位差∠GH的工具。
伯德图及相关参数如图2所示:图2 伯德图及相关参数伯德图有以下3个核心指标:●穿越频点:增益为0dB时对应的频率;●相位裕度:增益为0dB时对应的相位差;●增益裕度:相位为0°时对应的增益差。
由此可见,闭环系统的稳定性可以通过伯德图中的相位裕度,增益裕度,穿越频点来衡量。
机械工程控制基础第3阶段测试题

江南大学现代远程教育第三阶段测试卷考试科目:《机械工程控制基础》(第五章、第六章)(总分100分)时间:90分钟学习中心(教学点)批次:层次:专业:学号:身份证号:姓名:得分:一、单项选择题(本题共15小题,每小题2分,共30分。
)1、已知系统特征方程为 3s4+10s3+5s2+s+2=0,则该系统包含正实部特征根的个数为()。
A、0B、1C、2D、32、劳斯判据用()来判定系统的稳定性。
A、系统特征方程B、开环传递函数C、系统频率特性的Nyquist图D、系统开环频率特性的Nyquist图3、已知系统特征方程为 s4+s3+4s2+6s+9=0,则该系统()。
A、稳定B、不稳定C、临界稳定D、无法判断4、延时环节串联在闭环系统的前向通道时,系统稳定性()。
A、变好B、变坏C、不会改变D、时好时坏5、开环传递函数 G K(s)、闭环传递函数 G B(s)和辅助函数 F(s)=1+G K(s)三者之间的关系为()。
A、G K(s)绕(-1,j0)点的圈数就是G K(s)绕原点的圈数B、G K(s)绕原点的圈数就是G B(s)绕(-1,j0)点的圈数C、G K(s)绕(-1,j0)点的圈数就是F(s)=1+G K(s)绕原点的圈数D、G K(s)绕原点的圈数就是F(s)=1+G K(s)绕(-1,j0)点的圈数6、已知开环稳定的系统,其开环频率特性的Nyquist图如图所示,则该闭环系统()。
A、稳定B、不稳定C、临界稳定D、与系统初始状态有关7、设单位反馈系统的开环传递函数为 G K(s)= K/[s(s+1)(s+3)],则此系统稳定的K值范围为()。
A、K<0B、K>0C、2>K>0D、12>K>08、对于一阶系统,时间常数越大,则系统()。
A、系统瞬态过程越长B、系统瞬态过程越短C、稳态误差越小D、稳态误差越大9、系统稳定的充要条件()。
A、幅值裕度大于0分贝B、相位裕度大于0C、幅值裕度大于0分贝,且相位裕度大于0D、幅值裕度大于0分贝,或相位裕度大于010、以下方法可增加系统相对稳定性的是()。
自动控制原理习题
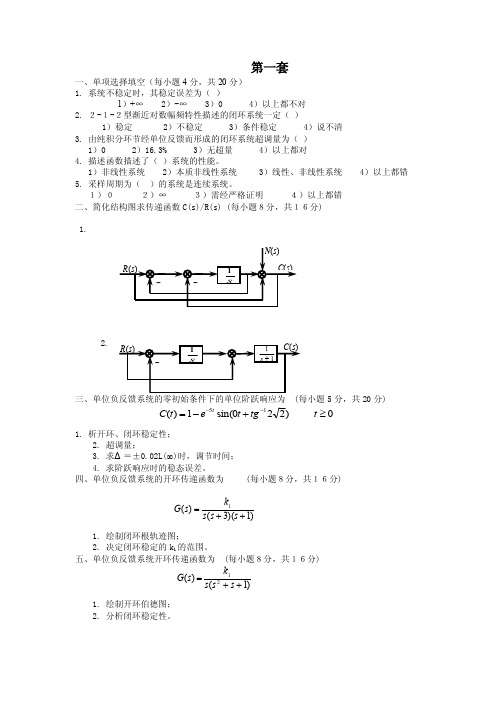
第一套一、单项选择填空(每小题4分,共20分) 1. 系统不稳定时,其稳定误差为( )1)+∞ 2)-∞ 3)0 4)以上都不对 2. 2-1-2型渐近对数幅频特性描述的闭环系统一定( )1)稳定 2)不稳定 3)条件稳定 4)说不清 3. 由纯积分环节经单位反馈而形成的闭环系统超调量为( ) 1)0 2)16.3% 3)无超量 4)以上都对 4. 描述函数描述了( )系统的性能。
1)非线性系统 2)本质非线性系统 3)线性、非线性系统 4)以上都错 5. 采样周期为( )的系统是连续系统。
1)0 2)∞ 3)需经严格证明 4)以上都错 二、简化结构图求传递函数C(s)/R(s) (每小题8分,共16分)1.2.三、单位负反馈系统的零初始条件下的单位阶跃响应为 (每小题5分,共20分)1. 析开环、闭环稳定性;2. 超调量;3. 求Δ=±0.02L(∞)时,调节时间;4. 求阶跃响应时的稳态误差。
四、单位负反馈系统的开环传递函数为 (每小题8分,共16分)1. 绘制闭环根轨迹图;2. 决定闭环稳定的k 1的范围。
五、单位负反馈系统开环传递函数为 (每小题8分,共16分)1. 绘制开环伯德图;2. 分析闭环稳定性。
0≥ +-=--t tg t e t C t )220sin(1)(15)1)(3()(1++=s s s k s G )1()(21++=s s s k s G第四套一、对自动控制系统基本的性能要求是什么?其中最基本的要求是什么?(5分)二、已知系统结构图如图1所示。
(20分) 1)求传递函数E(s)/R(s) 和 E(s)/N(s)。
2)若要消除干扰对误差的影响(即E(s)/N(s)=0),问G 0(s)=?图1三、已知系统结构图如图2所示,要求系统阻尼比0.6ζ=。
(20分) 1)确定K f 值并计算动态性能指标t p ,σ%,t s ; (提示:p dt πω=,%p e σ=4s nt ζω=)2)求在r (t )=t ,作用下系统的稳态误差。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Overshoot as a Function of Phase Margin
J. C. Daly
Electrical and Computer Engineering
University of Rhode Island
4/19/03
Figure 1
Figure 2 Amplifier frequency response.
PM w
t /w
eq
Q%OS
55o0.700 0.925 13.3% 60o0.580 0.817 8.7% 65o0.470 0.717 4.7% 70o0.360 0.622 1.4% 75o0.270 0.527 0.008%
When an amplifier with a gain A(s) is put in a
feedback loop as shown in Figure 1, the closed loop gain, V o /V in = A CL
(1)
The system is unstable when the loop gain, ß A(s), equals -1. That is, ß A(s) has a magnitude of one and a phase of -180
degrees. An unstable system oscillates. A system close to being unstable has a large ringing overshoot in response to a step input.
The phase margin is a measure of how close the phase of the loop gain is to -180 degrees, when the magnitude of the loop gain is one. The phase margin is the additional phase required to bring the phase of the loop gain to -180 degrees. Phase Margin = Phase of loop gain - (-180). The loop gain has a dominant pole at
. Higher order poles can be
represented by an equivalent pole at . The amplifier is approximated by a function with two poles as shown in Equation 2.
(2)
Since for frequencies of interest where the loop gain magnitude is close to unity,
(3)
And,
(4)
(5)
Defining ,
Table I
• PM is the phase margin. • w t is the unity gain frequency (rad/sec).
• w eq is the frequency of the equivalent higher order pole (rad/sec).
• Q is the system Quality factor.
• OS is the Over Shoot.
(6)
For frequencies of interest (frequencies close to the unity gain frequency), the amplifier gain can be written,
(7)
Plugging Equation 7 into Equation 1 results in the following expression for the closed loop gain.
(8)
Equation 8 is the transfer function for a second order system. The general form for the response of a second order system, where system properties are described by its Q and resonant frequence w o, is shown in Equation 10.
(9)
By comparing Equation 8 to Equation 9 we can get an expression for the resonant frequency and Q of the amplifier closed loop gain. (Equate coefficients of like powers of s in the dominators.)
(10)
(11)
The loop gain is the feedback factor ß multiplied by the amplifier gain A(s).
(12)
The phase margin is a function of the phase of the loop gain at the frequency where the magnitude of the loop gain is unity.
(13)
where is the loop gain unity gain frequency. It follows from Equations 12 and 13 that,
(14)
Also, solving for w ta and dividing by w eq,
(15)
It follows from Equations 11 and 15 that,
(16)
The phase of the loop gain (Equation 13) is.
Phase of loop gain(17)
The phase margin is the additional phase required to bring the phase of the loop gain to -180 degrees.
Phase Margin = Phase of loop gain - (-180).
Phase Margin(18)
A well known property of second order systems is that the percent overshoot is a function of the Q and is given by,
(19)
Both phase margin (Equation 18) and Q (Equation 16) are a function of w t / w eq. This allows us to use Equation 19 to create tables and plots of percent overshoot as a function of phase margin. As shown in Figures 3 and 4, and in Table I.
Figure 3 Overshoot as a function
of phase margin.
Plot generated using MATLAB code.Figure 4 Q as a function of phase margin.
Plot generated using MATLAB code.。
