Short-timescale Variability in the Broadband Emission of the Blazars Mkn421 and Mkn501
Brown运动的极限定理

摘 要Brown 运动的极限定理(Limit Theorem of Brownian Motion)是概率论极限理论的一个重要分支,对Brown 运动以及与Brown 运动相关随机过程轨道的性质的研究是一个广泛研究的课题.本文目的是研究布朗运动增量在一定条件下的极限定理,推广了前人的一些主要结果.本文的研究内容组织如下:第一章为绪论,介绍了Brown 运动的有关发展历史及已有的研究结果. 第二章为预备知识,介绍了一些记号与基本概念.第三章至第四章为本文研究的主要结果.在第三章,我们研究了布朗运动增量在Hölder 范数下的C-R 型局部泛函极限定理.第四章,研究了Brown 运动增量在Hölder 范数下关于,..r p C q s -容度的收敛速率.第五章,对本文工作总结及展望.关键词:Brown 运动;Hölder 范数;容度;收敛速率;AbstractLimit Theorem of Brownian motion is an important branch of Probability Limit Theorem. The topic on the path properties of Brownian motion and its relative stochastic process is widely researched. The purpose of this paper is to study Limit Theorem of Brownian motion and increments of a Brownian sheet under certain conditions. Some important results of predecessors are extended and improved. The content of this paper is organized as follows:The first chapter is an introduction which presents the development history and the existing research results of Brownian motion.The second chapter is preliminary knowledge which introduces signs and some basic concepts.The main results can be seen from chapter three to chapter four. The third chapter studies the theorem of C-R local functional limit for increments of a Brownian motion in Hölder norm. The forth chapter discusses the convergence rate of increments of a Brownian motion about the capacity of ,..r p C q s -in Hölder norm.The fifth chapter summarizes the content of this paper and makes the prospect.Key words: Brownian motion; Hölder norm; Capacity; The rate of convergence;第一章引言§1.1 Brown运动发展过程及有关应用Brown运动(Brownian motion,简称BM)在数学学科上也可以称为维纳过程,Brown运动作为具有连续时间参数和连续状态空间的一个随机过程,是随机过程学科中的最简单的、最基本的、最常见的随机过程之一.许多随机过程可看成Brown运动的推广或者泛函.Brown运动作为物理现象,首先由英国生物学家Robert Brown在1827年观察花粉微粒在液面上的“无规则运动”而提出,后来才由德耳索作出了正确的定性分析.在1905年,爱恩斯坦首次对这种“无规则运动”现象的物理规律,建立了一种数学模型,这一模型的问世使这一理论有了明显的发展.最后在Smoluchowski,Fokker,Planck,Burger,Furth,Ublenbeck等著名学者的努力下,这方面的理论工作得以迅速发展起来了.但在数学方面,由于缺少精确描述,因而进展较为缓慢,一直到了1918年才由Wiener提出了在Brown运动空间上定义测度与积分的精确且严格的数学定义,定义表明了Brown运动是一种独立增量过程,是一个具有连续时间参数和连续状态空间的随机过程.它是随机过程中最简单,最重要的特例,许多不同类型的重要随机过程都可以看做它的泛函或某种意义下的推广,这些工作推动了Brown运动研究的快速发展,并逐渐令其渗透到概率论的各个分支中,使之成为现代概率理论的重要篇章.在当今迅猛发展的时期,伴随着科学技术的快速发展和普及,又特别是计算机科学的广大应用,对Brown运动性质的探讨和研究意义深远且已取得了质的飞跃,从应用角度来看,工程技术,经济管理等广泛领域中都有“噪声”与涨落现象存在,它们往往涉及Brown运动,也就需要Brown运动的理论;又由于Brown运动与热传导方程有密切联系,使它成为概率论与分析联系的重要纽带.目前,六十年代中以来发展起来的Brown运动的极限定理已广泛地出现在多个领域中,如物理学、经济数学、通信理论、金融学、与数理统计等等学科.比如最经典的,也是较为突出的贡献就是将Brown运动与股票价格行为联系在一起,进而建立起Brown运动的股票价格数学理论模型,这是二十一世纪的一项具有突破性的重要意义的创新课题,给历史翻开了崭新的一页.当代资本市场理论的核心假设之一是Brown运动假设,市场理论认为证券期货价格具有随机性上下波动的特性,因此对Brown运动性质的研究在现代金融数学中起举足轻重的作用.因此诸多专家和学者对Brown运动及其相关的轨道性质进行了深入的研究.可见,对Brown运动的性质进行深入研究意义非常重大,这不仅极大的丰富了概率论的知识体系,而且为其实际应用提供了强而有力的理论指导.Brown运动的极限定理已经成为概率论极限理论学科中的一个比较热门的研究课题.国内外许多的概率论工作者纷纷对Brown运动的轨道的极限性质进行了广泛深入地研究.一些与Brown 运动有关的随机过程,比如广义Brown 运动[1-2]、Guass 过程[3-4]、扩散过程[5-6]、稳定过程[7]等的极限性质亦被大量研究.对Brown 运动轨道性质的研究是Brown 运动的极限定理主要研究内容之一,比如研究Brown 运动在一定的假设条件下的连续模定理[8-9]、Brown 运动Strassen 重对数律[10-11],或者Brown 运动增量有多大[12]以及增量有多小[13]的问题,还有一些泛函极限定理问题[14-15]等等.布朗运动的极限定理作为一门广泛研究的课题,随着人们的不断深入研究,将会有更多新结果.§1.2 与本文研究有关的结果对Brown 运动的轨道性质的研究是Brown 运动极限定理的重要内容,发展至今已有几十年的历史.在这几十年里,随着研究的不断深入,研究成果不断丰富和完善起来,对它的研究既深化和丰富了极限理论学科中经典理论的重要的基本结果,同时也开拓了对其他随机过程重对数律的研究.后来,对Brown 运动增量的极限定理也做了许多研究,其中最重要的内容是Csörgö-Révész 有关研究结果,我们介绍如下:设(){}0B B t t =≥;是d -维标准Brown 运动,记[][]()(){}0000d d C T f C T R f =∈=,,;;.设不减函数()():00u a ∞→∞,,,满足: (1) ()0u a u u ≤∈∞,,; (2)uua 非减; (3) ()log /limlog log u u u a u→∞=∞. 对1-维Brown 运动,Csörgö和Révész [12-13]得到如下结果. 如果u a 满足(1)、(2),则得到()()00limsup sup sup 1u uu u t u a s a B t s B t β→∞≤≤-≤≤+-=, ..a s , (1-2-1)与()()00liminf sup sup 1u uu u t u a s a B t s B t γ→∞≤≤-≤≤+-=, ..a s , (1-2-2)其中1/2log 2log u u u u u a a β-⎛⎫= ⎪⎝⎭且1/22log 8log u u u u u a a γπ-⎛⎫ ⎪⎪= ⎪ ⎪⎝⎭.若(3)也成立,则(1-2-1)与(1-2-2)的上下极限可换为极限.(1-2-1)泛函版本已被Révész [10]给出.Révész 结果如下:命题1.2.1 若条件(1)、(2)成立,则[]()0,1/limsup sup,0u u u t a u t u K β→∞∈-∆-=, ..a s (1-2-3)且对所有K ϕ∈,有[]()0,1/limsupinf,0u u t a u u t u βϕ∈-→∞∆-=, ..a s (1-2-4)其中:()()()()u t u s B ut a s B ut ∆=+-,,01t ≤≤,[]01s ∈,[](){}12'001d 1dK C t t ϕϕ=∈≤⎰,;后来,危启才将结果推广到Hölder 范数情形[15],结论如下:命题1.2.2若条件(1)、(2)成立,则 []()0,1/l i m s u ps u p ,0u u u t a ut u K αβ→∞∈-∆-=,..a s (1-2-5) 且对任意K ϕ∈,有[]()0,1/limsupinf,0u u t a u u t u αβϕ∈-→∞∆-=, ..a s (1-2-6)若条件(3)成立,则有[]()0,1/lim inf,0u u u t a u t u αβϕ→∞∈-∆-=, ..a s (1-2-7)后来,高付清、王清华[16]研究了命题1.2.1的收敛速率,其结果如下.命题1.2.3 设条件(1)、(2)成立,则对任意K ϕ∈,()1'0d 1t t ϕ<⎰,有[]()()0,1/log liminf 2loginf ,u u u t a u u u ut u b a βϕϕ→∞∈-∆-=, ..a s , (1-2-8) 其中()()1/212'0/21d d c b t t ϕϕ⎛⎫⎪= ⎪ ⎪-⎝⎭⎰,d c 是正常数,精确值见Ciesielski 和Taylor [17]. 更进一步,若条件(3)成立,则[]()()0,1/log lim 2loginf ,u u u t a u uu ut u b a βϕϕ→∞∈-∆-=, ..a s (1-2-9)特别在(1)、(2)成立时,()()1/200log log liminf infsup 1u uu u t u a s ad u u u a B t s B t c a →∞≤≤-≤≤⎛⎫ ⎪⎪+-= ⎪ ⎪⎝⎭, ..a s (1-2-10)若(3)成立,则()()1/200log log lim infsup 1u uu u t u a s ad u u u a B t s B t c a →∞≤≤-≤≤⎛⎫ ⎪⎪+-= ⎪ ⎪⎝⎭, ..a s (1-2-11)不久,高付清和王清华的研究结果被推广到Hölder 范数情形[18].命题 1.2.4如果u a 为定义于()0+∞,上的非减函数,且(1)、(2)成立,那么对任意f K ∈,且()1I f <,有[]()()10,1/l o g l i m i n f l o g i n f u u u t a uu u u t u fb f a ααβ-→∞∈-⎛⎫∆-= ⎪⎝⎭,, ..a s (1-2-12)其中()()()1/21k b f I f αα-⎛⎫= ⎪ ⎪-⎝⎭,()k α是一个正常数,精确值见Baldi 与Roynette [14].命题1.2.5 如果u a 为定义于()0+∞,上的非减函数,且 (1)、(2)与(3)成立,那么对任意f K ∈,()1I f <,有[]()()10,1/l o g l i m l o g i n f u u u t a uu u u t u fb f a ααβ-→∞∈-⎛⎫∆-= ⎪⎝⎭,, ..a s (1-2-13)其中()()()1/21k b f I f αα-⎛⎫= ⎪ ⎪-⎝⎭,()k α是正常数,精确值见Baldi 与Roynette [14].§1.3 本文的主要工作及其结构安排读研期间,在导师的指导和帮助之下,对Brown 运动的轨道的一些极限性质进行了大量的学习和探索.受到文献[15]、[16]、[22]等的启发,本文研究布朗运动的局部泛函极限定理,得到了布朗运动增量在Hölder 范数下的C-R 型局部泛函极限定理。
快速谱峭度的英文

快速谱峭度的英文Kurtosis is a statistical measure of the degree of peakedness, or the tails of a distribution relative to the normal distribution. In simple terms, it is a measure of the distribution’s outliers, or the amount of data that falls outside the typical range of values. Kurtosis can help usto understand the distribution of data, and it can assist in anomaly detection or flagging outliers.In statistics, there are several different ways to measure kurtosis, and one such measure is known as fast kurtosis.Fast kurtosis is a technique that is used to estimate kurtosis quickly and efficiently. This technique involves using a numerical algorithm to calculate the kurtosis of a given set of data. Compared to traditional methods, fast kurtosis can process large amounts of data quickly, and it isnot affected by the skewness of the data. This means that it can operate on both positively skewed and negatively skewed datasets with ease.The method of fast kurtosis uses a transform known as the fourth order moment spectrum. This transform works by generating a new set of data from the original input data, using a Fourier transformation. The new data set with the frequency domain representation is then used to calculate kurtosis quickly and efficiently. This method has the added benefit of being computationally efficient, meaning that it can be very fast, even when dealing with large data sets.Fast kurtosis has many practical applications in data science, finance, and engineering. It is commonly used in the stock market, where traders and analysts use it to flag potential anomalies in stock prices. These anomalies can signal an unexpected change in the market, which can indicate a buying or selling opportunity. Fast kurtosis is also used in econometrics, where it can be used to test for the presence of non-normality in economic data.In neuroscience, fast kurtosis is used to analyze the diffusion of water in brain tissue. Itis an important tool used to study neural connections, which can reveal information about neural processes and their changes over time. MRI scans are used to quickly analyze brain tissues, and fast kurtosis can help to identify nerve fiber bundles and other structures in the brain. This can be useful in the study of diseases such as Alzheimer's and other cognitive disorders.In conclusion, fast kurtosis is a valuable tool in statistical analysis, which enables researchers to gain insights into the kurtosis of a distribution, allowing them to identify outliers and anomalies quickly and efficiently. The speed of fast kurtosis makes it feasible to use in large datasets and in real-time scenarios. As such, it is widely used across many fields, including finance, engineering, and neuroscience. The practical applications of fast kurtosis ensure that it will remain an essential tool for the foreseeable future.。
多时间尺度气候变化

响,但自然温度的量值难以确定。
同样在一个城市,在水体附近(或公园里)的近 几十年气温趋势大约是其他观测气温趋势的一半[5]。
全球(或北半球)的平均气温趋势应该是所有地球表
面上气温趋势的面积平均值。一个比较客观的做法是
气候随时间的变化 与强迫F(t)之间在时间上不是同时
的对应关系[1],而有一个时间上的滞后
。在
大气中,L和D与大气流体的热力学性质有关,如扩散
过程等。地球上,一地气候的日气温逐小时变化,是
地球自转太阳辐射量变化的结果。描述大气热力、动
力性质的观测变量有多个,它们的变化既有天气部分
的扰动,也有气候部分的规则变化和观测误差。在一
的位相滞后太阳辐射准11a周期的位相1~2a。此外,
太阳活动和全球平均气温也都有准22a的位相循环周
期变化,气温变化的每小时速率大约是准11a变化的
一半。在全球气温变化的准11a、22a和海气耦合形成 的准60a周期中,准60a周期的气温变化幅度最大,为 0.2℃[4],变化的速率为3.8×10—7℃/h。
射不到的墙体北侧。到19世纪,温度计才进驻到了百 叶箱里。用塑料百叶箱代替木质百叶箱和用电子温度
计代替酒精温度计后,气温可降低0.4℃。志愿船舶 测量海水温度,木质吊桶与帆布吊桶取水和在船的不
同部位取水,测量到的海温也可相差0.4~0.5℃。随 着城市的发展,原来在郊区的那些气象观测仪器现在
已经在城市中心区了。气温升高也受到城市发展的影
1 引言
现代气候描述的是一段时间内气象要素和天气现 象的平均或统计状态,一段时间为月、季、年、数年 到数百年以上的尺度。气候以气温(冷、暖)和降水 (干、湿)两个基本的要素表示,通常用某一时段的 平均值和离差值表征。气候是某一时段内热平衡下的 大气多要素状态分布。
Annual and interannual (ENSO) variability of spatial scaling properties of (NDVI) in Amazonia
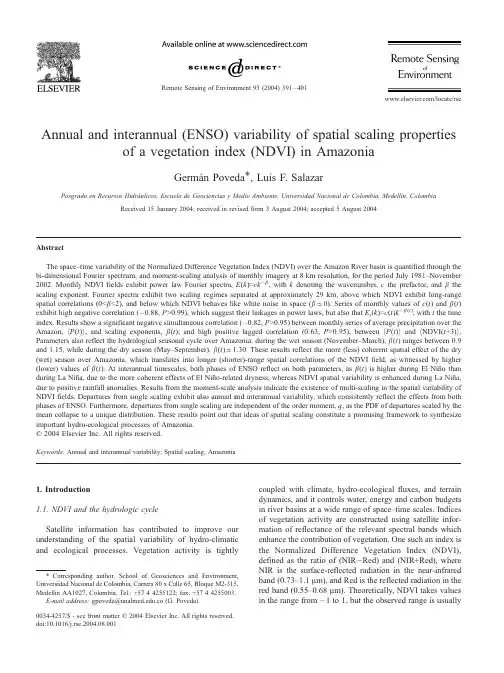
Annual and interannual (ENSO)variability of spatial scaling propertiesof a vegetation index (NDVI)in AmazoniaGerma ´n Poveda *,Luis F.SalazarPosgrado en Recursos Hidra ´ulicos,Escuela de Geociencias y Medio Ambiente,Universidad Nacional de Colombia,Medellı´n,ColombiaReceived 15January 2004;received in revised form 3August 2004;accepted 5August 2004AbstractThe space–time variability of the Normalized Difference Vegetation Index (NDVI)over the Amazon River basin is quantified through thebi-dimensional Fourier spectrum,and moment-scaling analysis of monthly imagery at 8km resolution,for the period July 1981–November 2002.Monthly NDVI fields exhibit power law Fourier spectra,E (k )=ck Àb ,with k denoting the wavenumber,c the prefactor,and b the scaling exponent.Fourier spectra exhibit two scaling regimes separated at approximately 29km,above which NDVI exhibit long-range spatial correlations (0b b b 2),and below which NDVI behaves like white noise in space (b g 0).Series of monthly values of c (t )and b (t )exhibit high negative correlation (À0.88,P N 0.99),which suggest their linkages in power laws,but also that E t (k )=c (t )k Àb (t ),with t the time index.Results show a significant negative simultaneous correlation (À0.82,P N 0.95)between monthly series of average precipitation over the Amazon,h P (t )i ,and scaling exponents,b (t );and high positive lagged correlation (0.63,P N 0.95),between h P (t )i and h NDVI(t +3)i .Parameters also reflect the hydrological seasonal cycle over Amazonia:during the wet season (November–March),b (t )ranges between 0.9and 1.15,while during the dry season (May–September),b (t )g 1.30.These results reflect the more (less)coherent spatial effect of the dry (wet)season over Amazonia,which translates into longer (shorter)-range spatial correlations of the NDVI field,as witnessed by higher (lower)values of b (t ).At interannual timescales,both phases of ENSO reflect on both parameters,as b (t )is higher during El Nin ˜o than during La Nin ˜a,due to the more coherent effects of El Nin ˜o-related dryness,whereas NDVI spatial variability is enhanced during La Nin ˜a,due to positive rainfall anomalies.Results from the moment-scale analysis indicate the existence of multi-scaling in the spatial variability of NDVI fields.Departures from single scaling exhibit also annual and interannual variability,which consistently reflect the effects from both phases of ENSO.Furthermore,departures from single scaling are independent of the order moment,q ,as the PDF of departures scaled by the mean collapse to a unique distribution.These results point out that ideas of spatial scaling constitute a promising framework to synthesize important hydro-ecological processes of Amazonia.D 2004Elsevier Inc.All rights reserved.Keywords:Annual and interannual variability;Spatial scaling;Amazonia1.Introduction1.1.NDVI and the hydrologic cycleSatellite information has contributed to improve our understanding of the spatial variability of hydro-climatic and ecological processes.Vegetation activity is tightlycoupled with climate,hydro-ecological fluxes,and terrain dynamics,and it controls water,energy and carbon budgets in river basins at a wide range of space–time scales.Indices of vegetation activity are constructed using satellite infor-mation of reflectance of the relevant spectral bands which enhance the contribution of vegetation.One such an index is the Normalized Difference Vegetation Index (NDVI),defined as the ratio of (NIR ÀRed)and (NIR+Red),where NIR is the surface-reflected radiation in the near-infrared band (0.73–1.1A m),and Red is the reflected radiation in the red band (0.55–0.68A m).Theoretically,NDVI takes values in the range from À1to 1,but the observed range is usually0034-4257/$-see front matter D 2004Elsevier Inc.All rights reserved.doi:10.1016/j.rse.2004.08.001*Corresponding author.School of Geosciences and Environment,Universidad Nacional de Colombia,Carrera 80x Calle 65,Bloque M2-315,Medellin AA1027,Colombia.Tel.:+5744255122;fax:+5744255003.E-mail address:gpoveda@.co (G.Poveda).Remote Sensing of Environment 93(2004)391–401smaller,with values around0for bare soil(low or no vegetation),and values of0.9or larger for dense vegetation. The work of Tucker(1979)pioneered the study of vegetation dynamics using red and near infrared spectral measurements.Sellers(1985)showed that NDVI is directly related to the photosynthetic capacity of plant canopies, which explains why NDVI is highly and directly correlated to the intercepted fraction of photosynthetically active radiation.As such,NDVI is independent of solar radiation, although variations in solar radiation can affect retrievals of NDVI.The meaning of diverse spectral vegetation indices is explained and summarized in Myneni et al.(1995).As NDVI represents the photosynthetic capacity or photosynthetic active radiation(PAR)absorption by green leaves,it is associated with fundamental hydro-ecological processes such as precipitation,which in turn is also directly linked to photosynthesis and hence plant growth.A recent work by Lotsch et al.(2003b)provides a comprehensive global analysis of NDVI and precipitation.Other variables pertaining to the hydrologic cycle have also been linked to NDVI,such as evaporation(Szilagyi et al.,1998;Lotsch et al.,2003b),and soil moisture(Nicholson&Farrar,1994; Farrar et al.,1994;Poveda et al.,2001).A strong relation-ship between evapotranspiration and NDVI have been identified in wet environments by Seevers and Ottmann (1994),and Nicholson et al.(1996),but also in water-limited environments,as reported by Tucker and Choudhury (1987),Malo and Nicholson(1990),Nicholson et al.(1994), Grist et al.(1997),Szilagyi et al.(1998),and Lotsch et al. (2003a).Changes in vegetation patterns have been studied at a global scale through NDVI estimates(Lucht et al., 2002).In turn,Nemani et al.(2003)identify those regions of the world where primary production is limited by water,by temperature or by both.1.2.Physical settingThe Amazon River basin provides an excellent example of the coupling and feedbacks in the land surface–atmos-phere system,due to its area larger than6.4million km2, constitute largest in the world,its tropical setting,and complex eco-hydro-climatological dynamics that exert a global influence.Scientific research towards understanding the hydro-climatic and ecological functioning of the Amazon is currently undergoing within the b Large-Scale Atmos-phere–Biosphere Experiment in Amazonia Q(LBA)(see Avissar and Nobre,2002;Roberts et al.,2003).Both observations and modelling results suggest strong changes in global,regional and local atmospheric circulation patterns associated with deforestation or perturbations in the land surface–atmosphere interactions over the Amazon(Salati& V ose,1984;Silva Dias et al.,1987;Zeng et al.,1996;Zhang et al.,1996;Poveda&Mesa,1997;Marengo&Nobre,2001; Werth&Avissar,2002;Nobre et al.,2004).The seasonal cycle of precipitation exhibits a wet season during Novem-ber–March and a dry season during May–September,as a result of the latitudinal migration of the Intertropical Convergence Zone(Obregon&Nobre,1990;Zeng,1999), which interacts with the seasonal cycle of moisture-laden low level winds from the Atlantic Ocean,but also with complex feedbacks of the land surface–atmosphere system,including the significant role of evapotranspiration in precipitation recycling(Salati,1985;Eltahir&Bras,1994).This work aims to quantify how the spatial statistics of NDVI reflect the seasonal hydro-climatic variability of the Amazon.1.3.Interannual variability at ENSO timescaleAt interannual timescales,tropical South America exhib-its coherent hydro-climatic anomalies during both phases of the El Nin˜o/Southern Oscillation(ENSO)(Aceituno,1988; Kiladis&Diaz,1989;Chu,1991;Marengo&Hastenrath, 1993;Ropelewski&Halpert,1996;Poveda et al.,2001; Waylen&Poveda,2002).With minor regional exceptions in timing and amplitude,the region experiences negative anomalies in rainfall,river discharges,and soil moisture during the warm phase of ENSO(El Nin˜o),and positive anomalies during the cold phase(La Nin˜a).Both large-scale forcing and land surface hydrology play a key role on the dynamics of hydro-climatic effects of ENSO over the region (Marengo&Hastenrath,1993;Poveda&Mesa,1997), which lag anomalies in the tropical Pacific sea surface temperatures by several months.The ENSO signal prop-agates to the east in northern South America,leading hydrological anomalies by1month over western Colombia (Poveda&Mesa,1997)and by6–10months in the Amazon River basin(Richey et al.,1989;Chu,1991;Eagleson, 1994).Consistently,NDVI diminishes over tropical South America during the occurrence of the warm phase of ENSO (Myneni et al.,1996;Asner et al.,2000;Poveda et al.,2001). This work aims to quantify how the spatial statistics of NDVI reflect the interannual hydro-climatic variability of the Amazon,associated with both phases of ENSO.1.4.Scaling theories of hydro-ecological processesScaling theories have provided important clues towards understanding and modelling the space–time dynamics of diverse bio-geophysical processes,such as vegetation sur-face fluxes(Katul et al.,2001),tropical convective storms (Yano et al.,2001),modeling of rainfall fields through fractal,multi-scaling,and random cascade models(Lovejoy, 1981,1982;Lovejoy&Schertzer,1991,1992;Gupta& Waymire,1990;Over&Gupta,1994;Perica&Foufoula-Georgiou,1996;Foufoula-Georgiou,1998;Deidda et al., 1999;Harris et al.,2000;Jotithyangkoon et al.,2000; Nordstrom&Gupta,2003),maximum annual river flows (Gupta&Waymire,1990;Gupta&Dawdy,1995;Goodrich et al.,1997;Ogden&Dawdy,2003),infiltration in porous media(Barenblatt,1996),low river flows(Furey&Gupta, 2000),ecological processes(Tilman&Kareiva,1997; Bascompte&Sole,1998),and vegetation dynamics(HarteG.Poveda,L.F.Salazar/Remote Sensing of Environment93(2004)391–401 392et al.,1999;Milne&Cohen,1999;Milne et al.,2002).For instance,in the study of river floods,Gupta(2004)has explained how scaling statistics in maximum annual river flows can be used to test different physical hypotheses covering complex runoff dynamics on channel networks.Diverse multi-scale statistical techniques are used to characterize and quantify the scale dependence of geo-biophysical fields,including Fourier spectra,structure and moment-scale functions.These functions are easily comput-able and allow an understanding of the spatial structure of the fields over a wide range of scales.Also,the use of multi-scale functions allows one to identify the range of scales where the scale dependence of modelled and observed variability may deviate,and the range of scales where the two agree(Harris et al.,2000).Towards those ends,we estimate the bi-dimensional Fourier spectra of monthly NDVI fields over Amazonia,and quantify the time variability of its parameters,and how they reflect the time–space variability of NDVI and precipitation fields at annual and interannual timescales.By the same token,we like to investigate whether monthly NDVI fields exhibit simple of multi-scaling properties in space,and how they reflect the annual and interannual variability of NDVI. Thirdly,we investigate the time correlation between series of monthly values of c and b with average values of NDVI and precipitation over the entire Amazon,so as to encapsulate the hydro-ecological dynamics of Amazonia. The data sets and methodologies are described in Section2, while results are presented in Section3,and the conclusions are provided in Section4.2.Data sets and methodologiesWe used digital maps of monthly NDVI from the NASA Global Inventory Modeling and Mapping Studies (GIMMS NDVI),covering the period July1981through November2002.The imagery,which consists of8km spatial resolution NDVI images,was provided by C.J. Tucker and his colleagues at NASA Goddard Space Flight Center.The GIMMS NDVI database exhibits a great deal of improvements with respect to previous NDVI data sets, including corrections for:(i)residual sensor degradation and sensor intercalibration differences;(ii)distortions caused by persistent cloud cover in tropical evergreen broadleaf forests;(iii)solar zenith angle and viewing angle effects;(iv)volcanic aerosols;(v)missing data in the Northern Hemisphere during winter using interpolation; and(vi)short-term atmospheric aerosol effects,atmos-pheric water vapor effects,and cloud cover.For details of the GIMMS NDVI data set,see Pinzo´n et al.(submitted for publication).The GIMMS NDVI data set has been rescaled in such a way that the original values in theÀ1 to1range are obtained as ndvi=(NDVIÀ1)/249À0.05, with values larger than1representing water bodies or bad data.Precipitation data for the Amazon basin were obtained from the data set produced by the Earth Observing System-Amazon Project(EOSAP)developed by Instituto Nacional de Pesquisas Espaciais(INPE),Brazil,and the University of Washington,and contains gridded monthly rainfall(0.28 latitudeÂ0.28longitude),for the period1972–1992.This data set was provided by the Global Hydrology and Climate Center of NASA.For details of this data set,see http:// /.With the purpose of implementing the spatial scaling analysis,a2048km scale region was defined inside the Amazon basin.The observed NDVI field for July1981is shown in Fig.1,aggregated at a32km scale.Character-ization of the spatial scaling properties of NDVI monthly fields was performed through estimation of the bi-dimen-sional Fourier spectrum,and moment-scale analysis.A detailed description of the methods is provided in the following section.2.1.Bi-dimensional Fourier spectrumMany geophysical phenomena exhibit power law decay-ing Fourier spectra,(Korvin,1992;Mandelbrot,1998),i.e., E kðÞf kÀb¼ckÀbð1Þwith k being the wavenumber,c is the prefactor,and b is the scaling exponent.The spectral slope,b,becomes a measure of roughness(Davis et al.,1996;Harris et al., 1996),with low spectral slopes corresponding to rougher, less correlated fields.Scaling exponents in Fourier spectra contain key insights on the dynamics underlying the physics of highly complex phenomena.For instance,the well-known behavior of dissipation of kinetic energy in turbulent flows,for which E(k)~k5/3(Kolmogorov,1941, 1962;Frisch,1995),whose scaling exponent,5/3,summa-rizes the rate at which kinetic energy is gradually trans-ferred from larger to smaller spatial scales,such that the mean kinetic energy per unit mass per unit time is conserved.Many other geophysical phenomena exhibit power law Fourier spectra,whose scaling exponents reflect different types of statistical memory and the scale of fluctuation which is inherent to their space–time correla-tions(Korvin,1992;Mesa&Poveda,1993;Turcotte, 1997;Mandelbrot,1998;Yano et al.,2001).The bi-dimensional power spectrum is computed using standard2-D Fast Fourier Transform(FFT)algorithms (Press et al.,1992).The Fourier power or energy spectrum, E(k x,k y)of a two-dimensional field,is found by multiplying the2-D FFT by its complex conjugate,where k x and k y are the wavenumber components(Harris et al.,2000).To facilitate visualization and comparison,the2-D power spectra from the NDVI fields are averaged angularly about k x=k y=0to produce the isotropic energy spectrum,E(k), with k¼ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffik2xþk2yq.Such isotropic energy spectrum does not mean that the field is isotropic,but rather that the angularG.Poveda,L.F.Salazar/Remote Sensing of Environment93(2004)391–401393averaging about k x =k y =0integrates the anisotropy (Harris et al.,2000).2.2.Moment-scaling analysisMoment-scaling analysis allows the quantification of the spatial intermittency (roughness)of a field,and provides a test for the type of spatial (single or multi-)scaling behavior of random fields (Over &Gupta,1994;Harris et al.,2000).Statistical self-similarity can be thought of as statistical similarity of a random field across multiple scales,then simple scaling is a type of statistical self-similarity.Consider a random field,{X (t );t a I },where I represents an index set,and an arbitrary scalar k N 0.The random field is defined to be simple scaling if the following holds,X k t ðÞ¼dk h X t ðÞð2Þwhere the equality is understood in the sense of all finite dimensional distribution functions.From the definition of statistical moments given by E [X q]=R x q f (x )d x ,q =1,2,3,...,it is concluded that for a simple scaling random field,X (t ),E X q k t ðÞ½ ¼k h q E X q t ðÞ½ ;q ¼1;2;3;Nor ;log E X q k t ðÞ½ ¼q h log k þc q t ðÞ;ð3Þwhere c q (t )=log E [X q (t )].Eq.(3)shows that simple scaling must satisfy two conditions:(i)log–log linearity;(ii)linear slope growth,i.e.,s (q )=q h ,whereas multi-scaling holds for a nonlinear slope growth.In our case,the expected value in Eq.(3)arises from the equation that defines the scaling moments of a field X j ,which are computed for a range of averaging scales,r ,with higher values of r implying examining the phenomena at finer spatial resolution.Therefore,M q r ðÞ¼hj X r x ;y ðÞj q ið4Þwhere X r represents field values at scale r ,q is the order of the moment,and h ...i denotes the expected value of NDVI over all pixels at scale r .Typically,the scale of the image is dyadically reduced from its original highest resolution (r =1/(1pixel))by successive spatial averaging of the field by a factor of 2at each step,i.e.,r =1/(2pixels)=0.5,r =1/(4pixels)=0.25,...,r =1/(256pixels)=3.9Â10À3.Scaling of the moments means that (Gupta &Waymire,1993),M q r ðÞf r Às q ðÞð5Þwhere s (q )is the moment scaling exponent function that is estimated by log–log linear regressions of the q th moment of the NDVI field,on a scan by scan basis,as |X r |vs.log r ,for each q .It is easy to check that s (1)=0since the mean of the entire field does not depend upon the scale.Thelog–logFig.1.Location of the study region depicting the NDVI field for July 1981,aggregated at a 32km scale.G.Poveda,L.F .Salazar /Remote Sensing of Environment 93(2004)391–401394linearity of log M q (r )vs.log r provides a test of the scaling hypothesis for the moment of order q .3.Results3.1.Bi-dimensional Fourier spectrumOur generalized results indicate that the Fourier spectra exhibit two regions characterized by different scaling exponents,b ,separated at the wavenumber k =0.034km À1,which corresponds to 28.6km.Fig.2shows the 2-D Fourier spectra for the September 1989NDVI field.At larger spatial scales,the NDVI fields exhibit long-range correlations characterized by 0b b b 2,whereas for larger wavenumbers (smaller spatial scales),the spectrum becomes scale independent,with b g 0,thus meaning that the spatial variability of NDVI behaves irregularly,as white noise in space.Long-range correlations in the spatial distribution of water and energy-limited vegetation have been identified for the Columbia River basin in the USA (Milne et al.,2002).Analysis of the time evolution of monthly estimated values of scaling exponents,b (t ),and prefactors,c (t ),t =1,...,257,indicates a high negative correlation coefficient (À0.88,P N 0.95),as shown in Fig.3,which means that c (t )=f [b (t )],with f [d ]representing a linear function.This result points out to the existence of a strong association between these two parameters in power laws and scaling relationships;an idea that was introduced in the context of the Hurst effect in geophysical records (Mesa &Poveda,1993),which deserves further investigation.Furthermore,our results indicate that both parameters of the Fourier spectra vary with time,and thus E t (k )=c (t )k Àb (t ),where k denotes the wavenumber,and t represents the time index.Time series of monthly values of average precipitation and NDVI over the Amazon were estimated by averaging values of each field for a fixed month,as,h P t ðÞi ¼1=nXn i ¼1p i !t;and h NDVI t ðÞi ¼1=nX n i ¼1ndvi i!tð6Þwhere n denotes the number of pixels with information foreach field:12,991for precipitation,and 65,536for NDVI.Results show a significant negative correlation (À0.82,P N 0.95)between monthly values of average precipitation,h P (t )i over the Amazon and scaling exponents,b (t ),as illustrated in Fig.4.Such negative correlation indicates that wet months exhibit rougher (less spatially correlated)NDVI fields,which are encapsulated in lower values of b (t ).On the contrary,dry periods are associated with more coherent and longer-range correlated NDVI fields,which are reflected in higher values of b (t ).Accordingly,the afore-mentioned seasonal cycle of average precipitation,and the concomitant spatial variability of NDVI are also reflectedinFig.2.Bi-dimensional Fourier spectra of the NDVI field for September 1989,with scaling exponent,b =1.11,and prefactor,c =0.034.E (k )has arbitrary units,as NDVI is dimensionless.Values of NDVI are rescaled is such a manner that original data are recovered as ndvi=(NDVI À1)/249–0.05.The range of spatial scales covers from 512to 16km.The dotted line separates the two scaling regions of the spectrum at k =0.035km À1,which corresponds to a spatial scale of 28.6km.Fig.3.Time evolution of prefactors,c (t ),and scaling exponents,b (t ),for estimated bi-dimensional Fourier spectra of NDVI monthly fields,during the study period.Simultaneous correlation coefficient is À0.88(P N0.99).Fig. 4.Time evolution of monthly mean precipitation,h P (t )i ,over Amazonia and scaling exponents,b (t ).Simultaneous correlation is À0.82(P N 0.95).G.Poveda,L.F .Salazar /Remote Sensing of Environment 93(2004)391–401395a high positive correlation coefficient (0.72,P N 0.95)between the monthly series of average precipitation,h P (t )i and that of 6-month lagged scaling exponents,hb (t +6)i (not shown here).Despite that no significant correlation (À0.062)appears between the series of h P (t )i and h NDVI(t )i ,there is a significant positive correlation at 3-month lag (0.63,P N 0.95),see Fig.5,when precipitation leads NDVI.Such time lag suggests an integrated timescale at which rainfall affects NDVI dynamics at basin scale.The physical origin of this observation lies in the complex interactions of the land surface–atmosphere system,which include the afore-mentioned important effect of precipitation recycling in Amazonia (Salati,1985;Eltahir &Bras,1994).This observation deserves further investigation.3.1.1.Annual and interannual timescalesThe average long-term annual cycle of b (t )and c (t )were estimated from the 257estimated values (July 1981–November 2002).There is a strong negatively correlated seasonal cycle of prefactors,c (t ),and scaling exponents,b (t ),as evidenced in Fig.6.During the wet season (November–March),the estimated values of b (t )lie between 0.9and 1.15,while during the dry season (May–September),higher values are on the order of b (t )=1.30.These results are explained by the more coherent spatial effects of the dry season over the Amazon basin,which produce long-range spatial correlations in the NDVI field,reflected in higher estimates of b (t ).The annual cycle of prefactors,c (t ),exhibit higher values (~0.09–0.10)during the wet season,and lower values (~0.02–0.30)during the dry season.An understanding of the physical processes that govern such a strong association between scaling exponents and prefactors at seasonal scales is a topic of further research.At interannual timescales,ENSO strongly affects vege-tation activity and NDVI variability in Amazonia (Gutman,1991;Kogan &Sullivan,1993;Myneni et al.,1996;Asner et al.,2000;Poveda et al.,2001;Pinzo ´n,2002).Fig.7shows the annual cycle of scaling exponents,b (t ),during two contrasting ENSO years,i.e.,the 1991–1992El Nin ˜o,and the 1988–1989La Nin ˜a,as well as the average during normal years.The annual cycle is defined from June (year 0)through May (year +1),to better capture the aforementioned delayed effects of both ENSO phases.Overall,results indicate that the phase of the annual cycle of b (t )remains unchanged during both phases of ENSO,but there is a clear-cut effect on its amplitude.This is evidenced by higher values of b (t )during El Nin ˜o as compared with those during La Nin ˜a and normal years,throughout the annual cycle.Interestingly enough,the highest values of the scaling exponent are attained during August for both ENSO phases,and the lower values appears in February–March during El Nin ˜o,and in November–February during La Nin ˜a.This observation can be explained by the spatially coherent dryness caused over the Amazon by the warm phase of ENSO.It is well known that,in general,the Amazon basin experiences strong droughts during El Nin ˜o,and positive rainfall anomalies during La Nin ˜a (Richey et al.,1989;Fig.5.Time evolution of average values of monthly precipitation,h P (t )i ,and NDVI h NDVI(t )i ,over Amazonia.The caption of Fig.2explains the range of NDVI values.The low simultaneous correlation coefficient (À0.06)increases to À0.63(P N 0.95),when precipitation lead values of NDVI by 3months.Fig.6.Long-term annual cycle of estimated prefactors,c (t ),and scaling exponents,b (t ),from the estimated 2-D Fourierspectra.Fig.7.Annual cycle of scaling exponents,b (t ),during the 1991–1992El Nin ˜o event,the 1988–1989La Nin ˜a event,and during normal years.G.Poveda,L.F .Salazar /Remote Sensing of Environment 93(2004)391–401396Marengo,1992;Marengo &Hastenrath,1993;Obregon &Nobre,1990;Poveda &Mesa,1997;Poveda et al.,2001),whose effects are stronger in northern and central Amazonia(Marengo et al.,1998).The most remarkable differences occur in the November–March wet season during both phases of ENSO.During this epoch,both ENSO phases attain their maximum amplitude,and the associated tele-connections are more strongly developed,which in con-junction with land surface–atmosphere feedbacks cause stronger hydro-ecological anomalies,which affect the NDVI response over the Amazon.Our results confirm the coherent large spatial scale effects of El Nin ˜o-related drought over the Amazon basin,as a result of the 1991–1992event.It is concluded that scaling exponents,b (t ),exhibit significant variability at ENSO timescales,which are consistent with and reflect the identified hydrological anomalies.Similar to the temporal behavior of h NDVI(t )i ,results for the scaling exponents confirm that NDVI fields are more spatially correlated during El Nin ˜o than during La Nin ˜a.The interannual variability associated with both phases of ENSO is consistently exemplified by the evolution of b (t )and c (t )during the 1997–1998El Nin ˜o event,and during the 1998–2000La Nin ˜a event,shown in Fig.3.3.2.Moment-scaling analysisMoment-scale analysis were performed after checking for the condition that b (t )b 2(Harris et al.,2000).Fig.8shows the results for July 1991,with the scaling of marginal moments with q =0.5,1.0,...,4(top),and the estimated s ðq ˆÞcurve (bottom).Results indicate that monthly NDVI fields exhibit multi-scaling behavior in space,as indicated by the nonlinear behavior of the s ðq ˆÞfunction.Deviations from simple scaling were quantified as D q ¼s ðq ˆÞobserved Às q ðÞtheoretical ,e.g.,the difference between the sample values of the function s ðq ˆÞ,with respect to the linear growth for simple scaling (see Fig.9).Two features are worth mentioning:(i)there is a clear-cut annualandFig.8.Scaling of marginal moments with q =0.5,1.0,...,4(top),and estimated s ðq ˆÞcurve (bottom),for NDVI in July 1991.The straight continuous line and 95%confidence intervals (dashed)in the bottom pannel denote the theoretical behavior for simplescaling.Fig.9.Time evolution of departures from simple scaling,of the estimated s ðq ˆÞcurve,for q =1.5,...,4.0,during the study period.G.Poveda,L.F .Salazar /Remote Sensing of Environment 93(2004)391–401397。
频闪视觉训练在体育运动中的应用现状及发展趋势研究

第40卷第1期2021年2月福建体育科技Fujian Sports Science and TechnologyVol.40No.1February2021频闪视觉训练在体育运动中的应用现状及发展趋势研究张致玮,贾谊(中北大学体育学院,山西太原030051)摘要:频闪视觉训练是一种新型的训练形式,该训练模式由个体在间歇性视觉条件下进行训练,目的是在正常视觉条件下提高后续能力。
介绍了频闪视觉训练的相关原理,列举和说明了频闪视觉训练在体育运动中的应用现状和使用效果。
大量的研究表明频闪视觉训练对不同运动项目、不同领域、不同人群的好处,这对进一步推广频闪视觉训练具有重要意义。
最后,讨论了频闪视觉训练现有研究的不足和未来的发展趋势。
关键词:频闪视觉训练;运动;应用现状;发展趋势文章编号:1004-8790(2021)01-0043-04中图分类号:G808.12文献标识码:A Research on the Application Status and Development Trend of Stroboscopic Vision Training in SportsZHANG Zhi-wei,JIA Yi(School of Physical and Education,North University,Taiyuan030051,China)Abstract:Stroboscopic vision training is a new form of training,which is trained by individuals under intermittent vision condition to improve subsequent performance under normal vision condition.This paper introduces the relevant principles of stroboscopic vision training,enumerates and illustrates the application status and effect of stroboscopic vision training in sports.A large number of studies have shown the benefits of stroboscopic visual training for different sports,different fields and different populations,which is of great significance to further popularize stroboscopic visual training.Finally,the deficiencies of current studies and the future development trend of stroboscopic vision training are discussed.Keywords:stroboscopic vision training;movement;application status;development trend人类所获取的感官信息80%以上来自视觉。
MJO对我国天气气候影响的新事实

MJO对我国天气气候影响的新事实任宏利;沈雨旸【摘要】在次季节时间尺度上,热带大气季节内振荡(MJO)是全球气候变率的首要模态。
MJO不仅对热带天气气候产生直接影响,还能够通过传播和激发大气遥相关等方式对热带外地区产生重要影响,成为目前次季节—季节气候预测最重要的可预报性来源。
MJO对于我国天气气候影响的探索由来已久,在很多方面有了显著进展,但仍需深入研究。
首先对MJO影响我国天气气候的过往研究进行了回顾,并进一步利用新的观测资料诊断分析,发现了MJO对我国气候影响的一些新事实。
初步结论包括:MJO对我国冬季降水的影响主要局限在江南—华南区域,而夏季扩展到南方和青藏高原地区;对冬季气温的影响较大,其范围覆盖了东北、华北以及西部广大区域,而夏季解释方差有所减小,其区域位于除了黄河流域以外的广大地区;在去掉高频噪音后,纯粹MJO信号对我国主要区域气温和降水低频变化的解释方差可接近30%;MJO对我国冬夏季温度降水的影响存在明显滞后效应,应在使用MJO信号进行我国气象要素预报时加以考虑。
%Madden-Julian Oscillation (MJO) or more generally called tropical Intra-Seasonal Oscillation (ISO), dominates climate variability worldwide on the subseasonal timescale. It is well-known that MJO can impact weather and climate not only in tropics, but also in extratropics through the propagation and excitation of atmospheric teleconnections, and is the most important predictability source in the subseasonal-to-seasonal prediction. A great many studies have focused on impacts of MJO on weather and climate in China in the past decades, and made a signiifcant progress in different aspects. However, further deep understanding is stillneeded. This study firstly reviews previous studies of impacts of MJO on China weather and climate, and then shows the new results regarding such impacts of MJO, based on diagnoses of new observations, then gives preliminary conclusionsas followings:. the impacts of MJO on rainfall are mainly limited in the regions from south of Yangtze River to the South of China in winter, but are extended to the more southern and Tibetan Plateau areas in summer. It shows a signiifcant inlfuence of MJO on winter temperature over the Northeast of China, the North of China, and most of the western China, while a relatively weakened impact on summer temperature over the large areas in China except for the Yellow River Basin. After removing high-frequency noise, pure MJO signal can explain about 30% variance of low-frequency surface air temperature and rainfall in China. Moreover, it is found that there is a delayed impact of MJO on temperature and rainfall in both winter and summer in China and it is need to be consideredin the future predictions and futher studies .【期刊名称】《气象科技进展》【年(卷),期】2016(006)003【总页数】9页(P97-105)【关键词】MJO;中国气候;降水;气温;影响【作者】任宏利;沈雨旸【作者单位】国家气候中心,中国气象局气候研究开放实验室,中国气象局-南京大学气候预测研究联合实验室,北京 100081;中国气象局公共气象服务中心,北京 100081【正文语种】中文时间尺度介于10~90 d的大气季节内振荡(Intra-seasonal oscillation,ISO)是气候系统中最重要的大气环流现象之一。
高斯朴素贝叶斯训练集精确度的英语
高斯朴素贝叶斯训练集精确度的英语Gaussian Naive Bayes (GNB) is a popular machine learning algorithm used for classification tasks. It is particularly well-suited for text classification, spam filtering, and recommendation systems. However, like any other machine learning algorithm, GNB's performance heavily relies on the quality of the training data. In this essay, we will delve into the factors that affect the training set accuracy of Gaussian Naive Bayes and explore potential solutions to improve its performance.One of the key factors that influence the training set accuracy of GNB is the quality and quantity of the training data. In order for the algorithm to make accurate predictions, it needs to be trained on a diverse and representative dataset. If the training set is too small or biased, the model may not generalize well to new, unseen data. This can result in low training set accuracy and poor performance in real-world applications. Therefore, it is crucial to ensure that the training data is comprehensive and well-balanced across different classes.Another factor that can impact the training set accuracy of GNB is the presence of irrelevant or noisy features in the dataset. When the input features contain irrelevant information or noise, it can hinder the algorithm's ability to identify meaningful patterns and make accurate predictions. To address this issue, feature selection and feature engineering techniques can be employed to filter out irrelevant features and enhance the discriminative power of the model. Byselecting the most informative features and transforming them appropriately, we can improve the training set accuracy of GNB.Furthermore, the assumption of feature independence in Gaussian Naive Bayes can also affect its training set accuracy. Although the 'naive' assumption of feature independence simplifies the model and makes it computationally efficient, it may not hold true in real-world datasets where features are often correlated. When features are not independent, it can lead to biased probability estimates and suboptimal performance. To mitigate this issue, techniques such as feature extraction and dimensionality reduction can be employed to decorrelate the input features and improve the training set accuracy of GNB.In addition to the aforementioned factors, the choice of hyperparameters and model tuning can also impact the training set accuracy of GNB. Hyperparameters such as the smoothing parameter (alpha) and the covariance type in the Gaussian distribution can significantly influence the model's performance. Therefore, it is important to carefully tune these hyperparameters through cross-validation andgrid search to optimize the training set accuracy of GNB. By selecting the appropriate hyperparameters, we can ensure that the model is well-calibrated and achieves high accuracy on the training set.Despite the challenges and limitations associated with GNB, there are several strategies that can be employed to improve its training set accuracy. By curating a high-quality training dataset, performing feature selection and engineering, addressing feature independence assumptions, and tuning model hyperparameters, we can enhance the performance of GNB and achieve higher training set accuracy. Furthermore, it is important to continuously evaluate and validate the model on unseen data to ensure that it generalizes well and performs robustly in real-world scenarios. By addressing these factors and adopting best practices in model training and evaluation, we can maximize the training set accuracy of Gaussian Naive Bayes and unleash its full potential in various applications.。
选择与坚持:跨期选择与延迟满足之比较
第2期
任天虹等 : 选择与坚持:跨期选择与延迟满足之比较
305
会通过预实验选择一对较为恰当的 SS 与 LL, 以 保证 SS 与 LL 之间的差异既要大到足以使被试愿 意选择后者 , 又要小到使 SS 对儿童有足够的诱惑 , 从而避免等待时间的天花板效应与地板效应 (Mischel & Underwood, 1974)。 动物实验在跨期选择与延迟满足的研究中都 有 所 涉 及 , 这 些动 物研 究 的 实验 范式 直 观 易 懂 , 对儿童被试的研究也颇有启示意义。随着研究内 容的扩展与研究范式的改进 , 跨期选择与延迟满 足在研究对象上有融合的趋势。 3.2 研究内容 跨期选择关注被试的时间折扣 (time discounting), 而延迟满足则更关注被试在等待时间上的 个 体 差 异 、 自 我 控 制 策 略 及 其 有 效 性 (Ainslie, 1975; Mischel et al., 1989)。如果说跨期选择的研 究者将其研究重点放在了计算、分析、推理、权 衡等较为高级的认知过程上 , 那么延迟满足的研 究者则将其重点放在了情绪、意志力、动机强度 等更为基础的本能反应上。 时间折扣是跨期选择研究的基本假设 , 也是 其研究的重要内容 , 它是指在跨期选择中 , 个体 首先会对延迟结果的价值根据其延迟的时间进行 一定的折扣, 然后再对两个结果进行比较(Frederick et al., 2002; Scholten & Read, 2010)。经济学家力 图找到某一通用的公式来描述折扣程度与结果及 延 迟 时 间 之间 的 关 系 , 表 现 为 数 理 模 型 的 优化 ; 心理学家则更关注外界因素对个体时间折扣程度 的影响 , 表现为认知神经机制的揭示。 延迟满足的研究者并不关注折扣程度 , 他们 更加关注等待时间上的个体差异 , 并在实验中细 致地检验被试自我控制策略的选择与使用状况 , 这些研究者热衷于以追踪研究揭示儿童在实验中 的表现与其个性及行为特征之间的关系 (Mischel et al., 1989)。 3.3 研究范式 跨期选择的研究关注被试的选择过程 , 它要 求被试做出一系列选择 ; 延迟满足则更关注被试 的坚持过程 , 要求被试坚持完成选择后的等待过 程。尽管在跨期选择的部分任务中也涉及到等待 过程 , 但是它与延迟满足有本质的区别。被试在 延迟满足任务中能够自主选择中止等待 , 而在跨 期选择任务中则只能消极等待延时结束 (Evans & Beran, 2007)。
Peters (2010) Episodic Future Thinking Reduces Reward Delay Discounting
NeuronArticleEpisodic Future Thinking ReducesReward Delay Discounting through an Enhancement of Prefrontal-Mediotemporal InteractionsJan Peters1,*and Christian Bu¨chel11NeuroimageNord,Department of Systems Neuroscience,University Medical Center Hamburg-Eppendorf,Hamburg20246,Germany*Correspondence:j.peters@uke.uni-hamburg.deDOI10.1016/j.neuron.2010.03.026SUMMARYHumans discount the value of future rewards over time.Here we show using functional magnetic reso-nance imaging(fMRI)and neural coupling analyses that episodic future thinking reduces the rate of delay discounting through a modulation of neural decision-making and episodic future thinking networks.In addition to a standard control condition,real subject-specific episodic event cues were presented during a delay discounting task.Spontaneous episodic imagery during cue processing predicted how much subjects changed their preferences toward more future-minded choice behavior.Neural valuation signals in the anterior cingulate cortex and functional coupling of this region with hippo-campus and amygdala predicted the degree to which future thinking modulated individual preference functions.A second experiment replicated the behavioral effects and ruled out alternative explana-tions such as date-based processing and temporal focus.The present data reveal a mechanism through which neural decision-making and prospection networks can interact to generate future-minded choice behavior.INTRODUCTIONThe consequences of choices are often delayed in time,and in many cases it pays off to wait.While agents normally prefer larger over smaller rewards,this situation changes when rewards are associated with costs,such as delays,uncertainties,or effort requirements.Agents integrate such costs into a value function in an individual manner.In the hyperbolic model of delay dis-counting(also referred to as intertemporal choice),for example, a subject-specific discount parameter accurately describes how individuals discount delayed rewards in value(Green and Myer-son,2004;Mazur,1987).Although the degree of delay discount-ing varies considerably between individuals,humans in general have a particularly pronounced ability to delay gratification, and many of our choices only pay off after months or even years. It has been speculated that the capacity for episodic future thought(also referred to as mental time travel or prospective thinking)(Bar,2009;Schacter et al.,2007;Szpunar et al.,2007) may underlie the human ability to make choices with high long-term benefits(Boyer,2008),yielding higher evolutionaryfitness of our species.At the neural level,a number of models have been proposed for intertemporal decision-making in humans.In the so-called b-d model(McClure et al.,2004,2007),a limbic system(b)is thought to place special weight on immediate rewards,whereas a more cognitive,prefrontal-cortex-based system(d)is more involved in patient choices.In an alternative model,the values of both immediate and delayed rewards are thought to be repre-sented in a unitary system encompassing medial prefrontal cortex(mPFC),posterior cingulate cortex(PCC),and ventral striatum(VS)(Kable and Glimcher,2007;Kable and Glimcher, 2010;Peters and Bu¨chel,2009).Finally,in the self-control model, values are assumed to be represented in structures such as the ventromedial prefrontal cortex(vmPFC)but are subject to top-down modulation by prefrontal control regions such as the lateral PFC(Figner et al.,2010;Hare et al.,2009).Both the b-d model and the self-control model predict that reduced impulsivity in in-tertemporal choice,induced for example by episodic future thought,would involve prefrontal cortex regions implicated in cognitive control,such as the lateral PFC or the anterior cingulate cortex(ACC).Lesion studies,on the other hand,also implicated medial temporal lobe regions in decision-making and delay discounting. In rodents,damage to the basolateral amygdala(BLA)increases delay discounting(Winstanley et al.,2004),effort discounting (Floresco and Ghods-Sharifi,2007;Ghods-Sharifiet al.,2009), and probability discounting(Ghods-Sharifiet al.,2009).Interac-tions between the ACC and the BLA in particular have been proposed to regulate behavior in order to allow organisms to overcome a variety of different decision costs,including delays (Floresco and Ghods-Sharifi,2007).In line with thesefindings, impairments in decision-making are also observed in humans with damage to the ACC or amygdala(Bechara et al.,1994, 1999;Manes et al.,2002;Naccache et al.,2005).Along similar lines,hippocampal damage affects decision-making.Disadvantageous choice behavior has recently been documented in patients suffering from amnesia due to hippo-campal lesions(Gupta et al.,2009),and rats with hippocampal damage show increased delay discounting(Cheung and Cardinal,2005;Mariano et al.,2009;Rawlins et al.,1985).These observations are of particular interest given that hippocampal138Neuron66,138–148,April15,2010ª2010Elsevier Inc.damage impairs the ability to imagine novel experiences (Hassa-bis et al.,2007).Based on this and a range of other studies,it has recently been proposed that hippocampus and parahippocam-pal cortex play a crucial role in the formation of vivid event repre-sentations,regardless of whether they lie in the past,present,or future (Schacter and Addis,2009).The hippocampus may thus contribute to decision-making through its role in self-projection into the future (Bar,2009;Schacter et al.,2007),allowing an organism to evaluate future payoffs through mental simulation (Johnson and Redish,2007;Johnson et al.,2007).Future thinking may thus affect intertemporal choice through hippo-campal involvement.Here we used model-based fMRI,analyses of functional coupling,and extensive behavioral procedures to investigate how episodic future thinking affects delay discounting.In Exper-iment 1,subjects performed a classical delay discounting task(Kable and Glimcher,2007;Peters and Bu¨chel,2009)that involved a series of choices between smaller immediate and larger delayed rewards,while brain activity was measured using fMRI.Critically,we introduced a novel episodic condition that involved the presentation of episodic cue words (tags )obtained during an extensive prescan interview,referring to real,subject-specific future events planned for the respective day of reward delivery.This design allowed us to assess individual discount rates separately for the two experimental conditions,allowing us to investigate neural mechanisms mediating changes in delay discounting associated with episodic thinking.In a second behavioral study,we replicated the behavioral effects of Exper-iment 1and addressed a number of alternative explanations for the observed effects of episodic tags on discount rates.RESULTSExperiment 1:Prescan InterviewOn day 1,healthy young volunteers (n =30,mean age =25,15male)completed a computer-based delay discounting proce-dure to estimate their individual discount rate (Peters and Bu ¨-chel,2009).This discount rate was used solely for the purpose of constructing subject-specific trials for the fMRI session (see Experimental Procedures ).Furthermore,participants compiled a list of events that they had planned in the next 7months (e.g.,vacations,weddings,parties,courses,and so forth)andrated them on scales from 1to 6with respect to personal rele-vance,arousal,and valence.For each participant,seven subject-specific events were selected such that the spacing between events increased with increasing delay to the episode,and that events were roughly matched based on personal rele-vance,arousal,and valence.Multiple regression analysis of these ratings across the different delays showed no linear effects (relevance:p =0.867,arousal:p =0.120,valence:p =0.977,see Figure S1available online).For each subject,a separate set of seven delays was computed that was later used as delays in the control condition.Median and range for the delays used in each condition are listed in Table S1(available online).For each event,a label was selected that would serve as a verbal tag for the fMRI session.Experiment 1:fMRI Behavioral ResultsOn day 2,volunteers performed two sessions of a delay dis-counting procedure while fMRI was measured using a 3T Siemens Scanner with a 32-channel head-coil.In each session,subjects made a total of 118choices between 20V available immediately and larger but delayed amounts.Subjects were told that one of their choices would be randomly selected and paid out following scanning,with the respective delay.Critically,in half the trials,an additional subject-specific episodic tag (see above,e.g.,‘‘vacation paris’’or ‘‘birthday john’’)was displayed based on the prescan interview (see Figure 1)indicating which event they had planned on the particular day (episodic condi-tion),whereas in the remaining trials,no episodic tag was pre-sented (control condition).Amount and waiting time were thus displayed in both conditions,but only the episodic condition involved the presentation of an additional subject-specific event tag.Importantly,nonoverlapping sets of delays were used in the two conditions.Following scanning,subjects rated for each episodic tag how often it evoked episodic associations during scanning (frequency of associations:1,never;to 6,always)and how vivid these associations were (vividness of associa-tions:1,not vivid at all;to 6,highly vivid;see Figure S1).Addition-ally,written reports were obtained (see Supplemental Informa-tion ).Multiple regression revealed no significant linear effects of delay on postscan ratings (frequency:p =0.224,vividness:p =0.770).We averaged the postscan ratings acrosseventsFigure 1.Behavioral TaskDuring fMRI,subjects made repeated choices between a fixed immediate reward of 20V and larger but delayed amounts.In the control condi-tion,amounts were paired with a waiting time only,whereas in the episodic condition,amounts were paired with a waiting time and a subject-specific verbal episodic tag indicating to the subjects which event they had planned at the respective day of reward delivery.Events were real and collected in a separate testing session prior to the day of scanning.NeuronEpisodic Modulation of Delay DiscountingNeuron 66,138–148,April 15,2010ª2010Elsevier Inc.139and the frequency/vividness dimensions,yielding an‘‘imagery score’’for each subject.Individual participants’choice data from the fMRI session were then analyzed byfitting hyperbolic discount functions to subject-specific indifference points to obtain discount rates (k-parameters),separately for the episodic and control condi-tions(see Experimental Procedures).Subjective preferences were well-characterized by hyperbolic functions(median R2 episodic condition=0.81,control condition=0.85).Discount functions of four exemplary subjects are shown in Figure2A. For both conditions,considerable variability in the discount rate was observed(median[range]of discount rates:control condition=0.014[0.003–0.19],episodic condition=0.013 [0.002–0.18]).To account for the skewed distribution of discount rates,all further analyses were conducted on the log-trans-formed k-parameters.Across subjects,log-transformed discount rates were significantly lower in the episodic condition compared with the control condition(t(29)=2.27,p=0.016),indi-cating that participants’choice behavior was less impulsive in the episodic condition.The difference in log-discount rates between conditions is henceforth referred to as the episodic tag effect.Fitting hyperbolic functions to the median indifference points across subjects also showed reduced discounting in the episodic condition(discount rate control condition=0.0099, episodic condition=0.0077).The size of the tag effect was not related to the discount rate in the control condition(p=0.56). We next hypothesized that the tag effect would be positively correlated with postscan ratings of episodic thought(imagery scores,see above).Robust regression revealed an increase in the size of the tag effect with increasing imagery scores (t=2.08,p=0.023,see Figure2B),suggesting that the effect of the tags on preferences was stronger the more vividly subjects imagined the episodes.Examples of written postscan reports are provided in the Supplemental Results for participants from the entire range of imagination ratings.We also correlated the tag effect with standard neuropsychological measures,the Sensation Seeking Scale(SSS)V(Beauducel et al.,2003;Zuck-erman,1996)and the Behavioral Inhibition Scale/Behavioral Approach Scale(BIS/BAS)(Carver and White,1994).The tag effect was positively correlated with the experience-seeking subscale of the SSS(p=0.026)and inversely correlated with the reward-responsiveness subscale of the BIS/BAS scales (p<0.005).Repeated-measures ANOVA of reaction times(RTs)as a func-tion of option value(lower,similar,or higher relative to the refer-ence option;see Experimental Procedures and Figure2C)did not show a main effect of condition(p=0.712)or a condition 3value interaction(p=0.220),but revealed a main effect of value(F(1.8,53.9)=16.740,p<0.001).Post hoc comparisons revealed faster RTs for higher-valued options relative to similarly (p=0.002)or lower valued options(p<0.001)but no difference between lower and similarly valued options(p=0.081).FMRI DataFMRI data were modeled using the general linear model(GLM) as implemented in SPM5.Subjective value of each decision option was calculated by multiplying the objective amount of each delayed reward with the discount fraction estimated behaviorally based on the choices during scanning,and included as a parametric regressor in the GLM.Note that discount rates were estimated separately for the control and episodic conditions(see above and Figure2),and we thus used condition-specific k-parameters for calculation of the subjective value regressor.Additional parametric regressors for inverse delay-to-reward and absolute reward magnitude, orthogonalized with respect to subjective value,were included in theGLM.Figure2.Behavioral Data from Experiment1Shown are experimentally derived discount func-tions from the fMRI session for four exemplaryparticipants(A),correlation with imagery scores(B),and reaction times(RTs)(C).(A)Hyperbolicfunctions werefit to the indifference points sepa-rately for the control(dashed lines)and episodic(solid lines,filled circles)conditions,and thebest-fitting k-parameters(discount rates)and R2values are shown for each subject.The log-trans-formed difference between discount rates wastaken as a measure of the effect of the episodictags on choice preferences.(B)Robust regressionrevealed an association between log-differences indiscount rates and imagery scores obtained frompostscan ratings(see text).(C)RTs were signifi-cantly modulated by option value(main effectvalue p<0.001)with faster responses in trialswith a value of the delayed reward higher thanthe20V reference amount.Note that althoughseven delays were used for each condition,somedata points are missing,e.g.,onlyfive delay indif-ference points for the episodic condition areplotted for sub20.This indicates that,for the twolongest delays,this subject never chose the de-layed reward.***p<0.005.Error bars=SEM.Neuron Episodic Modulation of Delay Discounting140Neuron66,138–148,April15,2010ª2010Elsevier Inc.Episodic Tags Activate the Future Thinking NetworkWe first analyzed differences in the condition regressors without parametric pared to those of the control condi-tion,BOLD responses to the presentation of the delayed reward in the episodic condition yielded highly significant activations (corrected for whole-brain volume)in an extensive network of brain regions previously implicated in episodic future thinking (Addis et al.,2007;Schacter et al.,2007;Szpunar et al.,2007)(see Figure 3and Table S2),including retrosplenial cortex (RSC)/PCC (peak MNI coordinates:À6,À54,14,peak z value =6.26),left lateral parietal cortex (LPC,À44,À66,32,z value =5.35),and vmPFC (À8,34,À12,z value =5.50).Distributed Neural Coding of Subjective ValueWe then replicated previous findings (Kable and Glimcher,2007;Kable and Glimcher,2010;Peters and Bu¨chel,2009)using a conjunction analysis (Nichols et al.,2005)searching for regions showing a positive correlation between the height of the BOLD response and subjective value in the control and episodic condi-tions in a parametric analysis (Figure 4A and Table S3).Note that this is a conservative analysis that requires that a given voxel exceed the statistical threshold in both contrasts separately.This analysis revealed clusters in the lateral orbitofrontal cortex (OFC,À36,50,À10,z value =4.50)and central OFC (À18,12,À14,z value =4.05),bilateral VS (right:10,8,0,z value =4.22;left:À10,8,À6,z value =3.51),mPFC (6,26,16,z value =3.72),and PCC (À2,À28,24,z value =4.09),representing subjective (discounted)value in both conditions.We next analyzed the neural tag effect,i.e.,regions in which the subjective value correlation was greater for the episodic condi-tion as compared with the control condition (Figure 4B and Table S4).This analysis revealed clusters in the left LPC (À66,À42,32,z value =4.96,),ACC (À2,16,36,z value =4.76),left dorsolateral prefrontal cortex (DLPFC,À38,36,36,z value =4.81),and right amygdala (24,2,À24,z value =3.75).Finally,we performed a triple-conjunction analysis,testing for regions that were correlated with subjective value in both conditions,but in which the value correlation increased in the episodic condition.Only left LPC showed this pattern (À66,À42,30,z value =3.55,see Figure 4C and Table S5),the same region that we previously identified as delay-specific in valuation (Petersand Bu¨chel,2009).There were no regions in which the subjective value correlation was greater in the control condition when compared with the episodic condition at p <0.001uncorrected.ACC Valuation Signals and Functional Connectivity Predict Interindividual Differences in Discount Function ShiftsWe next correlated differences in the neural tag effect with inter-individual differences in the size of the behavioral tag effect.To this end,we performed a simple regression analysis in SPM5on the single-subject contrast images of the neural tag effect (i.e.,subjective value correlation episodic >control)using the behavioral tag effect [log(k control )–log(k episodic )]as an explana-tory variable.This analysis revealed clusters in the bilateral ACC (right:18,34,18,z value =3.95,p =0.021corrected,left:À20,34,20,z value =3.52,Figure 5,see Table S6for a complete list).Coronal sections (Figure 5C)clearly show that both ACC clusters are located in gray matter of the cingulate sulcus.Because ACC-limbic interactions have previously been impli-cated in the control of choice behavior (Floresco and Ghods-Sharifi,2007;Roiser et al.,2009),we next analyzed functional coupling with the right ACC from the above regression contrast (coordinates 18,34,18,see Figure 6A)using a psychophysiolog-ical interaction analysis (PPI)(Friston et al.,1997).Note that this analysis was conducted on a separate first-level GLM in which control and episodic trials were modeled as 10s miniblocks (see Experimental Procedures for details).We first identified regions in which coupling with the ACC changed in the episodic condition compared with the control condition (see Table S7)and then performed a simple regression analysis on these coupling parameters using the behavioral tag effect as an explanatory variable.The tag effect was associated with increased coupling between ACC and hippocampus (À32,À18,À16,z value =3.18,p =0.031corrected,Figure 6B)and ACC and left amygdala (À26,À4,À26,z value =2.95,p =0.051corrected,Figure 6B,see Table S8for a complete list of activa-tions).The same regression analysis in a second PPI with the seed voxel placed in the contralateral ACC region from the same regression contrast (À20,34,22,see above)yielded qual-itatively similar,though subthreshold,results in these same structures (hippocampus:À28,À32,À6,z value =1.96,amyg-dala:À28,À6,À16,z value =1.97).Experiment 2We conducted an additional behavioral experiment to address a number of alternative explanations for the observed effects of tags on choice behavior.First,it could be argued thatepisodicFigure 3.Categorical Effect of Episodic Tags on Brain ActivityGreater activity in lateral parietal cortex (left)and posterior cingulate/retrosplenial and ventro-medial prefrontal cortex (right)was observed in the episodic condition compared with the control condition.p <0.05,FWE-corrected for whole-brain volume.NeuronEpisodic Modulation of Delay DiscountingNeuron 66,138–148,April 15,2010ª2010Elsevier Inc.141tags increase subjective certainty that a reward would be forth-coming.In Experiment 2,we therefore collected postscan ratings of reward confidence.Second,it could be argued that events,always being associated with a particular date,may have shifted temporal focus from delay-based to more date-based processing.This would represent a potential confound,because date-associated rewards are discounted less than delay-associated rewards (Read et al.,2005).We therefore now collected postscan ratings of temporal focus (date-based versus delay-based).Finally,Experiment 1left open the question of whether the tag effect depends on the temporal specificity of the episodic cues.We therefore introduced an additional exper-imental condition that involved the presentation of subject-specific temporally unspecific future event cues.These tags (henceforth referred to as unspecific tags)were obtained by asking subjects to imagine events that could realistically happen to them in the next couple of months,but that were not directly tied to a particular point in time (see Experimental Procedures ).Episodic Imagery,Not Temporal Specificity,Reward Confidence,or Temporal Focus,Predicts the Size of the Tag EffectIn total,data from 16participants (9female)are included.Anal-ysis of pretest ratings confirmed that temporally unspecific and specific tags were matched in terms of personal relevance,arousal,valence,and preexisting associations (all p >0.15).Choice preferences were again well described by hyperbolic functions (median R 2control =0.84,unspecific =0.81,specific =0.80).We replicated the parametric tag effect (i.e.,increasing effect of tags on discount rates with increasing posttest imagery scores)in this independent sample for both temporally specific (p =0.047,Figure 7A)and temporally unspecific (p =0.022,Figure 7A)tags,showing that the effect depends on future thinking,rather than being specifically tied to the temporal spec-ificity of the event cues.Following testing,subjects rated how certain they were that a particular reward would actually be forth-coming.Overall,confidence in the payment procedure washighFigure 4.Neural Representation of Subjective Value (Parametric Analysis)(A)Regions in which the correlation with subjective value (parametric analysis)was significant in both the control and the episodic conditions (conjunction analysis)included central and lateral orbitofrontal cortex (OFC),bilateral ventral striatum (VS),medial prefrontal cortex (mPFC),and posterior cingulate cortex(PCC),replicating previous studies (Kable and Glimcher,2007;Peters and Bu¨chel,2009).(B)Regions in which the subjective value correlation was greater for the episodic compared with the control condition included lateral parietal cortex (LPC),ante-rior cingulate cortex (ACC),dorsolateral prefrontal cortex (DLPFC),and the right amygdala (Amy).(C)A conjunction analysis revealed that only LPC activity was positively correlated with subjective value in both conditions,but showed a greater regression slope in the episodic condition.No regions showed a better correlation with subjective value in the control condition.Error bars =SEM.All peaks are significant at p <0.001,uncorrected;(A)and (B)are thresholded at p <0.001uncorrected and (C)is thresholded at p <0.005,uncorrected for display purposes.NeuronEpisodic Modulation of Delay Discounting142Neuron 66,138–148,April 15,2010ª2010Elsevier Inc.(Figure 7B),and neither unspecific nor specific tags altered these subjective certainty estimates (one-way ANOVA:F (2,45)=0.113,p =0.894).Subjects also rated their temporal focus as either delay-based or date-based (see Experimental Procedures ),i.e.,whether they based their decisions on the delay-to-reward that was actually displayed,or whether they attempted to convert delays into the corresponding dates and then made their choices based on these dates.There was no overall significant effect of condition on temporal focus (one-way ANOVA:F (2,45)=1.485,p =0.237,Figure 7C),but a direct comparison between the control and the temporally specific condition showed a significant difference (t (15)=3.18,p =0.006).We there-fore correlated the differences in temporal focus ratings between conditions (control:unspecific and control:specific)with the respective tag effects (Figure 7D).There were no correlations (unspecific:p =0.71,specific:p =0.94),suggesting that the observed differences in discounting cannot be attributed to differences in temporal focus.High-Imagery,but Not Low-Imagery,Subjects Adjust Their Discount Function in an Episodic ContextFor a final analysis,we pooled the samples of Experiments 1and 2(n =46subjects in total),using only the temporally specific tag data from Experiment 2.We performed a median split into low-and high-imagery participants according to posttest imagery scores (low-imagery subjects:n =23[15/8Exp1/Exp2],imagery range =1.5–3.4,high-imagery subjects:n =23[15/8Exp1/Exp2],imagery range =3.5–5).The tag effect was significantly greater than 0in the high-imagery group (t (22)=2.6,p =0.0085,see Figure 7D),where subjects reduced their discount rate by onaverage 16%in the presence of episodic tags.In the low-imagery group,on the other hand,the tag effect was not different from zero (t (22)=0.573,p =0.286),yielding a significant group difference (t (44)=2.40,p =0.011).DISCUSSIONWe investigated the interactions between episodic future thought and intertemporal decision-making using behavioral testing and fMRI.Experiment 1shows that reward delay dis-counting is modulated by episodic future event cues,and the extent of this modulation is predicted by the degree of sponta-neous episodic imagery during decision-making,an effect that we replicated in Experiment 2(episodic tag effect).The neuroi-maging data (Experiment 1)highlight two mechanisms that support this effect:(1)valuation signals in the lateral ACC and (2)neural coupling between ACC and hippocampus/amygdala,both predicting the size of the tag effect.The size of the tag effect was directly related to posttest imagery scores,strongly suggesting that future thinking signifi-cantly contributed to this effect.Pooling subjects across both experiments revealed that high-imagery subjects reduced their discount rate by on average 16%in the episodic condition,whereas low-imagery subjects did not.Experiment 2addressed a number of alternative accounts for this effect.First,reward confidence was comparable for all conditions,arguing against the possibility that the tags may have somehow altered subjec-tive certainty that a reward would be forthcoming.Second,differences in temporal focus between conditions(date-basedFigure 5.Correlation between the Neural and Behavioral Tag Effect(A)Glass brain and (B and C)anatomical projection of the correlation between the neural tag effect (subjective value correlation episodic >control)and the behav-ioral tag effect (log difference between discount rates)in the bilateral ACC (p =0.021,FWE-corrected across an anatomical mask of bilateral ACC).(C)Coronal sections of the same contrast at a liberal threshold of p <0.01show that both left and right ACC clusters encompass gray matter of the cingulate gyrus.(D)Scatter-plot depicting the linear relationship between the neural and the behavioral tag effect in the right ACC.(A)and (B)are thresholded at p <0.001with 10contiguous voxels,whereas (C)is thresholded at p <0.01with 10contiguousvoxels.Figure 6.Results of the Psychophysiolog-ical Interaction Analysis(A)The seed for the psychophysiological interac-tion (PPI)analysis was placed in the right ACC (18,34,18).(B)The tag effect was associated with increased ACC-hippocampal coupling (p =0.031,corrected across bilateral hippocampus)and ACC-amyg-dala coupling (p =0.051,corrected across bilateral amygdala).Maps are thresholded at p <0.005,uncorrected for display purposes and projected onto the mean structural scan of all participants;HC,hippocampus;Amy,Amygdala;rACC,right anterior cingulate cortex.NeuronEpisodic Modulation of Delay DiscountingNeuron 66,138–148,April 15,2010ª2010Elsevier Inc.143。
湍流燃烧模型
Contents
1. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 195 2. Balance equations . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 196
194
D. Veynante, L. Vervisch / Progress in Energy and Combustion Science 28 (2002) 193±266
6. Tools for turbulent combustion modeling . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 212 6.1. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 212 6.2. Scalar dissipation rate . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 214 6.3. Geometrical description . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 214 6.3.1. G-®eld equation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 214 6.3.2. Flame surface density description . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 216 6.3.3. Flame wrinkling description . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 218 6.4. Statistical approaches: probability density function . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 218 6.4.1. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 218 6.4.2. Presumed probability density functions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 219 6.4.3. Pdf balance equation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 219 6.4.4. Joint velocity/concentrations pdf . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 221 6.4.5. Conditional moment closure (CMC) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 221 6.5. Similarities and links between the tools . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 221
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :a s t r o -p h /9906200v 1 11 J u n 1999Short-timescale Variability in the Broadband Emission of the Blazars Mkn421and Mkn501M.J.Carson 1,B.McKernan 1,T.Yaqoob 2,D.J.Fegan 11Department of Physics,University College Dublin,Dublin 4,Ireland2Laboratory for High Energy Astrophysics,Code 660.2Goddard Space Flight Center,Greenbelt,MD 20771AbstractWe analyse ASCA x-ray data and Whipple γ-ray data from the blazars Mkn421and Mkn501for short-timescale variability.We find no evidence for statistically significant (>3σ)variability in these data,in either source,on timescales of less than ∼10minutes.1IntroductionThe recently detected TeV radiation from the Blazars Mkn421and Mkn501may be Comptonized syn-chrotron radiation (Comastri et al.,1997)or Comptonized ambient radiation (Sikora et al.,1997)or some mix-ture of the two.Alternatively,the γ-radiation may result from a proton-initiated cascade (Beall &Bednarek,1998).Models of the γ-radiation preict large qualitative differences in some source parameters,so a search for short-timescales of variability in these AGN may be important in constraining models of emission and ultimately in distinguishing between them.Short timescale variability may also constrain quantum gravity effects predicted by some string theories (Amelino-Camelia et al.,1998).Variability studies (using the χ2-test)have been carried out on data from Mkn501and Mkn421.The shortest observed timescales of variability in TeV γ-rays correspond to doubling times in rapid flares of ∼15minutes for Mkn421(McEnery,1997)and ∼2hours for Mkn501(Quinn et al.,1999)which may indicate a variability cut-off or a lack of instrumental or method sensitivity to real variability at timescales shorter than these.The shortest timescales so far observed in these sources at x-ray energies are ∼1day for Mkn501(Kataoka et al.,1999)and 0.5-1.0days for Mkn421(Takahashi,Madejski &Kubo,1999).The analysis reported here involves a broadband variability study of Mkn501and Mkn421using the Excess Pair Fraction (EPF)technique (Yaqoob et al.,1997).We analyse non-simultaneous Whipple TeV γ-ray data and ASCA x-ray data from these blazars.2EPF applied to x-ray data ASCA (Tanaka et al.1994)observed Mkn 421and Mkn 501,obtaining moderate energy resolution spectra in the 0.5–10keV band,with a time-resolution of bet-ter than 2s for two of the four instruments onboard (4s for the other two).Mkn 421was observed on eigh-teen occassions between 1993,May 10and 1997,June 3,with a total exposure time of ∼240×103s.The 1996data do not overlap with the TeV data reported in this paper.Mkn 501was observed on four occassions between 1996,March 21and 1996,April 2,with a to-tal exposure time of ∼49×103s.Additional ASCA observations of both sources exist but only those madeof the dates mentioned above were in the public archive at the time of writing.An excellent account of the Mkn 421ACSA observations and results can be found in Taka-hashi,Madejski,and Kubo (1999)and the ASCA results for Mkn 501can be found in Kataoka et al.(1999).We re-analysed the ASCA data ourselves,which were re-Figure 1:EPF for ASCA x-ray data on Mkn421.duced in the same manner as described in Yaqoob et al.(1998).We made EPF for each source following the methods described in Yaqoob et al.(1997),averagedThe results for both sources are shown in Figs.show theoretical EPF for a source with 0%,5%,and10%random amplitude variability respectively.The curves were generated as described in detail in Yaqoob et al.1997.It can be seen that in Mkn 421we can rule out variability at the 5%level with a confidencelevel greater than 3σfrom ∼500s down to timescalesof ∼11s.(Note:The kink at 32s is due to only two of the four ASCA instruments being used below this timescale because the two Solid State Spectrome-ters have less timing resolution than the two Gas Imag-ing Proportional Counters).For Mkn 501,for whichmuch less data are available,we can rule out variabil-ity at the 10%level with a confidence level greater than 3σfrom ∼400s down to timescales of ∼32s.Figure 2:Open circles show the Excess Pair Fraction (EPF)computed from all the ASCA Mkn 501observations (see text).3EPF applied to γ-ray data TeV data are recorded by the Whipple ˆIACT uses the well-established Extensive Air (EAS)technique to indirectly observe very high ergy γ-rays from sources such as AGN jets and nova remnants (Cawley et al.,1990).Most showers are due to the collision of high energy mic rays (hadrons or leptons),therefore the ray data,as observed by the IACT,have a very signal to noise ratio.Parameter cuts are used to inate most of the noise whilst retaining at least of the signal.The TeV data are typically ∼28minute intervals,at different elevations and various weather conditions.Only those data sets the most stable raw count rates were used.The data were taken from the period April 1996-May during which time the most significant flaring event ever seen at this energy was observed.The Mkn501data were taken from the period April 1997-May 1997,dur-Figure 3:Open circles show EPF for Whipple Mkn421data.The solid line is the theoretical EPF expected from a constant source and dashed lines are the theoretical EPF for 5%and 10%random amplitude variability.ing which time this AGN was at its most active since its discovery at this energy in 1995.The presence of strong flaring within these data is important for statistical purposes as the count rate from the AGN increases from typically ≤1γmin −1to ∼10γmin −1during a strong flaring state.Fig.(3)shows EPF for Mkn421.Approximately 1760minutes of data are used in this analysis at TeV en-ergies.None of the data points are greater than3σthat of a theoretical constant source with the samecount rate.Because of the28minute data binningtimescales less than14minutes can be consideredthis technique.Theγ-ray rate(∼3γmin−1)us probing timescales much lower than∼200Fig.(4)shows EPF for Mkn501with1900minutes of data.Again none of the dataare greater than3σfrom that of a constant sourcethe same mean count rate indicating a lack ofvariability in this source below∼10mins.Here theis∼9min−1and so the lower timescale belowthe statistics become too poor is∼100seconds.we can rule out variability in both sources belowminutes at a confidence level>3σat the10%variabil-Figure4:EPF for Whipple Mkn501data.ity level.4Discussion and ConclusionsOur results thus far show that there appears to be no statistically significant variability in either of the two AGN,below timescales of∼600s.From these results we conclude that either the statistics of the present data are insufficient to establish significant variability or the data does not vary on these timescales.If we assume that the source is not varying on timescales shorter than10minutes then we can estimate the minimum size of the emission region:the Doppler beaming factor is given by D=1/(1+z)Γb(1−βcosθ)where z is the redshift of the source,Γb is the Lorentz boost,β=v′/c(where v′is the velocity of the emitting region)and θis the angle between the jet axis and the observer.Causal arguments require that the size of the emission region R em is constrained to satisfyDR em≤cδtYaqoob,T.,et al.1997,ApJ490,L25 Yaqoob,T.,et al.1998,ApJ505,L87。
