南开大学光学工程内部课件Nov 9th
合集下载
光学-课件全集
a
n1
i1
a1
D B
n2
A
i2
n1 C
a2
d
由折射定律和几何关系可得出:
n1siin 1n2siin 2
AD AB siin1
AC C Bd/coi2s AB2dtain2
1、非相干叠加 独立光源的两束光或同一光源的不同部位所发出 的光的位相差“瞬息万变”
1
0
cosdt0
II1I2
叠加后光强等与两光束单独照射时的光强之和,
无干涉现象
2、相干叠加 满足相干条件的两束光叠加后
II1I22I1I2co s
位相差恒定,有干涉现象
若 I1 I2
§1.1.5 相干与不相干叠加
矢量合成方法
x1A 1cots (1)0
x 2 A 2 co t s 2) ( 0
A
AA 12A2 22A 1A2co2s0 (1)0
A2
y2
y2
y
0arcA A 1 1tc sa io1 1 n n s0 0 A A 2 2c sio2 n 2s 0 0O
3、光强 光波是电磁波。 光波中参与与物质相互作用(感光作用、生理
作用)的是 A 矢量,称为光矢量。 A 矢量的振动称为光振动。
光强:在光学中,通常把平均能流密度称为光强, 用 I 表示。
I A2
机械波的独立性和叠加性
发生干涉的条件: 1、频率相同 2、观察时间内波动不中断 3、相遇出振动方向几乎在同一直线上 干涉现行的特性:
2d
k0,1,2…
干涉加强
干涉减弱
明纹位置
暗纹位置
两相邻明(或暗)条纹间的距离称为条纹间距。
南开大学光学工程内部课件Oct 19th

E1 (r , t ) E01 exp i(k1 r t 1 ) E2 (r , t ) E02 exp i(k2 r t 2 ).
Two plane waves meet at P
Superposition of two beams
The transmitted light is incident onto a screen containing two narrow slits
Young’s Double Slit Experiment
The symmetric narrow slits, S1 and S2 act as the two light sources The waves from the two slits come from the same source S0 and therefore are always in phase.
m
= 0, ±1, ±2, …
Interference Equations
Y:measured vertically from the zeroth order maximum Assumptions
L >>d,
d >>λ
y =LtanθLsinθ
I I1 I 2 2 I1I 2 cos(kd sin ) I1 I 2 2 I1I 2 cos(kdy / L)
Other Coherent Sources
Currently, it is much more common to use a laser as a coherent source The laser produces an intense, coherent,
南开大学光学工程内部课件Nov-16th
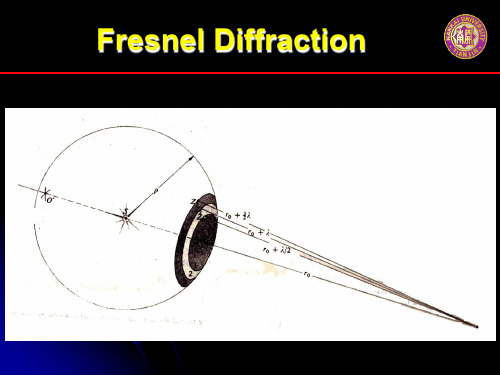
If the primary wave was simply to propagate
from S to P, it is
E
0
e i[t k ( r0 )]
r0
The two equations must be exactly the same. So we introduce a /2 phase difference between the primary wave and the secondary wave to make the two equations so.
dS d 2 ( sin )
Fresnel Dif2 2( r0 )cos
So
dS 2 rdr. Constant!!! r0
We have
El
(1)l 1
2Kl A r0
equation (a) becomes
E E1 Em
2
2
Fresnel Diffraction
From equation (b) we have
E
E1
E2 2
E m 1 2
Em
Since K() goes from 1 to 0 over a great many zones, we can neglect any variation between adjacent zones, i.e. │E1│= │E2│, │Em-1│= │Em│. So
Em 2
)
Em 2
(a)
or
E
E1
E2 2
(
E2 2
E3
E4 2
南开大学光学工程内部课件Lecture 2

—— Range Instrumentation prism, right.
BM 60 90 right
Ray Tracing
Reflection from Flat Surface
—— Range Instrumentation prism, left, roof.
BM 100 90 left roof
CR 180 roof
Ray Tracing
Reflection from Flat Surface
—— Rhomb prism. It has two reflective faces 斜方棱镜,又名菱形棱镜
BC 0 (Rhomb)
Ray Tracing
Reflection from Flat Surface
Reflection from Flat Surface
—— Isosceles prism, three reflective faces, roof
CR 45 roof (Schmidt)
Ray Tracing
Reflection from Flat Surface
—— Isosceles prism, three reflective faces, roof
Reflection Prism
—— Isosceles prism (Classification code: R), single reflective face. 等腰棱镜(代号:D), 一次反射型
The Dove Prism (AR45)
Ray Tracing
Reflection from Flat Surface
• In fact, if the UV and IR are included, most any substance will sow some absorption. So anomalous dispersion exist somewhere throughout the spectrum
BM 60 90 right
Ray Tracing
Reflection from Flat Surface
—— Range Instrumentation prism, left, roof.
BM 100 90 left roof
CR 180 roof
Ray Tracing
Reflection from Flat Surface
—— Rhomb prism. It has two reflective faces 斜方棱镜,又名菱形棱镜
BC 0 (Rhomb)
Ray Tracing
Reflection from Flat Surface
Reflection from Flat Surface
—— Isosceles prism, three reflective faces, roof
CR 45 roof (Schmidt)
Ray Tracing
Reflection from Flat Surface
—— Isosceles prism, three reflective faces, roof
Reflection Prism
—— Isosceles prism (Classification code: R), single reflective face. 等腰棱镜(代号:D), 一次反射型
The Dove Prism (AR45)
Ray Tracing
Reflection from Flat Surface
• In fact, if the UV and IR are included, most any substance will sow some absorption. So anomalous dispersion exist somewhere throughout the spectrum
南开大学光学——绪论

常胜江 办公地点:伯苓楼3区303
电话:23504571
上页
下页
Nankai University
光在各向同性介质界面上的 反射和折射
晶体的光学性质 光与物质的相互作用
下页
CHANG Sheng Jiang, Institute of Modern Optics, Nankai University
主要参考书:
1. 新概念物理教程-光学,赵凯华; ----高等教育出版社, 2. 光学 (上下册), 赵凯华,钟锡华 ; ----北京大学出版社; 3. 光学, 母国光等. ----高等教育出版社;
2
光与物质相互作用的性质及特点
1. 线性作用过程:
-----非强光光场的线性叠加等;
2. 非线性作用过程: -----强激光与大气相互作用过程;
上页
下页
CHANG Sheng Jiang, Institute of Modern Optics, Nankai University Nankai University
认为光是纵波,是一种在弹性媒质中传播的机械波;
------成功解释了光的反射和折射等现象;
下页
上页
CHANG Sheng Jiang, Institute of Modern Optics, Nankai University
2. 托马斯 杨 和菲涅尔 (19世纪初) :
发现并解释了干涉、衍射现象,把波动理论大大推 向前进; -----通过偏振现象确认光是一种横波; -----初步测定了波长;
绪
论
光学课程需要回答的问题:
1 2 3
光的本质是什么? 光与物质相互作用的性质及特点 光学能做什么?
上页
《光学基本知识讲座》课件

光学在军事中的应用
总结词
光学技术在军事侦察和武器系统中的应用
详细描述
光学技术在军事领域的应用包括红外侦察、 激光雷达、瞄准和测距等。这些技术提高了 军事侦察和武器系统的精度和效率,对现代
战争的胜负具有关键作用。
04
光学发展历程
光学发展史简介
古代光学
古代文明对光的研究和利用,如反射、折射等简单光 学现象的发现和应用。
全息摄影技术
总结词
全息摄影原理及应用
详细描述
全息摄影技术利用光的干涉和衍射原理,记 录并重现三维物体的光波信息。全息照片具 有立体感和视角任选的特性,广泛应用于产 品展示、艺术创作和安全识别等领域。
光学在医学中的应用
总结词
光学在医学诊断和治疗中的应用
详细描述
光学技术在医学领域具有广泛的应用 ,如光学显微镜用于细胞观察,激光 用于手术切割和眼科治疗,以及光学 成像技术用于无创检测和诊断。
文艺复兴时期
科学方法的兴起,对光的本质和传播方式的研究逐渐 深入。
19世纪
光学理论体系逐渐完善,如波动光学和几何光学的发 展。
光学重大发明和发现
01
02
03
牛顿的棱镜实验
揭示了白光是由不同颜色 的光组成,奠定了光谱学 的基础。
干涉现象的发现
为波动光学的建立提供了 重要依据。
激光的发明
开创了光学的新领域,对 科技、工业、医疗等领域 产生了深远影响。
实验材料
光源、衍射板、屏幕等 。
Hale Waihona Puke 实验步骤将光源对准衍射板中心 ,调整光源与衍射板距 离;观察衍射现象并记
录。
注意事项
注意保护眼睛,避免直 接照射光源;调整仪器
南开大学光学工程内部课件Sep_16th
Refraction at curved surface
Similar, the second or image focus is the axial point Fi where the image is formed when S0= . And the second or image focal length fi as equal to Si in the special case, we have
Why are focusing instruments necessary?
Refraction at curved surface
Imaging In order to image S at location P, the time it takes for each and every portion of a wavefront leaving S to converge at point P must be identical. So:
which followed with
n1 n2 1 n2 S i n1 S0 ( ) SM 2 MS ' R MS ' SM
Refraction at curved surface
Discussion Sign convention for spherical refraction surfaces and thin lenses
Refraction at curved surface
Fermat’s Principle maintains that the optical path length (OPLSS’) will be stationary (实际上,物与像之间根据费马原 理具有等光程性), i.e.:
南开大学光学工程内部课件Sep 28th
The objective lens has a short focal length, ƒo<1 cm The ocular lens (eyepiece) has a focal length, ƒe, of several cm L>> ƒo and ƒe
Compound Microscope
The image seen by the eye, I2, is virtual, inverted and very much enlarged
The magnification of the microscope is the product of the magnifications of the objective and the ocular lens
Normal
vision has a far point of infinity
Farsightedness
Also called hyperopia The image focuses behind the retina Can usually see far away objects clearly, but not nearby objects
m o
angle with lens angle without lens
Simple Magnifier
The angular magnification is at a maximum when the image formed by the lens is at the near point of the eye
Presbyopia and Astigmatism
Diopters
Compound Microscope
The image seen by the eye, I2, is virtual, inverted and very much enlarged
The magnification of the microscope is the product of the magnifications of the objective and the ocular lens
Normal
vision has a far point of infinity
Farsightedness
Also called hyperopia The image focuses behind the retina Can usually see far away objects clearly, but not nearby objects
m o
angle with lens angle without lens
Simple Magnifier
The angular magnification is at a maximum when the image formed by the lens is at the near point of the eye
Presbyopia and Astigmatism
Diopters
大学光学经典课件L1_绪论精品文档48页
在不同I 媒2 c n 质0 T 中0 T 有E 0 2 ( :1 II12c o s( nn2 21( EE002212t)))d t 2 c n0E 0 2
在相同介质中有:I nE02
4)相对光强:
I E02
注意:
光强是一个平均值
I
S
n
2c0
E02
5)光强定义为一个平均值的原因
响应时间:能够被感知或被记录所需的最短时间 人眼的响应时间:t0.1s 最好的仪器的响应时间大约: 109s 光波的振动周期:T1015s
学好光学课的重要意义
当今科研前沿的热门学科 光学课程是众多光学方面课程的基础启蒙课程
如:激光原理与技术,量子光学,信息学光纤 光学,集成光学,光谱学,光子开关术全息光 存储技术,光纤通信技术原理,非线性光学, 晶体光学,原子光学,光电信号检测技术等
光学课的特点
内容新:中学学得不多,光学发展很快,新 内容不断涌现
nc/
故
S 0 nE2 n E2
0
c0
真空中电磁波的波动方程: EE0cos(t)
可得:E 2 E 0 2 c o s 2 (t) 1 2 E 0 2 ( 1 c o s (2 (t)))
I S T 10 T c n 0E 2 d t T 10 T c n 0E 0 2c o s 2 (t)d t
tT
人眼和接收器只能感知光波的平均能流密度 有实际意义的是光波的平均能流
三、光 谱
1)单色光:仅有单一波长的光叫单色光,否则 是非单色光。
2)谱密度: d I~d i() dI
d
3)光谱:谱密度随波长变化的分布曲线
I
d
I
i()d
0
在相同介质中有:I nE02
4)相对光强:
I E02
注意:
光强是一个平均值
I
S
n
2c0
E02
5)光强定义为一个平均值的原因
响应时间:能够被感知或被记录所需的最短时间 人眼的响应时间:t0.1s 最好的仪器的响应时间大约: 109s 光波的振动周期:T1015s
学好光学课的重要意义
当今科研前沿的热门学科 光学课程是众多光学方面课程的基础启蒙课程
如:激光原理与技术,量子光学,信息学光纤 光学,集成光学,光谱学,光子开关术全息光 存储技术,光纤通信技术原理,非线性光学, 晶体光学,原子光学,光电信号检测技术等
光学课的特点
内容新:中学学得不多,光学发展很快,新 内容不断涌现
nc/
故
S 0 nE2 n E2
0
c0
真空中电磁波的波动方程: EE0cos(t)
可得:E 2 E 0 2 c o s 2 (t) 1 2 E 0 2 ( 1 c o s (2 (t)))
I S T 10 T c n 0E 2 d t T 10 T c n 0E 0 2c o s 2 (t)d t
tT
人眼和接收器只能感知光波的平均能流密度 有实际意义的是光波的平均能流
三、光 谱
1)单色光:仅有单一波长的光叫单色光,否则 是非单色光。
2)谱密度: d I~d i() dI
d
3)光谱:谱密度随波长变化的分布曲线
I
d
I
i()d
0
南开大学光学工程内部课件Oct 28th
Need Q ~ 105 or greater Led to super mirrors
polished to Angstroms ion beam machining polished to ~ 100 nanometers limited by grit size
Laser gyro developed for aircraft
tCCW = 8R 2c R
Travel time cw
tCW = 8R 2c - R
Time difference
Dt 4 A c2
Number of fringes
DN = 4 A cl
Fringe shift ~ 4 % for 2 rev/sec
Laser gyro
Interferometers
Interferometer
What is interferometer?
Interferometer is the optical setup which split incident light into two beams and then recombines them to create an interference fringe.
N=2L/ l =2L/ l
Phase change (in terms of wavelengths):
DN=Nm - N=2Ln/l- 2L/l= 2L/l (n-1)
The Michelson-Morley Experiment
1881
White light fringes
Adjust the mirrors to make the two path has the same path length, one can see white light fringes.
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Its reduced form is
E bc(
sin
)(
sin N
)e
i [t kR ( N 1 ) ]
The flux-density distribution function is
I ( ) I 0 (
sin
) (
2
sin N
)
2
Fraunhofer Diffraction
Fraunhofer Diffraction
The Double Slit
Two long slits of width b and center-to-center separation a
Fraunhofer Diffraction
Analysis Each aperture, by itself, would generate the same single-slit diffraction pattern on the screen . At any point on , the contribution from the two slits overlap and each must be equal in amplitude and different in phase. The density distribution should be a combination of a rapidly varying double-slit interference system, modulated by a singleslit diffraction pattern.
I ( ) / I ( 0) 1.0 0.047 0.017 0.008 ......
Fraunhofer Diffraction
3. If the source emits whit light, only in the region about =0 will all of the constituent colors overlap to yield White light.
Fraunhofer Diffraction
Analysis According to the Huygens-Fresnel principle, a differential area dS, within the aperture, may be envisioned as being covered with coherent secondary point sources. But dS is much smaller in extent than , so that all of the contributions at P remain in phase and interfere constructively.
Fraunhofer Diffraction
Slit Pattern Diffraction Pattern
Diffraction from multiple slits
Infinitely many equally spaced slits (a Shah function!) yields a far-field pattern that’s the Fourier transform, that is, the Shah function.
a b / 2
E ( z )dz
Fraunhofer Diffraction
Where c is the source intensity per unit length along the z-axis and E=exp(it-k ( R-z sin )). Integration of the formula yields
E bc(
sin
)( e
i (t kR )
e
i (t kR 2 )
)
with =(ka/2)sin, = (kb/2)sin. 2 and 2 are the phase difference between two nearly parallel rays arriving at a point P on , from the centers of two slits and from the edges of one of the slits, respectively.
Fraunhofer Diffraction
Basic model (or question) —— point-source array
E0 ( r0 ) E0 ( r )e
i ( kRt )
sin N / 2 ( ) sin / 2
sin 2 [ N ( kd / 2) sin ] I I0 sin 2 [( kd / 2) sin ]
Fraunhofer Diffraction
It may occur that an interference maximum and a diffraction minimum correspond to the same -value, in this case no light is available at the precise position to partake in the interference process, and the suppressed peak is said to be missing order
Fraunhofer Diffraction
The linear source
L D sin i (t kR) E e R
1 L D 2 sin 2 I ( ) ( ) ( ) 2 R I (0)sinc
2
(kD / 2) sin
Fraunhofer Diffraction
Fraunhofer Diffraction
3. Minima of zero flux-density Exist whenever (sinN/sin)=0 or when
2 ( N 1) ( N 1) , ,..., , ,... N N N N
Between consecutive principal maxima (i.e., over the range in of ), there will be N-1 Minima.
Fraunhofer ห้องสมุดไป่ตู้iffraction
a=3b
kx1/z
Fraunhofer Diffraction
a=3b
Fraunhofer Diffraction
Diffraction by many slits
N long, parallel, narrow slits each of width b and center-to-center separation a.
E j bc
Here,
sin
exp[i (t - kR 2 j )]
ka sin 2
kb sin 2 So we have
E E j bc
j
sin
e i (t kR ) (e i 2 ) j
j 0
N 1
Fraunhofer Diffraction
Discussion 1. The flux density in the =0 direction I(0) =N2I0
2. Principal maxima Occur when (sinN/sin)=N. i.e. when = 0, , 2, …… or equivalently asinm=m m= 0, 1, 2, ……
One slit
Two slits
Fraunhofer Diffraction
Fraunhofer Diffraction
Discussion 1. The =0 direction = = 0 I(0) = 4 I0
2. b gets vanishingly small In this case, kb<<1, then sin/=1. The equation of I() reduce to the flux-density expression for a pair of long line sources, i.e. Young’s experiment.
Fraunhofer Diffraction
With the coordination system at the center of the first slit, the total optical disturbance at a point on the screen is given by
Fraunhofer Diffraction
Solution The total contribution to the electric field, in the Fraunhofer approximation, is then
E c
b/ 2
b / 2
E ( z )dz c
a b / 2
Fraunhofer Diffraction
4. Subsidiary maxima Located approximately at points where sinN has its greatest value, namely
3 5 , ,... 2N 2N
There are N-2 subsidiary maxima between consecutive principal maxima.
F ( z ) exp{i[t k ( R sin )]}
E bc(
sin
)(
sin N
)e
i [t kR ( N 1 ) ]
The flux-density distribution function is
I ( ) I 0 (
sin
) (
2
sin N
)
2
Fraunhofer Diffraction
Fraunhofer Diffraction
The Double Slit
Two long slits of width b and center-to-center separation a
Fraunhofer Diffraction
Analysis Each aperture, by itself, would generate the same single-slit diffraction pattern on the screen . At any point on , the contribution from the two slits overlap and each must be equal in amplitude and different in phase. The density distribution should be a combination of a rapidly varying double-slit interference system, modulated by a singleslit diffraction pattern.
I ( ) / I ( 0) 1.0 0.047 0.017 0.008 ......
Fraunhofer Diffraction
3. If the source emits whit light, only in the region about =0 will all of the constituent colors overlap to yield White light.
Fraunhofer Diffraction
Analysis According to the Huygens-Fresnel principle, a differential area dS, within the aperture, may be envisioned as being covered with coherent secondary point sources. But dS is much smaller in extent than , so that all of the contributions at P remain in phase and interfere constructively.
Fraunhofer Diffraction
Slit Pattern Diffraction Pattern
Diffraction from multiple slits
Infinitely many equally spaced slits (a Shah function!) yields a far-field pattern that’s the Fourier transform, that is, the Shah function.
a b / 2
E ( z )dz
Fraunhofer Diffraction
Where c is the source intensity per unit length along the z-axis and E=exp(it-k ( R-z sin )). Integration of the formula yields
E bc(
sin
)( e
i (t kR )
e
i (t kR 2 )
)
with =(ka/2)sin, = (kb/2)sin. 2 and 2 are the phase difference between two nearly parallel rays arriving at a point P on , from the centers of two slits and from the edges of one of the slits, respectively.
Fraunhofer Diffraction
Basic model (or question) —— point-source array
E0 ( r0 ) E0 ( r )e
i ( kRt )
sin N / 2 ( ) sin / 2
sin 2 [ N ( kd / 2) sin ] I I0 sin 2 [( kd / 2) sin ]
Fraunhofer Diffraction
It may occur that an interference maximum and a diffraction minimum correspond to the same -value, in this case no light is available at the precise position to partake in the interference process, and the suppressed peak is said to be missing order
Fraunhofer Diffraction
The linear source
L D sin i (t kR) E e R
1 L D 2 sin 2 I ( ) ( ) ( ) 2 R I (0)sinc
2
(kD / 2) sin
Fraunhofer Diffraction
Fraunhofer Diffraction
3. Minima of zero flux-density Exist whenever (sinN/sin)=0 or when
2 ( N 1) ( N 1) , ,..., , ,... N N N N
Between consecutive principal maxima (i.e., over the range in of ), there will be N-1 Minima.
Fraunhofer ห้องสมุดไป่ตู้iffraction
a=3b
kx1/z
Fraunhofer Diffraction
a=3b
Fraunhofer Diffraction
Diffraction by many slits
N long, parallel, narrow slits each of width b and center-to-center separation a.
E j bc
Here,
sin
exp[i (t - kR 2 j )]
ka sin 2
kb sin 2 So we have
E E j bc
j
sin
e i (t kR ) (e i 2 ) j
j 0
N 1
Fraunhofer Diffraction
Discussion 1. The flux density in the =0 direction I(0) =N2I0
2. Principal maxima Occur when (sinN/sin)=N. i.e. when = 0, , 2, …… or equivalently asinm=m m= 0, 1, 2, ……
One slit
Two slits
Fraunhofer Diffraction
Fraunhofer Diffraction
Discussion 1. The =0 direction = = 0 I(0) = 4 I0
2. b gets vanishingly small In this case, kb<<1, then sin/=1. The equation of I() reduce to the flux-density expression for a pair of long line sources, i.e. Young’s experiment.
Fraunhofer Diffraction
With the coordination system at the center of the first slit, the total optical disturbance at a point on the screen is given by
Fraunhofer Diffraction
Solution The total contribution to the electric field, in the Fraunhofer approximation, is then
E c
b/ 2
b / 2
E ( z )dz c
a b / 2
Fraunhofer Diffraction
4. Subsidiary maxima Located approximately at points where sinN has its greatest value, namely
3 5 , ,... 2N 2N
There are N-2 subsidiary maxima between consecutive principal maxima.
F ( z ) exp{i[t k ( R sin )]}
